|
Spread (projective Geometry)
A frequently studied problem in discrete geometry is to identify ways in which an object can be covered by other simpler objects such as points, lines, and planes. In projective geometry, a specific instance of this problem that has numerous applications is determining whether, and how, a projective space can be covered by pairwise disjoint subspaces which have the same dimension; such a partition is called a spread. Specifically, a spread of a projective space PG(d,K), where d \geq 1 is an integer and K a division ring, is a set of r-dimensional subspaces, for some 0 < r < d such that every point of the space lies in exactly one of the elements of the spread. Spreads are particularly well-studied in projective geometries over finite fields, though some notable results apply to infinite projective geometries as well. In the finite case, the foundational work on spreads appears in André and independently in Bruck-Bose in connection wi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Discrete Geometry
Discrete geometry and combinatorial geometry are branches of geometry that study combinatorial properties and constructive methods of discrete geometric objects. Most questions in discrete geometry involve finite or discrete sets of basic geometric objects, such as points, lines, planes, circles, spheres, polygons, and so forth. The subject focuses on the combinatorial properties of these objects, such as how they intersect one another, or how they may be arranged to cover a larger object. Discrete geometry has a large overlap with convex geometry and computational geometry, and is closely related to subjects such as finite geometry, combinatorial optimization, digital geometry, discrete differential geometry, geometric graph theory, toric geometry, and combinatorial topology. History Although polyhedra and tessellations had been studied for many years by people such as Kepler and Cauchy, modern discrete geometry has its origins in the late 19th century. Early ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linear Map
In mathematics, and more specifically in linear algebra, a linear map (also called a linear mapping, linear transformation, vector space homomorphism, or in some contexts linear function) is a mapping V \to W between two vector spaces that preserves the operations of vector addition and scalar multiplication. The same names and the same definition are also used for the more general case of modules over a ring; see Module homomorphism. If a linear map is a bijection then it is called a . In the case where V = W, a linear map is called a (linear) '' endomorphism''. Sometimes the term refers to this case, but the term "linear operator" can have different meanings for different conventions: for example, it can be used to emphasize that V and W are real vector spaces (not necessarily with V = W), or it can be used to emphasize that V is a function space, which is a common convention in functional analysis. Sometimes the term ''linear function'' has the same meaning as ''lin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Quadratic Form
In mathematics, a quadratic form is a polynomial with terms all of degree two ("form" is another name for a homogeneous polynomial). For example, :4x^2 + 2xy - 3y^2 is a quadratic form in the variables and . The coefficients usually belong to a fixed field , such as the real or complex numbers, and one speaks of a quadratic form over . If K=\mathbb R, and the quadratic form takes zero only when all variables are simultaneously zero, then it is a definite quadratic form, otherwise it is an isotropic quadratic form. Quadratic forms occupy a central place in various branches of mathematics, including number theory, linear algebra, group theory (orthogonal group), differential geometry ( Riemannian metric, second fundamental form), differential topology ( intersection forms of four-manifolds), and Lie theory (the Killing form). Quadratic forms are not to be confused with a quadratic equation, which has only one variable and includes terms of degree two or less. A quadra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Sesquilinear Form
In mathematics, a sesquilinear form is a generalization of a bilinear form that, in turn, is a generalization of the concept of the dot product of Euclidean space. A bilinear form is linear in each of its arguments, but a sesquilinear form allows one of the arguments to be "twisted" in a semilinear manner, thus the name; which originates from the Latin numerical prefix ''sesqui-'' meaning "one and a half". The basic concept of the dot product – producing a scalar from a pair of vectors – can be generalized by allowing a broader range of scalar values and, perhaps simultaneously, by widening the definition of a vector. A motivating special case is a sesquilinear form on a complex vector space, . This is a map that is linear in one argument and "twists" the linearity of the other argument by complex conjugation (referred to as being antilinear in the other argument). This case arises naturally in mathematical physics applications. Another important case allows the scalars ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Isotropic Quadratic Form
In mathematics, a quadratic form over a field ''F'' is said to be isotropic if there is a non-zero vector on which the form evaluates to zero. Otherwise the quadratic form is anisotropic. More precisely, if ''q'' is a quadratic form on a vector space ''V'' over ''F'', then a non-zero vector ''v'' in ''V'' is said to be isotropic if . A quadratic form is isotropic if and only if there exists a non-zero isotropic vector (or null vector) for that quadratic form. Suppose that is quadratic space and ''W'' is a subspace of ''V''. Then ''W'' is called an isotropic subspace of ''V'' if ''some'' vector in it is isotropic, a totally isotropic subspace if ''all'' vectors in it are isotropic, and an anisotropic subspace if it does not contain ''any'' (non-zero) isotropic vectors. The of a quadratic space is the maximum of the dimensions of the totally isotropic subspaces. A quadratic form ''q'' on a finite-dimensional real vector space ''V'' is anisotropic if and only if ''q'' is a d ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polar Space
In mathematics, in the field of geometry, a polar space of rank ''n'' (), or ''projective index'' , consists of a set ''P'', conventionally called the set of points, together with certain subsets of ''P'', called ''subspaces'', that satisfy these axioms: * Every subspace is isomorphic to a projective geometry with and ''K'' a division ring. By definition, for each subspace the corresponding ''d'' is its dimension. * The intersection of two subspaces is always a subspace. * For each point ''p'' not in a subspace ''A'' of dimension of , there is a unique subspace ''B'' of dimension containing ''p'' and such that is -dimensional. The points in are exactly the points of ''A'' that are in a common subspace of dimension 1 with ''p''. * There are at least two disjoint subspaces of dimension . It is possible to define and study a slightly bigger class of objects using only relationship between points and lines: a polar space is a partial linear space (''P'',''L''), so that for eac ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Pencil (mathematics)
In geometry, a pencil is a family of geometric objects with a common property, for example the set of lines that pass through a given point in a plane, or the set of circles that pass through two given points in a plane. Although the definition of a pencil is rather vague, the common characteristic is that the pencil is completely determined by any two of its members. Analogously, a set of geometric objects that are determined by any three of its members is called a bundle. Thus, the set of all lines through a point in three-space is a bundle of lines, any two of which determine a pencil of lines. To emphasize the two dimensional nature of such a pencil, it is sometimes referred to as a ''flat pencil''. Any geometric object can be used in a pencil. The common ones are lines, planes, circles, conics, spheres, and general curves. Even points can be used. A pencil of points is the set of all points on a given line. A more common term for this set is a ''range'' of points. Pencil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Klein Quadric
In mathematics, the lines of a 3-dimensional projective space, ''S'', can be viewed as points of a 5-dimensional projective space, ''T''. In that 5-space, the points that represent each line in ''S'' lie on a quadric, ''Q'' known as the Klein quadric. If the underlying vector space of ''S'' is the 4-dimensional vector space ''V'', then ''T'' has as the underlying vector space the 6-dimensional exterior square Λ2''V'' of ''V''. The line coordinates obtained this way are known as Plücker coordinates. These Plücker coordinates satisfy the quadratic relation : p_ p_+p_p_+p_ p_ = 0 defining ''Q'', where : p_ = u_i v_j - u_j v_i are the coordinates of the line spanned by the two vectors ''u'' and ''v''. The 3-space, ''S'', can be reconstructed again from the quadric, ''Q'': the planes contained in ''Q'' fall into two equivalence classes, where planes in the same class meet in a point, and planes in different classes meet in a line or in the empty set. Let these classes be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
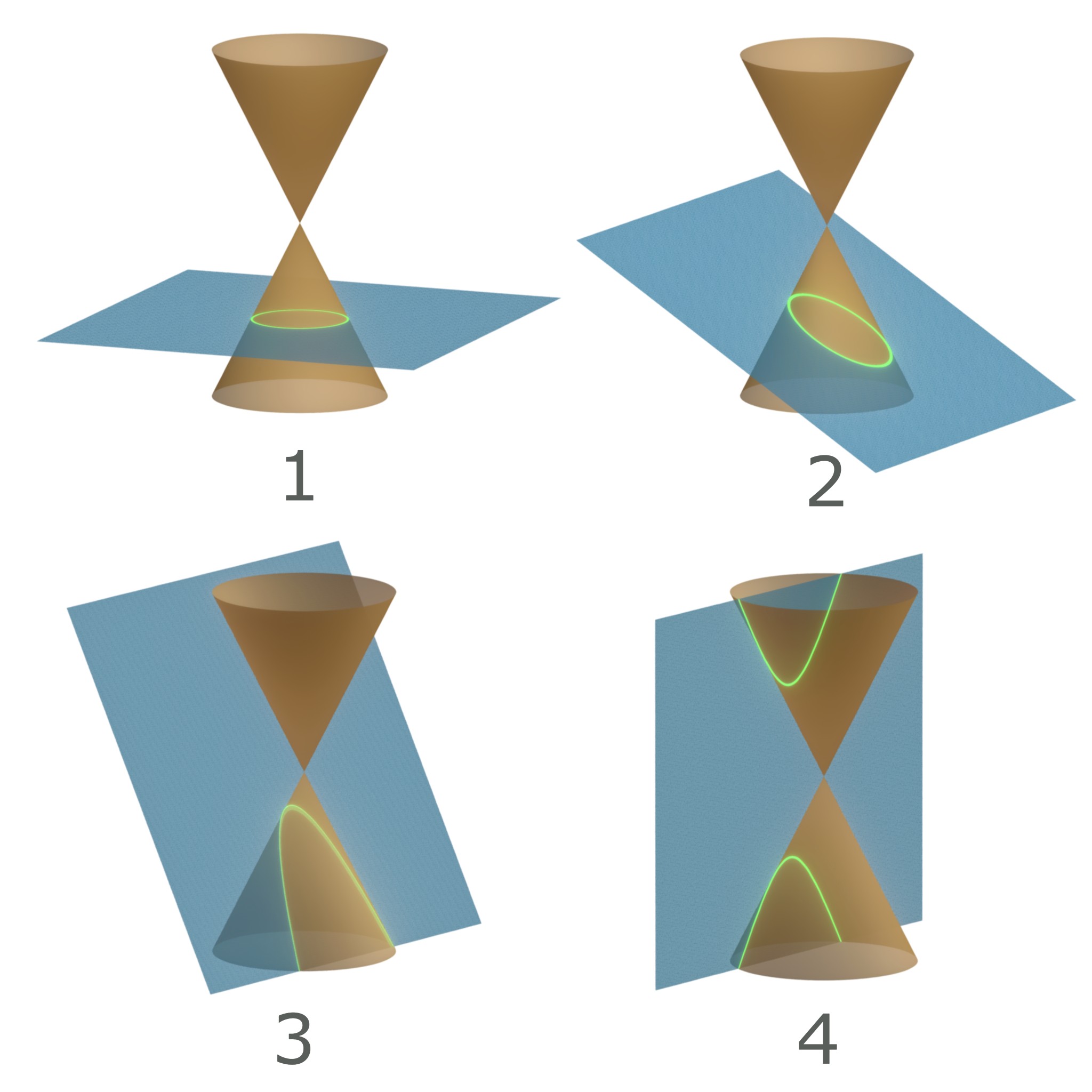
Conic Section
In mathematics, a conic section, quadratic curve or conic is a curve obtained as the intersection of the surface of a cone with a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, though historically it was sometimes called a fourth type. The ancient Greek mathematicians studied conic sections, culminating around 200 BC with Apollonius of Perga's systematic work on their properties. The conic sections in the Euclidean plane have various distinguishing properties, many of which can be used as alternative definitions. One such property defines a non-circular conic to be the set of those points whose distances to some particular point, called a '' focus'', and some particular line, called a ''directrix'', are in a fixed ratio, called the '' eccentricity''. The type of conic is determined by the value of the eccentricity. In analytic geometry, a conic may be defined as a plane algebrai ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
André Plane
In mathematics, André planes are a class of finite translation planes found by André. The Desarguesian plane and the Hall planes are examples of André planes; the two-dimensional regular nearfield planes are also André planes. Construction Let F = GF(q) be a finite field, and let K = GF(q^n) be a degree n extension field of F. Let \Gamma be the group of field automorphisms of K over F, and let \beta be an arbitrary mapping from F to \Gamma such that \beta(1)=1. Finally, let N be the norm function from K to F. Define a quasifield Q with the same elements and addition as K, but with multiplication defined via a \circ b = a^ \cdot b, where \cdot denotes the normal field multiplication in K. Using this quasifield to construct a plane yields an André plane. Properties # André planes exist for all proper prime powers p^n with p prime and n a positive integer greater than one. # Non-Desarguesian André planes exist for all proper prime powers except for 2^n where n is prime. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |