mathematics and architecture on:
[Wikipedia]
[Google]
[Amazon]

 Mathematics and architecture are related, since
Mathematics and architecture are related, since

 Vitruvius named three qualities required of architecture in his ''
Vitruvius named three qualities required of architecture in his ''
 The first Renaissance treatise on architecture was Leon Battista Alberti's 1450 (On the Art of Building); it became the first printed book on architecture in 1485. It was partly based on Vitruvius's ''De architectura'' and, via Nicomachus, Pythagorean arithmetic. Alberti starts with a cube, and derives ratios from it. Thus the diagonal of a face gives the ratio 1:, while the diameter of the sphere which circumscribes the cube gives 1:. Alberti also documented Filippo Brunelleschi's discovery of linear perspective, developed to enable the design of buildings which would look beautifully proportioned when viewed from a convenient distance.
The next major text was Sebastiano Serlio's ''Regole generali d'architettura'' (General Rules of Architecture); the first volume appeared in Venice in 1537; the 1545 volume (books1 and 2) covered geometry and perspective. Two of Serlio's methods for constructing perspectives were wrong, but this did not stop his work being widely used.
The first Renaissance treatise on architecture was Leon Battista Alberti's 1450 (On the Art of Building); it became the first printed book on architecture in 1485. It was partly based on Vitruvius's ''De architectura'' and, via Nicomachus, Pythagorean arithmetic. Alberti starts with a cube, and derives ratios from it. Thus the diagonal of a face gives the ratio 1:, while the diameter of the sphere which circumscribes the cube gives 1:. Alberti also documented Filippo Brunelleschi's discovery of linear perspective, developed to enable the design of buildings which would look beautifully proportioned when viewed from a convenient distance.
The next major text was Sebastiano Serlio's ''Regole generali d'architettura'' (General Rules of Architecture); the first volume appeared in Venice in 1537; the 1545 volume (books1 and 2) covered geometry and perspective. Two of Serlio's methods for constructing perspectives were wrong, but this did not stop his work being widely used.
 In 1570, Andrea Palladio published the influential '' I quattro libri dell'architettura'' (The Four Books of Architecture) in
In 1570, Andrea Palladio published the influential '' I quattro libri dell'architettura'' (The Four Books of Architecture) in
 Hyperboloid structures were used starting towards the end of the nineteenth century by Vladimir Shukhov for masts, lighthouses and cooling towers. Their striking shape is both aesthetically interesting and strong, using structural materials economically. Shukhov's first hyperboloidal tower was exhibited in
Hyperboloid structures were used starting towards the end of the nineteenth century by Vladimir Shukhov for masts, lighthouses and cooling towers. Their striking shape is both aesthetically interesting and strong, using structural materials economically. Shukhov's first hyperboloidal tower was exhibited in
 Denver International Airport's terminal building, completed in 1995, has a fabric roof supported as a minimal surface (i.e., its mean curvature is zero) by steel cables. It evokes
Denver International Airport's terminal building, completed in 1995, has a fabric roof supported as a minimal surface (i.e., its mean curvature is zero) by steel cables. It evokes
File:Bauhaus-Dessau Wohnheim Balkone.jpg, New Objectivity:
 The
The
 The mathematics of
The mathematics of

 The Parthenon is considered by authors such as John Julius Norwich "the most perfect Doric temple ever built". Its elaborate architectural refinements include "a subtle correspondence between the curvature of the stylobate, the taper of the naos walls and the ''entasis'' of the columns". '' Entasis'' refers to the subtle diminution in diameter of the columns as they rise. The stylobate is the platform on which the columns stand. As in other classical Greek temples, the platform has a slight parabolic upward curvature to shed rainwater and reinforce the building against earthquakes. The columns might therefore be supposed to lean outwards, but they actually lean slightly inwards so that if they carried on, they would meet about a kilometre and a half above the centre of the building; since they are all the same height, the curvature of the outer stylobate edge is transmitted to the architrave and roof above: "all follow the rule of being built to delicate curves".
The golden ratio was known in 300 B.C., when
The Parthenon is considered by authors such as John Julius Norwich "the most perfect Doric temple ever built". Its elaborate architectural refinements include "a subtle correspondence between the curvature of the stylobate, the taper of the naos walls and the ''entasis'' of the columns". '' Entasis'' refers to the subtle diminution in diameter of the columns as they rise. The stylobate is the platform on which the columns stand. As in other classical Greek temples, the platform has a slight parabolic upward curvature to shed rainwater and reinforce the building against earthquakes. The columns might therefore be supposed to lean outwards, but they actually lean slightly inwards so that if they carried on, they would meet about a kilometre and a half above the centre of the building; since they are all the same height, the curvature of the outer stylobate edge is transmitted to the architrave and roof above: "all follow the rule of being built to delicate curves".
The golden ratio was known in 300 B.C., when
 The historian of Islamic art Antonio Fernandez-Puertas suggests that the Alhambra, like the Great Mosque of Cordoba, was designed using the Hispano-Muslim foot or ''codo'' of about . In the palace's Court of the Lions, the proportions follow a series of surds. A rectangle with sides 1and has (by Pythagoras's theorem) a diagonal of , which describes the right triangle made by the sides of the court; the series continues with (giving a 1:2 ratio), and so on. The decorative patterns are similarly proportioned, generating squares inside circles and eight-pointed stars, generating six-pointed stars. There is no evidence to support earlier claims that the golden ratio was used in the Alhambra. The Court of the Lions is bracketed by the Hall of Two Sisters and the Hall of the Abencerrajes; a regular hexagon can be drawn from the centres of these two halls and the four inside corners of the Court of the Lions.
The Selimiye Mosque in
The historian of Islamic art Antonio Fernandez-Puertas suggests that the Alhambra, like the Great Mosque of Cordoba, was designed using the Hispano-Muslim foot or ''codo'' of about . In the palace's Court of the Lions, the proportions follow a series of surds. A rectangle with sides 1and has (by Pythagoras's theorem) a diagonal of , which describes the right triangle made by the sides of the court; the series continues with (giving a 1:2 ratio), and so on. The decorative patterns are similarly proportioned, generating squares inside circles and eight-pointed stars, generating six-pointed stars. There is no evidence to support earlier claims that the golden ratio was used in the Alhambra. The Court of the Lions is bracketed by the Hall of Two Sisters and the Hall of the Abencerrajes; a regular hexagon can be drawn from the centres of these two halls and the four inside corners of the Court of the Lions.
The Selimiye Mosque in

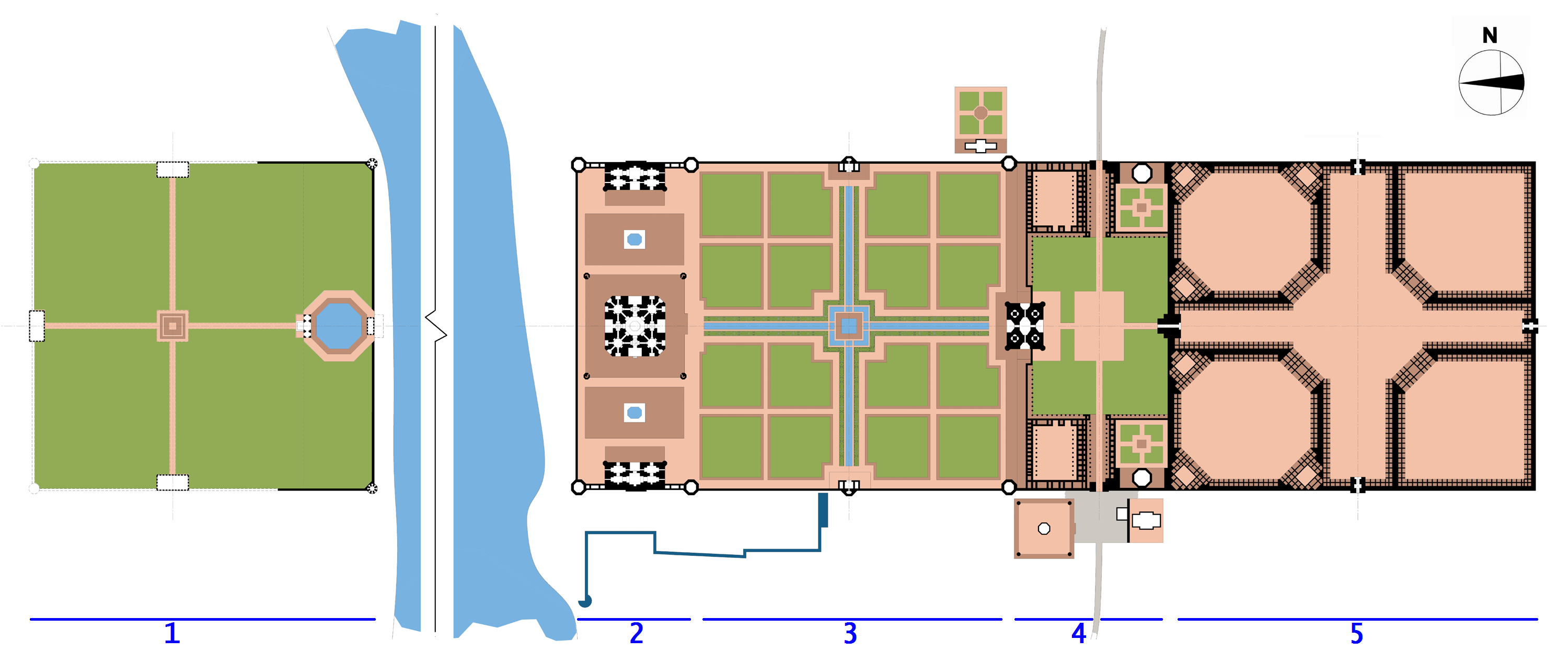 The Taj Mahal complex was laid out on a grid, subdivided into smaller grids. The historians of architecture Koch and Barraud agree with the traditional accounts that give the width of the complex as 374 Mughal yards or gaz, the main area being three 374-gaz squares. These were divided in areas like the bazaar and caravanserai into 17-gaz modules; the garden and terraces are in modules of 23 gaz, and are 368 gaz wide (16 x 23). The mausoleum, mosque and guest house are laid out on a grid of 7gaz. Koch and Barraud observe that if an octagon, used repeatedly in the complex, is given sides of 7units, then it has a width of 17 units, which may help to explain the choice of ratios in the complex.
The Taj Mahal complex was laid out on a grid, subdivided into smaller grids. The historians of architecture Koch and Barraud agree with the traditional accounts that give the width of the complex as 374 Mughal yards or gaz, the main area being three 374-gaz squares. These were divided in areas like the bazaar and caravanserai into 17-gaz modules; the garden and terraces are in modules of 23 gaz, and are 368 gaz wide (16 x 23). The mausoleum, mosque and guest house are laid out on a grid of 7gaz. Koch and Barraud observe that if an octagon, used repeatedly in the complex, is given sides of 7units, then it has a width of 17 units, which may help to explain the choice of ratios in the complex.
 The importance of water
The importance of water
File:Istanbul 036 (6498284165).jpg, The vaulting of the nave of Haghia Sophia, Istanbul ''( annotations''), 562
File:Battistero Firenze.jpg, The octagonal Baptistry of Saint John, Florence, completed in 1128
File:Jan Santini Aichel - Zelená Hora ground plan 2.jpg, Fivefold symmetries:

 Mathematics and architecture are related, since
Mathematics and architecture are related, since architecture
Architecture is the art and technique of designing and building, as distinguished from the skills associated with construction. It is both the process and the product of sketching, conceiving, planning, designing, and construction, constructi ...
, like some other arts, uses mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many ar ...
for several reasons. Apart from the mathematics needed when engineering building
A building or edifice is an enclosed Structure#Load-bearing, structure with a roof, walls and window, windows, usually standing permanently in one place, such as a house or factory. Buildings come in a variety of sizes, shapes, and functions, a ...
s, architects use geometry
Geometry (; ) is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. Geometry is, along with arithmetic, one of the oldest branches of mathematics. A mathematician w ...
: to define the spatial form of a building; from the Pythagoreans
Pythagoreanism originated in the 6th century BC, based on and around the teachings and beliefs held by Pythagoras and his followers, the Pythagoreans. Pythagoras established the first Pythagorean community in the Ancient Greece, ancient Greek co ...
of the sixth century BC onwards, to create architectural forms considered harmonious, and thus to lay out buildings and their surroundings according to mathematical, aesthetic
Aesthetics (also spelled esthetics) is the branch of philosophy concerned with the nature of beauty and taste, which in a broad sense incorporates the philosophy of art.Slater, B. H.Aesthetics ''Internet Encyclopedia of Philosophy,'' , acces ...
and sometimes religious principles; to decorate buildings with mathematical objects such as tessellations; and to meet environmental goals, such as to minimise wind speeds around the bases of tall buildings.
In ancient Egypt
Ancient Egypt () was a cradle of civilization concentrated along the lower reaches of the Nile River in Northeast Africa. It emerged from prehistoric Egypt around 3150BC (according to conventional Egyptian chronology), when Upper and Lower E ...
, ancient Greece
Ancient Greece () was a northeastern Mediterranean civilization, existing from the Greek Dark Ages of the 12th–9th centuries BC to the end of classical antiquity (), that comprised a loose collection of culturally and linguistically r ...
, India
India, officially the Republic of India, is a country in South Asia. It is the List of countries and dependencies by area, seventh-largest country by area; the List of countries by population (United Nations), most populous country since ...
, and the Islamic world
The terms Islamic world and Muslim world commonly refer to the Islamic community, which is also known as the Ummah. This consists of all those who adhere to the religious beliefs, politics, and laws of Islam or to societies in which Islam is ...
, buildings including pyramids, temples, mosques, palaces and mausoleum
A mausoleum is an external free-standing building constructed as a monument enclosing the burial chamber of a deceased person or people. A mausoleum without the person's remains is called a cenotaph. A mausoleum may be considered a type o ...
s were laid out with specific proportions for religious reasons. In Islamic architecture, geometric shapes and geometric tiling patterns are used to decorate buildings, both inside and outside. Some Hindu temples have a fractal
In mathematics, a fractal is a Shape, geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scale ...
-like structure where parts resemble the whole, conveying a message about the infinite in Hindu cosmology
Hindu cosmology is the description of the universe and its states of matter, cycles within time, physical structure, and effects on living entities according to Hindu texts. Hindu cosmology is also intertwined with the idea of a creator who allo ...
. In Chinese architecture
Chinese architecture () is the embodiment of an architectural style that has developed over millennia in China and has influenced architecture throughout East Asia. Since its emergence during the early ancient era, the structural principles of ...
, the tulou of Fujian province are circular, communal defensive structures. In the twenty-first century, mathematical ornamentation is again being used to cover public buildings.
In Renaissance architecture
Renaissance architecture is the European architecture of the period between the early 15th and early 16th centuries in different regions, demonstrating a conscious revival and development of certain elements of Ancient Greece, ancient Greek and ...
, symmetry
Symmetry () in everyday life refers to a sense of harmonious and beautiful proportion and balance. In mathematics, the term has a more precise definition and is usually used to refer to an object that is Invariant (mathematics), invariant und ...
and proportion were deliberately emphasized by architects such as Leon Battista Alberti
Leon Battista Alberti (; 14 February 1404 – 25 April 1472) was an Italian Renaissance humanist author, artist, architect, poet, Catholic priest, priest, linguistics, linguist, philosopher, and cryptography, cryptographer; he epitomised the natu ...
, Sebastiano Serlio and Andrea Palladio, influenced by Vitruvius
Vitruvius ( ; ; –70 BC – after ) was a Roman architect and engineer during the 1st century BC, known for his multi-volume work titled . As the only treatise on architecture to survive from antiquity, it has been regarded since the Renaissan ...
's ''De architectura
(''On architecture'', published as ''Ten Books on Architecture'') is a treatise on architecture written by the Ancient Rome, Roman architect and military engineer Vitruvius, Marcus Vitruvius Pollio and dedicated to his patron, the emperor Caesa ...
'' from ancient Rome
In modern historiography, ancient Rome is the Roman people, Roman civilisation from the founding of Rome, founding of the Italian city of Rome in the 8th century BC to the Fall of the Western Roman Empire, collapse of the Western Roman Em ...
and the arithmetic of the Pythagoreans from ancient Greece.
At the end of the nineteenth century, Vladimir Shukhov in Russia
Russia, or the Russian Federation, is a country spanning Eastern Europe and North Asia. It is the list of countries and dependencies by area, largest country in the world, and extends across Time in Russia, eleven time zones, sharing Borders ...
and Antoni Gaudí
Antoni Gaudí i Cornet ( , ; ; 25 June 1852 – 10 June 1926) was a Catalans, Catalan architect and designer from Spain, widely known as the greatest exponent of Catalan ''Modernisme''. Gaudí's works have a style, with most located in Barc ...
in Barcelona
Barcelona ( ; ; ) is a city on the northeastern coast of Spain. It is the capital and largest city of the autonomous community of Catalonia, as well as the second-most populous municipality of Spain. With a population of 1.6 million within c ...
pioneered the use of hyperboloid structures; in the Sagrada Família
The Basílica i Temple Expiatori de la Sagrada Família, otherwise known as Sagrada Família, is a church under construction in the Eixample district of Barcelona, Catalonia, Spain. It is the largest unfinished Catholic church in the world. Desi ...
, Gaudí also incorporated hyperbolic paraboloid
In geometry, a paraboloid is a quadric surface that has exactly one axial symmetry, axis of symmetry and no central symmetry, center of symmetry. The term "paraboloid" is derived from parabola, which refers to a conic section that has a similar p ...
s, tessellations, catenary arches, catenoids, helicoids, and ruled surfaces. In the twentieth century, styles such as modern architecture
Modern architecture, also called modernist architecture, or the modern movement, is an architectural movement and style that was prominent in the 20th century, between the earlier Art Deco and later postmodern movements. Modern architectur ...
and Deconstructivism
Deconstructivism is a postmodern architecture, postmodern architectural movement which appeared in the 1980s. It gives the impression of the fragmentation of the constructed building, commonly characterised by an absence of obvious harmony, ...
explored different geometries to achieve desired effects. Minimal surfaces have been exploited in tent-like roof coverings as at Denver International Airport, while Richard Buckminster Fuller
Richard Buckminster Fuller (; July 12, 1895 – July 1, 1983) was an American architect, systems theorist, writer, designer, inventor, philosopher, and futurist. He styled his name as R. Buckminster Fuller in his writings, publishing more th ...
pioneered the use of the strong thin-shell structures known as geodesic domes.
Connected fields
The architects Michael Ostwald and Kim Williams, considering the relationships betweenarchitecture
Architecture is the art and technique of designing and building, as distinguished from the skills associated with construction. It is both the process and the product of sketching, conceiving, planning, designing, and construction, constructi ...
and mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many ar ...
, note that the fields as commonly understood might seem to be only weakly connected, since architecture is a profession concerned with the practical matter of making buildings, while mathematics is the pure study of number and other abstract objects. But, they argue, the two are strongly connected, and have been since antiquity. In ancient Rome, Vitruvius
Vitruvius ( ; ; –70 BC – after ) was a Roman architect and engineer during the 1st century BC, known for his multi-volume work titled . As the only treatise on architecture to survive from antiquity, it has been regarded since the Renaissan ...
described an architect as a man who knew enough of a range of other disciplines, primarily geometry
Geometry (; ) is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. Geometry is, along with arithmetic, one of the oldest branches of mathematics. A mathematician w ...
, to enable him to oversee skilled artisans in all the other necessary areas, such as masons and carpenters. The same applied in the Middle Ages
In the history of Europe, the Middle Ages or medieval period lasted approximately from the 5th to the late 15th centuries, similarly to the post-classical period of global history. It began with the fall of the Western Roman Empire and ...
, where graduates learnt arithmetic
Arithmetic is an elementary branch of mathematics that deals with numerical operations like addition, subtraction, multiplication, and division. In a wider sense, it also includes exponentiation, extraction of roots, and taking logarithms.
...
, geometry and aesthetics
Aesthetics (also spelled esthetics) is the branch of philosophy concerned with the nature of beauty and taste (sociology), taste, which in a broad sense incorporates the philosophy of art.Slater, B. H.Aesthetics ''Internet Encyclopedia of Ph ...
alongside the basic syllabus of grammar, logic, and rhetoric (the trivium
The trivium is the lower division of the seven liberal arts and comprises grammar, logic, and rhetoric.
The trivium is implicit in ("On the Marriage of Philology and Mercury") by Martianus Capella, but the term was not used until the Carolin ...
) in elegant halls made by master builders who had guided many craftsmen. A master builder at the top of his profession was given the title of architect or engineer. In the Renaissance
The Renaissance ( , ) is a Periodization, period of history and a European cultural movement covering the 15th and 16th centuries. It marked the transition from the Middle Ages to modernity and was characterized by an effort to revive and sur ...
, the quadrivium of arithmetic, geometry, music and astronomy became an extra syllabus expected of the Renaissance man such as Leon Battista Alberti
Leon Battista Alberti (; 14 February 1404 – 25 April 1472) was an Italian Renaissance humanist author, artist, architect, poet, Catholic priest, priest, linguistics, linguist, philosopher, and cryptography, cryptographer; he epitomised the natu ...
. Similarly in England, Sir Christopher Wren
Sir Christopher Wren FRS (; – ) was an English architect, astronomer, mathematician and physicist who was one of the most highly acclaimed architects in the history of England. Known for his work in the English Baroque style, he was ac ...
, known today as an architect, was firstly a noted astronomer.
Williams and Ostwald, further overviewing the interaction of mathematics and architecture since 1500 according to the approach of the German sociologist Theodor Adorno
Theodor is a masculine given name. It is a German form of Theodore. It is also a variant of Teodor.
List of people with the given name Theodor
* Theodor Adorno, (1903–1969), German philosopher
* Theodor Aman, Romanian painter
* Theodor Blue ...
, identify three tendencies among architects, namely: to be ''revolutionary'', introducing wholly new ideas; ''reactionary'', failing to introduce change; or '' revivalist'', actually going backwards. They argue that architects have avoided looking to mathematics for inspiration in revivalist times. This would explain why in revivalist periods, such as the Gothic Revival
Gothic Revival (also referred to as Victorian Gothic or neo-Gothic) is an Architectural style, architectural movement that after a gradual build-up beginning in the second half of the 17th century became a widespread movement in the first half ...
in 19th century England, architecture had little connection to mathematics. Equally, they note that in reactionary times such as the Italian Mannerism
Mannerism is a style in European art that emerged in the later years of the Italian High Renaissance around 1520, spreading by about 1530 and lasting until about the end of the 16th century in Italy, when the Baroque style largely replaced it ...
of about 1520 to 1580, or the 17th century Baroque
The Baroque ( , , ) is a Western Style (visual arts), style of Baroque architecture, architecture, Baroque music, music, Baroque dance, dance, Baroque painting, painting, Baroque sculpture, sculpture, poetry, and other arts that flourished from ...
and Palladian movements, mathematics was barely consulted. In contrast, the revolutionary early 20th-century movements such as Futurism
Futurism ( ) was an Art movement, artistic and social movement that originated in Italy, and to a lesser extent in other countries, in the early 20th century. It emphasized dynamism, speed, technology, youth, violence, and objects such as the ...
and Constructivism actively rejected old ideas, embracing mathematics and leading to Modernist architecture. Towards the end of the 20th century, too, fractal
In mathematics, a fractal is a Shape, geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scale ...
geometry was quickly seized upon by architects, as was aperiodic tiling, to provide interesting and attractive coverings for buildings.
Architects use mathematics for several reasons, leaving aside the necessary use of mathematics in the engineering of buildings. Firstly, they use geometry because it defines the spatial form of a building.
Secondly, they use mathematics to design forms that are considered beautiful or harmonious. From the time of the Pythagoreans
Pythagoreanism originated in the 6th century BC, based on and around the teachings and beliefs held by Pythagoras and his followers, the Pythagoreans. Pythagoras established the first Pythagorean community in the Ancient Greece, ancient Greek co ...
with their religious philosophy of number, architects in ancient Greece
Ancient Greece () was a northeastern Mediterranean civilization, existing from the Greek Dark Ages of the 12th–9th centuries BC to the end of classical antiquity (), that comprised a loose collection of culturally and linguistically r ...
, ancient Rome
In modern historiography, ancient Rome is the Roman people, Roman civilisation from the founding of Rome, founding of the Italian city of Rome in the 8th century BC to the Fall of the Western Roman Empire, collapse of the Western Roman Em ...
, the Islamic world
The terms Islamic world and Muslim world commonly refer to the Islamic community, which is also known as the Ummah. This consists of all those who adhere to the religious beliefs, politics, and laws of Islam or to societies in which Islam is ...
and the Italian Renaissance
The Italian Renaissance ( ) was a period in History of Italy, Italian history between the 14th and 16th centuries. The period is known for the initial development of the broader Renaissance culture that spread across Western Europe and marked t ...
have chosen the proportions of the built environment – buildings and their designed surroundings – according to mathematical as well as aesthetic and sometimes religious principles. Thirdly, they may use mathematical objects such as tessellations to decorate buildings. Fourthly, they may use mathematics in the form of computer modelling to meet environmental goals, such as to minimise whirling air currents at the base of tall buildings.
Secular aesthetics
Ancient Rome

Vitruvius
The influential ancient Roman architect Vitruvius argued that the design of a building such as a temple depends on two qualities, proportion and ''symmetria''. Proportion ensures that each part of a building relates harmoniously to every other part. ''Symmetria'' in Vitruvius's usage means something closer to the English termmodularity
Modularity is the degree to which a system's components may be separated and recombined, often with the benefit of flexibility and variety in use. The concept of modularity is used primarily to reduce complexity by breaking a system into varying ...
than mirror symmetry, as again it relates to the assembling of (modular) parts into the whole building. In his Basilica at Fano
Fano () is a city and ''comune'' of the province of Pesaro and Urbino in the Marche region of Italy. It is a beach resort southeast of Pesaro, located where the ''Via Flaminia'' reaches the Adriatic Sea. It is the third city in the region by pop ...
, he uses ratios of small integers, especially the triangular numbers (1, 3, 6, 10, ...) to proportion the structure into (Vitruvian) modules. Thus the Basilica's width to length is 1:2; the aisle around it is as high as it is wide, 1:1; the columns are five feet thick and fifty feet high, 1:10.
 Vitruvius named three qualities required of architecture in his ''
Vitruvius named three qualities required of architecture in his ''De architectura
(''On architecture'', published as ''Ten Books on Architecture'') is a treatise on architecture written by the Ancient Rome, Roman architect and military engineer Vitruvius, Marcus Vitruvius Pollio and dedicated to his patron, the emperor Caesa ...
'', : firmness, usefulness (or "Commodity" in Henry Wotton's 17th century English), and delight. These can be used as categories for classifying the ways in which mathematics is used in architecture. Firmness encompasses the use of mathematics to ensure a building stands up, hence the mathematical tools used in design and to support construction, for instance to ensure stability and to model performance. Usefulness derives in part from the effective application of mathematics, reasoning about and analysing the spatial and other relationships in a design. Delight is an attribute of the resulting building, resulting from the embodying of mathematical relationships in the building; it includes aesthetic, sensual and intellectual qualities.
The Pantheon
The Pantheon in Rome has survived intact, illustrating classical Roman structure, proportion, and decoration. The main structure is a dome, the apex left open as a circular oculus to let in light; it is fronted by a short colonnade with a triangular pediment. The height to the oculus and the diameter of the interior circle are the same, , so the whole interior would fit exactly within a cube, and the interior could house a sphere of the same diameter. These dimensions make more sense when expressed inancient Roman units of measurement
The units of measurement of ancient Rome were generally consistent and well documented.
Length
The basic unit of Roman linear measurement was the ''pes'' (plural: ''pedes'') or Roman foot. Investigation of its relation to the English foot goes ...
: The dome spans 150 Roman feet); the oculus is 30 Roman feet in diameter; the doorway is 40 Roman feet high. The Pantheon remains the world's largest unreinforced concrete dome.
Renaissance
 The first Renaissance treatise on architecture was Leon Battista Alberti's 1450 (On the Art of Building); it became the first printed book on architecture in 1485. It was partly based on Vitruvius's ''De architectura'' and, via Nicomachus, Pythagorean arithmetic. Alberti starts with a cube, and derives ratios from it. Thus the diagonal of a face gives the ratio 1:, while the diameter of the sphere which circumscribes the cube gives 1:. Alberti also documented Filippo Brunelleschi's discovery of linear perspective, developed to enable the design of buildings which would look beautifully proportioned when viewed from a convenient distance.
The next major text was Sebastiano Serlio's ''Regole generali d'architettura'' (General Rules of Architecture); the first volume appeared in Venice in 1537; the 1545 volume (books1 and 2) covered geometry and perspective. Two of Serlio's methods for constructing perspectives were wrong, but this did not stop his work being widely used.
The first Renaissance treatise on architecture was Leon Battista Alberti's 1450 (On the Art of Building); it became the first printed book on architecture in 1485. It was partly based on Vitruvius's ''De architectura'' and, via Nicomachus, Pythagorean arithmetic. Alberti starts with a cube, and derives ratios from it. Thus the diagonal of a face gives the ratio 1:, while the diameter of the sphere which circumscribes the cube gives 1:. Alberti also documented Filippo Brunelleschi's discovery of linear perspective, developed to enable the design of buildings which would look beautifully proportioned when viewed from a convenient distance.
The next major text was Sebastiano Serlio's ''Regole generali d'architettura'' (General Rules of Architecture); the first volume appeared in Venice in 1537; the 1545 volume (books1 and 2) covered geometry and perspective. Two of Serlio's methods for constructing perspectives were wrong, but this did not stop his work being widely used.
 In 1570, Andrea Palladio published the influential '' I quattro libri dell'architettura'' (The Four Books of Architecture) in
In 1570, Andrea Palladio published the influential '' I quattro libri dell'architettura'' (The Four Books of Architecture) in Venice
Venice ( ; ; , formerly ) is a city in northeastern Italy and the capital of the Veneto Regions of Italy, region. It is built on a group of 118 islands that are separated by expanses of open water and by canals; portions of the city are li ...
. This widely printed book was largely responsible for spreading the ideas of the Italian Renaissance
The Italian Renaissance ( ) was a period in History of Italy, Italian history between the 14th and 16th centuries. The period is known for the initial development of the broader Renaissance culture that spread across Western Europe and marked t ...
throughout Europe, assisted by proponents like the English diplomat Henry Wotton with his 1624 ''The Elements of Architecture''. The proportions of each room within the villa were calculated on simple mathematical ratios like 3:4 and 4:5, and the different rooms within the house were interrelated by these ratios. Earlier architects had used these formulas for balancing a single symmetrical facade; however, Palladio's designs related to the whole, usually square, villa. Palladio permitted a range of ratios in the ''Quattro libri'', stating:
In 1615, Vincenzo Scamozzi published the late Renaissance treatise ''L'idea dell'architettura universale'' (The Idea of a Universal Architecture). He attempted to relate the design of cities and buildings to the ideas of Vitruvius and the Pythagoreans, and to the more recent ideas of Palladio.
Nineteenth century
 Hyperboloid structures were used starting towards the end of the nineteenth century by Vladimir Shukhov for masts, lighthouses and cooling towers. Their striking shape is both aesthetically interesting and strong, using structural materials economically. Shukhov's first hyperboloidal tower was exhibited in
Hyperboloid structures were used starting towards the end of the nineteenth century by Vladimir Shukhov for masts, lighthouses and cooling towers. Their striking shape is both aesthetically interesting and strong, using structural materials economically. Shukhov's first hyperboloidal tower was exhibited in Nizhny Novgorod
Nizhny Novgorod ( ; rus, links=no, Нижний Новгород, a=Ru-Nizhny Novgorod.ogg, p=ˈnʲiʐnʲɪj ˈnovɡərət, t=Lower Newtown; colloquially shortened to Nizhny) is a city and the administrative centre of Nizhny Novgorod Oblast an ...
in 1896.
Twentieth century
The early twentieth century movementModern architecture
Modern architecture, also called modernist architecture, or the modern movement, is an architectural movement and style that was prominent in the 20th century, between the earlier Art Deco and later postmodern movements. Modern architectur ...
, pioneered by Russian Constructivism, used rectilinear Euclidean (also called Cartesian) geometry. In the De Stijl
De Stijl (, ; 'The Style') was a Dutch art movement founded in 1917 by a group of artists and architects based in Leiden (Theo van Doesburg, Jacobus Oud, J.J.P. Oud), Voorburg (Vilmos Huszár, Jan Wils) and Laren, North Holland, Laren (Piet Mo ...
movement, the horizontal and the vertical were seen as constituting the universal. The architectural form consists of putting these two directional tendencies together, using roof planes, wall planes and balconies, which either slide past or intersect each other, as in the 1924 Rietveld Schröder House by Gerrit Rietveld.
Modernist architects were free to make use of curves as well as planes. Charles Holden's 1933 Arnos station has a circular ticket hall in brick with a flat concrete roof. In 1938, the Bauhaus painter László Moholy-Nagy
László Moholy-Nagy (; ; born László Weisz; July 20, 1895 – November 24, 1946) was a Kingdom of Hungary, Hungarian painter and photographer as well as a professor in the Bauhaus school. He was highly influenced by Constructivism (art), con ...
adopted Raoul Heinrich Francé's seven biotechnical elements, namely the crystal, the sphere, the cone, the plane, the (cuboidal) strip, the (cylindrical) rod, and the spiral, as the supposed basic building blocks of architecture inspired by nature.
Le Corbusier
Charles-Édouard Jeanneret (6 October 188727 August 1965), known as Le Corbusier ( , ; ), was a Swiss-French architectural designer, painter, urban planner and writer, who was one of the pioneers of what is now regarded as modern architecture ...
proposed an anthropometric
Anthropometry (, ) refers to the measurement of the human individual. An early tool of physical anthropology, it has been used for identification, for the purposes of understanding human physical variation, in paleoanthropology and in various a ...
scale of proportions in architecture, the Modulor, based on the supposed height of a man. Le Corbusier's 1955 Chapelle Notre-Dame du Haut uses free-form curves not describable in mathematical formulae. The shapes are said to be evocative of natural forms such as the prow
The bow () is the forward part of the hull (watercraft), hull of a ship or boat, the point that is usually most forward when the vessel is underway. The aft end of the boat is the stern.
Prow may be used as a synonym for bow or it may mean the f ...
of a ship or praying hands. The design is only at the largest scale: there is no hierarchy of detail at smaller scales, and thus no fractal dimension; the same applies to other famous twentieth-century buildings such as the Sydney Opera House
The Sydney Opera House is a multi-venue Performing arts center, performing arts centre in Sydney, New South Wales, Australia. Located on the foreshore of Sydney Harbour, it is widely regarded as one of the world's most famous and distinctive b ...
, Denver International Airport, and the Guggenheim Museum, Bilbao.
Contemporary architecture, in the opinion of the 90 leading architects who responded to a 2010 World Architecture Survey, is extremely diverse; the best was judged to be Frank Gehry's Guggenheim Museum, Bilbao.
 Denver International Airport's terminal building, completed in 1995, has a fabric roof supported as a minimal surface (i.e., its mean curvature is zero) by steel cables. It evokes
Denver International Airport's terminal building, completed in 1995, has a fabric roof supported as a minimal surface (i.e., its mean curvature is zero) by steel cables. It evokes Colorado
Colorado is a U.S. state, state in the Western United States. It is one of the Mountain states, sharing the Four Corners region with Arizona, New Mexico, and Utah. It is also bordered by Wyoming to the north, Nebraska to the northeast, Kansas ...
's snow-capped mountains and the teepee tents of Native Americans.
The architect Richard Buckminster Fuller
Richard Buckminster Fuller (; July 12, 1895 – July 1, 1983) was an American architect, systems theorist, writer, designer, inventor, philosopher, and futurist. He styled his name as R. Buckminster Fuller in his writings, publishing more th ...
is famous for designing strong thin-shell structures known as geodesic domes. The Montréal Biosphère dome is high; its diameter is .
Sydney Opera House has a dramatic roof consisting of soaring white vaults, reminiscent of ship's sails; to make them possible to construct using standardized components, the vaults are all composed of triangular sections of spherical shells with the same radius. These have the required uniform curvature
In mathematics, curvature is any of several strongly related concepts in geometry that intuitively measure the amount by which a curve deviates from being a straight line or by which a surface deviates from being a plane. If a curve or su ...
in every direction.
The late twentieth century movement Deconstructivism
Deconstructivism is a postmodern architecture, postmodern architectural movement which appeared in the 1980s. It gives the impression of the fragmentation of the constructed building, commonly characterised by an absence of obvious harmony, ...
creates deliberate disorder with what Nikos Salingaros in '' A Theory of Architecture'' calls random forms of high complexity by using non-parallel walls, superimposed grids and complex 2-D surfaces, as in Frank Gehry's Disney Concert Hall and Guggenheim Museum, Bilbao. Until the twentieth century, architecture students were obliged to have a grounding in mathematics. Salingaros argues that first "overly simplistic, politically-driven" Modernism
Modernism was an early 20th-century movement in literature, visual arts, and music that emphasized experimentation, abstraction, and Subjectivity and objectivity (philosophy), subjective experience. Philosophy, politics, architecture, and soc ...
and then "anti-scientific" Deconstructivism have effectively separated architecture from mathematics. He believes that this "reversal of mathematical values" is harmful, as the "pervasive aesthetic" of non-mathematical architecture trains people "to reject mathematical information in the built environment"; he argues that this has negative effects on society. Updated version of
Walter Gropius
Walter Adolph Georg Gropius (; 18 May 1883 – 5 July 1969) was a German-born American architect and founder of the Bauhaus, Bauhaus School, who is widely regarded as one of the pioneering masters of modernist architecture. He was a founder of ...
's Bauhaus, Dessau
Dessau is a district of the independent city of Dessau-Roßlau in Saxony-Anhalt at the confluence of the rivers Mulde and Elbe, in the ''States of Germany, Bundesland'' (Federal State) of Saxony-Anhalt. Until 1 July 2007, it was an independent ...
, 1925
File:Arnos Grove underground station 16 November 2012.jpg, Cylinder: Charles Holden's Arnos Grove tube station, 1933
File:RonchampCorbu.jpg, Modernism
Modernism was an early 20th-century movement in literature, visual arts, and music that emphasized experimentation, abstraction, and Subjectivity and objectivity (philosophy), subjective experience. Philosophy, politics, architecture, and soc ...
: Le Corbusier
Charles-Édouard Jeanneret (6 October 188727 August 1965), known as Le Corbusier ( , ; ), was a Swiss-French architectural designer, painter, urban planner and writer, who was one of the pioneers of what is now regarded as modern architecture ...
's Chapelle Notre Dame du Haut, 1955
File:Mtl. Biosphere in Sept. 2004.jpg, Geodesic dome: the Montréal Biosphère by R. Buckminster Fuller, 1967
File:Sydney Opera House Sails.jpg, Uniform curvature
In mathematics, curvature is any of several strongly related concepts in geometry that intuitively measure the amount by which a curve deviates from being a straight line or by which a surface deviates from being a plane. If a curve or su ...
: Sydney Opera House
The Sydney Opera House is a multi-venue Performing arts center, performing arts centre in Sydney, New South Wales, Australia. Located on the foreshore of Sydney Harbour, it is widely regarded as one of the world's most famous and distinctive b ...
, 1973
File:Image-Disney Concert Hall by Carol Highsmith edit-2.jpg, Deconstructivism
Deconstructivism is a postmodern architecture, postmodern architectural movement which appeared in the 1980s. It gives the impression of the fragmentation of the constructed building, commonly characterised by an absence of obvious harmony, ...
: Disney Concert Hall, Los Angeles, 2003
Religious principles
Ancient Egypt
pyramid
A pyramid () is a structure whose visible surfaces are triangular in broad outline and converge toward the top, making the appearance roughly a pyramid in the geometric sense. The base of a pyramid can be of any polygon shape, such as trian ...
s of ancient Egypt
Ancient Egypt () was a cradle of civilization concentrated along the lower reaches of the Nile River in Northeast Africa. It emerged from prehistoric Egypt around 3150BC (according to conventional Egyptian chronology), when Upper and Lower E ...
are tombs constructed with mathematical proportions, but which these were, and whether the Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite t ...
was used, are debated. The ratio of the slant height to half the base length of the Great Pyramid of Giza is less than 1% from the golden ratio
In mathematics, two quantities are in the golden ratio if their ratio is the same as the ratio of their summation, sum to the larger of the two quantities. Expressed algebraically, for quantities and with , is in a golden ratio to if
\fr ...
. If this was the design method, it would imply the use of Kepler's triangle (face angle 51°49'), but according to many historians of science, the golden ratio was not known until the time of the Pythagoreans
Pythagoreanism originated in the 6th century BC, based on and around the teachings and beliefs held by Pythagoras and his followers, the Pythagoreans. Pythagoras established the first Pythagorean community in the Ancient Greece, ancient Greek co ...
.
The proportions of some pyramids may have also been based on the 3:4:5 triangle (face angle 53°8'), known from the Rhind Mathematical Papyrus (c. 1650–1550 BC); this was first conjectured by historian Moritz Cantor in 1882. It is known that right angles were laid out accurately in ancient Egypt using knotted cords for measurement, that Plutarch
Plutarch (; , ''Ploútarchos'', ; – 120s) was a Greek Middle Platonist philosopher, historian, biographer, essayist, and priest at the Temple of Apollo (Delphi), Temple of Apollo in Delphi. He is known primarily for his ''Parallel Lives'', ...
recorded in '' Isis and Osiris'' (c. 100 AD) that the Egyptians admired the 3:4:5 triangle, and that a scroll from before 1700 BC demonstrated basic square
In geometry, a square is a regular polygon, regular quadrilateral. It has four straight sides of equal length and four equal angles. Squares are special cases of rectangles, which have four equal angles, and of rhombuses, which have four equal si ...
formulas. Historian Roger L. Cooke observes that "It is hard to imagine anyone being interested in such conditions without knowing the Pythagorean theorem," but also notes that no Egyptian text before 300 BC actually mentions the use of the theorem to find the length of a triangle's sides, and that there are simpler ways to construct a right angle. Cooke concludes that Cantor's conjecture remains uncertain; he guesses that the ancient Egyptians probably knew the Pythagorean theorem, but "there is no evidence that they used it to construct right angles."
Ancient India
Vaastu Shastra, the ancientIndia
India, officially the Republic of India, is a country in South Asia. It is the List of countries and dependencies by area, seventh-largest country by area; the List of countries by population (United Nations), most populous country since ...
n canons of architecture and town planning, employs symmetrical drawings called mandalas. Complex calculations are used to arrive at the dimensions of a building and its components. The designs are intended to integrate architecture with nature, the relative functions of various parts of the structure, and ancient beliefs utilizing geometric patterns ( yantra), symmetry and directional alignments. However, early builders may have come upon mathematical proportions by accident. The mathematician Georges Ifrah notes that simple "tricks" with string and stakes can be used to lay out geometric shapes, such as ellipses and right angles.
 The mathematics of
The mathematics of fractals
In mathematics, a fractal is a Shape, geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scale ...
has been used to show that the reason why existing buildings have universal appeal and are visually satisfying is because they provide the viewer with a sense of scale at different viewing distances. For example, in the tall gopuram gatehouses of Hindu
Hindus (; ; also known as Sanātanīs) are people who religiously adhere to Hinduism, also known by its endonym Sanātana Dharma. Jeffery D. Long (2007), A Vision for Hinduism, IB Tauris, , pp. 35–37 Historically, the term has also be ...
temples such as the Virupaksha Temple at Hampi built in the seventh century, and others such as the Kandariya Mahadev Temple at Khajuraho, the parts and the whole have the same character, with fractal dimension
In mathematics, a fractal dimension is a term invoked in the science of geometry to provide a rational statistical index of complexity detail in a pattern. A fractal pattern changes with the Scaling (geometry), scale at which it is measured.
It ...
in the range 1.7 to 1.8. The cluster of smaller towers (''shikhara'', lit. 'mountain') about the tallest, central, tower which represents the holy Mount Kailash
Mount Kailash (also Kailasa; ''Kangrinboqê'' or ''Gang Rinpoche''; ; ; , ) is a mountain in Ngari Prefecture, Tibet Autonomous Region of China. It lies in the Kailash Range (Gangdisê Mountains) of the Transhimalaya, in the western part ...
, abode of Lord Shiva
Shiva (; , ), also known as Mahadeva (; , , Help:IPA/Sanskrit, ɐɦaːd̪eːʋɐh and Hara, is one of the Hindu deities, principal deities of Hinduism. He is the God in Hinduism, Supreme Being in Shaivism, one of the major traditions w ...
, depicts the endless repetition of universes in Hindu cosmology
Hindu cosmology is the description of the universe and its states of matter, cycles within time, physical structure, and effects on living entities according to Hindu texts. Hindu cosmology is also intertwined with the idea of a creator who allo ...
. The religious studies scholar William J. Jackson observed of the pattern of towers grouped among smaller towers, themselves grouped among still smaller towers, that:
The Meenakshi Amman Temple is a large complex with multiple shrines, with the streets of Madurai
Madurai ( , , ), formerly known as Madura, is a major city in the States and union territories of India, Indian state of Tamil Nadu. It is the cultural capital of Tamil Nadu and the administrative headquarters of Madurai District, which is ...
laid out concentrically around it according to the shastras. The four gateways are tall towers ( gopurams) with fractal-like repetitive structure as at Hampi. The enclosures around each shrine are rectangular and surrounded by high stone walls.
Ancient Greece

Pythagoras
Pythagoras of Samos (; BC) was an ancient Ionian Greek philosopher, polymath, and the eponymous founder of Pythagoreanism. His political and religious teachings were well known in Magna Graecia and influenced the philosophies of P ...
(c. 569 – c. 475 B.C.) and his followers, the Pythagoreans, held that "all things are numbers". They observed the harmonies produced by notes with specific small-integer ratios of frequency, and argued that buildings too should be designed with such ratios. The Greek word ''symmetria'' originally denoted the harmony of architectural shapes in precise ratios from a building's smallest details right up to its entire design.
The Parthenon
The Parthenon (; ; ) is a former Ancient Greek temple, temple on the Acropolis of Athens, Athenian Acropolis, Greece, that was dedicated to the Greek gods, goddess Athena. Its decorative sculptures are considered some of the high points of c ...
is long, wide and high to the cornice. This gives a ratio of width to length of 4:9, and the same for height to width. Putting these together gives height:width:length of 16:36:81, or to the delight of the Pythagoreans 42:62:92. This sets the module as 0.858 m. A 4:9 rectangle can be constructed as three contiguous rectangles with sides in the ratio 3:4. Each half-rectangle is then a convenient 3:4:5 right triangle, enabling the angles and sides to be checked with a suitably knotted rope. The inner area (naos) similarly has 4:9 proportions ( wide by 48.3 m long); the ratio between the diameter of the outer columns, , and the spacing of their centres, , is also 4:9.
Euclid
Euclid (; ; BC) was an ancient Greek mathematician active as a geometer and logician. Considered the "father of geometry", he is chiefly known for the '' Elements'' treatise, which established the foundations of geometry that largely domina ...
described the method of geometric construction. It has been argued that the golden ratio was used in the design of the Parthenon and other ancient Greek buildings, as well as sculptures, paintings, and vases. More recent authors such as Nikos Salingaros, however, doubt all these claims. Experiments by the computer scientist George Markowsky failed to find any preference for the golden rectangle.
Islamic architecture
 The historian of Islamic art Antonio Fernandez-Puertas suggests that the Alhambra, like the Great Mosque of Cordoba, was designed using the Hispano-Muslim foot or ''codo'' of about . In the palace's Court of the Lions, the proportions follow a series of surds. A rectangle with sides 1and has (by Pythagoras's theorem) a diagonal of , which describes the right triangle made by the sides of the court; the series continues with (giving a 1:2 ratio), and so on. The decorative patterns are similarly proportioned, generating squares inside circles and eight-pointed stars, generating six-pointed stars. There is no evidence to support earlier claims that the golden ratio was used in the Alhambra. The Court of the Lions is bracketed by the Hall of Two Sisters and the Hall of the Abencerrajes; a regular hexagon can be drawn from the centres of these two halls and the four inside corners of the Court of the Lions.
The Selimiye Mosque in
The historian of Islamic art Antonio Fernandez-Puertas suggests that the Alhambra, like the Great Mosque of Cordoba, was designed using the Hispano-Muslim foot or ''codo'' of about . In the palace's Court of the Lions, the proportions follow a series of surds. A rectangle with sides 1and has (by Pythagoras's theorem) a diagonal of , which describes the right triangle made by the sides of the court; the series continues with (giving a 1:2 ratio), and so on. The decorative patterns are similarly proportioned, generating squares inside circles and eight-pointed stars, generating six-pointed stars. There is no evidence to support earlier claims that the golden ratio was used in the Alhambra. The Court of the Lions is bracketed by the Hall of Two Sisters and the Hall of the Abencerrajes; a regular hexagon can be drawn from the centres of these two halls and the four inside corners of the Court of the Lions.
The Selimiye Mosque in Edirne
Edirne (; ), historically known as Orestias, Adrianople, is a city in Turkey, in the northwestern part of the Edirne Province, province of Edirne in Eastern Thrace. Situated from the Greek and from the Bulgarian borders, Edirne was the second c ...
, Turkey, was built by Mimar Sinan to provide a space where the mihrab could be seen from anywhere inside the building. The very large central space is accordingly arranged as an octagon, formed by eight enormous pillars, and capped by a circular dome of diameter and high. The octagon is formed into a square with four semidomes, and externally by four exceptionally tall minarets, tall. The building's plan is thus a circle, inside an octagon, inside a square.
Mughal architecture

Mughal architecture
Mughal architecture is the style of architecture developed in the Mughal Empire in the 16th, 17th and 18th centuries throughout the ever-changing extent of their empire in the Indian subcontinent. It developed from the architectural styles of ea ...
, as seen in the abandoned imperial city of Fatehpur Sikri and the Taj Mahal
The Taj Mahal ( ; ; ) is an ivory-white marble mausoleum on the right bank of the river Yamuna in Agra, Uttar Pradesh, India. It was commissioned in 1631 by the fifth Mughal Empire, Mughal emperor, Shah Jahan () to house the tomb of his belo ...
complex, has a distinctive mathematical order and a strong aesthetic based on symmetry and harmony.
The Taj Mahal exemplifies Mughal architecture, both representing paradise
In religion and folklore, paradise is a place of everlasting happiness, delight, and bliss. Paradisiacal notions are often laden with pastoral imagery, and may be cosmogonical, eschatological, or both, often contrasted with the miseries of human ...
and displaying the Mughal Emperor
The emperors of the Mughal Empire, who were all members of the Timurid dynasty (House of Babur), ruled the empire from its inception on 21 April 1526 to its dissolution on 21 September 1857. They were supreme monarchs of the Mughal Empire in ...
Shah Jahan
Shah Jahan I, (Shahab-ud-Din Muhammad Khurram; 5 January 1592 – 22 January 1666), also called Shah Jahan the Magnificent, was the Emperor of Hindustan from 1628 until his deposition in 1658. As the fifth Mughal emperor, his reign marked the ...
's power through its scale, symmetry and costly decoration. The white marble mausoleum
A mausoleum is an external free-standing building constructed as a monument enclosing the burial chamber of a deceased person or people. A mausoleum without the person's remains is called a cenotaph. A mausoleum may be considered a type o ...
, decorated with pietra dura, the great gate (''Darwaza-i rauza''), other buildings, the gardens and paths together form a unified hierarchical design. The buildings include a mosque
A mosque ( ), also called a masjid ( ), is a place of worship for Muslims. The term usually refers to a covered building, but can be any place where Salah, Islamic prayers are performed; such as an outdoor courtyard.
Originally, mosques were si ...
in red sandstone on the west, and an almost identical building, the Jawab or 'answer' on the east to maintain the bilateral symmetry of the complex. The formal charbagh ('fourfold garden') is in four parts, symbolising the four rivers of Paradise, and offering views and reflections of the mausoleum. These are divided in turn into 16 parterres.
 The Taj Mahal complex was laid out on a grid, subdivided into smaller grids. The historians of architecture Koch and Barraud agree with the traditional accounts that give the width of the complex as 374 Mughal yards or gaz, the main area being three 374-gaz squares. These were divided in areas like the bazaar and caravanserai into 17-gaz modules; the garden and terraces are in modules of 23 gaz, and are 368 gaz wide (16 x 23). The mausoleum, mosque and guest house are laid out on a grid of 7gaz. Koch and Barraud observe that if an octagon, used repeatedly in the complex, is given sides of 7units, then it has a width of 17 units, which may help to explain the choice of ratios in the complex.
The Taj Mahal complex was laid out on a grid, subdivided into smaller grids. The historians of architecture Koch and Barraud agree with the traditional accounts that give the width of the complex as 374 Mughal yards or gaz, the main area being three 374-gaz squares. These were divided in areas like the bazaar and caravanserai into 17-gaz modules; the garden and terraces are in modules of 23 gaz, and are 368 gaz wide (16 x 23). The mausoleum, mosque and guest house are laid out on a grid of 7gaz. Koch and Barraud observe that if an octagon, used repeatedly in the complex, is given sides of 7units, then it has a width of 17 units, which may help to explain the choice of ratios in the complex.
Christian architecture
TheChristian
A Christian () is a person who follows or adheres to Christianity, a Monotheism, monotheistic Abrahamic religion based on the life and teachings of Jesus in Christianity, Jesus Christ. Christians form the largest religious community in the wo ...
patriarchal basilica
In Ancient Roman architecture, a basilica (Greek Basiliké) was a large public building with multiple functions that was typically built alongside the town's forum. The basilica was in the Latin West equivalent to a stoa in the Greek Eas ...
of Haghia Sophia in Byzantium
Byzantium () or Byzantion () was an ancient Greek city in classical antiquity that became known as Constantinople in late antiquity and Istanbul today. The Greek name ''Byzantion'' and its Latinization ''Byzantium'' continued to be used as a n ...
(now Istanbul
Istanbul is the List of largest cities and towns in Turkey, largest city in Turkey, constituting the country's economic, cultural, and historical heart. With Demographics of Istanbul, a population over , it is home to 18% of the Demographics ...
), first constructed in 537 (and twice rebuilt), was for a thousand years the largest cathedral ever built. It inspired many later buildings including Sultan Ahmed and other mosques in the city. The Byzantine architecture
Byzantine architecture is the architecture of the Byzantine Empire, or Eastern Roman Empire, usually dated from 330 AD, when Constantine the Great established a new Roman capital in Byzantium, which became Constantinople, until the Fall of Cons ...
includes a nave crowned by a circular dome and two half-domes, all of the same diameter (), with a further five smaller half-domes forming an apse
In architecture, an apse (: apses; from Latin , 'arch, vault'; from Ancient Greek , , 'arch'; sometimes written apsis; : apsides) is a semicircular recess covered with a hemispherical Vault (architecture), vault or semi-dome, also known as an ' ...
and four rounded corners of a vast rectangular interior. This was interpreted by mediaeval architects as representing the mundane below (the square base) and the divine heavens above (the soaring spherical dome). The emperor Justinian
Justinian I (, ; 48214 November 565), also known as Justinian the Great, was Roman emperor from 527 to 565.
His reign was marked by the ambitious but only partly realized ''renovatio imperii'', or "restoration of the Empire". This ambition was ...
used two geometers, Isidore of Miletus and Anthemius of Tralles as architects; Isidore compiled the works of Archimedes
Archimedes of Syracuse ( ; ) was an Ancient Greece, Ancient Greek Greek mathematics, mathematician, physicist, engineer, astronomer, and Invention, inventor from the ancient city of Syracuse, Sicily, Syracuse in History of Greek and Hellenis ...
on solid geometry
Solid geometry or stereometry is the geometry of Three-dimensional space, three-dimensional Euclidean space (3D space).
A solid figure is the region (mathematics), region of 3D space bounded by a two-dimensional closed surface; for example, a ...
, and was influenced by him.
 The importance of water
The importance of water baptism
Baptism (from ) is a Christians, Christian sacrament of initiation almost invariably with the use of water. It may be performed by aspersion, sprinkling or affusion, pouring water on the head, or by immersion baptism, immersing in water eit ...
in Christianity was reflected in the scale of baptistry architecture. The oldest, the Lateran Baptistry in Rome, built in 440, set a trend for octagonal baptistries; the baptismal font
A baptismal font is an Church architecture, ecclesiastical architectural element, which serves as a receptacle for baptismal water used for baptism, as a part of Christian initiation for both rites of Infant baptism, infant and Believer's bapti ...
inside these buildings was often octagonal, though Italy's largest baptistry, at Pisa, built between 1152 and 1363, is circular, with an octagonal font. It is high, with a diameter of (a ratio of 8:5). Saint Ambrose wrote that fonts and baptistries were octagonal "because on the eighth day, by rising, Christ loosens the bondage of death and receives the dead from their graves."
Saint Augustine
Augustine of Hippo ( , ; ; 13 November 354 – 28 August 430) was a theologian and philosopher of Berbers, Berber origin and the bishop of Hippo Regius in Numidia (Roman province), Numidia, Roman North Africa. His writings deeply influenced th ...
similarly described the eighth day as "everlasting ... hallowed by the resurrection of Christ". The octagonal Baptistry of Saint John, Florence, built between 1059 and 1128, is one of the oldest buildings in that city, and one of the last in the direct tradition of classical antiquity; it was extremely influential in the subsequent Florentine Renaissance, as major architects including Francesco Talenti, Alberti and Brunelleschi used it as the model of classical architecture.
The number five is used "exuberantly" in the 1721 Pilgrimage Church of St John of Nepomuk at Zelená hora, near Žďár nad Sázavou
Žďár nad Sázavou (; ) is a town in the Vysočina Region of the Czech Republic. It has about 20,000 inhabitants. The town is an industrial and tourist centre. It is known for the Pilgrimage Church of Saint John of Nepomuk, which is a UNESCO Wor ...
in the Czech republic, designed by Jan Blažej Santini Aichel. The nave is circular, surrounded by five pairs of columns and five oval domes alternating with ogival apses. The church further has five gates, five chapels, five altars and five stars; a legend claims that when Saint John of Nepomuk was martyred, five stars appeared over his head. The fivefold architecture may also symbolise the five wounds of Christ and the five letters of "Tacui" (Latin: "I kept silence" bout secrets of the confessional">confessional.html" ;"title="bout secrets of the confessional">bout secrets of the confessional.
Antoni Gaudí
Antoni Gaudí i Cornet ( , ; ; 25 June 1852 – 10 June 1926) was a Catalans, Catalan architect and designer from Spain, widely known as the greatest exponent of Catalan ''Modernisme''. Gaudí's works have a style, with most located in Barc ...
used a wide variety of geometric structures, some being minimal surfaces, in the Sagrada Família
The Basílica i Temple Expiatori de la Sagrada Família, otherwise known as Sagrada Família, is a church under construction in the Eixample district of Barcelona, Catalonia, Spain. It is the largest unfinished Catholic church in the world. Desi ...
, Barcelona
Barcelona ( ; ; ) is a city on the northeastern coast of Spain. It is the capital and largest city of the autonomous community of Catalonia, as well as the second-most populous municipality of Spain. With a population of 1.6 million within c ...
, started in 1882 (and not completed as of 2023). These include hyperbolic paraboloid
In geometry, a paraboloid is a quadric surface that has exactly one axial symmetry, axis of symmetry and no central symmetry, center of symmetry. The term "paraboloid" is derived from parabola, which refers to a conic section that has a similar p ...
s and hyperboloids of revolution, tessellations, catenary arches, catenoids, helicoids, and ruled surfaces. This varied mix of geometries is creatively combined in different ways around the church. For example, in the Passion Façade of Sagrada Família, Gaudí assembled stone "branches" in the form of hyperbolic paraboloids, which overlap at their tops (directrices) without, therefore, meeting at a point. In contrast, in the colonnade there are hyperbolic paraboloidal surfaces that smoothly join other structures to form unbounded surfaces. Further, Gaudí exploits natural patterns, themselves mathematical, with column">patterns in nature">natural patterns, themselves mathematical, with columns derived from the shapes of trees, and lintels made from unmodified basalt naturally cracked (by cooling from molten rock) into List of places with columnar jointed volcanics, hexagonal columns.
The 1971 Cathedral of Saint Mary of the Assumption, San Francisco has a saddle roof composed of eight segments of hyperbolic paraboloids, arranged so that the bottom horizontal cross section of the roof is a square and the top cross section is a Christian cross
The Christian cross, seen as representing the crucifixion of Jesus, is a religious symbol, symbol of Christianity. It is related to the crucifix, a cross that includes a ''corpus'' (a representation of Jesus' body, usually three-dimensional) a ...
. The building is a square on a side, and high. The 1970 Cathedral of Brasília by Oscar Niemeyer makes a different use of a hyperboloid structure; it is constructed from 16 identical concrete beams, each weighing 90 tonnes, arranged in a circle to form a hyperboloid of revolution, the white beams creating a shape like hands praying to heaven. Only the dome is visible from outside: most of the building is below ground.
Several medieval churches in Scandinavia are circular, including four on the Danish island of Bornholm
Bornholm () is a List of islands of Denmark, Danish island in the Baltic Sea, to the east of the rest of Denmark, south of Sweden, northeast of Germany and north of Poland.
Strategically located, Bornholm has been fought over for centuries. I ...
. One of the oldest of these, Østerlars Church from , has a circular nave around a massive circular stone column, pierced with arches and decorated with a fresco. The circular structure has three storeys and was apparently fortified, the top storey having served for defence.
Jan Santini Aichel
Jan Blažej Santini Aichel, also spelled Aichl (, ; 3 February 1677 – 7 December 1723) was a Czechs, Czech architect of Italians, Italian descent, whose major works are representative of the unique Czech Baroque architecture#Baroque G ...
's Pilgrimage Church of St John of Nepomuk at Zelená hora, 1721
File:Sagfampassion.jpg, Passion façade of Antoni Gaudí
Antoni Gaudí i Cornet ( , ; ; 25 June 1852 – 10 June 1926) was a Catalans, Catalan architect and designer from Spain, widely known as the greatest exponent of Catalan ''Modernisme''. Gaudí's works have a style, with most located in Barc ...
's Sagrada Família
The Basílica i Temple Expiatori de la Sagrada Família, otherwise known as Sagrada Família, is a church under construction in the Eixample district of Barcelona, Catalonia, Spain. It is the largest unfinished Catholic church in the world. Desi ...
, Barcelona
Barcelona ( ; ; ) is a city on the northeastern coast of Spain. It is the capital and largest city of the autonomous community of Catalonia, as well as the second-most populous municipality of Spain. With a population of 1.6 million within c ...
, started 1882
File:Catedral1 Rodrigo Marfan.jpg, Oscar Niemeyer's Cathedral of Brasília, 1970
File:St Mary's Cathedral - San Francisco.jpg, The Cathedral of Saint Mary of the Assumption, San Francisco, 1971
File:Oesterlarsfresco.jpg, Central column of Østerlars Nordic round church in Bornholm
Bornholm () is a List of islands of Denmark, Danish island in the Baltic Sea, to the east of the rest of Denmark, south of Sweden, northeast of Germany and north of Poland.
Strategically located, Bornholm has been fought over for centuries. I ...
, Denmark
Mathematical decoration
Islamic architectural decoration
Islamic buildings are often decorated with geometric patterns which typically make use of several mathematical tessellations, formed of ceramic tiles ( girih, zellige) that may themselves be plain or decorated with stripes. Symmetries such as stars with six, eight, or multiples of eight points are used in Islamic patterns. Some of these are based on the "Khatem Sulemani" or Solomon's seal motif, which is an eight-pointed star made of two squares, one rotated 45 degrees from the other on the same centre. Islamic patterns exploit many of the 17 possible wallpaper groups; as early as 1944, Edith Müller showed that the Alhambra made use of 11 wallpaper groups in its decorations, while in 1986Branko Grünbaum
Branko Grünbaum (; 2 October 1929 – 14 September 2018) was a Croatian-born mathematician of Jewish descentmuqarnas vaulting in the Sheikh Lotfollah Mosque,
File:London MMB «T1 Ravensbourne College.jpg, Ravensbourne College, London, 2010
File:Harpa.JPG, Harpa Concert and Conference Centre, Iceland, 2011
File:Umimirai Library.jpg, Kanazawa Umimirai Library, Japan, 2011
File:Museo Soumaya Plaza Carso V.jpg, Museo Soumaya, México, 2011
File:Coevorden.jpg, Coevorden fortification plan. 17th century
File:Palmanova1600.jpg, Palmanova,
 In
In
 Architects may also select the form of a building to meet environmental goals. For example, Foster and Partners' 30 St Mary Axe, London, known as " The Gherkin" for its cucumber-like shape, is a solid of revolution designed using parametric modelling. Its geometry was chosen not purely for aesthetic reasons, but to minimise whirling air currents at its base. Despite the building's apparently curved surface, all the panels of glass forming its skin are flat, except for the lens at the top. Most of the panels are
Architects may also select the form of a building to meet environmental goals. For example, Foster and Partners' 30 St Mary Axe, London, known as " The Gherkin" for its cucumber-like shape, is a solid of revolution designed using parametric modelling. Its geometry was chosen not purely for aesthetic reasons, but to minimise whirling air currents at its base. Despite the building's apparently curved surface, all the panels of glass forming its skin are flat, except for the lens at the top. Most of the panels are
Nexus Network Journal: Architecture and Mathematics Online
The International Society of the Arts, Mathematics, and Architecture
National University of Singapore: Mathematics in Art and Architecture
{{Mathematical art Mathematics and culture Architectural theory
Isfahan
Isfahan or Esfahan ( ) is a city in the Central District (Isfahan County), Central District of Isfahan County, Isfahan province, Iran. It is the capital of the province, the county, and the district. It is located south of Tehran. The city ...
, 1603–1619
File:Louvre Abu Dhabi under construction (cropped).jpg, Louvre Abu Dhabi under construction in 2015, its dome built up of layers of stars made of octagons, triangles, and squares
Modern architectural decoration
Towards the end of the 20th century, novel mathematical constructs such as fractal geometry and aperiodic tiling were seized upon by architects to provide interesting and attractive coverings for buildings. In 1913, the Modernist architect Adolf Loos had declared that "Ornament is a crime", influencing architectural thinking for the rest of the 20th century. In the 21st century, architects are again starting to explore the use of ornament. 21st century ornamentation is extremely diverse. Henning Larsen's 2011 Harpa Concert and Conference Centre, Reykjavik has what looks like a crystal wall of rock made of large blocks of glass. Foreign Office Architects' 2010 Ravensbourne College, London is tessellated decoratively with 28,000 anodised aluminium tiles in red, white and brown, interlinking circular windows of differing sizes. The tessellation uses three types of tile, an equilateral triangle and two irregular pentagons. Kazumi Kudo's Kanazawa Umimirai Library creates a decorative grid made of small circular blocks of glass set into plain concrete walls.Defence
Europe
The architecture offortification
A fortification (also called a fort, fortress, fastness, or stronghold) is a military construction designed for the defense of territories in warfare, and is used to establish rule in a region during peacetime. The term is derived from Lati ...
s evolved from medieval fortresses, which had high masonry walls, to low, symmetrical star fort
A bastion fort or ''trace italienne'' (a phrase derived from non-standard French, meaning 'Italian outline') is a fortification in a style developed during the early modern period in response to the ascendancy of gunpowder weapons such as c ...
s able to resist artillery
Artillery consists of ranged weapons that launch Ammunition, munitions far beyond the range and power of infantry firearms. Early artillery development focused on the ability to breach defensive walls and fortifications during sieges, and l ...
bombardment between the mid-fifteenth and nineteenth centuries. The geometry of the star shapes was dictated by the need to avoid dead zones where attacking infantry could shelter from defensive fire; the sides of the projecting points were angled to permit such fire to sweep the ground, and to provide crossfire (from both sides) beyond each projecting point. Well-known architects who designed such defences include Michelangelo
Michelangelo di Lodovico Buonarroti Simoni (6March 147518February 1564), known mononymously as Michelangelo, was an Italian sculptor, painter, architect, and poet of the High Renaissance. Born in the Republic of Florence, his work was inspir ...
, Baldassare Peruzzi, Vincenzo Scamozzi and Sébastien Le Prestre de Vauban.
The architectural historian Siegfried Giedion argued that the star-shaped fortification had a formative influence on the patterning of the Renaissance ideal city: "The Renaissance was hypnotized by one city type which for a century and a half—from Filarete to Scamozzi—was impressed upon all utopian schemes: this is the star-shaped city."
Italy
Italy, officially the Italian Republic, is a country in Southern Europe, Southern and Western Europe, Western Europe. It consists of Italian Peninsula, a peninsula that extends into the Mediterranean Sea, with the Alps on its northern land b ...
, a Venetian city within a star fort
A bastion fort or ''trace italienne'' (a phrase derived from non-standard French, meaning 'Italian outline') is a fortification in a style developed during the early modern period in response to the ascendancy of gunpowder weapons such as c ...
. 17th century
File:Neuf-Brisach 007 850.jpg, Neuf-Brisach, Alsace
Alsace (, ; ) is a cultural region and a territorial collectivity in the Grand Est administrative region of northeastern France, on the west bank of the upper Rhine, next to Germany and Switzerland. In January 2021, it had a population of 1,9 ...
, one of the Fortifications of Vauban
China
 In
In Chinese architecture
Chinese architecture () is the embodiment of an architectural style that has developed over millennia in China and has influenced architecture throughout East Asia. Since its emergence during the early ancient era, the structural principles of ...
, the tulou of Fujian province are circular, communal defensive structures with mainly blank walls and a single iron-plated wooden door, some dating back to the sixteenth century. The walls are topped with roofs that slope gently both outwards and inwards, forming a ring. The centre of the circle is an open cobbled courtyard, often with a well, surrounded by timbered galleries up to five stories high.
Environmental goals
 Architects may also select the form of a building to meet environmental goals. For example, Foster and Partners' 30 St Mary Axe, London, known as " The Gherkin" for its cucumber-like shape, is a solid of revolution designed using parametric modelling. Its geometry was chosen not purely for aesthetic reasons, but to minimise whirling air currents at its base. Despite the building's apparently curved surface, all the panels of glass forming its skin are flat, except for the lens at the top. Most of the panels are
Architects may also select the form of a building to meet environmental goals. For example, Foster and Partners' 30 St Mary Axe, London, known as " The Gherkin" for its cucumber-like shape, is a solid of revolution designed using parametric modelling. Its geometry was chosen not purely for aesthetic reasons, but to minimise whirling air currents at its base. Despite the building's apparently curved surface, all the panels of glass forming its skin are flat, except for the lens at the top. Most of the panels are quadrilateral
In Euclidean geometry, geometry a quadrilateral is a four-sided polygon, having four Edge (geometry), edges (sides) and four Vertex (geometry), corners (vertices). The word is derived from the Latin words ''quadri'', a variant of four, and ''l ...
s, as they can be cut from rectangular glass with less wastage than triangular panels.
The traditional yakhchal (ice pit) of Persia
Iran, officially the Islamic Republic of Iran (IRI) and also known as Persia, is a country in West Asia. It borders Iraq to the west, Turkey, Azerbaijan, and Armenia to the northwest, the Caspian Sea to the north, Turkmenistan to the nort ...
functioned as an evaporative cooler. Above ground, the structure had a domed shape, but had a subterranean storage space for ice and sometimes food as well. The subterranean space and the thick heat-resistant construction insulated the storage space year round. The internal space was often further cooled with windcatchers.
See also
* Black Rock City * Mathematics and art *Patterns in nature
Patterns in nature are visible regularities of form found in the natural world. These patterns recur in different contexts and can sometimes be modelled mathematically. Natural patterns include symmetries, trees, spirals, meanders, wave ...
Notes
References
External links
Nexus Network Journal: Architecture and Mathematics Online
The International Society of the Arts, Mathematics, and Architecture
National University of Singapore: Mathematics in Art and Architecture
{{Mathematical art Mathematics and culture Architectural theory