In the

mathematical Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics ...

field of

point-set topology In mathematics, general topology is the branch of topology that deals with the basic set-theoretic definitions and constructions used in topology. It is the foundation of most other branches of topology, including differential topology, geomet ...

, a continuum (plural: "continua") is a nonempty

compact Compact as used in politics may refer broadly to a pact or treaty; in more specific cases it may refer to: * Interstate compact * Blood compact, an ancient ritual of the Philippines * Compact government, a type of colonial rule utilized in British ...

connected

metric space In mathematics, a metric space is a set together with a notion of '' distance'' between its elements, usually called points. The distance is measured by a function called a metric or distance function. Metric spaces are the most general sett ...

, or, less frequently, a compact connected

Hausdorff space In topology and related branches of mathematics, a Hausdorff space ( , ), separated space or T2 space is a topological space where, for any two distinct points, there exist neighbourhoods of each which are disjoint from each other. Of the many ...

. Continuum theory is the branch of

topology In mathematics, topology (from the Greek words , and ) is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing ho ...

devoted to the study of continua.

Definitions

* A continuum that contains more than one point is called nondegenerate. * A subset ''A'' of a continuum ''X'' such that ''A'' itself is a continuum is called a subcontinuum of ''X''. A space homeomorphic to a subcontinuum of the

Euclidean plane In mathematics, the Euclidean plane is a Euclidean space of dimension two. That is, a geometric setting in which two real quantities are required to determine the position of each point ( element of the plane), which includes affine notions ...

R² is called a planar continuum. * A continuum ''X'' is homogeneous if for every two points ''x'' and ''y'' in ''X'', there exists a homeomorphism ''h'': ''X'' → ''X'' such that ''h''(''x'') = ''y''. * A Peano continuum is a continuum that is

locally connected In topology and other branches of mathematics, a topological space ''X'' is locally connected if every point admits a neighbourhood basis consisting entirely of open, connected sets. Background Throughout the history of topology, connectedne ...

at each point. * An indecomposable continuum is a continuum that cannot be represented as the union of two proper subcontinua. A continuum ''X'' is hereditarily indecomposable if every subcontinuum of ''X'' is indecomposable. * The dimension of a continuum usually means its

topological dimension In mathematics, the Lebesgue covering dimension or topological dimension of a topological space is one of several different ways of defining the dimension of the space in a topologically invariant way. Informal discussion For ordinary Euclidean ...

. A one-dimensional continuum is often called a curve.

Examples

* An arc is a space homeomorphic to the

closed interval In mathematics, a (real) interval is a set of real numbers that contains all real numbers lying between any two numbers of the set. For example, the set of numbers satisfying is an interval which contains , , and all numbers in between. Othe ...

,1 If ''h'': ,1→ ''X'' is a homeomorphism and ''h''(0) = ''p'' and ''h''(1) = ''q'' then ''p'' and ''q'' are called the endpoints of ''X''; one also says that ''X'' is an arc from ''p'' to ''q''. An arc is the simplest and most familiar type of a continuum. It is one-dimensional,

arcwise connected In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint non-empty open subsets. Connectedness is one of the principal topological properties that ...

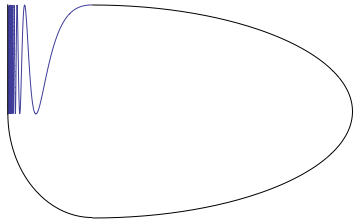
, and locally connected. * The

topologist's sine curve In the branch of mathematics known as topology, the topologist's sine curve or Warsaw sine curve is a topological space with several interesting properties that make it an important textbook example. It can be defined as the graph of the functi ...

is a subset of the plane that is the union of the graph of the function ''f''(''x'') = sin(1/''x''), 0 < ''x'' ≤ 1 with the segment −1 ≤ ''y'' ≤ 1 of the ''y''-axis. It is a one-dimensional continuum that is not arcwise connected, and it is locally disconnected at the points along the ''y''-axis. * The

Warsaw circle Shape theory is a branch of topology that provides a more global view of the topological spaces than homotopy theory. The two coincide on compacta dominated homotopically by finite polyhedra. Shape theory associates with the Čech homology theory ...

is obtained by "closing up" the

by an arc connecting (0,−1) and (1,sin(1)). It is a one-dimensional continuum whose

homotopy group In mathematics, homotopy groups are used in algebraic topology to classify topological spaces. The first and simplest homotopy group is the fundamental group, denoted \pi_1(X), which records information about loops in a space. Intuitively, homot ...

s are all trivial, but it is not a

contractible space In mathematics, a topological space ''X'' is contractible if the identity map on ''X'' is null-homotopic, i.e. if it is homotopic to some constant map. Intuitively, a contractible space is one that can be continuously shrunk to a point within ...

* An ''n''-cell is a space homeomorphic to the closed ball in the

Euclidean space Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's ''Elements'', it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean sp ...

R^''n''. It is contractible and is the simplest example of an ''n''-dimensional continuum. * An ''n''-sphere is a space homeomorphic to the standard

n-sphere In mathematics, an -sphere or a hypersphere is a topological space that is homeomorphic to a ''standard'' -''sphere'', which is the set of points in -dimensional Euclidean space that are situated at a constant distance from a fixed point, ca ...

in the (''n'' + 1)-dimensional Euclidean space. It is an ''n''-dimensional homogeneous continuum that is not contractible, and therefore different from an ''n''-cell. * The

Hilbert cube In mathematics, the Hilbert cube, named after David Hilbert, is a topological space that provides an instructive example of some ideas in topology. Furthermore, many interesting topological spaces can be embedded in the Hilbert cube; that is, ...

is an infinite-dimensional continuum. *

Solenoid upright=1.20, An illustration of a solenoid upright=1.20, Magnetic field created by a seven-loop solenoid (cross-sectional view) described using field lines A solenoid () is a type of electromagnet formed by a helix, helical coil of wire whose ...

s are among the simplest examples of indecomposable homogeneous continua. They are neither arcwise connected nor locally connected. * The Sierpinski carpet, also known as the ''Sierpinski universal curve'', is a one-dimensional planar Peano continuum that contains a homeomorphic image of any one-dimensional planar continuum. * The

pseudo-arc In general topology, the pseudo-arc is the simplest nondegenerate hereditarily indecomposable continuum. The pseudo-arc is an arc-like homogeneous continuum, and played a central role in the classification of homogeneous planar continua. R. H. Bi ...

is a homogeneous hereditarily indecomposable planar continuum.

Properties

There are two fundamental techniques for constructing continua, by means of ''nested intersections'' and ''inverse limits''. :* If is a nested family of continua, i.e. ''X''_''n'' ⊇ ''X''_''n''+1, then their intersection is a continuum. :* If is an inverse sequence of continua ''X''_''n'', called the coordinate spaces, together with

continuous map In mathematics, a continuous function is a function such that a continuous variation (that is a change without jump) of the argument induces a continuous variation of the value of the function. This means that there are no abrupt changes in va ...

s ''f''_''n'': ''X''_''n''+1 → ''X''_''n'', called the bonding maps, then its

inverse limit In mathematics, the inverse limit (also called the projective limit) is a construction that allows one to "glue together" several related objects, the precise gluing process being specified by morphisms between the objects. Thus, inverse limits ...

is a continuum. A finite or countable product of continua is a continuum.

References

Sources

* Sam B. Nadler, Jr, ''Continuum theory. An introduction''. Pure and Applied Mathematics, Marcel Dekker. .

External links

Open problems in continuum theory

Examples in continuum theory

Continuum Theory and Topological Dynamics
M. Barge and J. Kennedy, in Open Problems in Topology, J. van Mill and G.M. Reed (Editors) Elsevier Science Publishers B.V. (North-Holland), 1990.
Hyperspacewiki
{{Topology de:Kontinuum (Mathematik)#Kontinua in der Topologie