|
Z Table
In statistics, a standard normal table, also called the unit normal table or Z table, is a mathematical table for the values of , the cumulative distribution function of the normal distribution. It is used to find the probability that a statistic is observed below, above, or between values on the standard normal distribution, and by extension, any normal distribution. Since probability tables cannot be printed for every normal distribution, as there are an infinite variety of normal distributions, it is common practice to convert a normal to a standard normal (known as a z-score) and then use the standard normal table to find probabilities. Normal and standard normal distribution Normal distributions are symmetrical, bell-shaped distributions that are useful in describing real-world data. The ''standard'' normal distribution, represented by , is the normal distribution having a mean of 0 and a standard deviation of 1. Conversion If is a random variable from a normal distri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistics
Statistics (from German language, German: ', "description of a State (polity), state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a scientific, industrial, or social problem, it is conventional to begin with a statistical population or a statistical model to be studied. Populations can be diverse groups of people or objects such as "all people living in a country" or "every atom composing a crystal". Statistics deals with every aspect of data, including the planning of data collection in terms of the design of statistical survey, surveys and experimental design, experiments. When census data (comprising every member of the target population) cannot be collected, statisticians collect data by developing specific experiment designs and survey sample (statistics), samples. Representative sampling assures that inferences and conclusions can reasonably extend from the sample ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Standard Deviation
In statistics, the standard deviation is a measure of the amount of variation of the values of a variable about its Expected value, mean. A low standard Deviation (statistics), deviation indicates that the values tend to be close to the mean (also called the expected value) of the set, while a high standard deviation indicates that the values are spread out over a wider range. The standard deviation is commonly used in the determination of what constitutes an outlier and what does not. Standard deviation may be abbreviated SD or std dev, and is most commonly represented in mathematical texts and equations by the lowercase Greek alphabet, Greek letter Sigma, σ (sigma), for the population standard deviation, or the Latin script, Latin letter ''s'', for the sample standard deviation. The standard deviation of a random variable, Sample (statistics), sample, statistical population, data set, or probability distribution is the square root of its variance. (For a finite population, v ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Error Function
In mathematics, the error function (also called the Gauss error function), often denoted by , is a function \mathrm: \mathbb \to \mathbb defined as: \operatorname z = \frac\int_0^z e^\,\mathrm dt. The integral here is a complex Contour integration, contour integral which is path-independent because \exp(-t^2) is Holomorphic function, holomorphic on the whole complex plane \mathbb. In many applications, the function argument is a real number, in which case the function value is also real. In some old texts, the error function is defined without the factor of \frac. This nonelementary integral is a sigmoid function, sigmoid function that occurs often in probability, statistics, and partial differential equations. In statistics, for non-negative real values of , the error function has the following interpretation: for a real random variable that is normal distribution, normally distributed with mean 0 and standard deviation \frac, is the probability that falls in the range . ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Phi (letter)
Phi ( ; uppercase Φ, lowercase φ or ϕ; ''pheî'' ; Modern Greek: ''fi'' ) is the twenty-first letter of the Greek alphabet. In Archaic and Classical Greek (c. 9th to 4th century BC), it represented an aspirated voiceless bilabial plosive (), which was the origin of its usual romanization as . During the later part of Classical Antiquity, in Koine Greek (c. 4th century BC to 4th century AD), its pronunciation shifted to a voiceless bilabial fricative (), and by the Byzantine Greek period (c. 4th century AD to 15th century AD) it developed its modern pronunciation as a voiceless labiodental fricative (). The romanization of the Modern Greek phoneme is therefore usually . It may be that phi originated as the letter qoppa (Ϙ, ϙ), and initially represented the sound before shifting to Classical Greek . In traditional Greek numerals, phi has a value of 500 () or 500,000 (). The Cyrillic letter Ef (Ф, ф) descends from phi. Like other Greek letters, lowercase phi (enc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normal Cumulative Distribution Function
In probability theory and statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is f(x) = \frac e^\,. The parameter is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma^2 is the variance. The standard deviation of the distribution is (sigma). A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converge ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Z Cumulative From Minus Infinity
Z, or z, is the twenty-sixth and last letter of the Latin alphabet. It is used in the modern English alphabet, in the alphabets of other Western European languages, and in others worldwide. Its usual names in English are ''zed'' (), which is most commonly used in British English, and ''zee'' (), most commonly used in American English, with an occasional archaic variant ''izzard'' ()."Z", ''Oxford English Dictionary,'' 2nd edition (1989); ''Merriam-Webster's Third New International Dictionary of the English Language, Unabridged'' (1993); "zee", ''op. cit''. Name In most English-speaking countries, including Australia, Canada, India, Ireland, New Zealand, South Africa and the United Kingdom, the letter's name is ''zed'' , reflecting its derivation from the Greek letter ''zeta'' (this dates to Latin, which borrowed Y and Z from Greek), but in American English its name is ''zee'' , analogous to the names for B, C, D, etc., and deriving from a late 17th-century English dialecta ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Z-score
In statistics, the standard score or ''z''-score is the number of standard deviations by which the value of a raw score (i.e., an observed value or data point) is above or below the mean value of what is being observed or measured. Raw scores above the mean have positive standard scores, while those below the mean have negative standard scores. It is calculated by subtracting the population mean from an individual raw score and then dividing the difference by the population standard deviation. This process of converting a raw score into a standard score is called standardizing or normalizing (however, "normalizing" can refer to many types of ratios; see ''Normalization'' for more). Standard scores are most commonly called ''z''-scores; the two terms may be used interchangeably, as they are in this article. Other equivalent terms in use include z-value, z-statistic, normal score, standardized variable and pull in high energy physics. Computing a z-score requires knowledge ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mean
A mean is a quantity representing the "center" of a collection of numbers and is intermediate to the extreme values of the set of numbers. There are several kinds of means (or "measures of central tendency") in mathematics, especially in statistics. Each attempts to summarize or typify a given group of data, illustrating the magnitude and sign of the data set. Which of these measures is most illuminating depends on what is being measured, and on context and purpose. The ''arithmetic mean'', also known as "arithmetic average", is the sum of the values divided by the number of values. The arithmetic mean of a set of numbers ''x''1, ''x''2, ..., x''n'' is typically denoted using an overhead bar, \bar. If the numbers are from observing a sample of a larger group, the arithmetic mean is termed the '' sample mean'' (\bar) to distinguish it from the group mean (or expected value) of the underlying distribution, denoted \mu or \mu_x. Outside probability and statistics, a wide rang ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Table
Mathematical tables are lists of numbers showing the results of a calculation with varying arguments. Trigonometric tables were used in ancient Greece and India for applications to astronomy and celestial navigation, and continued to be widely used until electronic calculators became cheap and plentiful in the 1970s, in order to simplify and drastically speed up computation. Tables of logarithms and trigonometric functions were common in math and science textbooks, and specialized tables were published for numerous applications. History and use The first tables of trigonometric functions known to be made were by Hipparchus (c.190 – c.120 BCE) and Menelaus (c.70–140 CE), but both have been lost. Along with the surviving table of Ptolemy (c. 90 – c.168 CE), they were all tables of chords and not of half-chords, that is, the sine function. The table produced by the Indian mathematician Āryabhaṭa (476–550 CE) is considered the first sine table ever constructed. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
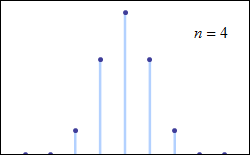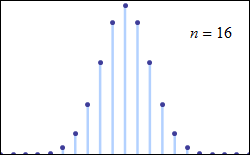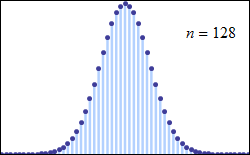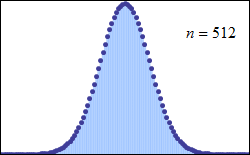
Normal Distribution
In probability theory and statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is f(x) = \frac e^\,. The parameter is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma^2 is the variance. The standard deviation of the distribution is (sigma). A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Z-score
In statistics, the standard score or ''z''-score is the number of standard deviations by which the value of a raw score (i.e., an observed value or data point) is above or below the mean value of what is being observed or measured. Raw scores above the mean have positive standard scores, while those below the mean have negative standard scores. It is calculated by subtracting the population mean from an individual raw score and then dividing the difference by the population standard deviation. This process of converting a raw score into a standard score is called standardizing or normalizing (however, "normalizing" can refer to many types of ratios; see ''Normalization'' for more). Standard scores are most commonly called ''z''-scores; the two terms may be used interchangeably, as they are in this article. Other equivalent terms in use include z-value, z-statistic, normal score, standardized variable and pull in high energy physics. Computing a z-score requires knowledge ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |