|
Relationships Among Probability Distributions
In probability theory and statistics, there are several relationships among probability distributions. These relations can be categorized in the following groups: *One distribution is a special case of another with a broader parameter space *Transforms (function of a random variable); *Combinations (function of several variables); *Approximation (limit) relationships; *Compound relationships (useful for Bayesian inference); *Duality; * Conjugate priors. Special case of distribution parametrization * A binomial distribution with parameters ''n'' = 1 and ''p'' is a Bernoulli distribution with parameter ''p''. * A negative binomial distribution with parameters ''n'' = 1 and ''p'' is a geometric distribution with parameter ''p''. * A gamma distribution with shape parameter ''α'' = 1 and rate parameter ''β'' is an exponential distribution with rate parameter ''β''. * A gamma distribution with shape parameter ''α'' = ''v''/2 and rate parameter ''β'' = ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Relationships Among Some Of Univariate Probability Distributions
Relationship most often refers to: * Family relations and relatives: consanguinity * Interpersonal relationship, a strong, deep, or close association or acquaintance between two or more people * Correlation and dependence, relationships in mathematics and statistics between two variables or sets of data * Semantic relationship, an ontology component * Romance (love), a connection between two people driven by love and/or sexual attraction Relationship or Relationships may also refer to: Arts and media * "Relationship" (song), by Young Thug featuring Future * "Relationships", an episode of the British TV series ''As Time Goes By'' * The Relationship, an American rock band ** ''The Relationship'' (album), their 2010 album * The Relationships, an English band who played at the 2009 Truck Festival * ''Relationships'', a 1994 album by BeBe & CeCe Winans * ''Relationships'', a 2001 album by Georgie Fame * "Relationship", a song by Lakeside on the 1987 album ''Power'' * "Relations ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Continuous Uniform Distribution
In probability theory and statistics, the continuous uniform distribution or rectangular distribution is a family of symmetric probability distributions. The distribution describes an experiment where there is an arbitrary outcome that lies between certain bounds. The bounds are defined by the parameters, ''a'' and ''b'', which are the minimum and maximum values. The interval can either be closed (e.g. , b or open (e.g. (a, b)). Therefore, the distribution is often abbreviated ''U'' (''a'', ''b''), where U stands for uniform distribution. The difference between the bounds defines the interval length; all intervals of the same length on the distribution's support are equally probable. It is the maximum entropy probability distribution for a random variable ''X'' under no constraint other than that it is contained in the distribution's support. Definitions Probability density function The probability density function of the continuous uniform distribution is: : f(x)=\begin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
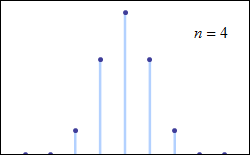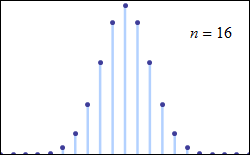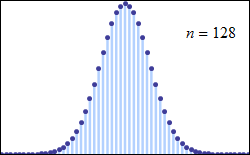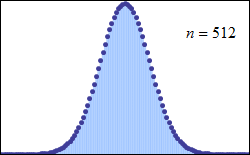
Normal Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rayleigh Distribution
In probability theory and statistics, the Rayleigh distribution is a continuous probability distribution for nonnegative-valued random variables. Up to rescaling, it coincides with the chi distribution with two degrees of freedom. The distribution is named after Lord Rayleigh (). A Rayleigh distribution is often observed when the overall magnitude of a vector is related to its directional components. One example where the Rayleigh distribution naturally arises is when wind velocity is analyzed in two dimensions. Assuming that each component is uncorrelated, normally distributed with equal variance, and zero mean, then the overall wind speed (vector magnitude) will be characterized by a Rayleigh distribution. A second example of the distribution arises in the case of random complex numbers whose real and imaginary components are independently and identically distributed Gaussian with equal variance and zero mean. In that case, the absolute value of the complex number is Ra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Power Law
In statistics, a power law is a functional relationship between two quantities, where a relative change in one quantity results in a proportional relative change in the other quantity, independent of the initial size of those quantities: one quantity varies as a power of another. For instance, considering the area of a square in terms of the length of its side, if the length is doubled, the area is multiplied by a factor of four. Empirical examples The distributions of a wide variety of physical, biological, and man-made phenomena approximately follow a power law over a wide range of magnitudes: these include the sizes of craters on the moon and of solar flares, the foraging pattern of various species, the sizes of activity patterns of neuronal populations, the frequencies of words in most languages, frequencies of family names, the species richness in clades of organisms, the sizes of power outages, volcanic eruptions, human judgments of stimulus intensity and many other quantit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Error Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal distributi ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Logistic Distribution
Logistic may refer to: Mathematics * Logistic function, a sigmoid function used in many fields ** Logistic map, a recurrence relation that sometimes exhibits chaos ** Logistic regression, a statistical model using the logistic function ** Logit, the inverse of the logistic function ** Logistic distribution, the derivative of the logistic function, a continuous probability distribution, used in probability theory and statistics * Mathematical logic, subfield of mathematics exploring the applications of formal logic to mathematics Other uses * Logistics, the management of resources and their distributions ** Logistic engineering, the scientific study of logistics ** Military logistics, the study of logistics at the service of military units and operations See also *Logic (other) Logic is the study of the principles and criteria of valid inference and demonstration Logic may also refer to: *Mathematical logic, a branch of mathematics that grew out of symbolic log ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Erlang Distribution
The Erlang distribution is a two-parameter family of continuous probability distributions with support x \in exponential distribution">exponential variables with mean 1/\lambda each. Equivalently, it is the distribution of the time until the ''k''th event of a Poisson process with a rate of \lambda. The Erlang and Poisson distributions are complementary, in that while the Poisson distribution counts the number of events that occur in a fixed amount of time, the Erlang distribution counts the amount of time until the occurrence of a fixed number of events. When k=1, the distribution simplifies to the exponential distribution. The Erlang distribution is a special case of the gamma distribution wherein the shape of the distribution is discretised. The Erlang distribution was developed by Agner Krarup Erlang, A. K. Erlang to examine the number of telephone calls which might be made at the same time to the operators of the switching stations. This work on telephone Teletraffic en ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normal Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Lomax Distribution
The Lomax distribution, conditionally also called the Pareto Type II distribution, is a heavy-tail probability distribution used in business, economics, actuarial science, queueing theory and Internet traffic modeling. It is named after K. S. Lomax. It is essentially a Pareto distribution that has been shifted so that its support begins at zero. Characterization Probability density function The probability density function (pdf) for the Lomax distribution is given by :p(x) = \leftright, \qquad x \geq 0, with shape parameter \alpha > 0 and scale parameter \lambda > 0. The density can be rewritten in such a way that more clearly shows the relation to the Pareto Type I distribution. That is: :p(x) = . Non-central moments The \nuth non-central moment E\left ^\nu\right/math> exists only if the shape parameter \alpha strictly exceeds \nu, when the moment has the value :E\left(X^\nu\right) = \frac Related distributions Relation to the Pareto distribution The Lo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Burr Distribution
In probability theory, statistics and econometrics, the Burr Type XII distribution or simply the Burr distribution is a continuous probability distribution for a non-negative random variable. It is also known as the Singh–Maddala distribution and is one of a number of different distributions sometimes called the "generalized log-logistic distribution". It is most commonly used to model household income, see for example: Household income in the U.S. and compare to magenta graph at right. The Burr (Type XII) distribution has probability density function: : \begin f(x;c,k) & = ck\frac \\ ptf(x;c,k,\lambda) & = \frac \left( \frac \right)^ \left + \left(\frac\right)^c\right \end and cumulative distribution function In probability theory and statistics, the cumulative distribution function (CDF) of a real-valued random variable X, or just distribution function of X, evaluated at x, is the probability that X will take a value less than or equal to x. Ev ...: :F(x;c, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Cauchy Distribution
The Cauchy distribution, named after Augustin Cauchy, is a continuous probability distribution. It is also known, especially among physicists, as the Lorentz distribution (after Hendrik Lorentz), Cauchy–Lorentz distribution, Lorentz(ian) function, or Breit–Wigner distribution. The Cauchy distribution f(x; x_0,\gamma) is the distribution of the -intercept of a ray issuing from (x_0,\gamma) with a uniformly distributed angle. It is also the distribution of the ratio of two independent normally distributed random variables with mean zero. The Cauchy distribution is often used in statistics as the canonical example of a " pathological" distribution since both its expected value and its variance are undefined (but see below). The Cauchy distribution does not have finite moments of order greater than or equal to one; only fractional absolute moments exist., Chapter 16. The Cauchy distribution has no moment generating function. In mathematics, it is closely related to the ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |