|
GNU Archimedes
Archimedes is a TCAD package for use by engineers to design and simulate submicron and mesoscopic semiconductor devices. Archimedes is free software and thus it can be copied, modified and redistributed under GPL. Archimedes uses the Ensemble Monte Carlo method and is able to simulate physics effects and transport for electrons and heavy holes in Silicon, Germanium, GaAs, InSb, AlSb, AlAs, AlxInxSb, AlxIn(1-x)Sb, AlP, AlSb, GaP, GaSb, InP and their compounds (III-V semiconductor materials), along with Silicon Oxide. Applied and/or self-consistent electrostatic and magnetic fields are handled with the Poisson and Faraday equations. The GNU project has announced in May 2012 that the software package ''Aeneas'' will be substituted by Archimedes, making this one the GNU package for Monte Carlo semiconductor devices simulations. Introduction Archimedes is the GNU package for semiconductor device simulations that has been released for the first time on 2005 under GPL. It has bee ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
GNU Project
The GNU Project () is a free software, mass collaboration project announced by Richard Stallman on September 27, 1983. Its goal is to give computer users freedom and control in their use of their computers and Computer hardware, computing devices by collaboratively developing and publishing software that gives everyone the rights to freely run the software, copy and distribute it, study it, and modify it. GNU software grants these rights in its GNU General Public License, license. In order to ensure that the ''entire'' software of a computer grants its users all freedom rights (use, share, study, modify), even the most fundamental and important part, the operating system (including all its numerous utility programs) needed to be free software. According to its manifesto, the founding goal of the project was to build a free operating system, and if possible, "everything useful that normally comes with a Unix system so that one could get along without any software that is not fre ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Boltzmann Transport Equation
The Boltzmann equation or Boltzmann transport equation (BTE) describes the statistical behaviour of a thermodynamic system not in a state of equilibrium, devised by Ludwig Boltzmann in 1872.Encyclopaedia of Physics (2nd Edition), R. G. Lerner, G. L. Trigg, VHC publishers, 1991, ISBN (Verlagsgesellschaft) 3-527-26954-1, ISBN (VHC Inc.) 0-89573-752-3. The classic example of such a system is a fluid with temperature gradients in space causing heat to flow from hotter regions to colder ones, by the random but biased transport of the particles making up that fluid. In the modern literature the term Boltzmann equation is often used in a more general sense, referring to any kinetic equation that describes the change of a macroscopic quantity in a thermodynamic system, such as energy, charge or particle number. The equation arises not by analyzing the individual positions and momenta of each particle in the fluid but rather by considering a probability distribution for the position ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Density Of States
In solid state physics and condensed matter physics, the density of states (DOS) of a system describes the number of modes per unit frequency range. The density of states is defined as D(E) = N(E)/V , where N(E)\delta E is the number of states in the system of volume V whose energies lie in the range from E to E+\delta E. It is mathematically represented as a distribution by a probability density function, and it is generally an average over the space and time domains of the various states occupied by the system. The density of states is directly related to the dispersion relations of the properties of the system. High DOS at a specific energy level means that many states are available for occupation. Generally, the density of states of matter is continuous. In isolated systems however, such as atoms or molecules in the gas phase, the density distribution is discrete, like a spectral density. Local variations, most often due to distortions of the original system, are often re ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Fermi's Golden Rule
In quantum physics, Fermi's golden rule is a formula that describes the transition rate (the probability of a transition per unit time) from one energy eigenstate of a quantum system to a group of energy eigenstates in a continuum, as a result of a weak perturbation. This transition rate is effectively independent of time (so long as the strength of the perturbation is independent of time) and is proportional to the strength of the coupling between the initial and final states of the system (described by the square of the matrix element of the perturbation) as well as the density of states. It is also applicable when the final state is discrete, i.e. it is not part of a continuum, if there is some decoherence in the process, like relaxation or collision of the atoms, or like noise in the perturbation, in which case the density of states is replaced by the reciprocal of the decoherence bandwidth. General Although the rule is named after Enrico Fermi, most of the work leading to it i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Scattering
Scattering is a term used in physics to describe a wide range of physical processes where moving particles or radiation of some form, such as light or sound, are forced to deviate from a straight trajectory by localized non-uniformities (including particles and radiation) in the medium through which they pass. In conventional use, this also includes deviation of reflected radiation from the angle predicted by the law of reflection. Reflections of radiation that undergo scattering are often called ''diffuse reflections'' and unscattered reflections are called ''specular'' (mirror-like) reflections. Originally, the term was confined to light scattering (going back at least as far as Isaac Newton in the 17th century). As more "ray"-like phenomena were discovered, the idea of scattering was extended to them, so that William Herschel could refer to the scattering of "heat rays" (not then recognized as electromagnetic in nature) in 1800. John Tyndall, a pioneer in light scattering researc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Band Structure
In solid-state physics, the electronic band structure (or simply band structure) of a solid describes the range of energy levels that electrons may have within it, as well as the ranges of energy that they may not have (called ''band gaps'' or ''forbidden bands''). Band theory derives these bands and band gaps by examining the allowed quantum mechanical wave functions for an electron in a large, periodic lattice of atoms or molecules. Band theory has been successfully used to explain many physical properties of solids, such as electrical resistivity and optical absorption, and forms the foundation of the understanding of all solid-state devices (transistors, solar cells, etc.). Why bands and band gaps occur The electrons of a single, isolated atom occupy atomic orbitals each of which has a discrete energy level. When two or more atoms join together to form a molecule, their atomic orbitals overlap and hybridize. Similarly, if a large number ''N'' of identical atoms c ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Monte Carlo Method
Monte Carlo methods, or Monte Carlo experiments, are a broad class of computational algorithms that rely on repeated random sampling to obtain numerical results. The underlying concept is to use randomness to solve problems that might be deterministic in principle. They are often used in physical and mathematical problems and are most useful when it is difficult or impossible to use other approaches. Monte Carlo methods are mainly used in three problem classes: optimization, numerical integration, and generating draws from a probability distribution. In physics-related problems, Monte Carlo methods are useful for simulating systems with many coupled degrees of freedom, such as fluids, disordered materials, strongly coupled solids, and cellular structures (see cellular Potts model, interacting particle systems, McKean–Vlasov processes, kinetic models of gases). Other examples include modeling phenomena with significant uncertainty in inputs such as the calculation of ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Momentum Space
In physics and geometry, there are two closely related vector spaces, usually three-dimensional but in general of any finite dimension. Position space (also real space or coordinate space) is the set of all ''position vectors'' r in space, and has dimensions of length; a position vector defines a point in space. (If the position vector of a point particle varies with time, it will trace out a path, the trajectory of a particle.) Momentum space is the set of all '' momentum vectors'' p a physical system can have; the momentum vector of a particle corresponds to its motion, with units of asslength]imesup>−1. Mathematically, the duality between position and momentum is an example of ''Pontryagin duality''. In particular, if a function is given in position space, ''f''(r), then its Fourier transform obtains the function in momentum space, ''φ''(p). Conversely, the inverse Fourier transform of a momentum space function is a position space function. These quantities and ideas tra ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Distribution Function (physics)
:''This article describes the ''distribution function'' as used in physics. You may be looking for the related mathematical concepts of cumulative distribution function or probability density function.'' In molecular kinetic theory in physics, a system's distribution function is a function of seven variables, f(x,y,z,t;v_x,v_y,v_z), which gives the number of particles per unit volume in single-particle phase space. It is the number of particles per unit volume having approximately the velocity \mathbf=(v_x,v_y,v_z) near the position \mathbf=(x,y,z) and time t. The usual normalization of the distribution function is :n(x,y,z,t) = \int f \,dv_x \,dv_y \,dv_z, :N(t) = \int n \,dx \,dy \,dz, where, ''N'' is the total number of particles, and ''n'' is the number density of particles – the number of particles per unit volume, or the density divided by the mass of individual particles. A distribution function may be specialised with respect to a particular set of dimensions. E.g. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Monte Carlo Methods For Electron Transport
The Monte Carlo method for electron transport is a Semiclassical physics, semiclassical Monte Carlo (MC) approach of modeling semiconductor transport. Assuming the carrier motion consists of free flights interrupted by scattering mechanisms, a computer is utilized to simulate the trajectories of particles as they move across the device under the influence of an electric field using classical mechanics. The scattering events and the duration of particle flight is determined through the use of random numbers. Background Boltzmann transport equation The Boltzmann transport equation model has been the main tool used in the analysis of transport in semiconductors. The BTE equation is given by: : \frac + \frac \nabla_k E(k) \nabla_r f + \frac \nabla_k f = \left[\frac\right]_\mathrm : v = \frac \nabla_k E(k) The Distribution function (physics), distribution function, ''f'', is a dimensionless function which is used to extract all observable of interest and gives a full depictio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Linux
Linux ( or ) is a family of open-source Unix-like operating systems based on the Linux kernel, an operating system kernel first released on September 17, 1991, by Linus Torvalds. Linux is typically packaged as a Linux distribution, which includes the kernel and supporting system software and libraries, many of which are provided by the GNU Project. Many Linux distributions use the word "Linux" in their name, but the Free Software Foundation uses the name "GNU/Linux" to emphasize the importance of GNU software, causing some controversy. Popular Linux distributions include Debian, Fedora Linux, and Ubuntu, the latter of which itself consists of many different distributions and modifications, including Lubuntu and Xubuntu. Commercial distributions include Red Hat Enterprise Linux and SUSE Linux Enterprise. Desktop Linux distributions include a windowing system such as X11 or Wayland, and a desktop environment such as GNOME or KDE Plasma. Distributions intended for ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mesoscopic
Mesoscopic physics is a subdiscipline of condensed matter physics that deals with materials of an intermediate size. These materials range in size between the nanoscale for a quantity of atoms (such as a molecule) and of materials measuring micrometres. The lower limit can also be defined as being the size of individual atoms. At the micrometre level are bulk materials. Both mesoscopic and macroscopic objects contain many atoms. Whereas average properties derived from constituent materials describe macroscopic objects, as they usually obey the laws of classical mechanics, a mesoscopic object, by contrast, is affected by thermal fluctuations around the average, and its electronic behavior may require modeling at the level of quantum mechanics.Sci-Tech Dictionary. McGraw-Hill Dictionary of Scientific and Technical Terms. 2003. McGraw-Hill Companies, Inc A macroscopic electronic device, when scaled down to a meso-size, starts revealing quantum mechanical properties. For example, at ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
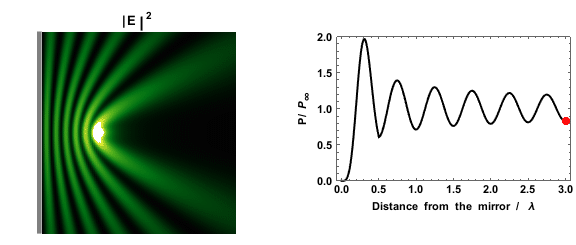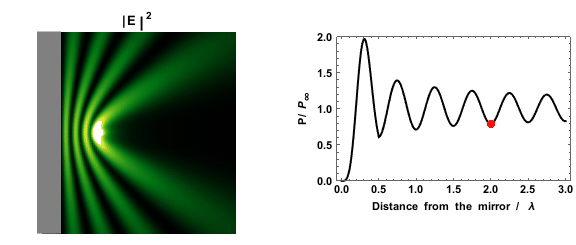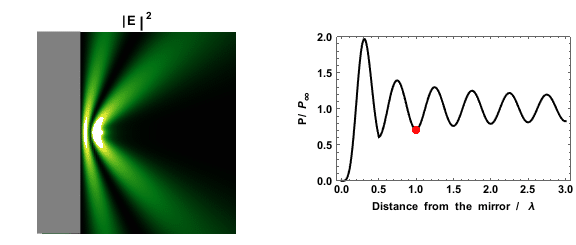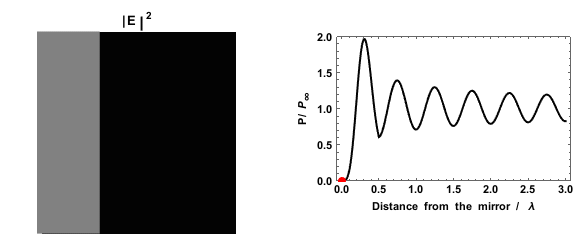



.jpg)