|
Colin Adams (mathematician)
Colin Conrad Adams (born October 13, 1956) is a mathematician primarily working in the areas of hyperbolic 3-manifolds and knot theory. His book, ''The Knot Book'', has been praised for its accessible approach to advanced topics in knot theory. He is currently Francis Christopher Oakley Third Century Professor of Mathematics at Williams College, where he has been since 1985. He writes "Mathematically Bent", a column of math for the '' Mathematical Intelligencer''. His nephew is popular American singer Still Woozy. Academic career Adams received a B.Sc. from MIT in 1978 and a Ph.D. in mathematics from the University of Wisconsin–Madison in 1983. His dissertation was entitled "Hyperbolic Structures on Link Complements" and supervised by James Cannon. In 2012 he became a fellow of the American Mathematical Society. [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics
Mathematics is an area of knowledge that includes the topics of numbers, formulas and related structures, shapes and the spaces in which they are contained, and quantities and their changes. These topics are represented in modern mathematics with the major subdisciplines of number theory, algebra, geometry, and analysis, respectively. There is no general consensus among mathematicians about a common definition for their academic discipline. Most mathematical activity involves the discovery of properties of abstract objects and the use of pure reason to prove them. These objects consist of either abstractions from nature orin modern mathematicsentities that are stipulated to have certain properties, called axioms. A ''proof'' consists of a succession of applications of deductive rules to already established results. These results include previously proved theorems, axioms, andin case of abstraction from naturesome basic properties that are considered true starting poin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
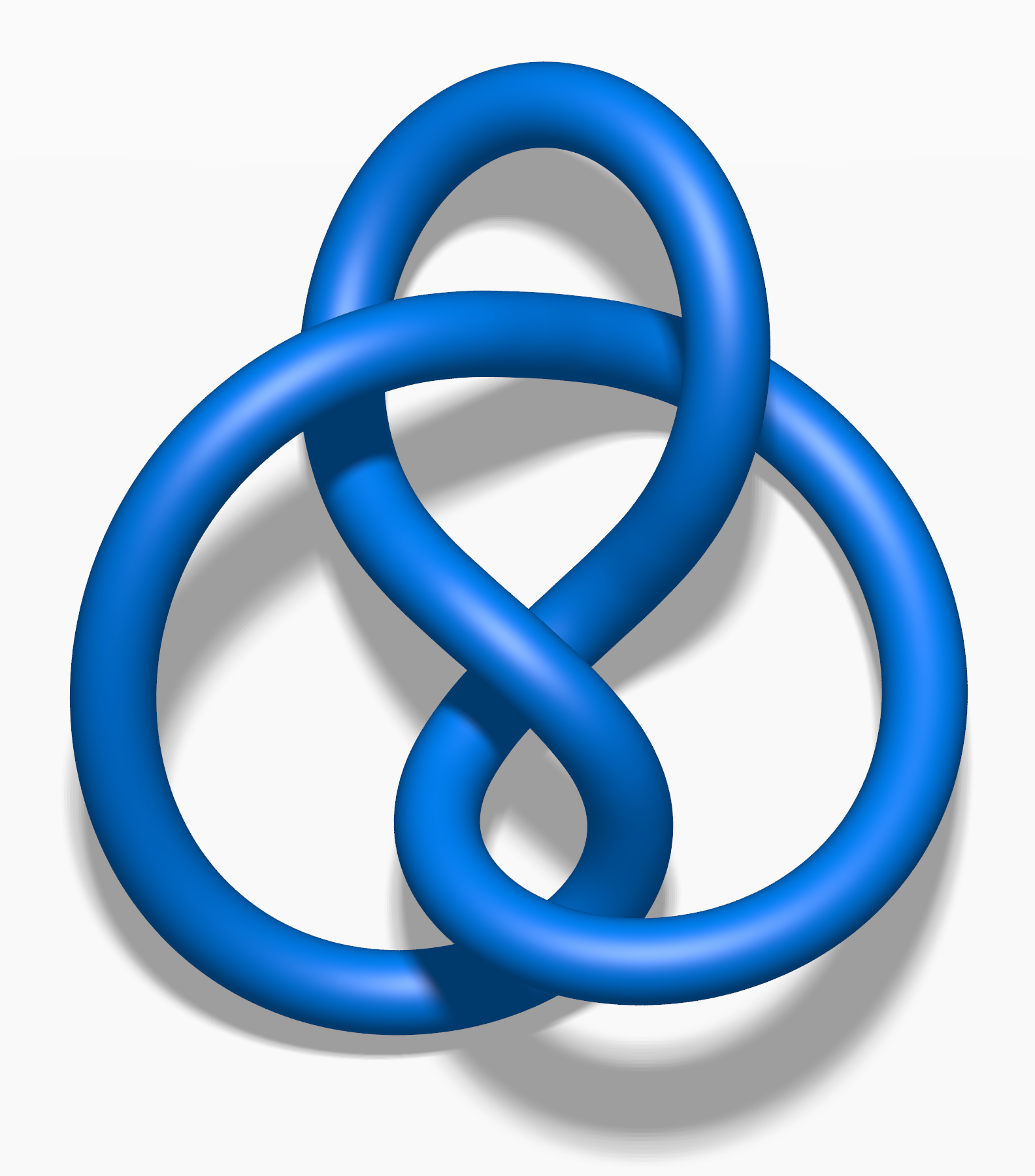
Figure-eight Knot (mathematics)
Figure 8 (figure of 8 in British English) may refer to: * 8 (number), in Arabic numerals Entertainment * ''Figure 8'' (album), a 2000 album by Elliott Smith * "Figure of Eight" (song), a 1989 song by Paul McCartney * ''Figure Eight EP'', a 2008 EP by This Et Al * "Figure 8" (song), a 2012 song by Ellie Goulding from ''Halcyon'' * "Figure Eight", an episode and song from the children's educational series ''Schoolhouse Rock!'' * "Figure of Eight", song by Status Quo from ''In Search of the Fourth Chord'' * "Figure 8", a song by FKA Twigs from the EP '' M3LL155X'' Geography * Figure Eight Island, North Carolina, United States * Figure Eight Lake, Alberta, Canada * Figure-Eight Loops, feature of the Historic Columbia River Highway in Guy W. Talbot State Park Mathematics and sciences * Figure-eight knot (mathematics), in knot theory * ∞, symbol meaning infinity * Lemniscate, various types of mathematical curve that resembles a figure 8 * Figure 8, a two-lobed Lissajo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Massachusetts Institute Of Technology School Of Science Alumni
Massachusetts (Massachusett language, Massachusett: ''Muhsachuweesut [Massachusett writing systems, məhswatʃəwiːsət],'' English: , ), officially the Commonwealth of Massachusetts, is the most populous U.S. state, state in the New England region of the Northeastern United States. It borders on the Atlantic Ocean and Gulf of Maine to the east, Connecticut and Rhode Island to the south, New Hampshire and Vermont to the north, and New York (state), New York to the west. The state's capital and List of municipalities in Massachusetts, most populous city, as well as its cultural and financial center, is Boston. Massachusetts is also home to the urban area, urban core of Greater Boston, the largest metropolitan area in New England and a region profoundly influential upon American History of the United States, history, academia, and the Economy of the United States, research economy. Originally dependent on agriculture, fishing, and trade. Massachusetts was transformed into a manuf ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
University Of Wisconsin–Madison College Of Letters And Science Alumni
A university () is an institution of higher (or tertiary) education and research which awards academic degrees in several academic disciplines. ''University'' is derived from the Latin phrase ''universitas magistrorum et scholarium'', which roughly means "community of teachers and scholars". Universities typically offer both undergraduate and postgraduate programs. The first universities in Europe were established by Catholic Church monks. The University of Bologna (), Italy, which was founded in 1088, is the first university in the sense of: *being a high degree-awarding institute. *using the word ''universitas'' (which was coined at its foundation). *having independence from the ecclesiastic schools and issuing secular as well as non-secular degrees (with teaching conducted by both clergy and non-clergy): grammar, rhetoric, logic, theology, canon law, notarial law.Hunt Janin: "The university in medieval life, 1179–1499", McFarland, 2008, , p. 55f.de Ridder-Symoens, Hil ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
21st-century American Mathematicians
The 1st century was the century spanning AD 1 (Roman numerals, I) through AD 100 (Roman numerals, C) according to the Julian calendar. It is often written as the or to distinguish it from the 1st century BC (or BCE) which preceded it. The 1st century is considered part of the Classical era, epoch, or History by period, historical period. The 1st century also saw the Christianity in the 1st century, appearance of Christianity. During this period, Europe, North Africa and the Near East fell under increasing domination by the Roman Empire, which continued expanding, most notably conquering Britain under the emperor Claudius (AD 43). The reforms introduced by Augustus during his long reign stabilized the empire after the turmoil of the previous century's civil wars. Later in the century the Julio-Claudian dynasty, which had been founded by Augustus, came to an end with the suicide of Nero in AD 68. There followed the famous Year of Four Emperors, a brief period of civil war and inst ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Living People
Related categories * :Year of birth missing (living people) / :Year of birth unknown * :Date of birth missing (living people) / :Date of birth unknown * :Place of birth missing (living people) / :Place of birth unknown * :Year of death missing / :Year of death unknown * :Date of death missing / :Date of death unknown * :Place of death missing / :Place of death unknown * :Missing middle or first names See also * :Dead people * :Template:L, which generates this category or death years, and birth year and sort keys. : {{DEFAULTSORT:Living people 21st-century people People by status ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
1956 Births
Events January * January 1 – The Anglo-Egyptian Condominium ends in Sudan. * January 8 – Operation Auca: Five U.S. evangelical Christian missionaries, Nate Saint, Roger Youderian, Ed McCully, Jim Elliot and Pete Fleming, are killed for trespassing by the Huaorani people of Ecuador, shortly after making contact with them. * January 16 – Egyptian leader Gamal Abdel Nasser vows to reconquer Palestine. * January 25– 26 – Finnish troops reoccupy Porkkala, after Soviet troops vacate its military base. Civilians can return February 4. * January 26 – The 1956 Winter Olympics open in Cortina d'Ampezzo, Italy. February * February 11 – British spies Guy Burgess and Donald Maclean resurface in the Soviet Union, after being missing for 5 years. * February 14– 25 – The 20th Congress of the Communist Party of the Soviet Union is held in Moscow. * February 16 – The 1956 World Figure Skating Championships open in Garmisch, West Germany. * February 22 – Elvis P ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Abigail Thompson
Abigail A. Thompson (born 1958 in Norwalk, Connecticut) is an American mathematician. She works as a professor of mathematics at the University of California, Davis, where she specializes in knot theory and low-dimensional topology. Education and career Thompson graduated from Wellesley College in 1979, and earned her Ph.D. in 1986 from Rutgers University under the joint supervision of Martin Scharlemann and Julius L. Shaneson. After visiting positions at the Hebrew University of Jerusalem and the University of California, Berkeley, she joined the University of California Davis faculty in 1988. Thompson had a postdoctoral fellowship with the National Science Foundation from 1988 to 1991 and a Sloan Foundation Fellowship from 1991 to 1993. She was a member of the Institute for Advanced Study in 1990-1991, 2000-2001, and 2015-2016. She became the Chair of the Department of Mathematics at UC Davis in 2017. She is one of the current vice presidents of the American Mathematical Soc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Joel Hass
Joel Hass is an American mathematician and a professor of mathematics and at the University of California, Davis.Faculty profile an math department contact information UC Davis, retrieved 2012-07-03. His work focuses on geometric and topological problems in dimension 3. Biography Hass received his from the in 1981 under the supervision of |
Hyperbolic Link
In mathematics, a hyperbolic link is a link in the 3-sphere with complement that has a complete Riemannian metric of constant negative curvature, i.e. has a hyperbolic geometry. A hyperbolic knot is a hyperbolic link with one component. As a consequence of the work of William Thurston, it is known that every knot is precisely one of the following: hyperbolic, a torus knot, or a satellite knot. As a consequence, hyperbolic knots can be considered plentiful. A similar heuristic applies to hyperbolic links. As a consequence of Thurston's hyperbolic Dehn surgery theorem, performing Dehn surgeries on a hyperbolic link enables one to obtain many more hyperbolic 3-manifolds. Examples *Borromean rings are hyperbolic. *Every non-split, prime, alternating link that is not a torus link is hyperbolic by a result of William Menasco. * 41 knot (the figure-eight knot) * 52 knot (the three-twist knot) * 61 knot (the stevedore knot) * 62 knot * 63 knot * 74 knot * 10 161 knot (the "Pe ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |