|
B-tree
In computer science, a B-tree is a self-balancing tree data structure that maintains sorted data and allows searches, sequential access, insertions, and deletions in logarithmic time. The B-tree generalizes the binary search tree, allowing for nodes with more than two children. Unlike other self-balancing binary search trees, the B-tree is well suited for storage systems that read and write relatively large blocks of data, such as databases and file systems. Origin B-trees were invented by Rudolf Bayer and Edward M. McCreight while working at Boeing Research Labs, for the purpose of efficiently managing index pages for large random-access files. The basic assumption was that indices would be so voluminous that only small chunks of the tree could fit in main memory. Bayer and McCreight's paper, ''Organization and maintenance of large ordered indices'', was first circulated in July 1970 and later published in '' Acta Informatica''. Bayer and McCreight never explained what, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
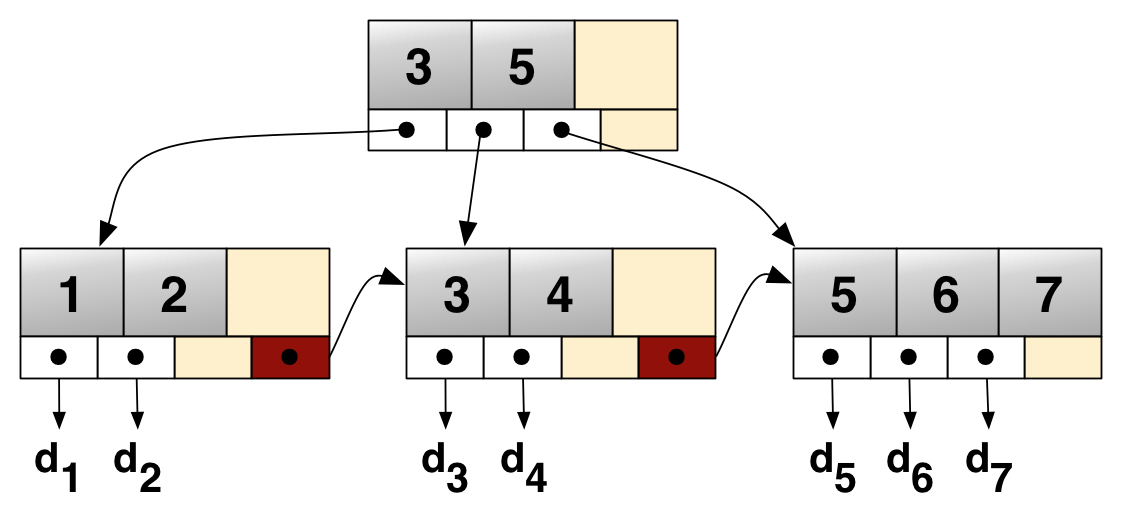
B+ Tree
A B+ tree is an m-ary tree with a variable but often large number of children per node. A B+ tree consists of a root, internal nodes and leaves. The root may be either a leaf or a node with two or more children. A B+ tree can be viewed as a B-tree in which each node contains only keys (not key–value pairs), and to which an additional level is added at the bottom with linked leaves. The primary value of a B+ tree is in storing data for efficient retrieval in a block-oriented storage context — in particular, filesystems. This is primarily because unlike binary search trees, B+ trees have very high fanout (number of pointers to child nodes in a node, typically on the order of 100 or more), which reduces the number of I/O operations required to find an element in the tree. History There is no single paper introducing the B+ tree concept. Instead, the notion of maintaining all data in leaf nodes is repeatedly brought up as an interesting variant. Douglas Comer notes in an ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rudolf Bayer
Rudolf Bayer (born 3 March 1939) is a German computer scientist. He is professor emeritus of Informatics at the Technical University of Munich where he had been employed since 1972. He is noted for inventing three data sorting structures: the B-tree (with Edward M. McCreight), the UB-tree (with Volker Markl) and the red–black tree. Bayer is a recipient of 2001 ACM SIGMOD Edgar F. Codd Innovations Award. In 2005 he was elected as a fellow of the Gesellschaft für Informatik The German Informatics Society (GI) (german: Gesellschaft für Informatik) is a German professional society for computer science, with around 20,000 personal and 250 corporate members. It is the biggest organized representation of its kind in the .... retrieved 2012-03-09. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
2–3 Tree
In computer science, a 2–3 tree is a tree data structure, where every node with children (internal node) has either two children (2-node) and one data element or three children (3-nodes) and two data elements. A 2–3 tree is a B-tree of order 3. Nodes on the outside of the tree (leaf nodes) have no children and one or two data elements. 2–3 trees were invented by John Hopcroft in 1970. 2–3 trees are required to be balanced, meaning that each leaf is at the same level. It follows that each right, center, and left subtree of a node contains the same or close to the same amount of data. Definitions We say that an internal node is a 2-node if it has ''one'' data element and ''two'' children. We say that an internal node is a 3-node if it has ''two'' data elements and ''three'' children. A 4-node, with three data elements, may be temporarily created during manipulation of the tree but is never persistently stored in the tree. Image:2-3-4 tree 2-node.svg, 2 node Image:2-3-4-t ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Binary Search
In computer science, binary search, also known as half-interval search, logarithmic search, or binary chop, is a search algorithm that finds the position of a target value within a sorted array. Binary search compares the target value to the middle element of the array. If they are not equal, the half in which the target cannot lie is eliminated and the search continues on the remaining half, again taking the middle element to compare to the target value, and repeating this until the target value is found. If the search ends with the remaining half being empty, the target is not in the array. Binary search runs in logarithmic time in the worst case, making O(\log n) comparisons, where n is the number of elements in the array. Binary search is faster than linear search except for small arrays. However, the array must be sorted first to be able to apply binary search. There are specialized data structures designed for fast searching, such as hash tables, that can be searched ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Order Statistic Tree
In computer science, an order statistic tree is a variant of the binary search tree (or more generally, a B-tree) that supports two additional operations beyond insertion, lookup and deletion: * Select(''i'') – find the ''ith smallest element stored in the tree * Rank(''x'') – find the rank of element ''x'' in the tree, i.e. its index in the sorted list of elements of the tree Both operations can be performed in worst case time when a self-balancing tree is used as the base data structure. Augmented search tree implementation To turn a regular search tree into an order statistic tree, the nodes of the tree need to store one additional value, which is the size of the subtree rooted at that node (i.e., the number of nodes below it). All operations that modify the tree must adjust this information to preserve the invariant that size = size eft[x + size[right[x + 1 where size[nil">">eft[x<_a>_+_size[right[x.html" ;"title=".html" ;"title="eft[x">eft[x + size[ri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Database
In computing, a database is an organized collection of data stored and accessed electronically. Small databases can be stored on a file system, while large databases are hosted on computer clusters or cloud storage. The design of databases spans formal techniques and practical considerations, including data modeling, efficient data representation and storage, query languages, security and privacy of sensitive data, and distributed computing issues, including supporting concurrent access and fault tolerance. A database management system (DBMS) is the software that interacts with end users, applications, and the database itself to capture and analyze the data. The DBMS software additionally encompasses the core facilities provided to administer the database. The sum total of the database, the DBMS and the associated applications can be referred to as a database system. Often the term "database" is also used loosely to refer to any of the DBMS, the database system or an appli ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Index (database)
A database index is a data structure that improves the speed of data retrieval operations on a database table at the cost of additional writes and storage space to maintain the index data structure. Indexes are used to quickly locate data without having to search every row in a database table every time a database table is accessed. Indexes can be created using one or more columns of a database table, providing the basis for both rapid random lookups and efficient access of ordered records. An index is a copy of selected columns of data, from a table, that is designed to enable very efficient search. An index normally includes a "key" or direct link to the original row of data from which it was copied, to allow the complete row to be retrieved efficiently. Some databases extend the power of indexing by letting developers create indexes on column values that have been transformed by functions or expressions. For example, an index could be created on upper(last_name), which wou ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Relational Database
A relational database is a (most commonly digital) database based on the relational model of data, as proposed by E. F. Codd in 1970. A system used to maintain relational databases is a relational database management system (RDBMS). Many relational database systems are equipped with the option of using the SQL (Structured Query Language) for querying and maintaining the database. History The term "relational database" was first defined by E. F. Codd at IBM in 1970. Codd introduced the term in his research paper "A Relational Model of Data for Large Shared Data Banks". In this paper and later papers, he defined what he meant by "relational". One well-known definition of what constitutes a relational database system is composed of Codd's 12 rules. However, no commercial implementations of the relational model conform to all of Codd's rules, so the term has gradually come to describe a broader class of database systems, which at a minimum: # Present the data to the user as rel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Self-balancing Binary Search Tree
In computer science, a self-balancing binary search tree (BST) is any node-based binary search tree that automatically keeps its height (maximal number of levels below the root) small in the face of arbitrary item insertions and deletions.Donald Knuth. ''The Art of Computer Programming'', Volume 3: ''Sorting and Searching'', Second Edition. Addison-Wesley, 1998. . Section 6.2.3: Balanced Trees, pp.458–481. These operations when designed for a self-balancing binary search tree, contain precautionary measures against boundlessly increasing tree height, so that these abstract data structures receive the attribute "self-balancing". For height-balanced binary trees, the height is defined to be logarithmic \mathcal O(\log n) in the number n of items. This is the case for many binary search trees, such as AVL trees and red–black trees. Splay trees and treaps are self-balancing but not height-balanced, as their height is not guaranteed to be logarithmic in the number of items. S ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Binary Search Tree
In computer science, a binary search tree (BST), also called an ordered or sorted binary tree, is a rooted binary tree data structure with the key of each internal node being greater than all the keys in the respective node's left subtree and less than the ones in its right subtree. The time complexity of operations on the binary search tree is directly proportional to the height of the tree. Binary search trees allow binary search for fast lookup, addition, and removal of data items. Since the nodes in a BST are laid out so that each comparison skips about half of the remaining tree, the lookup performance is proportional to that of binary logarithm. BSTs were devised in the 1960s for the problem of efficient storage of labeled data and are attributed to Conway Berners-Lee and David Wheeler. The performance of a binary search tree is dependent on the order of insertion of the nodes into the tree since arbitrary insertions may lead to degeneracy; several variations of th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Internal Node
In computer science, a tree is a widely used abstract data type that represents a hierarchical tree structure with a set of connected nodes. Each node in the tree can be connected to many children (depending on the type of tree), but must be connected to exactly one parent, except for the ''root'' node, which has no parent. These constraints mean there are no cycles or "loops" (no node can be its own ancestor), and also that each child can be treated like the root node of its own subtree, making recursion a useful technique for tree traversal. In contrast to linear data structures, many trees cannot be represented by relationships between neighboring nodes in a single straight line. Binary trees are a commonly used type, which constrain the number of children for each parent to exactly two. When the order of the children is specified, this data structure corresponds to an ordered tree in graph theory. A value or pointer to other data may be associated with every node in the tr ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
.svg "Click for more on -> Internal Node")