|
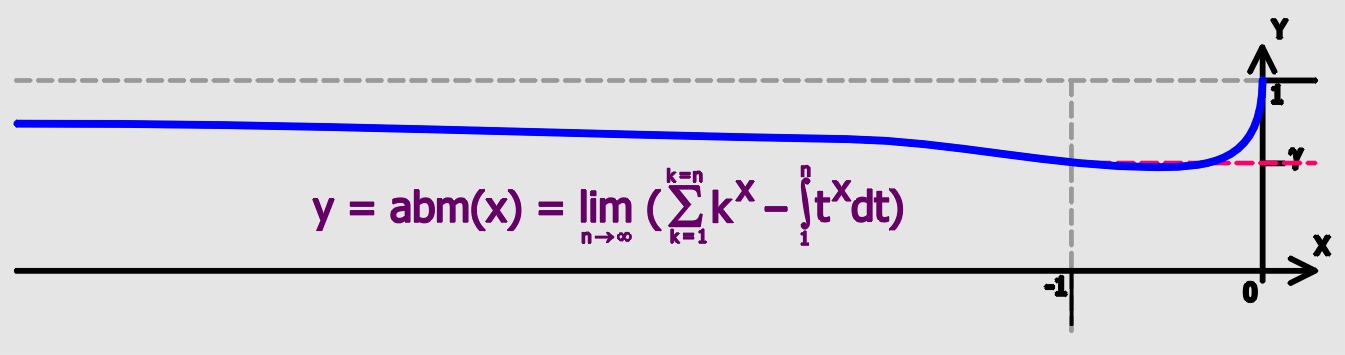
Buchstab Function
The Buchstab function (or Buchstab's function) is the unique continuous function \omega: \R_\rightarrow \R_ defined by the delay differential equation :\omega(u)=\frac 1 u, \qquad\qquad\qquad 1\le u\le 2, : (u\omega(u))=\omega(u-1), \qquad u\ge 2. In the second equation, the derivative at ''u'' = 2 should be taken as ''u'' approaches 2 from the right. It is named after Alexander Buchstab, who wrote about it in 1937. Asymptotics The Buchstab function approaches e^ \approx 0.561 rapidly as u\to\infty, where \gamma is the Euler–Mascheroni constant. In fact, :, \omega(u)-e^, \le \frac, \qquad u\ge 1, where ''ρ'' is the Dickman function. Also, \omega(u)-e^ oscillates in a regular way, alternating between extrema and zeroes; the extrema alternate between positive maxima and negative minima. The interval between consecutive extrema approaches 1 as ''u'' approaches infinity, as does the interval between consecutive zeroes.p. 131, Cheer and Goldston 1990. Applications The ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Delay Differential Equation
In mathematics, delay differential equations (DDEs) are a type of differential equation in which the derivative of the unknown function at a certain time is given in terms of the values of the function at previous times. DDEs are also called time-delay systems, systems with aftereffect or dead-time, hereditary systems, equations with deviating argument, or differential-difference equations. They belong to the class of systems with the functional state, i.e. partial differential equations (PDEs) which are infinite dimensional, as opposed to ordinary differential equations (ODEs) having a finite dimensional state vector. Four points may give a possible explanation of the popularity of DDEs: # Aftereffect is an applied problem: it is well known that, together with the increasing expectations of dynamic performances, engineers need their models to behave more like the real process. Many processes include aftereffect phenomena in their inner dynamics. In addition, actuators, sensor ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Alexander Buchstab
Aleksandr Adol'fovich Buchstab (October 4, 1905 – February 27, 1990;. russian: Александр Адольфович Бухштаб, variously transliterated as Bukhstab, Buhštab, or Bukhshtab) was a Soviet mathematician who worked in number theory and was "known for his work in sieve methods". He is the namesake of the Buchstab function, which he wrote about in 1937. Buchstab was born in Stavropol; his father was a physician. He studied at the Rostov Polytechnic Institute and Rostov University before moving to the faculty of mechanics and mathematics at Moscow State University, where he earned a degree in 1928. He worked at the Moscow Higher Technical College from 1928 until 1930, and then from 1930 to 1939 at Azerbaijan University, where was the chair of algebra and function theory and then dean of physics and mathematics. During this period, Buchstab also did graduate studies at Moscow State under the supervision of Aleksandr Khinchin. He defended his candidacy in ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Euler–Mascheroni Constant
Euler's constant (sometimes also called the Euler–Mascheroni constant) is a mathematical constant usually denoted by the lowercase Greek letter gamma (). It is defined as the limiting difference between the harmonic series and the natural logarithm, denoted here by \log: :\begin \gamma &= \lim_\left(-\log n + \sum_^n \frac1\right)\\ px&=\int_1^\infty\left(-\frac1x+\frac1\right)\,dx. \end Here, \lfloor x\rfloor represents the floor function. The numerical value of Euler's constant, to 50 decimal places, is: : History The constant first appeared in a 1734 paper by the Swiss mathematician Leonhard Euler, titled ''De Progressionibus harmonicis observationes'' (Eneström Index 43). Euler used the notations and for the constant. In 1790, Italian mathematician Lorenzo Mascheroni used the notations and for the constant. The notation appears nowhere in the writings of either Euler or Mascheroni, and was chosen at a later time perhaps because of the constant's connect ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Dickman Function
In analytic number theory, the Dickman function or Dickman–de Bruijn function ''ρ'' is a special function used to estimate the proportion of smooth numbers up to a given bound. It was first studied by actuary Karl Dickman, who defined it in his only mathematical publication, which is not easily available, and later studied by the Dutch mathematician Nicolaas Govert de Bruijn. Definition The Dickman–de Bruijn function \rho(u) is a continuous function that satisfies the delay differential equation :u\rho'(u) + \rho(u-1) = 0\, with initial conditions \rho(u) = 1 for 0 ≤ ''u'' ≤ 1. Properties Dickman proved that, when a is fixed, we have :\Psi(x, x^)\sim x\rho(a)\, where \Psi(x,y) is the number of ''y''- smooth (or ''y''-friable) integers below ''x''. Ramaswami later gave a rigorous proof that for fixed ''a'', \Psi(x,x^) was asymptotic to x \rho(a), with the error bound :\Psi(x,x^)=x\rho(a)+O(x/\log x) in big O notation. Applications ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Rough Number
A ''k''-rough number, as defined by Finch in 2001 and 2003, is a positive integer whose prime factors are all greater than or equal to ''k''. ''k''-roughness has alternately been defined as requiring all prime factors to strictly exceed ''k''.p. 130, Naccache and Shparlinski 2009. Examples (after Finch) #Every odd positive integer is 3-rough. #Every positive integer that is congruent to 1 or 5 mod 6 is 5-rough. #Every positive integer is 2-rough, since all its prime factors, being prime numbers, exceed 1. See also * Buchstab function, used to count rough numbers * Smooth number Notes References * Finch's definition from Number Theory Archives* "Divisibility, Smoothness and Cryptographic Applications", D. Naccache and I. E. Shparlinski, pp. 115–173 in ''Algebraic Aspects of Digital Communications'', eds. Tanush Shaska and Engjell Hasimaj, IOS Press, 2009, . The On-Line Encyclopedia of Integer Sequences The On-Line Encyclopedia of Integer Sequences (OEIS) is an online ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Matematicheskii Sbornik
''Matematicheskii Sbornik'' (russian: Математический сборник, abbreviated ''Mat. Sb.'') is a peer reviewed Russian mathematical journal founded by the Moscow Mathematical Society in 1866. It is the oldest successful Russian mathematical journal. The English translation is ''Sbornik: Mathematics''. It is also sometimes cited under the alternative name ''Izdavaemyi Moskovskim Matematicheskim Obshchestvom'' or its French translation ''Recueil mathématique de la Société mathématique de Moscou'', but the name ''Recueil mathématique'' is also used for an unrelated journal, '' Mathesis''. Yet another name, ''Sovetskii Matematiceskii Sbornik'', was listed in a statement in the journal in 1931 apologizing for the former editorship of Dmitri Egorov, who had been recently discredited for his religious views; however, this name was never actually used by the journal. The first editor of the journal was Nikolai Brashman, who died before its first issue (dedicated to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Wolfram MathWorld
''MathWorld'' is an online mathematics reference work, created and largely written by Eric W. Weisstein. It is sponsored by and licensed to Wolfram Research, Inc. and was partially funded by the National Science Foundation's National Science Digital Library grant to the University of Illinois at Urbana–Champaign. History Eric W. Weisstein, the creator of the site, was a physics and astronomy student who got into the habit of writing notes on his mathematical readings. In 1995 he put his notes online and called it "Eric's Treasure Trove of Mathematics." It contained hundreds of pages/articles, covering a wide range of mathematical topics. The site became popular as an extensive single resource on mathematics on the web. Weisstein continuously improved the notes and accepted corrections and comments from online readers. In 1998, he made a contract with CRC Press and the contents of the site were published in print and CD-ROM form, titled "CRC Concise Encyclopedia of Mathem ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Springer Science+Business Media
Springer Science+Business Media, commonly known as Springer, is a German multinational publishing company of books, e-books and peer-reviewed journals in science, humanities, technical and medical (STM) publishing. Originally founded in 1842 in Berlin, it expanded internationally in the 1960s, and through mergers in the 1990s and a sale to venture capitalists it fused with Wolters Kluwer and eventually became part of Springer Nature in 2015. Springer has major offices in Berlin, Heidelberg, Dordrecht, and New York City. History Julius Springer founded Springer-Verlag in Berlin in 1842 and his son Ferdinand Springer grew it from a small firm of 4 employees into Germany's then second largest academic publisher with 65 staff in 1872.Chronology ". Springer Science+Business Media. In 1964, Springer expanded its business internationally, o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematics Of Computation
''Mathematics of Computation'' is a bimonthly mathematics journal focused on computational mathematics. It was established in 1943 as ''Mathematical Tables and other Aids to Computation'', obtaining its current name in 1960. Articles older than five years are available electronically free of charge. Abstracting and indexing The journal is abstracted and indexed in Mathematical Reviews ''Mathematical Reviews'' is a journal published by the American Mathematical Society (AMS) that contains brief synopses, and in some cases evaluations, of many articles in mathematics, statistics, and theoretical computer science. The AMS also pu ..., Zentralblatt MATH, Science Citation Index, CompuMath Citation Index, and Current Contents/Physical, Chemical & Earth Sciences. According to the '' Journal Citation Reports'', the journal has a 2020 impact factor of 2.417. References External links * Delayed open access journals English-language journals Mathematics journals Public ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Acta Arithmetica
''Acta Arithmetica'' is a scientific journal of mathematics publishing papers on number theory Number theory (or arithmetic or higher arithmetic in older usage) is a branch of pure mathematics devoted primarily to the study of the integers and integer-valued functions. German mathematician Carl Friedrich Gauss (1777–1855) said, "Math .... It was established in 1935 by Salomon Lubelski and Arnold Walfisz. The journal is published by the Institute of Mathematics of the Polish Academy of Sciences. References External links Online archives(Library of Science, Issues: 1935–2000) 1935 establishments in Poland Mathematics journals Publications established in 1935 Polish Academy of Sciences academic journals Multilingual journals Biweekly journals Academic journals associated with learned and professional societies {{math-journal-stub ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Analytic Number Theory
In mathematics, analytic number theory is a branch of number theory that uses methods from mathematical analysis to solve problems about the integers. It is often said to have begun with Peter Gustav Lejeune Dirichlet's 1837 introduction of Dirichlet ''L''-functions to give the first proof of Dirichlet's theorem on arithmetic progressions. It is well known for its results on prime numbers (involving the Prime Number Theorem and Riemann zeta function) and additive number theory (such as the Goldbach conjecture and Waring's problem). Branches of analytic number theory Analytic number theory can be split up into two major parts, divided more by the type of problems they attempt to solve than fundamental differences in technique. * Multiplicative number theory deals with the distribution of the prime numbers, such as estimating the number of primes in an interval, and includes the prime number theorem and Dirichlet's theorem on primes in arithmetic progressions. * Additive n ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |