Stokes number on:
[Wikipedia]
[Google]
[Amazon]
 The Stokes number (Stk), named after
The Stokes number (Stk), named after
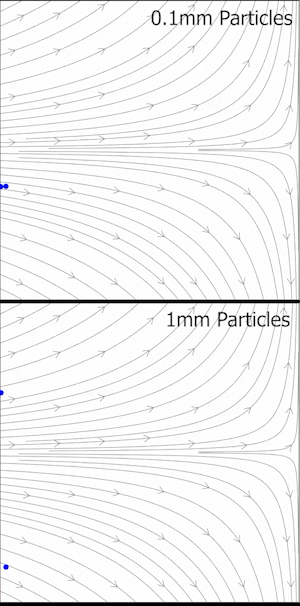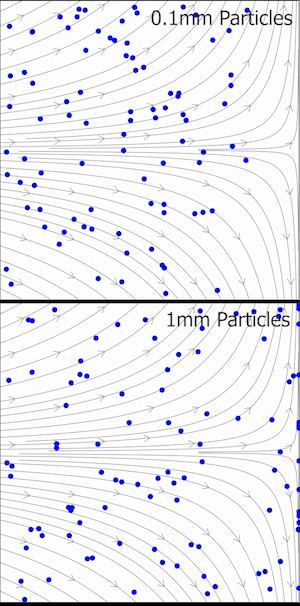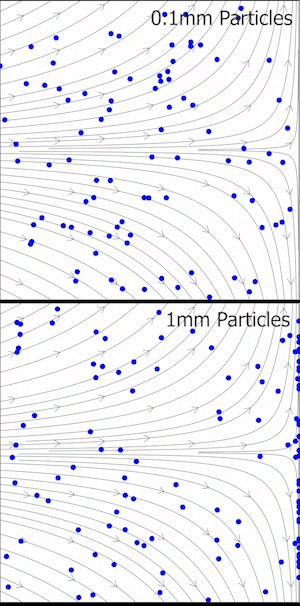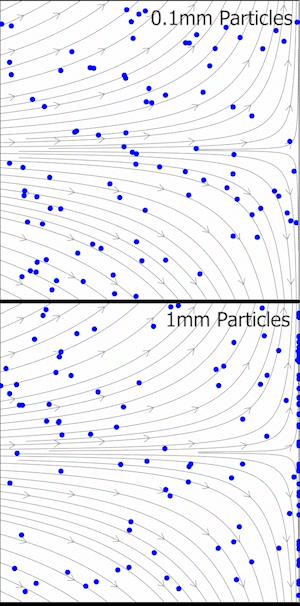 The Stokes number provides a means of estimating the quality of Particle Image Velocimetry data sets, as previously discussed. However, a definition of a characteristic velocity or length scale may not be evident in all applications. Thus, a deeper insight of how a tracking delay arises could be drawn by simply defining the differential equations of a particle in the Stokes regime. A particle moving with the fluid at some velocity will encounter a variable fluid velocity field as it advects. Let's assume the velocity of the fluid, in the Lagrangian frame of reference of the particle, is . It is the difference between these velocities that will generate the drag force necessary to correct the particle path:
:
The stokes drag force is then:
:
The particle mass is:
:
Thus, the particle acceleration can be found through Newton's second law:
:
Note the relaxation time can be replaced to yield:
:
The first-order differential equation above can be solved through the
The Stokes number provides a means of estimating the quality of Particle Image Velocimetry data sets, as previously discussed. However, a definition of a characteristic velocity or length scale may not be evident in all applications. Thus, a deeper insight of how a tracking delay arises could be drawn by simply defining the differential equations of a particle in the Stokes regime. A particle moving with the fluid at some velocity will encounter a variable fluid velocity field as it advects. Let's assume the velocity of the fluid, in the Lagrangian frame of reference of the particle, is . It is the difference between these velocities that will generate the drag force necessary to correct the particle path:
:
The stokes drag force is then:
:
The particle mass is:
:
Thus, the particle acceleration can be found through Newton's second law:
:
Note the relaxation time can be replaced to yield:
:
The first-order differential equation above can be solved through the 
 Considering the limiting particle free-stream Reynolds numbers, as then and therefore . Thus as expected there correction factor is unity in the Stokesian drag regime. Wessel & Righi evaluated for from the empirical correlation for drag on a sphere from Schiller & Naumann.
Where the constant . The conventional Stokes number will significantly underestimate the drag force for large particle free-stream Reynolds numbers. Thus overestimating the tendency for particles to depart from the fluid flow direction. This will lead to errors in subsequent calculations or experimental comparisons.
Considering the limiting particle free-stream Reynolds numbers, as then and therefore . Thus as expected there correction factor is unity in the Stokesian drag regime. Wessel & Righi evaluated for from the empirical correlation for drag on a sphere from Schiller & Naumann.
Where the constant . The conventional Stokes number will significantly underestimate the drag force for large particle free-stream Reynolds numbers. Thus overestimating the tendency for particles to depart from the fluid flow direction. This will lead to errors in subsequent calculations or experimental comparisons.
 The Stokes number (Stk), named after
The Stokes number (Stk), named after George Gabriel Stokes
Sir George Gabriel Stokes, 1st Baronet, (; 13 August 1819 – 1 February 1903) was an Irish migration to Great Britain, Irish English physicist and mathematician. Born in County Sligo, Ireland, Stokes spent all of his career at the University ...
, is a dimensionless number
A dimensionless quantity (also known as a bare quantity, pure quantity, or scalar quantity as well as quantity of dimension one) is a quantity to which no physical dimension is assigned, with a corresponding SI unit of measurement of one (or 1) ...
characterising the behavior of particles suspended in a fluid flow. The Stokes number is defined as the ratio of the characteristic time of a particle (or droplet
A drop or droplet is a small column of liquid, bounded completely or almost completely by free surfaces. A drop may form when liquid accumulates at the lower end of a tube or other surface boundary, producing a hanging drop called a pendant ...
) to a characteristic time of the flow or of an obstacle, or
:
where is the relaxation time
In the physical sciences, relaxation usually means the return of a perturbed system into equilibrium.
Each relaxation process can be categorized by a relaxation time τ. The simplest theoretical description of relaxation as function of time ' ...
of the particle (the time constant in the exponential decay of the particle velocity due to drag), is the fluid velocity of the flow well away from the obstacle and is the characteristic dimension of the obstacle (typically its diameter). A particle with a low Stokes number follows fluid streamlines (perfect advection
In the field of physics, engineering, and earth sciences, advection is the transport of a substance or quantity by bulk motion of a fluid. The properties of that substance are carried with it. Generally the majority of the advected substance is al ...
), while a particle with a large Stokes number is dominated by its inertia and continues along its initial trajectory.
In the case of Stokes flow, which is when the particle (or droplet) Reynolds number
In fluid mechanics, the Reynolds number () is a dimensionless quantity that helps predict fluid flow patterns in different situations by measuring the ratio between inertial and viscous forces. At low Reynolds numbers, flows tend to be domi ...
is less than unity, the particle drag coefficient
In fluid dynamics, the drag coefficient (commonly denoted as: c_\mathrm, c_x or c_) is a dimensionless quantity that is used to quantify the drag or resistance of an object in a fluid environment, such as air or water. It is used in the drag equ ...
is inversely proportional to the Reynolds number itself. In that case, the characteristic time of the particle can be written as
:
where is the particle density
Density (volumetric mass density or specific mass) is the substance's mass per unit of volume. The symbol most often used for density is ''ρ'' (the lower case Greek letter rho), although the Latin letter ''D'' can also be used. Mathematical ...
, is the particle diameter and is the fluid dynamic viscosity
The viscosity of a fluid is a measure of its resistance to deformation at a given rate. For liquids, it corresponds to the informal concept of "thickness": for example, syrup has a higher viscosity than water.
Viscosity quantifies the inter ...
.
In experimental fluid dynamics, the Stokes number is a measure of flow tracer fidelity in particle image velocimetry (PIV) experiments where very small particles are entrained in turbulent flows and optically observed to determine the speed and direction of fluid movement (also known as the velocity field
In continuum mechanics the flow velocity in fluid dynamics, also macroscopic velocity in statistical mechanics, or drift velocity in electromagnetism, is a vector field used to mathematically describe the motion of a continuum. The length of the f ...
of the fluid). For acceptable tracing accuracy, the particle response time should be faster than the smallest time scale of the flow. Smaller Stokes numbers represent better tracing accuracy; for , particles will detach from a flow especially where the flow decelerates abruptly. For , particles follow fluid streamlines closely. If , tracing accuracy errors are below 1%.
Relaxation time and tracking error in Particle Image Velocimetry
Laplace transform
In mathematics, the Laplace transform, named after its discoverer Pierre-Simon Laplace (), is an integral transform
In mathematics, an integral transform maps a function from its original function space into another function space via integra ...
method:
:
:
The solution above, in the frequency domain, characterizes a first-order system with a characteristic time of . Thus, the −3 dB gain (cut-off) frequency will be:
:
The cut-off frequency and the particle transfer function, plotted on the side panel, allows for the assessment of PIV error in unsteady flow applications and its effect on turbulence spectral quantities and kinetic energy.

Particles through a shock wave
The bias error in particle tracking discussed in the previous section is evident in the frequency domain, but it can be difficult to appreciate in cases where the particle motion is being tracked to perform flow field measurements (like in particle image velocimetry). A simple but insightful solution to the above-mentioned differential equation is possible when the forcing function is a Heaviside step function; representing particles going through a shockwave. In this case, is the flow velocity upstream of the shock; whereas is the velocity drop across the shock. The step response for a particle is a simple exponential: : To convert the velocity as a function of time to a particle velocity distribution as a function of distance, let's assume a 1-dimensional velocity jump in the direction. Let's assume is positioned where the shock wave is, and then integrate the previous equation to get: : : Considering a relaxation time of (time to 95% velocity change), we have: : : This means the particle velocity would be settled to within 5% of the downstream velocity at from the shock. In practice, this means a shock wave would look, to a PIV system, blurred by approximately this distance. For example, consider a normal shock wave ofMach number
Mach number (M or Ma) (; ) is a dimensionless quantity in fluid dynamics representing the ratio of flow velocity past a boundary to the local speed of sound.
It is named after the Moravian physicist and philosopher Ernst Mach.
: \mathrm = \frac ...
at a stagnation temperature of 298 K. A propyleneglycol particle of would blur the flow by ; whereas a would blur the flow by (which would, in most cases, yield unacceptable PIV results).
Although a shock wave is the worst-case scenario of abrupt deceleration of a flow, it illustrates the effect of particle tracking error in PIV, which results in a blurring of the velocity fields acquired at the length scales of order .
Non-Stokesian drag regime
The preceding analysis will not be accurate in the ultra-Stokesian regime. i.e. if the particle Reynolds number is much greater than unity. Assuming a Mach number much less than unity, a generalized form of the Stokes number was demonstrated by Israel & Rosner. Where is the "particle free-stream Reynolds number", An additional function was defined by; this describes the non-Stokesian drag correction factor, It follows that this function is defined by, Considering the limiting particle free-stream Reynolds numbers, as then and therefore . Thus as expected there correction factor is unity in the Stokesian drag regime. Wessel & Righi evaluated for from the empirical correlation for drag on a sphere from Schiller & Naumann.
Where the constant . The conventional Stokes number will significantly underestimate the drag force for large particle free-stream Reynolds numbers. Thus overestimating the tendency for particles to depart from the fluid flow direction. This will lead to errors in subsequent calculations or experimental comparisons.
Considering the limiting particle free-stream Reynolds numbers, as then and therefore . Thus as expected there correction factor is unity in the Stokesian drag regime. Wessel & Righi evaluated for from the empirical correlation for drag on a sphere from Schiller & Naumann.
Where the constant . The conventional Stokes number will significantly underestimate the drag force for large particle free-stream Reynolds numbers. Thus overestimating the tendency for particles to depart from the fluid flow direction. This will lead to errors in subsequent calculations or experimental comparisons.
Application to anisokinetic sampling of particles
For example, the selective capture of particles by an aligned, thin-walled circular nozzle is given by Belyaev and Levin as: : where is particle concentration, is speed, and the subscript 0 indicates conditions far upstream of the nozzle. The characteristic distance is the diameter of the nozzle. Here the Stokes number is calculated, : where is the particle's settling velocity, is the sampling tubes inner diameter, and is the acceleration of gravity.References
Further reading
* * * * {{NonDimFluMech Discrete-phase flow Aerosols Dimensionless numbers of fluid mechanics Fluid dynamics