|
Legendre's Theorem On Spherical Triangles
In geometry, Legendre's theorem on spherical triangles, named after Adrien-Marie Legendre, is stated as follows: : Let ABC be a spherical triangle on the ''unit'' sphere with ''small'' sides ''a'', ''b'', ''c''. Let A'B'C' be the planar triangle with the same sides. Then the angles of the spherical triangle exceed the corresponding angles of the planar triangle by approximately one third of the spherical excess (the spherical excess is the amount by which the sum of the three angles exceeds ). The theorem was very important in simplifying the heavy numerical work in calculating the results of traditional (pre-GPS and pre-computer) geodetic surveys from about 1800 until the middle of the twentieth century. The theorem was stated by who provided a proof (1798) in a supplement to the report of the measurement of the French meridional arc used in the definition of the metre . Legendre does not claim that he was the originator of the theorem despite the attribution to him. ma ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is called a '' geometer''. Until the 19th century, geometry was almost exclusively devoted to Euclidean geometry, which includes the notions of point, line, plane, distance, angle, surface, and curve, as fundamental concepts. During the 19th century several discoveries enlarged dramatically the scope of geometry. One of the oldest such discoveries is Carl Friedrich Gauss' ("remarkable theorem") that asserts roughly that the Gaussian curvature of a surface is independent from any specific embedding in a Euclidean space. This implies that surfaces can be studied ''intrinsically'', that is, as stand-alone spaces, and has been expanded into the theory of manifolds and Riemannian geometry. Later in the 19th century, it appeared that geom ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Adrien-Marie Legendre
Adrien-Marie Legendre (; ; 18 September 1752 – 9 January 1833) was a French mathematician who made numerous contributions to mathematics. Well-known and important concepts such as the Legendre polynomials and Legendre transformation are named after him. Life Adrien-Marie Legendre was born in Paris on 18 September 1752 to a wealthy family. He received his education at the Collège Mazarin in Paris, and defended his thesis in physics and mathematics in 1770. He taught at the École Militaire in Paris from 1775 to 1780 and at the École Normale from 1795. At the same time, he was associated with the Bureau des Longitudes. In 1782, the Berlin Academy awarded Legendre a prize for his treatise on projectiles in resistant media. This treatise also brought him to the attention of Lagrange. The '' Académie des sciences'' made Legendre an adjoint member in 1783 and an associate in 1785. In 1789, he was elected a Fellow of the Royal Society. He assisted with the Anglo-French ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
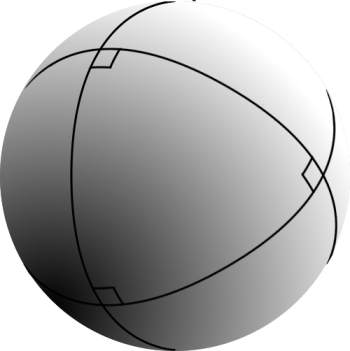
Spherical Excess
Spherical trigonometry is the branch of spherical geometry that deals with the metrical relationships between the sides and angles of spherical triangles, traditionally expressed using trigonometric functions. On the sphere, geodesics are great circles. Spherical trigonometry is of great importance for calculations in astronomy, geodesy, and navigation. The origins of spherical trigonometry in Greek mathematics and the major developments in Islamic mathematics are discussed fully in History of trigonometry and Mathematics in medieval Islam. The subject came to fruition in Early Modern times with important developments by John Napier, Delambre and others, and attained an essentially complete form by the end of the nineteenth century with the publication of Todhunter's textbook ''Spherical trigonometry for the use of colleges and Schools''. Since then, significant developments have been the application of vector methods, quaternion methods, and the use of numerical methods. P ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spherical Trigonometry Legendre
A sphere () is a geometrical object that is a three-dimensional analogue to a two-dimensional circle. A sphere is the set of points that are all at the same distance from a given point in three-dimensional space.. That given point is the centre of the sphere, and is the sphere's radius. The earliest known mentions of spheres appear in the work of the ancient Greek mathematicians. The sphere is a fundamental object in many fields of mathematics. Spheres and nearly-spherical shapes also appear in nature and industry. Bubbles such as soap bubbles take a spherical shape in equilibrium. The Earth is often approximated as a sphere in geography, and the celestial sphere is an important concept in astronomy. Manufactured items including pressure vessels and most curved mirrors and lenses are based on spheres. Spheres roll smoothly in any direction, so most balls used in sports and toys are spherical, as are ball bearings. Basic terminology As mentioned earlier is the sphere' ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Metre
The metre ( British spelling) or meter ( American spelling; see spelling differences) (from the French unit , from the Greek noun , "measure"), symbol m, is the primary unit of length in the International System of Units (SI), though its prefixed forms are also used relatively frequently. The metre was originally defined in 1793 as one ten-millionth of the distance from the equator to the North Pole along a great circle, so the Earth's circumference is approximately km. In 1799, the metre was redefined in terms of a prototype metre bar (the actual bar used was changed in 1889). In 1960, the metre was redefined in terms of a certain number of wavelengths of a certain emission line of krypton-86. The current definition was adopted in 1983 and modified slightly in 2002 to clarify that the metre is a measure of proper length. From 1983 until 2019, the metre was formally defined as the length of the path travelled by light in a vacuum in of a second. After the 2019 ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Charles Marie De La Condamine
Charles Marie de La Condamine (28 January 1701 – 4 February 1774) was a French explorer, geographer, and mathematician. He spent ten years in territory which is now Ecuador, measuring the length of a degree of latitude at the equator and preparing the first map of the Amazon region based on astro-geodetic observations. Furthermore he was a contributor to the ''Encyclopédie''.'' Biography Charles Marie de La Condamine was born in Paris as a son of well-to-do parents, Charles de La Condamine and Louise Marguerite Chourses. He studied at the Collège Louis-le-Grand where he was trained in humanities as well as in mathematics. After finishing his studies, he enlisted in the army and fought in the war against Spain (1719). After returning from the war, he became acquainted with scientific circles in Paris. On 12 December 1730 he became a member of the Académie des Sciences and was appointed Assistant Chemist at the Academy. In 1729 La Condamine and his friend Voltaire exploit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
French Geodesic Mission
The French Geodesic Mission to the Equator (french: Expédition géodésique française en Équateur, also called the French Geodesic Mission to Peru and the Spanish-French Geodesic Mission) was an 18th-century expedition to what is now Ecuador carried out for the purpose of performing an arc measurement, measuring the length of a degree of latitude near the Equator, by which the Earth radius can be inferred. The mission was one of the first geodesic (or ''geodetic'') missions carried out under modern scientific principles, and the first major international scientific expedition. Background In the 18th century, there was significant debate in the scientific community, specifically in the French Academy of Sciences (''Académie des sciences''), as to whether the circumference of the Earth was greater around the Equator or around the poles. French astronomer Jacques Cassini held to the view that the polar circumference was greater. Louis XV, the King of France and the academy s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Spherical Trigonometry
Spherical trigonometry is the branch of spherical geometry that deals with the metrical relationships between the sides and angles of spherical triangles, traditionally expressed using trigonometric functions. On the sphere, geodesics are great circles. Spherical trigonometry is of great importance for calculations in astronomy, geodesy, and navigation. The origins of spherical trigonometry in Greek mathematics and the major developments in Islamic mathematics are discussed fully in History of trigonometry and Mathematics in medieval Islam. The subject came to fruition in Early Modern times with important developments by John Napier, Delambre and others, and attained an essentially complete form by the end of the nineteenth century with the publication of Todhunter's textbook ''Spherical trigonometry for the use of colleges and Schools''. Since then, significant developments have been the application of vector methods, quaternion methods, and the use of numerical methods. ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |


.jpg)
