|
Tutte
William Thomas Tutte OC FRS FRSC (; 14 May 1917 – 2 May 2002) was an English and Canadian codebreaker and mathematician. During the Second World War, he made a brilliant and fundamental advance in cryptanalysis of the Lorenz cipher, a major Nazi German cipher system which was used for top-secret communications within the Wehrmacht High Command. The high-level, strategic nature of the intelligence obtained from Tutte's crucial breakthrough, in the bulk decrypting of Lorenz-enciphered messages specifically, contributed greatly, and perhaps even decisively, to the defeat of Nazi Germany. He also had a number of significant mathematical accomplishments, including foundation work in the fields of graph theory and matroid theory. Tutte's research in the field of graph theory proved to be of remarkable importance. At a time when graph theory was still a primitive subject, Tutte commenced the study of matroids and developed them into a theory by expanding from the work that Hassle ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte Polynomial
The Tutte polynomial, also called the dichromate or the Tutte–Whitney polynomial, is a graph polynomial. It is a polynomial in two variables which plays an important role in graph theory. It is defined for every undirected graph G and contains information about how the graph is connected. It is denoted by T_G. The importance of this polynomial stems from the information it contains about G. Though originally studied in algebraic graph theory as a generalization of counting problems related to graph coloring and nowhere-zero flow, it contains several famous other specializations from other sciences such as the Jones polynomial from knot theory and the partition functions of the Potts model from statistical physics. It is also the source of several central computational problems in theoretical computer science. The Tutte polynomial has several equivalent definitions. It is equivalent to Whitney’s rank polynomial, Tutte’s own dichromatic polynomial and Fortuin–Kasteley ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
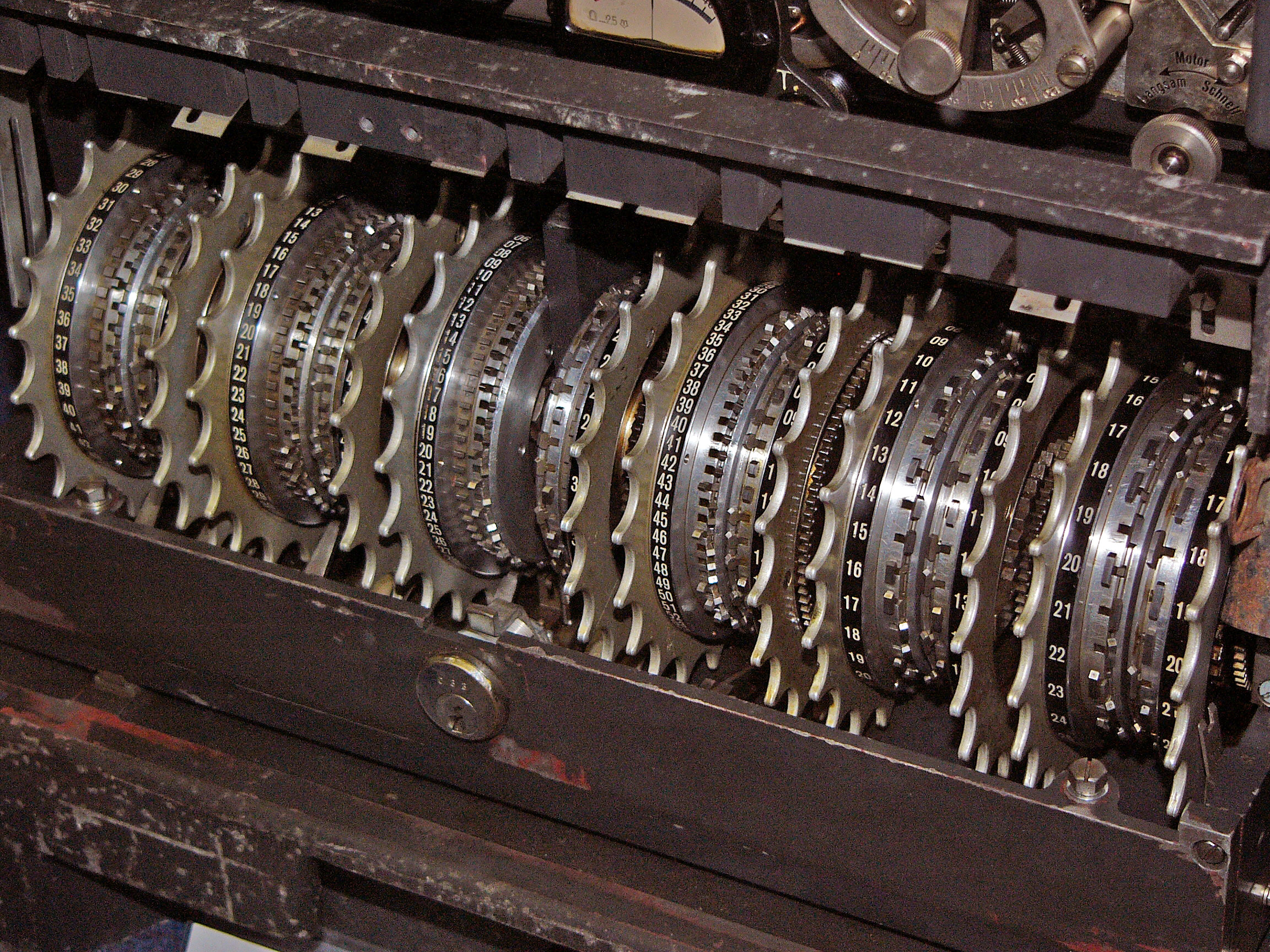
Cryptanalysis Of The Lorenz Cipher
Cryptanalysis of the Lorenz cipher was the process that enabled the British to read high-level German army messages during World War II. The British Government Code and Cypher School (GC&CS) at Bletchley Park decrypted many communications between the '' Oberkommando der Wehrmacht'' (OKW, German High Command) in Berlin and their army commands throughout occupied Europe, some of which were signed "Adolf Hitler, Führer". These were intercepted non-Morse radio transmissions that had been enciphered by the Lorenz SZ teleprinter rotor stream cipher attachments. Decrypts of this traffic became an important source of "Ultra" intelligence, which contributed significantly to Allied victory. For its high-level secret messages, the German armed services enciphered each character using various online ''Geheimschreiber'' (secret writer) stream cipher machines at both ends of a telegraph link using the 5-bit International Telegraphy Alphabet No. 2 (ITA2). These machines were subsequently ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte–Coxeter Graph
In the mathematical field of graph theory, the Tutte–Coxeter graph or Tutte eight-cage or Cremona–Richmond graph is a 3-regular graph with 30 vertices and 45 edges. As the unique smallest cubic graph of girth 8, it is a cage and a Moore graph. It is bipartite, and can be constructed as the Levi graph of the generalized quadrangle ''W''2 (known as the Cremona–Richmond configuration). The graph is named after William Thomas Tutte and H. S. M. Coxeter; it was discovered by Tutte (1947) but its connection to geometric configurations was investigated by both authors in a pair of jointly published papers (Tutte 1958; Coxeter 1958a). All the cubic distance-regular graphs are known. The Tutte–Coxeter is one of the 13 such graphs. It has crossing number 13, book thickness 3 and queue number 2.Wolz, Jessica; ''Engineering Linear Layouts with SAT.'' Master Thesis, University of Tübingen, 2018 Constructions and automorphisms A particularly simple combinatorial constructio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte Embedding
In graph drawing and geometric graph theory, a Tutte embedding or barycentric embedding of a simple, 3-vertex-connected, planar graph is a crossing-free straight-line embedding with the properties that the outer face is a convex polygon and that each interior vertex is at the average (or barycenter) of its neighbors' positions. If the outer polygon is fixed, this condition on the interior vertices determines their position uniquely as the solution to a system of linear equations. Solving the equations geometrically produces a planar embedding. Tutte's spring theorem, proven by , states that this unique solution is always crossing-free, and more strongly that every face of the resulting planar embedding is convex. It is called the spring theorem because such an embedding can be found as the equilibrium position for a system of springs representing the edges of the graph. Example Let ''G'' be the graph of a cube, and (selecting one of its quadrilateral faces as the outer face) ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte Graph
In the mathematical field of graph theory, the Tutte graph is a 3-regular graph with 46 vertices and 69 edges named after W. T. Tutte. It has chromatic number 3, chromatic index 3, girth 4 and diameter 8. The Tutte graph is a cubic polyhedral graph, but is non- hamiltonian. Therefore, it is a counterexample to Tait's conjecture that every 3-regular polyhedron has a Hamiltonian cycle. Published by Tutte in 1946, it is the first counterexample constructed for this conjecture. Other counterexamples were found later, in many cases based on Grinberg's theorem. Construction From a small planar graph called the Tutte fragment, W. T. Tutte constructed a non-Hamiltonian polyhedron, by putting together three such fragments. The "compulsory" edges of the fragments, that must be part of any Hamiltonian path through the fragment, are connected at the central vertex; because any cycle can use only two of these three edges, there can be no Hamiltonian cycle. The resulting graph is 3-con ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte 12-cage
In the mathematical field of graph theory, the Tutte 12-cage or Benson graph is a 3-regular graph with 126 vertices and 189 edges named after W. T. Tutte. The Tutte 12-cage is the unique (3-12)- cage . It was discovered by C. T. Benson in 1966. It has chromatic number 2 (bipartite), chromatic index 3, girth 12 (as a 12-cage) and diameter 6. Its crossing number is 170 and has been conjectured to be the smallest cubic graph with this crossing number. Construction The Tutte 12-cage is a cubic Hamiltonian graph and can be defined by the LCF notation 7, 27, –13, –59, –35, 35, –11, 13, –53, 53, –27, 21, 57, 11, –21, –57, 59, –17sup>7. There are, up to isomorphism, precisely two generalized hexagons of order ''(2,2)'' as proved by Cohen and Tits. They are the split Cayley hexagon ''H(2)'' and its point-line dual. Clearly both of them have the same incidence graph, which is in fact isomorphic to the Tutte 12-cage. The Balaban ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte Theorem
In the mathematical discipline of graph theory the Tutte theorem, named after William Thomas Tutte, is a characterization of finite graphs with perfect matchings. It is a generalization of Hall's marriage theorem from bipartite to arbitrary graphs. It is a special case of the Tutte–Berge formula. Intuition Our goal is to characterize all graphs that do not have a perfect matching. Let us start with the most obvious case of a graph without a perfect matching: a graph with an odd number of vertices. In such a graph, any matching leaves at least one unmatched vertex, so it cannot be perfect. A slightly more general case is a disconnected graph in which one or more components have an odd number of vertices (even if the total number of vertices is even). Let us call such components ''odd components''. In any matching, each vertex can only be matched to vertices in the same component. Therefore, any matching leaves at least one unmatched vertex in every odd component, so it can ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte–Berge Formula
In the mathematical discipline of graph theory the Tutte–Berge formula is a characterization of the size of a maximum matching in a graph. It is a generalization of Tutte theorem on perfect matchings, and is named after W. T. Tutte (who proved Tutte's theorem) and Claude Berge (who proved its generalization). Statement The theorem states that the size of a maximum matching of a graph G=(V,E) equals \frac \min_ \left(, U, -\operatorname(G-U)+, V, \right), where \operatorname(H) counts how many of the connected components of the graph H have an odd number of vertices. Equivalently, the number of ''unmatched'' vertices in a maximum matching equals\max_ \left(\operatorname(G-U)-, U, \right) . Explanation Intuitively, for any subset U of the vertices, the only way to completely cover an odd component of G-U by a matching is for one of the matched edges covering the component to be incident to U. If, instead, some odd component had no matched edge connecting it to U, th ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hanani–Tutte Theorem
In topological graph theory, the Hanani–Tutte theorem is a result on the parity of edge crossings in a graph drawing. It states that every drawing in the plane of a non-planar graph contains a pair of independent edges (not both sharing an endpoint) that cross each other an odd number of times. Equivalently, it can be phrased as a planarity criterion: a graph is planar if and only if it has a drawing in which every pair of independent edges crosses evenly (or not at all).. History The result is named after Haim Hanani, who proved in 1934 that every drawing of the two minimal non-planar graphs ''K''5 and ''K''3,3 has a pair of edges with an odd number of crossings, and after W. T. Tutte, who stated the full theorem explicitly in 1970. A parallel development of similar ideas in algebraic topology has been credited to Egbert van Kampen, Arnold S. Shapiro, and Wu Wenjun. Applications One consequence of the theorem is that testing whether a graph is planar may be formulated as ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Peripheral Cycle
In graph theory, a peripheral cycle (or peripheral circuit) in an undirected graph is, intuitively, a cycle that does not separate any part of the graph from any other part. Peripheral cycles (or, as they were initially called, peripheral polygons, because Tutte called cycles "polygons") were first studied by , and play important roles in the characterization of planar graphs and in generating the cycle spaces of nonplanar graphs. Definitions A peripheral cycle C in a graph G can be defined formally in one of several equivalent ways: *C is peripheral if it is a simple cycle in a connected graph with the property that, for every two edges e_1 and e_2 in G\setminus C, there exists a path in G that starts with e_1, ends with e_2, and has no interior vertices belonging to C.. *C is peripheral if it is an induced cycle with the property that the subgraph G\setminus C formed by deleting the edges and vertices of C is connected. *If C is any subgraph of G, a ''bridge'' of C is a mini ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte's Fragment
In mathematics, Tait's conjecture states that "Every 3-connected planar cubic graph has a Hamiltonian cycle (along the edges) through all its vertices". It was proposed by and disproved by , who constructed a counterexample with 25 faces, 69 edges and 46 vertices. Several smaller counterexamples, with 21 faces, 57 edges and 38 vertices, were later proved minimal by . The condition that the graph be 3-regular is necessary due to polyhedra such as the rhombic dodecahedron, which forms a bipartite graph with six degree-four vertices on one side and eight degree-three vertices on the other side; because any Hamiltonian cycle would have to alternate between the two sides of the bipartition, but they have unequal numbers of vertices, the rhombic dodecahedron is not Hamiltonian. The conjecture was significant, because if true, it would have implied the four color theorem: as Tait described, the four-color problem is equivalent to the problem of finding 3-edge-colorings of bridgeles ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Tutte Homotopy Theorem
In mathematics, the Tutte homotopy theorem, introduced by , generalises the concept of "path" from graphs to matroids, and states roughly that closed paths can be written as compositions of elementary closed paths, so that in some sense they are homotopic to the trivial closed path. Statement A matroid on a set ''Q'' is specified by a class of non-empty subsets ''M'' of ''Q'', called circuits, such that no element of ''M'' contains another, and if ''X'' and ''Y'' are in ''M'', ''a'' is in ''X'' and ''Y'', ''b'' is in ''X'' but not in ''Y'', then there is some ''Z'' in ''M'' containing ''b'' but not ''a'' and contained in ''X''∪''Y''. The subsets of ''Q'' that are unions of circuits are called flats (this is the language used in Tutte's original paper, however in modern usage the flats of a matroid mean something different). The elements of ''M'' are called 0-flats, the minimal non-empty flats that are not 0-flats are called 1-flats, the minimal nonempty flats that are not 0- ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |