Unit Cell Length on:
[Wikipedia]
[Google]
[Amazon]
In
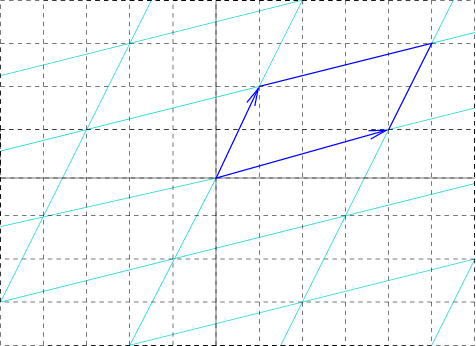 For any 2-dimensional lattice, the unit cells are parallelograms, which in special cases may have orthogonal angles, equal lengths, or both. Four of the five two-dimensional
For any 2-dimensional lattice, the unit cells are parallelograms, which in special cases may have orthogonal angles, equal lengths, or both. Four of the five two-dimensional
 For any 3-dimensional lattice, the conventional unit cells are parallelepipeds, which in special cases may have orthogonal angles, or equal lengths, or both. Seven of the fourteen three-dimensional
For any 3-dimensional lattice, the conventional unit cells are parallelepipeds, which in special cases may have orthogonal angles, or equal lengths, or both. Seven of the fourteen three-dimensional
geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, biology
Biology is the scientific study of life. It is a natural science with a broad scope but has several unifying themes that tie it together as a single, coherent field. For instance, all organisms are made up of cells that process hereditar ...
, mineralogy
Mineralogy is a subject of geology specializing in the scientific study of the chemistry, crystal structure, and physical (including optical) properties of minerals and mineralized artifacts. Specific studies within mineralogy include the proce ...
and solid state physics, a unit cell is a repeating unit formed by the vectors spanning the points of a lattice. Despite its suggestive name, the unit cell (unlike a unit vector, for example) does not necessarily have unit size, or even a particular size at all. Rather, the primitive cell is the closest analogy to a unit vector, since it has a determined size for a given lattice and is the basic building block from which larger cells are constructed.
The concept is used particularly in describing crystal structure
In crystallography, crystal structure is a description of the ordered arrangement of atoms, ions or molecules in a crystalline material. Ordered structures occur from the intrinsic nature of the constituent particles to form symmetric patterns t ...
in two and three dimensions, though it makes sense in all dimensions. A lattice can be characterized by the geometry of its unit cell, which is a section of the tiling (a parallelogram or parallelepiped) that generates the whole tiling using only translations.
There are two special cases of the unit cell: the primitive cell and the conventional cell. The primitive cell is a unit cell corresponding to a single lattice point, it is the smallest possible unit cell. In some cases, the full symmetry of a crystal structure is not obvious from the primitive cell, in which cases a conventional cell may be used. A conventional cell (which may or may not be primitive) is a unit cell with the full symmetry of the lattice and may include more than one lattice point. The conventional unit cells are parallelotopes in ''n'' dimensions.
Primitive cell
A primitive cell is a unit cell that contains exactly one lattice point. For unit cells generally, lattice points that are shared by cells are counted as of the lattice points contained in each of those cells; so for example a primitive unit cell in three dimensions which has lattice points only at its eight vertices is considered to contain of each of them. An alternative conceptualization is to consistently pick only one of the lattice points to belong to the given unit cell (so the other lattice points belong to adjacent unit cells). The ''primitive translation vectors'' , , span a lattice cell of smallest volume for a particular three-dimensional lattice, and are used to define a crystal translation vector : where , , are integers, translation by which leaves the lattice invariant. That is, for a point in the lattice , the arrangement of points appears the same from as from . Since the primitive cell is defined by the primitive axes (vectors) , , , the volume of the primitive cell is given by the parallelepiped from the above axes as : Usually, primitive cells in two and three dimensions are chosen to take the shape parallelograms and parallelepipeds, with an atom at each corner of the cell. This choice of primitive cell is not unique, but volume of primitive cells will always be given by the expression above.Wigner–Seitz cell
In addition to the parallelepiped primitive cells, for every Bravais lattice there is another kind of primitive cell called the Wigner–Seitz cell. In the Wigner–Seitz cell, the lattice point is at the center of the cell, and for most Bravais lattices, the shape is not a parallelogram or parallelepiped. This is a type ofVoronoi cell
In mathematics, a Voronoi diagram is a partition of a plane into regions close to each of a given set of objects. In the simplest case, these objects are just finitely many points in the plane (called seeds, sites, or generators). For each seed ...
. The Wigner–Seitz cell of the reciprocal lattice in momentum space is called the Brillouin zone.
Conventional cell
For each particular lattice, a conventional cell has been chosen on a case-by-case basis by crystallographers based on convenience of calculation. These conventional cells may have additional lattice points located in the middle of the faces or body of the unit cell. The number of lattice points, as well as the volume of the conventional cell is an integer multiple (1, 2, 3, or 4) of that of the primitive cell.Two dimensions
 For any 2-dimensional lattice, the unit cells are parallelograms, which in special cases may have orthogonal angles, equal lengths, or both. Four of the five two-dimensional
For any 2-dimensional lattice, the unit cells are parallelograms, which in special cases may have orthogonal angles, equal lengths, or both. Four of the five two-dimensional Bravais lattice
In geometry and crystallography, a Bravais lattice, named after , is an infinite array of discrete points generated by a set of discrete translation operations described in three dimensional space by
: \mathbf = n_1 \mathbf_1 + n_2 \mathbf_2 + n ...
s are represented using conventional primitive cells, as shown below.
The centered rectangular lattice also has a primitive cell in the shape of a rhombus, but in order to allow easy discrimination on the basis of symmetry, it is represented by a conventional cell which contains two lattice points.
Three dimensions
Bravais lattice
In geometry and crystallography, a Bravais lattice, named after , is an infinite array of discrete points generated by a set of discrete translation operations described in three dimensional space by
: \mathbf = n_1 \mathbf_1 + n_2 \mathbf_2 + n ...
s are represented using conventional primitive cells, as shown below.
The other seven Bravais lattices (known as the centered lattices) also have primitive cells in the shape of a parallelepiped, but in order to allow easy discrimination on the basis of symmetry, they are represented by conventional cells which contain more than one lattice point.
See also
* Wigner–Seitz cell *Bravais lattice
In geometry and crystallography, a Bravais lattice, named after , is an infinite array of discrete points generated by a set of discrete translation operations described in three dimensional space by
: \mathbf = n_1 \mathbf_1 + n_2 \mathbf_2 + n ...
* Wallpaper group
A wallpaper is a mathematical object covering a whole Euclidean plane by repeating a motif indefinitely, in manner that certain isometries keep the drawing unchanged. To a given wallpaper there corresponds a group of such congruent transformat ...
* Space group
In mathematics, physics and chemistry, a space group is the symmetry group of an object in space, usually in three dimensions. The elements of a space group (its symmetry operations) are the rigid transformations of an object that leave it ...
Notes
References
{{Crystallography Crystallography Mineralogy