Sphericon on:
[Wikipedia]
[Google]
[Amazon]

 In
In
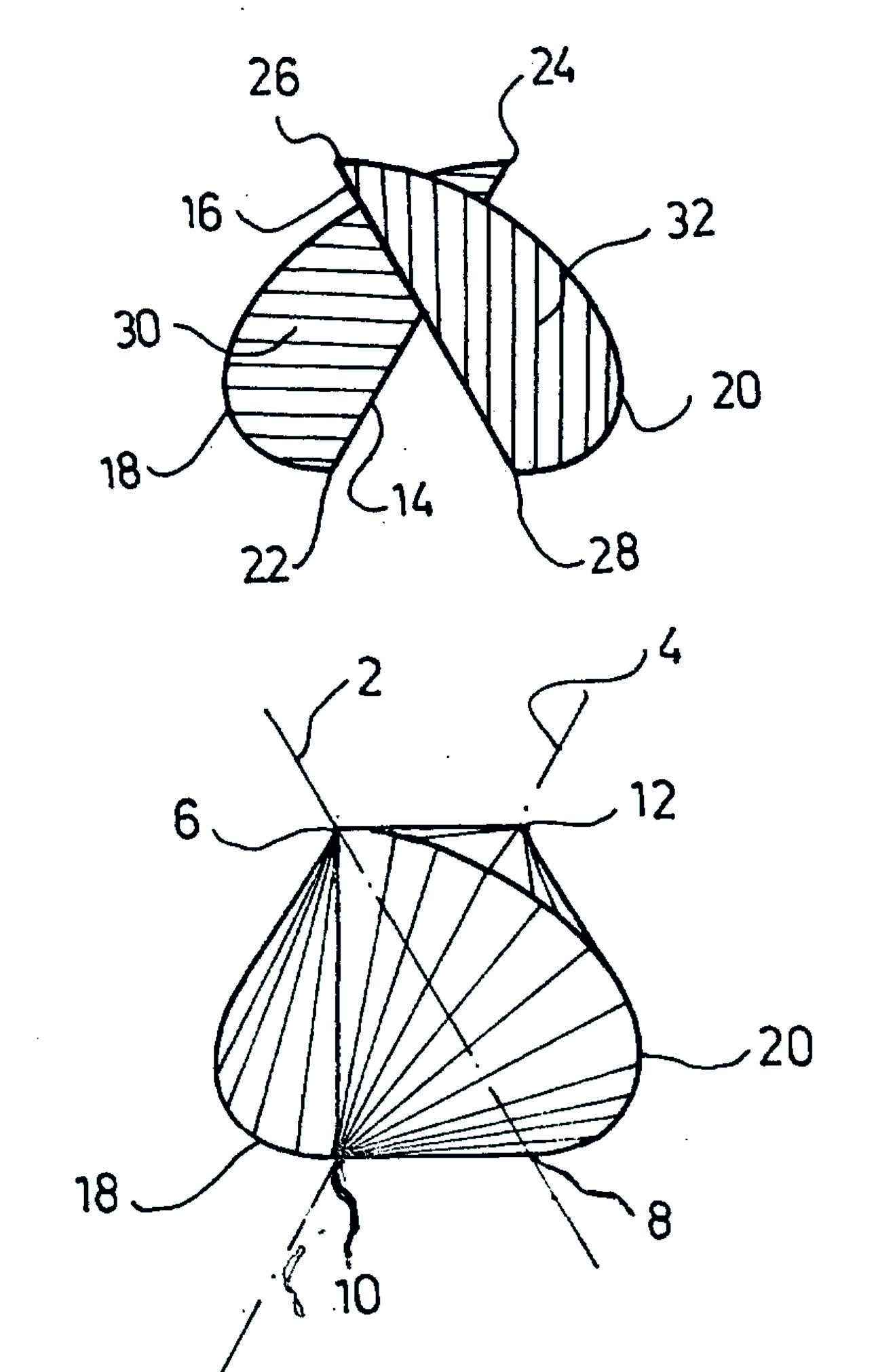 Around 1969, Colin Roberts (a carpenter from the UK) made a sphericon out of wood while attempting to carve a
Around 1969, Colin Roberts (a carpenter from the UK) made a sphericon out of wood while attempting to carve a
Sphericon construction animation at the National Curve Bank website.
Paper model of a sphericon
Make a sphericon
using
A Sphericon in Motion
showing the characteristic wobbly motion as it rolls across a flat surface Geometric shapes
solid geometry
Solid geometry or stereometry is the geometry of Three-dimensional space, three-dimensional Euclidean space (3D space).
A solid figure is the region (mathematics), region of 3D space bounded by a two-dimensional closed surface; for example, a ...
, the sphericon is a solid that has a continuous developable surface
In mathematics, a developable surface (or torse: archaic) is a smooth surface with zero Gaussian curvature. That is, it is a surface that can be flattened onto a plane without distortion (i.e. it can be bent without stretching or compression). ...
with two congruent
Congruence may refer to:
Mathematics
* Congruence (geometry), being the same size and shape
* Congruence or congruence relation, in abstract algebra, an equivalence relation on an algebraic structure that is compatible with the structure
* In modu ...
, semi-circular edges, and four vertices that define a square
In geometry, a square is a regular polygon, regular quadrilateral. It has four straight sides of equal length and four equal angles. Squares are special cases of rectangles, which have four equal angles, and of rhombuses, which have four equal si ...
. It is a member of a special family of rollers that, while being rolled on a flat surface, bring all the points of their surface to contact with the surface they are rolling on. It was discovered independently by carpenter Colin Roberts (who named it) in the UK in 1969, by dancer and sculptor Alan Boeding of MOMIX
MOMIX, a dance company based in Washington, Connecticut, was founded in 1981 by Choreographer Moses Pendleton. MOMIX was conceptualised 'out of work' Pendleton did for the celebration of Erik Satie at the Paris Opera in 1978. The company is n ...
in 1979, and by inventor David Hirsch, who patented it in Israel in 1980.
Construction
The sphericon may be constructed from abicone
In geometry, a bicone or dicone (from , and Greek: ''di-'', both meaning "two") is the three-dimensional surface of revolution of a rhombus around one of its axes of symmetry. Equivalently, a bicone is the surface created by joining two con ...
(a double cone
In geometry, a cone is a three-dimensional figure that tapers smoothly from a flat base (typically a circle) to a point not contained in the base, called the '' apex'' or '' vertex''.
A cone is formed by a set of line segments, half-lines ...
) with an apex
The apex is the highest point of something. The word may also refer to:
Arts and media Fictional entities
* Apex (comics)
A-Bomb
Abomination
Absorbing Man
Abraxas
Abyss
Abyss is the name of two characters appearing in Ameri ...
angle of 90 degrees, by splitting the bicone along a plane through both apexes, rotating one of the two halves by 90 degrees, and reattaching the two halves.
Alternatively, the surface of a sphericon can be formed by cutting and gluing a paper template in the form of four circular sector
A circular sector, also known as circle sector or disk sector or simply a sector (symbol: ⌔), is the portion of a disk (a closed region bounded by a circle) enclosed by two radii and an arc, with the smaller area being known as the ''minor ...
s (with central angle
A central angle is an angle whose apex (vertex) is the center O of a circle and whose legs (sides) are radii intersecting the circle in two distinct points A and B. Central angles are subtended by an arc between those two points, and the arc l ...
s ) joined edge-to-edge.
Geometric properties
Thesurface area
The surface area (symbol ''A'') of a solid object is a measure of the total area that the surface of the object occupies. The mathematical definition of surface area in the presence of curved surfaces is considerably more involved than the d ...
of a sphericon with radius is given by
:.
The volume
Volume is a measure of regions in three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch) ...
is given by
:,
exactly half the volume of a sphere
A sphere (from Ancient Greek, Greek , ) is a surface (mathematics), surface analogous to the circle, a curve. In solid geometry, a sphere is the Locus (mathematics), set of points that are all at the same distance from a given point in three ...
with the same radius.
History
 Around 1969, Colin Roberts (a carpenter from the UK) made a sphericon out of wood while attempting to carve a
Around 1969, Colin Roberts (a carpenter from the UK) made a sphericon out of wood while attempting to carve a Möbius strip
In mathematics, a Möbius strip, Möbius band, or Möbius loop is a Surface (topology), surface that can be formed by attaching the ends of a strip of paper together with a half-twist. As a mathematical object, it was discovered by Johann Bened ...
without a hole.
In 1979, David Hirsch invented a device for generating a meander motion. The device consisted of two perpendicular half discs joined at their axes of symmetry. While examining various configurations of this device, he discovered that the form created by joining the two half discs, exactly at their diameter
In geometry, a diameter of a circle is any straight line segment that passes through the centre of the circle and whose endpoints lie on the circle. It can also be defined as the longest Chord (geometry), chord of the circle. Both definitions a ...
centers, is actually a skeletal structure of a solid made of two half bicones, joined at their square cross-sections with an offset angle of 90 degrees, and that the two objects have exactly the same meander motion. Hirsch filed a patent in Israel in 1980, and a year later, a pull toy named Wiggler Duck, based on Hirsch's device, was introduced by Playskool
Playskool is an American brand of educational toys and games for preschoolers. The former Playskool manufacturing company was a subsidiary of the Milton Bradley Company and was headquartered in Chicago, Illinois. Playskool's last remaining plant ...
Company.
In 1999, Colin Roberts sent Ian Stewart a package containing a letter and two sphericon models. In response, Stewart wrote an article "Cone with a Twist" in his Mathematical Recreations column of ''Scientific American
''Scientific American'', informally abbreviated ''SciAm'' or sometimes ''SA'', is an American popular science magazine. Many scientists, including Albert Einstein and Nikola Tesla, have contributed articles to it, with more than 150 Nobel Pri ...
''. This sparked quite a bit of interest in the shape, and has been used by Tony Phillips to develop theories about mazes. Roberts' name for the shape, the sphericon, was taken by Hirsch as the name for his company, Sphericon Ltd.
In popular culture
In 1979, modern dancer Alan Boeding designed his "Circle Walker" sculpture from two crosswise semicircles, askeletal
A skeleton is the structural frame that supports the body of most animals. There are several types of skeletons, including the exoskeleton, which is a rigid outer shell that holds up an organism's shape; the endoskeleton, a rigid internal fram ...
version of the sphericon. He began dancing with a scaled-up version of the sculpture in 1980 as part of an MFA program in sculpture at Indiana University
Indiana University (IU) is a state university system, system of Public university, public universities in the U.S. state of Indiana. The system has two core campuses, five regional campuses, and two regional centers under the administration o ...
, and after he joined the MOMIX
MOMIX, a dance company based in Washington, Connecticut, was founded in 1981 by Choreographer Moses Pendleton. MOMIX was conceptualised 'out of work' Pendleton did for the celebration of Erik Satie at the Paris Opera in 1978. The company is n ...
dance company in 1984 the piece became incorporated into the company's performances. The company's later piece "Dream Catcher" is based around a similar Boeding sculpture whose linked teardrop shapes incorporate the skeleton and rolling motion of the oloid
An oloid is a three-dimensional curved geometric object that was discovered by Paul Schatz in 1929. It is the convex hull of a skeletal frame made by placing two linked congruent circles in perpendicular planes, so that the center of each circl ...
, a similar rolling shape formed from two perpendicular circles each passing through the center of the other.
In 2008, British woodturner David Springett published the book "Woodturning Full Circle", which explains how sphericons (and other unusual solid forms, such as streptohedrons) can be made on a wood lathe.
References
External links
{{wiktionary, sphericonSphericon construction animation at the National Curve Bank website.
Paper model of a sphericon
Make a sphericon
using
regular polygon
In Euclidean geometry, a regular polygon is a polygon that is Equiangular polygon, direct equiangular (all angles are equal in measure) and Equilateral polygon, equilateral (all sides have the same length). Regular polygons may be either ''convex ...
s with different numbers of sidesA Sphericon in Motion
showing the characteristic wobbly motion as it rolls across a flat surface Geometric shapes