Slope (mathematics) on:
[Wikipedia]
[Google]
[Amazon]
 In
In
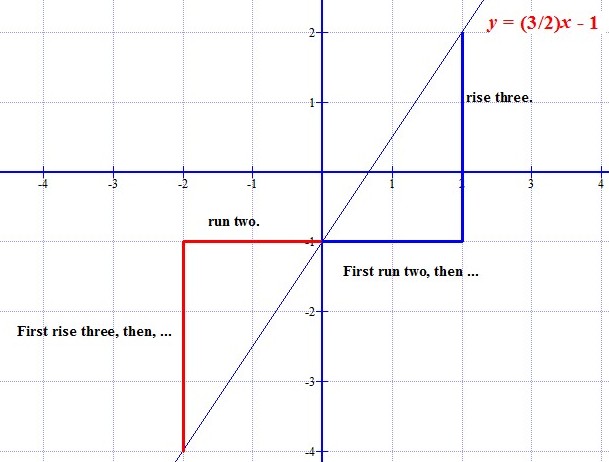 The slope of a line in the plane containing the ''x'' and ''y'' axes is generally represented by the letter ''m'', and is defined as the change in the ''y'' coordinate divided by the corresponding change in the ''x'' coordinate, between two distinct points on the line. This is described by the following equation:
:
(The Greek letter ''
The slope of a line in the plane containing the ''x'' and ''y'' axes is generally represented by the letter ''m'', and is defined as the change in the ''y'' coordinate divided by the corresponding change in the ''x'' coordinate, between two distinct points on the line. This is described by the following equation:
:
(The Greek letter ''

 The concept of a slope is central to
The concept of a slope is central to
 An extension of the idea of angle follows from the difference of slopes. Consider the
An extension of the idea of angle follows from the difference of slopes. Consider the
File:Nederlands verkeersbord J6.svg, Slope warning sign in the
mathematics
Mathematics is a field of study that discovers and organizes methods, Mathematical theory, theories and theorems that are developed and Mathematical proof, proved for the needs of empirical sciences and mathematics itself. There are many ar ...
, the slope or gradient of a line is a number that describes the direction of the line on a plane. Often denoted by the letter ''m'', slope is calculated as the ratio
In mathematics, a ratio () shows how many times one number contains another. For example, if there are eight oranges and six lemons in a bowl of fruit, then the ratio of oranges to lemons is eight to six (that is, 8:6, which is equivalent to the ...
of the vertical change to the horizontal change ("rise over run") between two distinct points on the line, giving the same number for any choice of points.
The line may be physical – as set by a road surveyor, pictorial as in a diagram
A diagram is a symbolic Depiction, representation of information using Visualization (graphics), visualization techniques. Diagrams have been used since prehistoric times on Cave painting, walls of caves, but became more prevalent during the Age o ...
of a road or roof, or abstract.
An application of the mathematical concept is found in the grade or gradient
In vector calculus, the gradient of a scalar-valued differentiable function f of several variables is the vector field (or vector-valued function) \nabla f whose value at a point p gives the direction and the rate of fastest increase. The g ...
in geography
Geography (from Ancient Greek ; combining 'Earth' and 'write', literally 'Earth writing') is the study of the lands, features, inhabitants, and phenomena of Earth. Geography is an all-encompassing discipline that seeks an understanding o ...
and civil engineering
Civil engineering is a regulation and licensure in engineering, professional engineering discipline that deals with the design, construction, and maintenance of the physical and naturally built environment, including public works such as roads ...
.
The ''steepness'', incline, or grade of a line is the absolute value
In mathematics, the absolute value or modulus of a real number x, is the non-negative value without regard to its sign. Namely, , x, =x if x is a positive number, and , x, =-x if x is negative (in which case negating x makes -x positive), ...
of its slope: greater absolute value indicates a steeper line. The line trend is defined as follows:
*An "increasing" or "ascending" line goes from left to right and has positive slope: .
*A "decreasing" or "descending" line goes from left to right and has negative slope: .
Special directions are:
*A "(square) diagonal
In geometry, a diagonal is a line segment joining two vertices of a polygon or polyhedron, when those vertices are not on the same edge. Informally, any sloping line is called diagonal. The word ''diagonal'' derives from the ancient Greek � ...
" line has unit slope:
*A "horizontal" line (the graph of a constant function
In mathematics, a constant function is a function whose (output) value is the same for every input value.
Basic properties
As a real-valued function of a real-valued argument, a constant function has the general form or just For example, ...
) has zero slope: .
*A "vertical" line has undefined or infinite slope (see below).
If two points of a road have altitudes ''y''1 and ''y''2, the rise is the difference (''y''2 − ''y''1) = Δ''y''. Neglecting the Earth's curvature, if the two points have horizontal distance ''x''1 and ''x''2 from a fixed point, the run is (''x''2 − ''x''1) = Δ''x''. The slope between the two points is the difference ratio:
:
Through trigonometry
Trigonometry () is a branch of mathematics concerned with relationships between angles and side lengths of triangles. In particular, the trigonometric functions relate the angles of a right triangle with ratios of its side lengths. The fiel ...
, the slope ''m'' of a line is related to its angle
In Euclidean geometry, an angle can refer to a number of concepts relating to the intersection of two straight Line (geometry), lines at a Point (geometry), point. Formally, an angle is a figure lying in a Euclidean plane, plane formed by two R ...
of inclination ''θ'' by the tangent function
:
Thus, a 45° rising line has slope ''m ='' +1, and a 45° falling line has slope ''m ='' −1.
Generalizing this, differential calculus
In mathematics, differential calculus is a subfield of calculus that studies the rates at which quantities change. It is one of the two traditional divisions of calculus, the other being integral calculus—the study of the area beneath a curve. ...
defines the slope of a plane curve at a point as the slope of its tangent line
In geometry, the tangent line (or simply tangent) to a plane curve at a given point is, intuitively, the straight line that "just touches" the curve at that point. Leibniz defined it as the line through a pair of infinitely close points o ...
at that point. When the curve is approximated by a series of points, the slope of the curve may be approximated by the slope of the secant line
In geometry, a secant is a line (geometry), line that intersects a curve at a minimum of two distinct Point (geometry), points..
The word ''secant'' comes from the Latin word ''secare'', meaning ''to cut''. In the case of a circle, a secant inter ...
between two nearby points. When the curve is given as the graph of an algebraic expression, calculus gives formulas for the slope at each point. Slope is thus one of the central ideas of calculus and its applications to design.
Notation
There seems to be no clear answer as to why the letter ''m'' is used for slope, but it first appears in English in O'Brien (1844) who introduced the equation of a line as , and it can also be found in Todhunter (1888) who wrote "''y'' = ''mx'' + ''c''".Definition
 The slope of a line in the plane containing the ''x'' and ''y'' axes is generally represented by the letter ''m'', and is defined as the change in the ''y'' coordinate divided by the corresponding change in the ''x'' coordinate, between two distinct points on the line. This is described by the following equation:
:
(The Greek letter ''
The slope of a line in the plane containing the ''x'' and ''y'' axes is generally represented by the letter ''m'', and is defined as the change in the ''y'' coordinate divided by the corresponding change in the ''x'' coordinate, between two distinct points on the line. This is described by the following equation:
:
(The Greek letter ''delta
Delta commonly refers to:
* Delta (letter) (Δ or δ), the fourth letter of the Greek alphabet
* D (NATO phonetic alphabet: "Delta"), the fourth letter in the Latin alphabet
* River delta, at a river mouth
* Delta Air Lines, a major US carrier ...
'', Δ, is commonly used in mathematics to mean "difference" or "change".)
Given two points and , the change in from one to the other is (''run''), while the change in is (''rise''). Substituting both quantities into the above equation generates the formula:
:
The formula fails for a vertical line, parallel to the axis (see Division by zero
In mathematics, division by zero, division (mathematics), division where the divisor (denominator) is 0, zero, is a unique and problematic special case. Using fraction notation, the general example can be written as \tfrac a0, where a is the di ...
), where the slope can be taken as infinite, so the slope of a vertical line is considered undefined.
Examples
Suppose a line runs through two points: ''P'' = (1, 2) and ''Q'' = (13, 8). By dividing the difference in -coordinates by the difference in -coordinates, one can obtain the slope of the line: : :Since the slope is positive, the direction of the line is increasing. Since , ''m'', < 1, the incline is not very steep (incline < 45°). As another example, consider a line which runs through the points (4, 15) and (3, 21). Then, the slope of the line is : :Since the slope is negative, the direction of the line is decreasing. Since , ''m'', > 1, this decline is fairly steep (decline > 45°).Algebra and geometry
Examples
For example, consider a line running through points (2,8) and (3,20). This line has a slope, , of : One can then write the line's equation, in point-slope form: : or: : The angle θ between −90° and 90° that this line makes with the -axis is : Consider the two lines: and . Both lines have slope . They are not the same line. So they are parallel lines. Consider the two lines and . The slope of the first line is . The slope of the second line is . The product of these two slopes is −1. So these two lines are perpendicular.Statistics
Instatistics
Statistics (from German language, German: ', "description of a State (polity), state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a s ...
, the gradient of the least-squares regression best-fitting line for a given sample of data may be written as:
:,
This quantity ''m'' is called as the '' regression slope'' for the line . The quantity is Pearson's correlation coefficient, is the standard deviation
In statistics, the standard deviation is a measure of the amount of variation of the values of a variable about its Expected value, mean. A low standard Deviation (statistics), deviation indicates that the values tend to be close to the mean ( ...
of the y-values and is the standard deviation
In statistics, the standard deviation is a measure of the amount of variation of the values of a variable about its Expected value, mean. A low standard Deviation (statistics), deviation indicates that the values tend to be close to the mean ( ...
of the x-values. This may also be written as a ratio of covariances:
:
Calculus
 The concept of a slope is central to
The concept of a slope is central to differential calculus
In mathematics, differential calculus is a subfield of calculus that studies the rates at which quantities change. It is one of the two traditional divisions of calculus, the other being integral calculus—the study of the area beneath a curve. ...
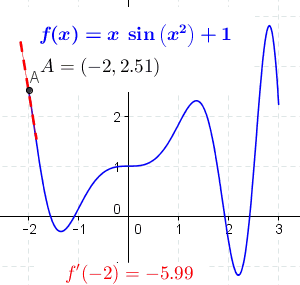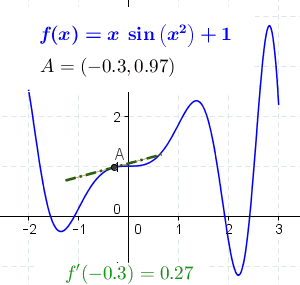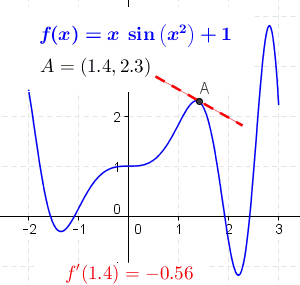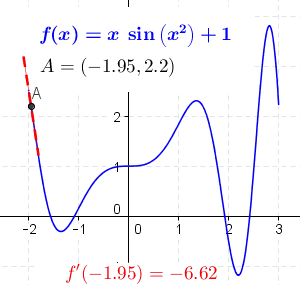
. For non-linear functions, the rate of change varies along the curve. The derivative
In mathematics, the derivative is a fundamental tool that quantifies the sensitivity to change of a function's output with respect to its input. The derivative of a function of a single variable at a chosen input value, when it exists, is t ...
of the function at a point is the slope of the line tangent
In geometry, the tangent line (or simply tangent) to a plane curve at a given point is, intuitively, the straight line that "just touches" the curve at that point. Leibniz defined it as the line through a pair of infinitely close points o ...
to the curve at the point and is thus equal to the rate of change of the function at that point.
If we let Δ''x'' and Δ''y'' be the distances (along the ''x'' and ''y'' axes, respectively) between two points on a curve, then the slope given by the above definition,
:,
is the slope of a secant line
In geometry, a secant is a line (geometry), line that intersects a curve at a minimum of two distinct Point (geometry), points..
The word ''secant'' comes from the Latin word ''secare'', meaning ''to cut''. In the case of a circle, a secant inter ...
to the curve. For a line, the secant between any two points is the line itself, but this is not the case for any other type of curve.
For example, the slope of the secant intersecting ''y'' = ''x''2 at (0,0) and (3,9) is 3. (The slope of the tangent at is also 3 − ''a'' consequence of the mean value theorem
In mathematics, the mean value theorem (or Lagrange's mean value theorem) states, roughly, that for a given planar arc (geometry), arc between two endpoints, there is at least one point at which the tangent to the arc is parallel to the secant lin ...
.)
By moving the two points closer together so that Δ''y'' and Δ''x'' decrease, the secant line more closely approximates a tangent line to the curve, and as such the slope of the secant approaches that of the tangent. Using differential calculus
In mathematics, differential calculus is a subfield of calculus that studies the rates at which quantities change. It is one of the two traditional divisions of calculus, the other being integral calculus—the study of the area beneath a curve. ...
, we can determine the limit, or the value that Δ''y''/Δ''x'' approaches as Δ''y'' and Δ''x'' get closer to zero; it follows that this limit is the exact slope of the tangent. If ''y'' is dependent on ''x'', then it is sufficient to take the limit where only Δ''x'' approaches zero. Therefore, the slope of the tangent is the limit of Δ''y''/Δ''x'' as Δ''x'' approaches zero, or d''y''/d''x''. We call this limit the derivative
In mathematics, the derivative is a fundamental tool that quantifies the sensitivity to change of a function's output with respect to its input. The derivative of a function of a single variable at a chosen input value, when it exists, is t ...
.
:
The value of the derivative at a specific point on the function provides us with the slope of the tangent at that precise location. For example, let ''y'' = ''x''2. A point on this function is (−2,4). The derivative of this function is . So the slope of the line tangent to ''y'' at (−2,4) is . The equation of this tangent line is: or .
Difference of slopes
shear mapping
In plane geometry, a shear mapping is an affine transformation that displaces each point in a fixed direction by an amount proportional to its signed distance function, signed distance from a given straight line, line parallel (geometry), paral ...
:
Then is mapped to . The slope of is zero and the slope of is . The shear mapping added a slope of . For two points on with slopes and , the image
:
has slope increased by , but the difference of slopes is the same before and after the shear. This invariance of slope differences makes slope an angular invariant measure, on a par with circular angle (invariant under rotation) and hyperbolic angle, with invariance group of squeeze mapping
In linear algebra, a squeeze mapping, also called a squeeze transformation, is a type of linear map that preserves Euclidean area of regions in the Cartesian plane, but is ''not'' a rotation (mathematics), rotation or shear mapping.
For a fixed p ...
s.
Slope (pitch) of a roof
The slope of a roof, traditionally and commonly called the roof pitch, in carpentry and architecture in the US is commonly described in terms of integer fractions of one foot (geometric tangent, rise over run), a legacy of British imperial measure. Other units are in use in other locales, with similar conventions. For details, see roof pitch.Slope of a road or railway
There are two common ways to describe the steepness of aroad
A road is a thoroughfare used primarily for movement of traffic. Roads differ from streets, whose primary use is local access. They also differ from stroads, which combine the features of streets and roads. Most modern roads are paved.
Th ...
or railroad
Rail transport (also known as train transport) is a means of transport using wheeled vehicles running in railway track, tracks, which usually consist of two parallel steel railway track, rails. Rail transport is one of the two primary means of ...
. One is by the angle between 0° and 90° (in degrees), and the other is by the slope in a percentage. See also steep grade railway and rack railway.
The formulae for converting a slope given as a percentage into an angle in degrees and vice versa are:
: (this is the inverse function of tangent; see trigonometry
Trigonometry () is a branch of mathematics concerned with relationships between angles and side lengths of triangles. In particular, the trigonometric functions relate the angles of a right triangle with ratios of its side lengths. The fiel ...
)
and
:
where ''angle'' is in degrees and the trigonometric functions operate in degrees. For example, a slope of 100 % or 1000 ‰ is an angle of 45°.
A third way is to give one unit of rise in say 10, 20, 50 or 100 horizontal units, e.g. 1:10. 1:20, 1:50 or 1:100 (or "1 ''in'' 10", "1 ''in'' 20", etc.) 1:10 is steeper than 1:20. For example, steepness of 20% means 1:5 or an incline with angle 11.3°.
Roads and railways have both longitudinal slopes and cross slopes.
Netherlands
, Terminology of the Low Countries, informally Holland, is a country in Northwestern Europe, with Caribbean Netherlands, overseas territories in the Caribbean. It is the largest of the four constituent countries of the Kingdom of the Nether ...
File:PL road sign A-23.svg, Slope warning sign in Poland
Poland, officially the Republic of Poland, is a country in Central Europe. It extends from the Baltic Sea in the north to the Sudetes and Carpathian Mountains in the south, bordered by Lithuania and Russia to the northeast, Belarus and Ukrai ...
File: Skloník-klesání.jpg, A 1371-meter distance of a railroad with a 20 ‰ slope. Czech Republic
The Czech Republic, also known as Czechia, and historically known as Bohemia, is a landlocked country in Central Europe. The country is bordered by Austria to the south, Germany to the west, Poland to the northeast, and Slovakia to the south ...
File: Railway gradient post.jpg, Steam-age railway gradient post indicating a slope in both directions at Meols railway station, United Kingdom
Other uses
The concept of a slope or gradient is also used as a basis for developing other applications in mathematics: * Gradient descent, a first-order iterative optimization algorithm for finding the minimum of a function * Gradient theorem, theorem that a line integral through a gradient field can be evaluated by evaluating the original scalar field at the endpoints of the curve *Gradient method In optimization, a gradient method is an algorithm
In mathematics and computer science, an algorithm () is a finite sequence of Rigour#Mathematics, mathematically rigorous instructions, typically used to solve a class of specific Computational ...
, an algorithm to solve problems with search directions defined by the gradient of the function at the current point
* Conjugate gradient method, an algorithm for the numerical solution of particular systems of linear equations
* Nonlinear conjugate gradient method, generalizes the conjugate gradient method to nonlinear optimization
* Stochastic gradient descent, iterative method for optimizing a differentiable objective function
See also
*Euclidean distance
In mathematics, the Euclidean distance between two points in Euclidean space is the length of the line segment between them. It can be calculated from the Cartesian coordinates of the points using the Pythagorean theorem, and therefore is o ...
* Grade
* Inclined plane
* Linear function
In mathematics, the term linear function refers to two distinct but related notions:
* In calculus and related areas, a linear function is a function whose graph is a straight line, that is, a polynomial function of degree zero or one. For di ...
* Line of greatest slope
* Mediant
* Slope definitions
* Theil–Sen estimator, a line with the median
The median of a set of numbers is the value separating the higher half from the lower half of a Sample (statistics), data sample, a statistical population, population, or a probability distribution. For a data set, it may be thought of as the “ ...
slope among a set of sample points
References
External links
* interactive {{Calculus topics Elementary mathematics Analytic geometry Ratios