Scale Height on:
[Wikipedia]
[Google]
[Amazon]
 In
In
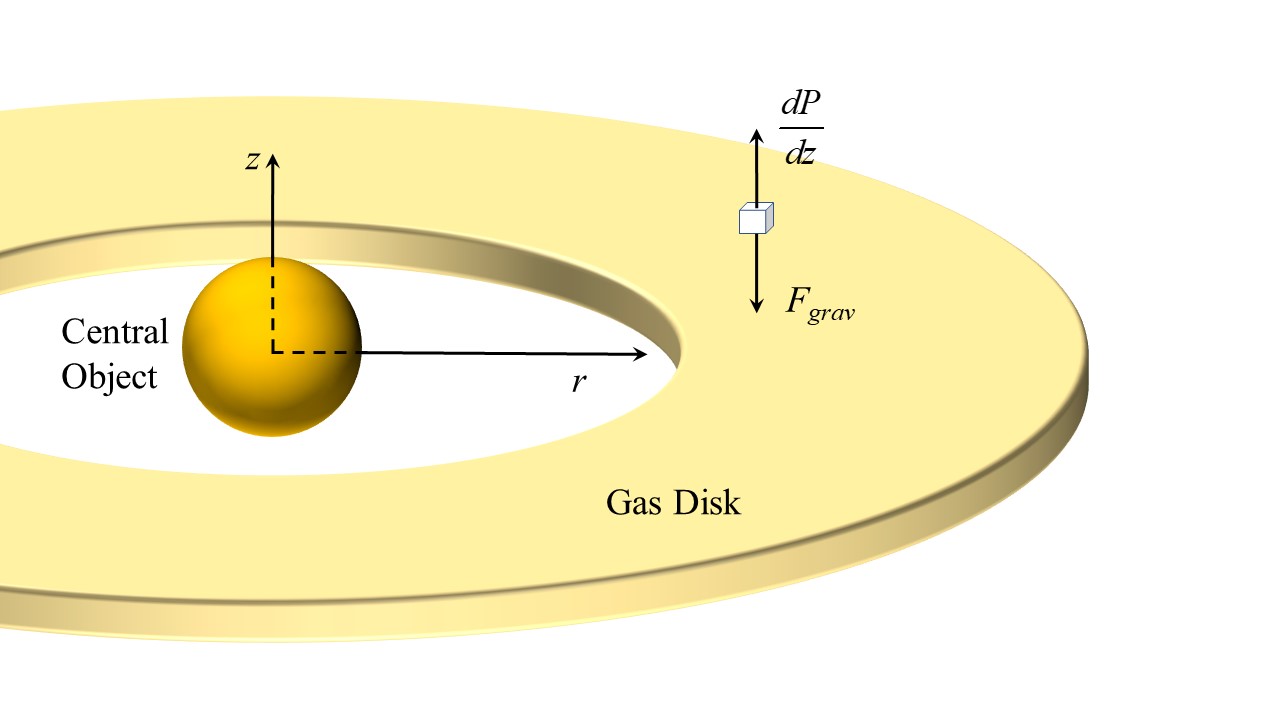 For a disk of gas around a condensed central object, such as, for example, a protostar, one can derive a disk scale height which is somewhat analogous to the planetary scale height. We start with a disc of gas that has a mass which is small relative to the central object. We assume that the disc is in hydrostatic equilibrium with the ''z'' component of gravity from the star, where the gravity component is pointing to the midplane of the disk:
where:
* ''G'' =
For a disk of gas around a condensed central object, such as, for example, a protostar, one can derive a disk scale height which is somewhat analogous to the planetary scale height. We start with a disc of gas that has a mass which is small relative to the central object. We assume that the disc is in hydrostatic equilibrium with the ''z'' component of gravity from the star, where the gravity component is pointing to the midplane of the disk:
where:
* ''G'' =
atmospheric
An atmosphere () is a layer of gas or layers of gases that envelop a planet, and is held in place by the gravity of the planetary body. A planet retains an atmosphere when the gravity is great and the temperature of the atmosphere is low. A s ...
, earth, and planetary sciences, a scale height, usually denoted by the capital letter ''H'', is a distance ( vertical or radial) over which a physical quantity
A physical quantity is a physical property of a material or system that can be quantified by measurement. A physical quantity can be expressed as a ''value'', which is the algebraic multiplication of a ' Numerical value ' and a ' Unit '. For examp ...
decreases by a factor of e (the base of natural logarithms, approximately 2.718).
Scale height used in a simple atmospheric pressure model
For planetary atmospheres, scale height is the increase in altitude for which the atmospheric pressure decreases by a factor of ''e''. The scale height remains constant for a particular temperature. It can be calculated by or equivalently where: * ''k''B = Boltzmann constant = * ''R'' = gas constant * ''T'' = mean atmospheric temperature in kelvins = 250 K for Earth * ''m'' = mean mass of a molecule (units kg) * ''M'' = mean mass of one mol of atmospheric particles = 0.029 kg/mol for Earth * ''g'' = acceleration due to gravity at the current location (m/s2) The pressure (force per unit area) at a given altitude is a result of the weight of the overlying atmosphere. If at a height of ''z'' the atmosphere has density ''ρ'' and pressure ''P'', then moving upwards an infinitesimally small height ''dz'' will decrease the pressure by amount ''dP'', equal to the weight of a layer of atmosphere of thickness ''dz''. Thus: where ''g'' is the acceleration due to gravity. For small ''dz'' it is possible to assume ''g'' to be constant; the minus sign indicates that as the height increases the pressure decreases. Therefore, using the equation of state for an ideal gas of mean molecular mass ''M'' at temperature ''T'', the density can be expressed as Combining these equations gives which can then be incorporated with the equation for ''H'' given above to give: which will not change unless the temperature does. Integrating the above and assuming ''P''0 is the pressure at height ''z'' = 0 (pressure at sea level) the pressure at height ''z'' can be written as: This translates as the pressure decreasing exponentially with height. In Earth's atmosphere, the pressure at sea level ''P''0 averages about , the mean molecular mass of dry air is 28.964 u and hence m = 28.964 × = . As a function of temperature, the scale height of Earth's atmosphere is therefore ''H''/''T'' = ''k''/''mg'' = (1.38/(4.808×9.81))×103 = . This yields the following scale heights for representative air temperatures. *''T'' = 290 K, ''H'' = 8500 m *''T'' = 273 K, ''H'' = 8000 m *''T'' = 260 K, ''H'' = 7610 m *''T'' = 210 K, ''H'' = 6000 m These figures should be compared with the temperature and density of Earth's atmosphere plotted at NRLMSISE-00, which shows the air density dropping from 1200 g/m3 at sea level to 0.53 = 0.125 g/m3 at 70 km, a factor of 9600, indicating an average scale height of 70/ln(9600) = 7.64 km, consistent with the indicated average air temperature over that range of close to 260 K. Note: * Density is related to pressure by the ideal gas laws. Therefore, density will also decrease exponentially with height from a sea level value of ''ρ''0 roughly equal to 1.2 kg m−3 * At heights over 100 km, an atmosphere may no longer be well mixed. Then each chemical species has its own scale height. * Here temperature and gravitational acceleration were assumed to be constant but both may vary over large distances.Planetary examples
Approximate atmospheric scale heights for selected Solar System bodies follow. * Venus: 15.9 km * Earth: 8.5 km * Mars: 11.1 km * Jupiter: 27 km *Saturn
Saturn is the sixth planet from the Sun and the second-largest in the Solar System, after Jupiter. It is a gas giant with an average radius of about nine and a half times that of Earth. It has only one-eighth the average density of Earth; h ...
: 59.5 km
**Titan
Titan most often refers to:
* Titan (moon), the largest moon of Saturn
* Titans, a race of deities in Greek mythology
Titan or Titans may also refer to:
Arts and entertainment
Fictional entities
Fictional locations
* Titan in fiction, fictiona ...
: 21 km
* Uranus: 27.7 km
*Neptune
Neptune is the eighth planet from the Sun and the farthest known planet in the Solar System. It is the fourth-largest planet in the Solar System by diameter, the third-most-massive planet, and the densest giant planet. It is 17 times ...
: 19.1–20.3 km
* Pluto: ~50 km
Scale height for a thin disk
 For a disk of gas around a condensed central object, such as, for example, a protostar, one can derive a disk scale height which is somewhat analogous to the planetary scale height. We start with a disc of gas that has a mass which is small relative to the central object. We assume that the disc is in hydrostatic equilibrium with the ''z'' component of gravity from the star, where the gravity component is pointing to the midplane of the disk:
where:
* ''G'' =
For a disk of gas around a condensed central object, such as, for example, a protostar, one can derive a disk scale height which is somewhat analogous to the planetary scale height. We start with a disc of gas that has a mass which is small relative to the central object. We assume that the disc is in hydrostatic equilibrium with the ''z'' component of gravity from the star, where the gravity component is pointing to the midplane of the disk:
where:
* ''G'' = Gravitational constant
The gravitational constant (also known as the universal gravitational constant, the Newtonian constant of gravitation, or the Cavendish gravitational constant), denoted by the capital letter , is an empirical physical constant involved in ...
≈
* ''r'' = the radial cylindrical coordinate for the distance from the center of the star or centrally condensed object
* ''z'' = the height/altitude cylindrical coordinate for the distance from the disk midplane (or center of the star)
* ''M''* = the mass of the star/centrally condensed object
* ''P'' = the pressure of the gas in the disk
* = the gas mass density in the disk
In the thin disk approximation, and the hydrostatic equilibrium equation is
To determine the gas pressure, one can use the ideal gas law:
with:
* ''T'' = the gas temperature in the disk, where the temperature is a function of ''r'', but independent of ''z''
* = the mean molecular mass of the gas
Using the ideal gas law and the hydrostatic equilibrium equation, gives:
which has the solution
where is the gas mass density at the midplane of the disk at a distance ''r'' from the center of the star and is the disk scale height with
with the solar mass
The solar mass () is a standard unit of mass in astronomy, equal to approximately . It is often used to indicate the masses of other stars, as well as stellar clusters, nebulae, galaxies and black holes. It is approximately equal to the mass ...
, the astronomical unit and the atomic mass unit.
As an illustrative approximation, if we ignore the radial variation in the temperature, , we see that and that the disk increases in altitude as one moves radially away from the central object.
Due to the assumption that the gas temperature in the disk, ''T'', is independent of ''z'', is sometimes known as the isothermal disk scale height.
Disk scale height in a magnetic field
Amagnetic field
A magnetic field is a vector field that describes the magnetic influence on moving electric charges, electric currents, and magnetic materials. A moving charge in a magnetic field experiences a force perpendicular to its own velocity and to ...
in a thin gas disk around a central object can change the scale height of the disk. For example, if a non-perfectly conducting disk is rotating through a poloidal magnetic field (i.e., the initial magnetic field is perpendicular to the plane of the disk), then a toroidal (i.e., parallel to the disk plane) magnetic field will be produced within the disk, which will ''pinch'' and compress the disk. In this case, the gas density of the disk is:
where the ''cut-off'' density has the form
where
* is the permeability of free space
* is the electrical conductivity
Electrical resistivity (also called specific electrical resistance or volume resistivity) is a fundamental property of a material that measures how strongly it resists electric current. A low resistivity indicates a material that readily allow ...
of the disk
* is the magnetic flux density of the poloidal field in the direction
* is the rotational angular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
of the central object (if the poloidal magnetic field is independent of the central object then can be set to zero)
* is the keplerian angular velocity
In physics, angular velocity or rotational velocity ( or ), also known as angular frequency vector,(UP1) is a pseudovector representation of how fast the angular position or orientation of an object changes with time (i.e. how quickly an objec ...
of the disk at a distance from the central object.
These formulae give the maximum height, , of the magnetized disk as
while the e-folding magnetic scale height, , is
See also
* Time constantReferences
{{reflist, colwidth=30em Atmospheric dynamics