Photon statistics on:
[Wikipedia]
[Google]
[Amazon]
Photon statistics is the theoretical and experimental study of the statistical distributions produced in
 On substituting the intensity probability distribution of thermal light for P(I), Mandel's formula becomes
:
Using the following formula to evaluate the integral
The probability distribution for n photon counts from a thermal light source is
:
where is the average number of counts. This last distribution is known as the Bose-Einstein distribution. The variance of the distribution can be shown to be
:
In contrast with the Poisson distribution for a coherent light source, the Bose-Einstein distribution has characteristic of thermal light.
On substituting the intensity probability distribution of thermal light for P(I), Mandel's formula becomes
:
Using the following formula to evaluate the integral
The probability distribution for n photon counts from a thermal light source is
:
where is the average number of counts. This last distribution is known as the Bose-Einstein distribution. The variance of the distribution can be shown to be
:
In contrast with the Poisson distribution for a coherent light source, the Bose-Einstein distribution has characteristic of thermal light.
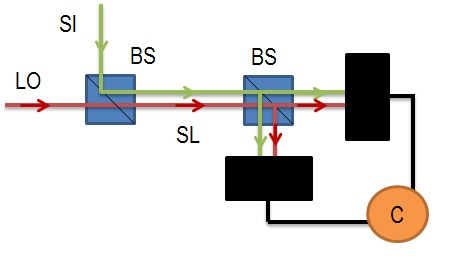 Light that is governed by sub-Poisson statistics cannot be described by classical electromagnetic theory and is defined by . The advent of ultrafast photodetectors has made it possible to measure the sub-Poissonian nature of light. An example of light exhibiting sub-Poissonian statistics is squeezed light. Recently researchers have shown that sub-Poissonian light can be induced in a quantum dot exhibiting resonance fluorescence. A technique used to measure the sub-Poissonian structure of light is a homodyne intensity correlation scheme. In this scheme a local oscillator and signal field are superimposed via a beam splitter. The superimposed light is then split by another beam splitter and each signal is recorded by individual photodetectors connected to correlator from which the intensity correlation can be measured. Evidence of the sub-Poissonian nature of light is shown by obtaining a negative intensity correlation as was shown in.
Light that is governed by sub-Poisson statistics cannot be described by classical electromagnetic theory and is defined by . The advent of ultrafast photodetectors has made it possible to measure the sub-Poissonian nature of light. An example of light exhibiting sub-Poissonian statistics is squeezed light. Recently researchers have shown that sub-Poissonian light can be induced in a quantum dot exhibiting resonance fluorescence. A technique used to measure the sub-Poissonian structure of light is a homodyne intensity correlation scheme. In this scheme a local oscillator and signal field are superimposed via a beam splitter. The superimposed light is then split by another beam splitter and each signal is recorded by individual photodetectors connected to correlator from which the intensity correlation can be measured. Evidence of the sub-Poissonian nature of light is shown by obtaining a negative intensity correlation as was shown in.
photon counting
Photon counting is a technique in which individual photons are counted using a single-photon detector (SPD). A single-photon detector emits a pulse of signal for each detected photon, in contrast to a normal photodetector, which generates an analo ...
experiments, which use photodetector
Photodetectors, also called photosensors, are sensors of light or other electromagnetic radiation. There is a wide variety of photodetectors which may be classified by mechanism of detection, such as photoelectric or photochemical effects, or by ...
s to analyze the intrinsic statistical nature of photons in a light source. In these experiments, light incident on the photodetector generates photoelectrons and a counter registers electrical pulses generating a statistical distribution of photon counts. Low intensity disparate light sources can be differentiated by the corresponding statistical distributions produced in the detection process.
Three regimes of statistical distributions can be obtained depending on the properties of the light source: Poissonian
In probability theory and statistics, the Poisson distribution is a discrete probability distribution that expresses the probability of a given number of events occurring in a fixed interval of time or space if these events occur with a known co ...
, super-Poissonian, and sub-Poissonian. The regimes are defined by the relationship between the variance and average number of photon counts for the corresponding distribution. Both Poissonian and super-Poissonian light can be described by a semi-classical theory in which the light source is modeled as an electromagnetic wave and the atom is modeled according to quantum mechanics. In contrast, sub-Poissonian light requires the quantization of the electromagnetic field
The quantization of the electromagnetic field, means that an electromagnetic field consists of discrete energy parcels, photons. Photons are massless particles of definite energy, definite momentum, and definite spin.
To explain the photoelect ...
for a proper description and thus is a direct measure of the particle nature of light.
Poissonian light
In classical electromagnetic theory, an ideal source of light with constant intensity can be modeled by a spatially and temporally coherent electromagnetic wave of a single frequency. Such a light source can be modeled by,M. Fox, ''Quantum Optics: An Introduction'', Oxford University Press, New York, 2006 : where is the frequency of the field and is a time independent phase shift. The analogue in quantum mechanics is the coherent state : By projecting the coherent state onto theFock state
In quantum mechanics, a Fock state or number state is a quantum state that is an element of a Fock space with a well-defined number of particles (or quanta). These states are named after the Soviet physicist Vladimir Fock. Fock states play an imp ...
, we can find the probability of finding photons using the Born rule
The Born rule (also called Born's rule) is a key postulate of quantum mechanics which gives the probability that a measurement of a quantum system will yield a given result. In its simplest form, it states that the probability density of findi ...
, which gives
:
The above result is a Poissonian distribution with which is a distinct feature of the coherent state.
Super-Poissonian light
Light that is governed by super-Poissonian statistics exhibits a statistical distribution with variance . An example of light that exhibits super-Poissonian statistics is thermal light. The intensity of thermal light fluctuates randomly and the fluctuations give rise to super-Poissonian statistics, as shown below by calculating the distribution of the intensity fluctuations.I. Deutsch, Quantum Optics Course Fall 2015, http://info.phys.unm.edu/~ideutsch/Classes/Phys566F15/Lectures/Phys566_Lect02.pdf. Retrieved 9 December 2015 Using the intensity distribution together withMandel's formula Mandel's (a.k.a. Mandel's Shoe Stores and Mandel's Fascinating Slippers) was a chain of shoe stores in the Southwestern United States for many decades of the 20th century. For a time it advertised its wares as "Mandel's Fascinating Slippers". Mauric ...
which describes the probability of the number of photon counts registered by a photodetector, the statistical distribution of photons in thermal light can be obtained.
Thermal light can be modeled as a collection of harmonic oscillators. Suppose the -th oscillator emits an electromagnetic field with phase . Using the theory of superposition of fields, the total field produced by the oscillators is
:
After pulling out all the variables that are independent of the summation index , a random complex amplitude can be defined by
:
where was rewritten in terms of its magnitude and its phase . Because the oscillators are uncorrelated, the phase of the superposed field will be random. Therefore, the complex amplitude is a stochastic variable. It represents the sum of the uncorrelated phases of the oscillators which models the intensity fluctuations in thermal light. On the complex plane, it represents a two dimensional random walker with representing the steps taken. For large a random walker has a Gaussian probability distribution. Thus, the joint probability distribution
Given two random variables that are defined on the same probability space, the joint probability distribution is the corresponding probability distribution on all possible pairs of outputs. The joint distribution can just as well be considere ...
for the real and imaginary parts of the complex random variable can be represented as,
:
:
After steps, the expectation value of the radius squared is . The expectation value which can be thought of as all directions being equally likely. Rewriting the probability distribution in terms of results in
:
With the probability distribution above, we can now find the average intensity of the field (where several constants have been omitted for clarity)
:
:
The instantaneous intensity of the field is given by
:
Because the electric field and thus the intensity are dependent on the stochastic complex variable . The probability of obtaining an intensity in between and is
:
where is the infinitesimal element on the complex plane. This infinitesimal element can be rewritten as
:
The above intensity distribution can now be written as
:
:
:
This last expression represents the intensity distribution for thermal light. The last step in showing thermal light satisfies the variance condition for super-Poisson statistics is to use Mandel's formula. The formula describes the probability of observing n photon counts and is given by
:
The factor where is the quantum efficiency describes the efficiency of the photon counter. A perfect detector would have . is the intensity incident on an area A of the photodetector and is given byJ. W. Goodman, ''Statistical Optics'', Wiley, New York, (1985) 238-256, 466-468
: On substituting the intensity probability distribution of thermal light for P(I), Mandel's formula becomes
:
Using the following formula to evaluate the integral
The probability distribution for n photon counts from a thermal light source is
:
where is the average number of counts. This last distribution is known as the Bose-Einstein distribution. The variance of the distribution can be shown to be
:
In contrast with the Poisson distribution for a coherent light source, the Bose-Einstein distribution has characteristic of thermal light.
On substituting the intensity probability distribution of thermal light for P(I), Mandel's formula becomes
:
Using the following formula to evaluate the integral
The probability distribution for n photon counts from a thermal light source is
:
where is the average number of counts. This last distribution is known as the Bose-Einstein distribution. The variance of the distribution can be shown to be
:
In contrast with the Poisson distribution for a coherent light source, the Bose-Einstein distribution has characteristic of thermal light.
Sub-Poissonian light
 Light that is governed by sub-Poisson statistics cannot be described by classical electromagnetic theory and is defined by . The advent of ultrafast photodetectors has made it possible to measure the sub-Poissonian nature of light. An example of light exhibiting sub-Poissonian statistics is squeezed light. Recently researchers have shown that sub-Poissonian light can be induced in a quantum dot exhibiting resonance fluorescence. A technique used to measure the sub-Poissonian structure of light is a homodyne intensity correlation scheme. In this scheme a local oscillator and signal field are superimposed via a beam splitter. The superimposed light is then split by another beam splitter and each signal is recorded by individual photodetectors connected to correlator from which the intensity correlation can be measured. Evidence of the sub-Poissonian nature of light is shown by obtaining a negative intensity correlation as was shown in.
Light that is governed by sub-Poisson statistics cannot be described by classical electromagnetic theory and is defined by . The advent of ultrafast photodetectors has made it possible to measure the sub-Poissonian nature of light. An example of light exhibiting sub-Poissonian statistics is squeezed light. Recently researchers have shown that sub-Poissonian light can be induced in a quantum dot exhibiting resonance fluorescence. A technique used to measure the sub-Poissonian structure of light is a homodyne intensity correlation scheme. In this scheme a local oscillator and signal field are superimposed via a beam splitter. The superimposed light is then split by another beam splitter and each signal is recorded by individual photodetectors connected to correlator from which the intensity correlation can be measured. Evidence of the sub-Poissonian nature of light is shown by obtaining a negative intensity correlation as was shown in.
References
{{Reflist Optical metrology Photonics