J-invariant

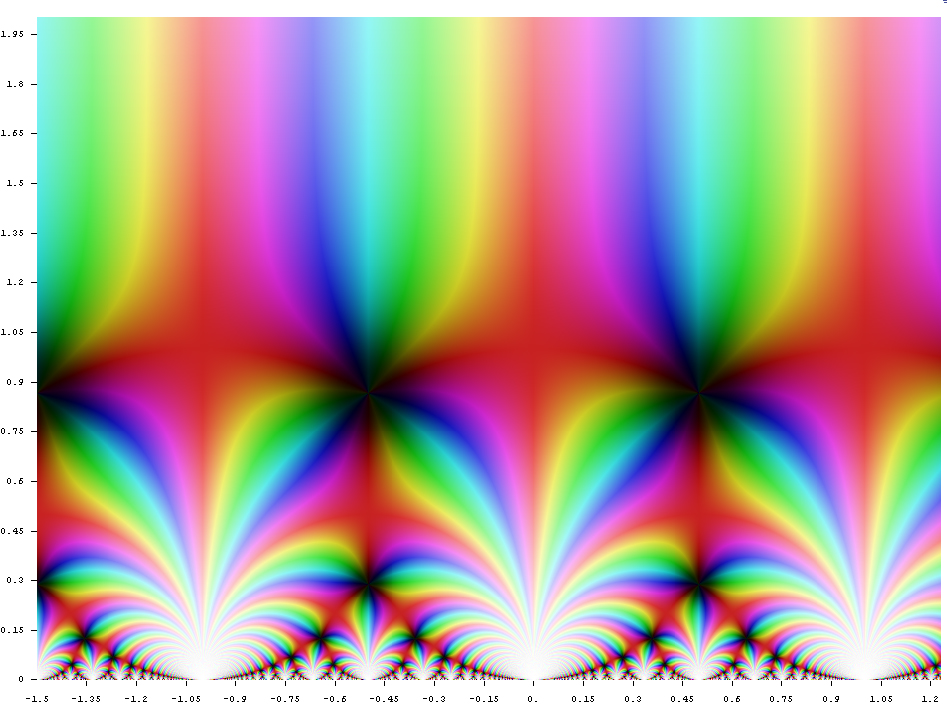In mathematics, Felix Klein's -invariant or function, regarded as a function of a complex variable , is a modular function of weight zero for defined on the upper half-plane of complex numbers. It is the unique such function which is holomorphic away from a simple pole at the cusp such that

:$j\left(e^\right) = 0, \quad j(i) = 1728 = 12^3.$

Rational functions of are modular, and in fact give all modular functions. Classically, the -invariant was studied as a parameterization of elliptic curves over , but it also has surprising connections to the symmetries of the Monster group (this connection is referred to as monstrous moonshine).

Definition

The -invariant can be defined as a function on the upper half-plane

:$j(\tau) = 1728 \frac = 1728 \frac = 1728 \frac$

with the third definition implying $j(\tau)$ can be expressed as a cube, also since 1728 = $12^3$.

The given functions are the modular discriminant $\Delta(\tau) = g_2(\tau)^3 - 27g_3(\tau)^2 = (2\pi)^\,\eta^(\tau)$, Dedekind eta function $\eta(\tau)$, and modular invariants,

:$g_2(\tau) = 60G_4(\tau) = 60\sum_ \left(m + n\tau\right)^$
:$g_3(\tau) = 140G_6(\tau) = 140\sum_ \left(m + n\tau\right)^$

where $G_4(\tau)$, $G_6(\tau)$ are Fourier series,

:\begin
G_4(\tau)&=\frac\, E_4(\tau) \\ ptG_6(\tau)&=\frac\, E_6(\tau)
\end

and $E_4(\tau)$, $E_6(\tau)$ are Eisenstein series,

:\begin
E_4(\tau)&= 1+ 240\sum_^\infty \frac \\ ptE_6(\tau)&= 1- 504\sum_^\infty \frac
\end

and $q=e^$ (the square of the nome). The -invariant can then be directly expressed in terms of the Eisenstein series as,

:$j(\tau) = 1728 \frac$

with no numerical factor other than 1728. This implies a third way to define the modular discriminant,

:$\Delta(\tau) = (2\pi)^\,\frac$

For example, using the definitions above and $\tau = 2i$, then the Dedekind eta function $\eta(2i)$ has the exact value,

:$\eta(2i) = \frac$

implying the transcendental numbers,

:$g_2(2i) = \frac,\qquad g_3(2i) = \frac$

but yielding the algebraic number (in fact, an integer),

:$j(2i) = 1728 \frac = 66^3.$

In general, this can be motivated by viewing each as representing an isomorphism class of elliptic curves. Every elliptic curve over is a complex torus, and thus can be identified with a rank 2 lattice; that is, a two-dimensional lattice of . This lattice can be rotated and scaled (operations that preserve the isomorphism class), so that it is generated by and . This lattice corresponds to the elliptic curve $y^2=4x^3-g_2(\tau)x-g_3(\tau)$ (see Weierstrass elliptic functions).

Note that is defined everywhere in as the modular discriminant is non-zero. This is due to the corresponding cubic polynomial having distinct roots.

The fundamental region

It can be shown that is a modular form of weight twelve, and one of weight four, so that its third power is also of weight twelve. Thus their quotient, and therefore , is a modular function of weight zero, in particular a holomorphic function invariant under the action of . Quotienting out by its centre yields the modular group, which we may identify with the projective special linear group .

By a suitable choice of transformation belonging to this group,

:$\tau \mapsto \frac, \qquad ad-bc =1,$

we may reduce to a value giving the same value for , and lying in the fundamental region for , which consists of values for satisfying the conditions

:$\begin , \tau, &\ge 1 \\ -\tfrac &< \mathfrak(\tau) \le \tfrac \\ -\tfrac &< \mathfrak(\tau) < 0 \Rightarrow , \tau, > 1 \end$

The function when restricted to this region still takes on every value in the complex numbers exactly once. In other words, for every in , there is a unique τ in the fundamental region such that . Thus, has the property of mapping the fundamental region to the entire complex plane.

Additionally two values produce the same elliptic curve iff for some . This means provides a bijection from the set of elliptic curves over to the complex plane.

As a Riemann surface, the fundamental region has genus , and every ( level one) modular function is a rational function in ; and, conversely, every rational function in is a modular function. In other words, the field of modular functions is .

Class field theory and

The -invariant has many remarkable properties:

*If is any CM point, that is, any element of an imaginary quadratic field with positive imaginary part (so that is defined), then is an algebraic integer. These special values are called singular moduli.
* The field extension is abelian, that is, it has an abelian Galois group.
* Let be the lattice in generated by It is easy to see that all of the elements of which fix under multiplication form a ring with units, called an order. The other lattices with generators associated in like manner to the same order define the algebraic conjugates of over . Ordered by inclusion, the unique maximal order in is the ring of algebraic integers of , and values of having it as its associated order lead to unramified extensions of .

These classical results are the starting point for the theory of complex multiplication.

Transcendence properties

In 1937 Theodor Schneider proved the aforementioned result that if is a quadratic irrational number in the upper half plane then is an algebraic integer. In addition he proved that if is an algebraic number but not imaginary quadratic then is transcendental.

The function has numerous other transcendental properties. Kurt Mahler conjectured a particular transcendence result that is often referred to as Mahler's conjecture, though it was proved as a corollary of results by Yu. V. Nesterenko and Patrice Phillipon in the 1990s. Mahler's conjecture was that if was in the upper half plane then and were never both simultaneously algebraic. Stronger results are now known, for example if is algebraic then the following three numbers are algebraically independent, and thus at least two of them transcendental:

:$j(\tau), \frac, \frac$

The -expansion and moonshine

Several remarkable properties of have to do with its -expansion (Fourier series expansion), written as a Laurent series in terms of , which begins:

:$j(\tau) = q^ + 744 + 196884 q + 21493760 q^2 + 864299970 q^3 + 20245856256 q^4 + \cdots$

Note that has a simple pole at the cusp, so its -expansion has no terms below .

All the Fourier coefficients are integers, which results in several almost integers, notably Ramanujan's constant:

:$e^ \approx 640320^3 + 744$.

The asymptotic formula for the coefficient of is given by

:$\frac$,

as can be proved by the Hardy–Littlewood circle method.

Moonshine

More remarkably, the Fourier coefficients for the positive exponents of are the dimensions of the graded part of an infinite-dimensional graded algebra representation of the monster group called the '' moonshine module'' – specifically, the coefficient of is the dimension of grade- part of the moonshine module, the first example being the Griess algebra, which has dimension 196,884, corresponding to the term . This startling observation, first made by John McKay, was the starting point for moonshine theory.

The study of the Moonshine conjecture led John Horton Conway and Simon P. Norton to look at the genus-zero modular functions. If they are normalized to have the form

:$q^ + (q)$

then John G. Thompson showed that there are only a finite number of such functions (of some finite level), and Chris J. Cummins later showed that there are exactly 6486 of them, 616 of which have integral coefficients.

Alternate expressions

We have

:$j(\tau) = \frac$

where and is the modular lambda function

:$\lambda(\tau) = \frac = k^2(\tau)$

a ratio of Jacobi theta functions , and is the square of the elliptic modulus .Chandrasekharan (1985) p.108 The value of is unchanged when is replaced by any of the six values of the cross-ratio:

:$\left\lbrace \right\rbrace$

The branch points of are at , so that is a Belyi function.

Expressions in terms of theta functions

Define the nome and the Jacobi theta function,

:$\vartheta(0; \tau) = \vartheta_(0; \tau) = 1 + 2 \sum_^\infty \left(e^\right)^ = \sum_^\infty q^$

from which one can derive the auxiliary theta functions. Let,

:$\begin a &= \theta_(q) = \vartheta_(0; \tau) \\ b &= \theta_(q) = \vartheta_(0; \tau) \\ c &= \theta_(q) = \vartheta_(0; \tau) \end$

where and are alternative notations, and . Then we have the for modular invariants , ,

:$\begin g_2(\tau) &= \tfrac\pi^4 \left(a^8 + b^8 + c^8\right) \\ g_3(\tau) &= \tfrac\pi^6 \sqrt \\ \end$

and modular discriminant,

:$\Delta = g_2^3-27g_3^2 = (2\pi)^ \left(\tfraca b c\right)^8 = (2\pi)^\eta(\tau)^$

with Dedekind eta function . The can then be rapidly computed,

:$j(\tau) = 1728\frac = 32 \frac$

Algebraic definition

So far we have been considering as a function of a complex variable. However, as an invariant for isomorphism classes of elliptic curves, it can be defined purely algebraically. Let

:$y^2 + a_1 xy + a_3 y = x^3 + a_2 x^2 + a_4 x + a_6$

be a plane elliptic curve over any field. Then we may perform successive transformations to get the above equation into the standard form (note that this transformation can only be made when the characteristic of the field is not equal to 2 or 3). The resulting coefficients are:

:$\begin b_2 &= a_1^2 + 4a_2,\quad &b_4 &= a_1a_3 + 2a_4,\\ b_6 &= a_3^2 + 4a_6,\quad &b_8 &= a_1^2a_6 - a_1a_3a_4 + a_2a_3^2 + 4a_2a_6 - a_4^2,\\ c_4 &= b_2^2 - 24b_4,\quad &c_6 &= -b_2^3 + 36b_2b_4 - 216b_6, \end$

where and . We also have the discriminant

:$\Delta = -b_2^2b_8 + 9b_2b_4b_6 - 8b_4^3 - 27b_6^2.$

The -invariant for the elliptic curve may now be defined as

:$j = \frac$

In the case that the field over which the curve is defined has characteristic different from 2 or 3, this is equal to

:$j = 1728\frac.$

Inverse function

The inverse function of the -invariant can be expressed in terms of the hypergeometric function (see also the article Picard–Fuchs equation). Explicitly, given a number , to solve the equation for can be done in at least four ways.

Method 1: Solving the sextic in ,

:$j(\tau) = \frac = \frac$

where , and is the modular lambda function so the sextic can be solved as a cubic in . Then,

:$\tau = i \ \frac=i\frac$

for any of the six values of , where is the arithmetic–geometric mean.The equality holds if the arithmetic–geometric mean $\operatorname(a,b)$ of complex numbers $a,b$ (such that $a,b\ne 0;a\ne \pm b$) is defined as follows: Let $a_0=a$, $b_0=b$, $a_=(a_n+b_n)/2$, $b_=\pm\sqrt$ where the signs are chosen such that $, a_n-b_n, \le, a_n+b_n,$ for all $n\in\mathbb$. If $, a_n-b_n, =, a_n+b_n,$, the sign is chosen such that $\Im (b_n/a_n)>0$. Then $\operatorname(a,b)=\lim_a_n=\lim_b_n$. When $a,b$ are positive real (with $a\ne b$), this definition coincides with the usual definition of the arithmetic–geometric mean for positive real numbers. Se
The Arithmetic-Geometric Mean of Gauss
by David A. Cox.

Method 2: Solving the quartic in ,

:$j(\tau) = \frac$

then for any of the four roots,

:$\tau = \frac \frac$

Method 3: Solving the cubic in ,

:$j(\tau) = \frac$

then for any of the three roots,

:$\tau = \frac \frac$

Method 4: Solving the quadratic in ,

:$j(\tau)=\frac$

then,

:$\tau = i \ \frac$

One root gives , and the other gives , but since , it makes no difference which is chosen. The latter three methods can be found in Ramanujan's theory of elliptic functions to alternative bases.

The inversion is applied in high-precision calculations of elliptic function periods even as their ratios become unbounded. A related result is the expressibility via quadratic radicals of the values of at the points of the imaginary axis whose magnitudes are powers of 2 (thus permitting compass and straightedge constructions). The latter result is hardly evident since the modular equation for of order 2 is cubic.

Pi formulas

The Chudnovsky brothers found in 1987,.

:$\frac = \frac \sum_^\infty \frac$

a proof of which uses the fact that

:$j\left(\frac\right) = -640320^3.$

For similar formulas, see the Ramanujan–Sato series.

Special values

The -invariant vanishes at the "corner" of the fundamental domain:

:$\quad j\!\left( \tfrac \right) = 0.$

Here are a few more special values given in terms of the alternative notation , the first four well known:

:\begin
J(i) &= J \left( \tfrac \right) = 1 \\
J\left(\sqrti\right) &= \left(\tfrac\right)^3 \\
J(2i) &= \left(\tfrac\right)^3 \\
J\left(2\sqrti\right) &= \tfrac \left(19 + 13\sqrt \right)^3\\
J(4i) &= \tfrac \left(724 + 513\sqrt \right)^3\\
J\left( \tfrac \right) &= \tfrac \left(724 - 513\sqrt \right)^3\\
J\left( \tfrac \right) &= \tfrac \left(19 - 13\sqrt \right)^3\\
J(3i) &= \tfrac \left(2 + \sqrt\right)^2 \left(21 + 20\sqrt\right )^3 \\
J\left(2\sqrti\right) &= \tfrac \left(30 + 17\sqrt\right)^3\\
J\left( \tfrac \right) &= -\tfrac \left(651 + 142\sqrt \right)^3\\
J\left(\tfrac \right) &= \tfrac \left(23 - 4\sqrt\right)^2 \left(-77 + 15\sqrt \right)^3\\
J\left(\sqrti\right) &= \tfrac \left(3 + \sqrt \right)^5 \left( 17 + 7\sqrt + 59\sqrt + 35\sqrt\right)^3\\
J\left( \tfrac \right) &= \tfrac \left(7 + 5\sqrt + 3\sqrt + 2\sqrt \right)^4 \left( 55 + 30\sqrt + 12\sqrt + 10\sqrt \right)^3\\
J\left( \tfrac \right) &= \tfrac \left(7 + 5\sqrt - 3\sqrt - 2\sqrt \right)^4 \left( 55 + 30\sqrt - 12\sqrt - 10\sqrt \right)^3\\
J\left( \tfrac \right) &= \tfrac \left(7 - 5\sqrt + 3\sqrt - 2\sqrt \right)^4 \left( 55 - 30\sqrt + 12\sqrt - 10\sqrt \right)^3\\
J\left( \tfrac \right) &= \tfrac \left(7 - 5\sqrt - 3\sqrt + 2\sqrt \right)^4 \left( 55 - 30\sqrt - 12\sqrt + 10\sqrt \right)^3\\
J\left(\tfrac\right) &= \left(1 - \frac1 \left (13 + \sqrt)\sqrt[3 + (13 - \sqrt) \sqrt[3">.html" ;"title=" (13 + \sqrt)\sqrt[3"> (13 + \sqrt)\sqrt[3 + (13 - \sqrt) \sqrt[38\right]^2\right)^3\\
J(\sqrti) &= \left(1 + \tfrac\left(303 + 220\sqrt + 139\sqrt + 96\sqrt\right)^2 \right)^3 \\
J(7i) &= \left( 1 + \tfrac \sqrt \left(3 + \sqrt \right)^3 \left(13 + 3\sqrt + \left(6 + \sqrt \right)\sqrt right)^2 \right)^3\\
J(8i) &= \left( 1 + \tfrac \sqrt \left (1 + \sqrt \right) \left(123 + 104\sqrt + 88\sqrt + 73\sqrt right)^2 \right)^3\\
J(10i) &= \left(1 + \tfrac\left(2402 + 1607\sqrt + 1074\sqrt + 719\sqrt right)^2 \right)^3\\
J \left( \tfrac \right) &= \left(1 + \tfrac\left(2402 - 1607\sqrt + 1074\sqrt - 719\sqrt right)^2 \right)^3\\
J(2\sqrti) &= \left(1+\tfrac\left(1+\sqrt\right)^5\left(5+\sqrt\right)^5\left(793+907\sqrt+237\sqrt+103\sqrt\right)^2\right)^3\\
J\left( \tfrac \right) &= \left( 1 - 9 \left ( 9892538 + 4424079\sqrt + 1544955\sqrt + 690925\sqrt \right )^2 \right)^3\\
J\left( \tfrac \right) &= \left( 1 - 9 \left ( 22297077 + 9971556\sqrt + \left ( 3571365 + 1597163\sqrt \right ) \sqrt \right)^2 \right)^3\\
\end

Failure to classify elliptic curves over other fields

The $j$-invariant is only sensitive to isomorphism classes of elliptic curves over the complex numbers, or more generally, an algebraically closed field. Over other fields there exist examples of elliptic curves whose $j$-invariant is the same, but are non-isomorphic. For example, let $E_1,E_2$ be the elliptic curves associated to the polynomials

$\begin E_1: &\text y^2 = x^3 - 25x \\ E_2: &\text y^2 = x^3 - 4x, \end$

both having $j$-invariant $1728$. Then, the rational points of $E_2$ can be computed as:

$E_2(\mathbb) = \$

since $x^3 - 4x = x(x^2 - 4) = x(x-2)(x+2).$ There are no rational solutions with $y = a \neq 0$. This can be shown using Cardano's formula to show that in that case the solutions to $x^3 - 4x - a^2$ are all irrational.

On the other hand, on the set of points

$\$

the equation for $E_2$ becomes $36n^2 = -64n^3 + 100n$. Dividing by $4n$ to eliminate the $(0,0)$ solution, the quadratic formula gives the rational solutions:

$n = \frac = \frac.$

If these curves are considered over $\mathbb(\sqrt)$, there is an isomorphism $E_1(\mathbb(\sqrt)) \cong E_2(\mathbb(\sqrt))$ sending

$(x,y)\mapsto (\mu^2x,\mu^3y) \ \text\ \mu = \frac.$

References

Notes

Other

*. Provides a very readable introduction and various interesting identities.
**
*. Provides a variety of interesting algebraic identities, including the inverse as a hypergeometric series.
* Introduces the j-invariant and discusses the related class field theory.
*. Includes a list of the 175 genus-zero modular functions.
*. Provides a short review in the context of modular forms.
*{{citation, first=Theodor, last=Schneider, author-link=Theodor Schneider, title=Arithmetische Untersuchungen elliptischer Integrale, journal=Math. Annalen, volume=113, year=1937, pages=1–13, mr=1513075, doi=10.1007/BF01571618, s2cid=121073687.

Modular forms
Elliptic functions
Moonshine theory