grand antiprism on:
[Wikipedia]
[Google]
[Amazon]
In
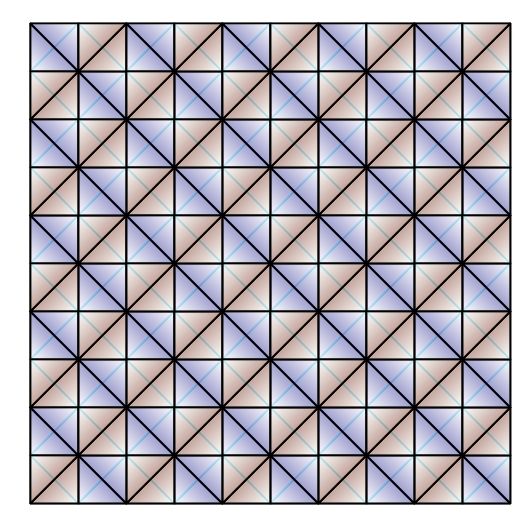 In addition the 300 tetrahedra can be partitioned into 10 disjoint Boerdijk–Coxeter helices of 30 cells each that close back on each other. The two pentagonal antiprism tubes, plus the 10 BC helices, form an irregular discrete Hopf fibration of the grand antiprism that Hopf maps to the faces of a pentagonal antiprism. The two tubes map to the two pentagonal faces and the 10 BC helices map to the 10 triangular faces.
The structure of the grand antiprism is analogous to that of the 3-dimensional
In addition the 300 tetrahedra can be partitioned into 10 disjoint Boerdijk–Coxeter helices of 30 cells each that close back on each other. The two pentagonal antiprism tubes, plus the 10 BC helices, form an irregular discrete Hopf fibration of the grand antiprism that Hopf maps to the faces of a pentagonal antiprism. The two tubes map to the two pentagonal faces and the 10 BC helices map to the 10 triangular faces.
The structure of the grand antiprism is analogous to that of the 3-dimensional
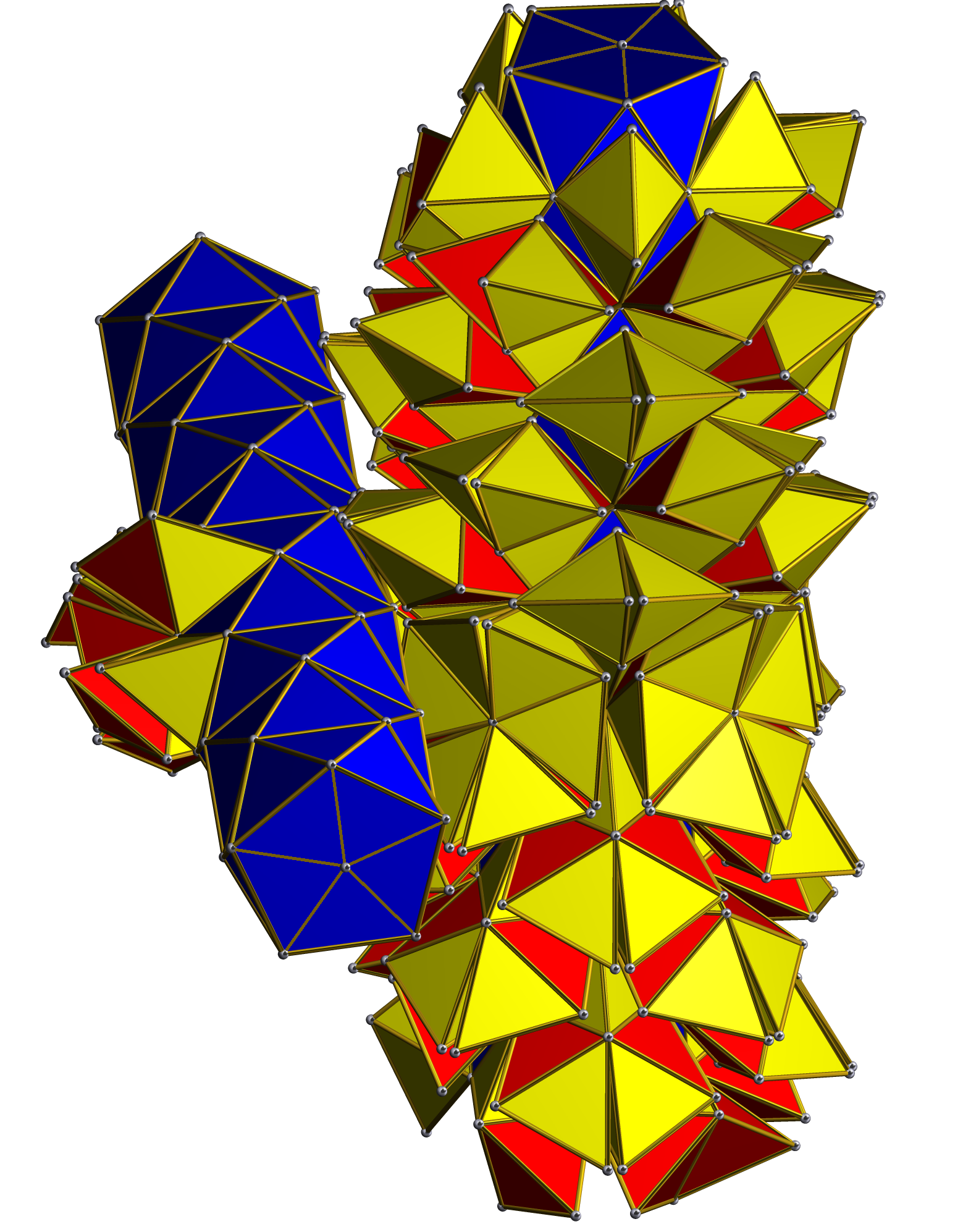 The grand antiprism can be constructed by ''diminishing'' the 600-cell: subtracting 20 pyramids whose bases are three-dimensional pentagonal antiprisms. Conversely, the two rings of pentagonal antiprisms in the grand antiprism may be triangulated by 10 tetrahedra joined to the triangular faces of each antiprism, and a circle of 5 tetrahedra between every pair of antiprisms, joining the 10 tetrahedra of each, yielding 150 tetrahedra per ring. These combined with the 300 tetrahedra that join the two rings together yield the 600 tetrahedra of the 600-cell.
This diminishing may be realized by removing two rings of 10 vertices from the 600-cell, each lying in mutually orthogonal planes. Each ring of removed vertices creates a stack of pentagonal antiprisms on the
The grand antiprism can be constructed by ''diminishing'' the 600-cell: subtracting 20 pyramids whose bases are three-dimensional pentagonal antiprisms. Conversely, the two rings of pentagonal antiprisms in the grand antiprism may be triangulated by 10 tetrahedra joined to the triangular faces of each antiprism, and a circle of 5 tetrahedra between every pair of antiprisms, joining the 10 tetrahedra of each, yielding 150 tetrahedra per ring. These combined with the 300 tetrahedra that join the two rings together yield the 600 tetrahedra of the 600-cell.
This diminishing may be realized by removing two rings of 10 vertices from the 600-cell, each lying in mutually orthogonal planes. Each ring of removed vertices creates a stack of pentagonal antiprisms on the
Kaleidoscopes: Selected Writings of H.S.M. Coxeter
', edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ** (Paper 23) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes II'', ath. Zeit. 188 (1985) 559-5912.8 The Grand Antiprism * * * John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, ''The Symmetries of Things'' 2008, (Chapter 26) The Grand Antiprism
Grand Antiprism and Quaternions
Mehmet Koca, Mudhahir Al-Ajmi, Nazife Ozdes Koca (2009); Mehmet Koca et al. 2009 J. Phys. A: Math. Theor. 42 495201
In the Belly of the Grand Antiprism
(middle section, describing the analogy with the icosahedron and the pentagonal antiprism) {{Polytopes Uniform 4-polytopes
geometry
Geometry (; ) is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. Geometry is, along with arithmetic, one of the oldest branches of mathematics. A mathematician w ...
, the grand antiprism or pentagonal double antiprismoid is a uniform 4-polytope
In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedron, uniform polyhedra, and faces are regular polygons.
There are 47 non-Prism (geometry), prism ...
(4-dimensional uniform polytope
In elementary geometry, a polytope is a geometric object with flat sides ('' faces''). Polytopes are the generalization of three-dimensional polyhedra to any number of dimensions. Polytopes may exist in any general number of dimensions as an ...
) bounded by 320 cells: 20 pentagonal antiprisms, and 300 tetrahedra
In geometry, a tetrahedron (: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular Face (geometry), faces, six straight Edge (geometry), edges, and four vertex (geometry), vertices. The tet ...
. It is an anomalous, non-Wythoffian uniform 4-polytope, discovered in 1965 by John H. Conway and Michael Guy. Topologically, under its highest symmetry, the pentagonal antiprisms have ''D5d'' symmetry and there are two types of tetrahedra, one with ''S4'' symmetry and one with ''Cs'' symmetry.
Alternate names
* Pentagonal double antiprismoid Norman W. Johnson * Gap (Jonathan Bowers: for grand antiprism)Structure
20 stacked pentagonal antiprisms occur in two disjoint rings of 10 antiprisms each. The antiprisms in each ring are joined to each other via their pentagonal faces. The two rings are mutually perpendicular, in a structure similar to aduoprism
In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an -polytope and an -polytope is an -polytope, wher ...
.
The 300 tetrahedra join the two rings to each other, and are laid out in a 2-dimensional arrangement topologically equivalent to the 2-torus and the ridge of the duocylinder. These can be further divided into three sets. 100 face mate to one ring, 100 face mate to the other ring, and 100 are centered at the exact midpoint of the duocylinder and edge mate to both rings. This latter set forms a flat torus and can be "unrolled" into a flat 10×10 square array of tetrahedra that meet only at their edges and vertices. See figure below.  In addition the 300 tetrahedra can be partitioned into 10 disjoint Boerdijk–Coxeter helices of 30 cells each that close back on each other. The two pentagonal antiprism tubes, plus the 10 BC helices, form an irregular discrete Hopf fibration of the grand antiprism that Hopf maps to the faces of a pentagonal antiprism. The two tubes map to the two pentagonal faces and the 10 BC helices map to the 10 triangular faces.
The structure of the grand antiprism is analogous to that of the 3-dimensional
In addition the 300 tetrahedra can be partitioned into 10 disjoint Boerdijk–Coxeter helices of 30 cells each that close back on each other. The two pentagonal antiprism tubes, plus the 10 BC helices, form an irregular discrete Hopf fibration of the grand antiprism that Hopf maps to the faces of a pentagonal antiprism. The two tubes map to the two pentagonal faces and the 10 BC helices map to the 10 triangular faces.
The structure of the grand antiprism is analogous to that of the 3-dimensional antiprism
In geometry, an antiprism or is a polyhedron composed of two Parallel (geometry), parallel Euclidean group, direct copies (not mirror images) of an polygon, connected by an alternating band of triangles. They are represented by the Conway po ...
s. However, the grand antiprism is the only convex uniform analogue of the antiprism in 4 dimensions (although the 16-cell may be regarded as a regular analogue of the ''digonal antiprism
In geometry, an antiprism or is a polyhedron composed of two Parallel (geometry), parallel Euclidean group, direct copies (not mirror images) of an polygon, connected by an alternating band of triangles. They are represented by the Conway po ...
''). The only nonconvex uniform 4-dimensional antiprism analogue uses pentagrammic crossed-antiprisms instead of pentagonal antiprisms, and is called the '' pentagrammic double antiprismoid''.
Vertex figure
The vertex figure of the grand antiprism is a sphenocorona or ''dissected regular icosahedron'': a regular icosahedron with two adjacent vertices removed. In their place 8 triangles are replaced by a pair of trapezoids, edge lengths φ, 1, 1, 1 (where φ is thegolden ratio
In mathematics, two quantities are in the golden ratio if their ratio is the same as the ratio of their summation, sum to the larger of the two quantities. Expressed algebraically, for quantities and with , is in a golden ratio to if
\fr ...
), joined together along their edge of length φ, to give a tetradecahedron whose faces are the 2 trapezoid
In geometry, a trapezoid () in North American English, or trapezium () in British English, is a quadrilateral that has at least one pair of parallel sides.
The parallel sides are called the ''bases'' of the trapezoid. The other two sides are ...
s and the 12 remaining equilateral triangle
An equilateral triangle is a triangle in which all three sides have the same length, and all three angles are equal. Because of these properties, the equilateral triangle is a regular polygon, occasionally known as the regular triangle. It is the ...
s.
Construction
 The grand antiprism can be constructed by ''diminishing'' the 600-cell: subtracting 20 pyramids whose bases are three-dimensional pentagonal antiprisms. Conversely, the two rings of pentagonal antiprisms in the grand antiprism may be triangulated by 10 tetrahedra joined to the triangular faces of each antiprism, and a circle of 5 tetrahedra between every pair of antiprisms, joining the 10 tetrahedra of each, yielding 150 tetrahedra per ring. These combined with the 300 tetrahedra that join the two rings together yield the 600 tetrahedra of the 600-cell.
This diminishing may be realized by removing two rings of 10 vertices from the 600-cell, each lying in mutually orthogonal planes. Each ring of removed vertices creates a stack of pentagonal antiprisms on the
The grand antiprism can be constructed by ''diminishing'' the 600-cell: subtracting 20 pyramids whose bases are three-dimensional pentagonal antiprisms. Conversely, the two rings of pentagonal antiprisms in the grand antiprism may be triangulated by 10 tetrahedra joined to the triangular faces of each antiprism, and a circle of 5 tetrahedra between every pair of antiprisms, joining the 10 tetrahedra of each, yielding 150 tetrahedra per ring. These combined with the 300 tetrahedra that join the two rings together yield the 600 tetrahedra of the 600-cell.
This diminishing may be realized by removing two rings of 10 vertices from the 600-cell, each lying in mutually orthogonal planes. Each ring of removed vertices creates a stack of pentagonal antiprisms on the convex hull
In geometry, the convex hull, convex envelope or convex closure of a shape is the smallest convex set that contains it. The convex hull may be defined either as the intersection of all convex sets containing a given subset of a Euclidean space, ...
. This relationship is analogous to how a pentagonal antiprism can be constructed from an icosahedron
In geometry, an icosahedron ( or ) is a polyhedron with 20 faces. The name comes . The plural can be either "icosahedra" () or "icosahedrons".
There are infinitely many non- similar shapes of icosahedra, some of them being more symmetrical tha ...
by removing two opposite vertices, thereby removing 5 triangles from the opposite 'poles' of the icosahedron, leaving the 10 equatorial triangles and two pentagons on the top and bottom.
(The snub 24-cell
In geometry, the snub 24-cell or snub disicositetrachoron is a convex uniform 4-polytope composed of 120 regular Tetrahedron, tetrahedral and 24 Regular icosahedron, icosahedral cell (mathematics), cells. Five tetrahedra and three icosahedra meet ...
can also be constructed by another diminishing of the 600-cell, removing 24 icosahedral pyramids. Equivalently, this may be realized as taking the convex hull of the vertices remaining after 24 vertices, corresponding to those of an inscribed 24-cell
In four-dimensional space, four-dimensional geometry, the 24-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol . It is also called C24, or the icositetrachoron, octaplex (short for "octa ...
, are removed from the 600-cell.)
Alternatively, it can also be constructed from the decagonal ditetragoltriate (the convex hull of two perpendicular nonuniform 10-10 duoprisms where the ratio of the two decagons are in the golden ratio
In mathematics, two quantities are in the golden ratio if their ratio is the same as the ratio of their summation, sum to the larger of the two quantities. Expressed algebraically, for quantities and with , is in a golden ratio to if
\fr ...
) via an alternation process. The decagonal prisms alternate into pentagonal antiprisms, the rectangular trapezoprisms alternate into tetrahedra
In geometry, a tetrahedron (: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular Face (geometry), faces, six straight Edge (geometry), edges, and four vertex (geometry), vertices. The tet ...
with two new regular tetrahedra
In geometry, a tetrahedron (: tetrahedra or tetrahedrons), also known as a triangular pyramid, is a polyhedron composed of four triangular Face (geometry), faces, six straight Edge (geometry), edges, and four vertex (geometry), vertices. The tet ...
(representing a non-corealmic triangular bipyramid) created at the deleted vertices. This is the only uniform solution for the p-gonal double antiprismoids alongside its conjugate, the pentagrammic double antiprismoid from the decagrammic ditetragoltriate.
Projections
These are two perspective projections, projecting the polytope into ahypersphere
In mathematics, an -sphere or hypersphere is an - dimensional generalization of the -dimensional circle and -dimensional sphere to any non-negative integer .
The circle is considered 1-dimensional and the sphere 2-dimensional because a point ...
, and applying a stereographic projection
In mathematics, a stereographic projection is a perspective transform, perspective projection of the sphere, through a specific point (geometry), point on the sphere (the ''pole'' or ''center of projection''), onto a plane (geometry), plane (th ...
into 3-space.
See also
*600-cell
In geometry, the 600-cell is the convex regular 4-polytope (four-dimensional analogue of a Platonic solid) with Schläfli symbol .
It is also known as the C600, hexacosichoron and hexacosihedroid.
It is also called a tetraplex (abbreviated from ...
* Snub 24-cell
In geometry, the snub 24-cell or snub disicositetrachoron is a convex uniform 4-polytope composed of 120 regular Tetrahedron, tetrahedral and 24 Regular icosahedron, icosahedral cell (mathematics), cells. Five tetrahedra and three icosahedra meet ...
* Uniform 4-polytope
In geometry, a uniform 4-polytope (or uniform polychoron) is a 4-dimensional polytope which is vertex-transitive and whose cells are uniform polyhedron, uniform polyhedra, and faces are regular polygons.
There are 47 non-Prism (geometry), prism ...
* Duoprism
In geometry of 4 dimensions or higher, a double prism or duoprism is a polytope resulting from the Cartesian product of two polytopes, each of two dimensions or higher. The Cartesian product of an -polytope and an -polytope is an -polytope, wher ...
* Duocylinder
Notes
References
*Kaleidoscopes: Selected Writings of H.S.M. Coxeter
', edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ** (Paper 23) H.S.M. Coxeter, ''Regular and Semi-Regular Polytopes II'', ath. Zeit. 188 (1985) 559-5912.8 The Grand Antiprism * * * John H. Conway, Heidi Burgiel, Chaim Goodman-Strauss, ''The Symmetries of Things'' 2008, (Chapter 26) The Grand Antiprism
Grand Antiprism and Quaternions
Mehmet Koca, Mudhahir Al-Ajmi, Nazife Ozdes Koca (2009); Mehmet Koca et al. 2009 J. Phys. A: Math. Theor. 42 495201
External links
In the Belly of the Grand Antiprism
(middle section, describing the analogy with the icosahedron and the pentagonal antiprism) {{Polytopes Uniform 4-polytopes