focal surface on:
[Wikipedia]
[Google]
[Amazon]
For a 
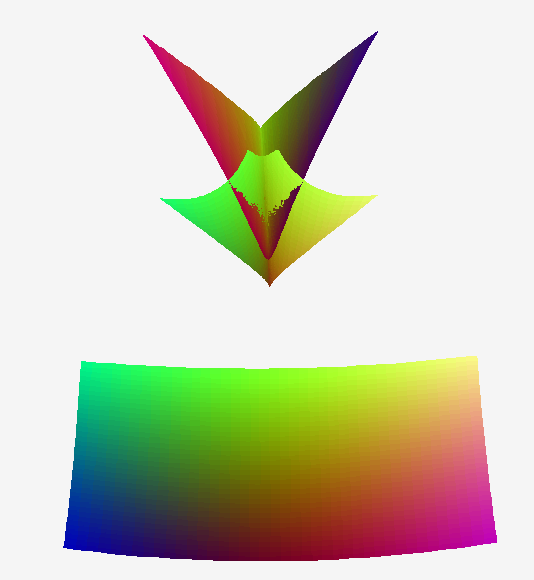As the principal curvatures are the eigenvalues of the second fundamental form, there are two at each point, and these give rise to two points of the focal surface on each
For a surface
A surface, as the term is most generally used, is the outermost or uppermost layer of a physical object or space. It is the portion or region of the object that can first be perceived by an observer using the senses of sight and touch, and is ...
in three dimension the focal surface, surface of centers or evolute is formed by taking the centers of the curvature spheres, which are the tangent
In geometry, the tangent line (or simply tangent) to a plane curve at a given point is, intuitively, the straight line that "just touches" the curve at that point. Leibniz defined it as the line through a pair of infinitely close points o ...
ial sphere
A sphere (from Ancient Greek, Greek , ) is a surface (mathematics), surface analogous to the circle, a curve. In solid geometry, a sphere is the Locus (mathematics), set of points that are all at the same distance from a given point in three ...
s whose radii are the reciprocals of one of the principal curvature
In differential geometry, the two principal curvatures at a given point of a surface (mathematics), surface are the maximum and minimum values of the curvature as expressed by the eigenvalues of the shape operator at that point. They measure how ...
s at the point of tangency. Equivalently it is the surface formed by the centers of the circles which osculate the curvature lines.
As the principal curvatures are the eigenvalues of the second fundamental form, there are two at each point, and these give rise to two points of the focal surface on each normal direction
In geometry, a normal is an object (e.g. a line, ray, or vector) that is perpendicular to a given object. For example, the normal line to a plane curve at a given point is the infinite straight line perpendicular to the tangent line to the cur ...
to the surface. Away from umbilical point
In the differential geometry of surfaces in three dimensions, umbilics or umbilical points are points on a surface that are locally spherical. At such points the normal curvatures in all directions are equal, hence, both principal curvatures are e ...
s, these two points of the focal surface are distinct; at umbilical points the two sheets come together. When the surface has a ridge
A ridge is a long, narrow, elevated geomorphologic landform, structural feature, or a combination of both separated from the surrounding terrain by steep sides. The sides of a ridge slope away from a narrow top, the crest or ridgecrest, wi ...
the focal surface has a cuspidal edge, three such edges pass through an elliptical umbilic and only one through a hyperbolic umbilic. At points where the Gaussian curvature
In differential geometry, the Gaussian curvature or Gauss curvature of a smooth Surface (topology), surface in three-dimensional space at a point is the product of the principal curvatures, and , at the given point:
K = \kappa_1 \kappa_2.
For ...
is zero, one sheet of the focal surface will have a point at infinity corresponding to the zero principal curvature.
If is a point of the given surface, the unit normal and the principal curvature
In differential geometry, the two principal curvatures at a given point of a surface (mathematics), surface are the maximum and minimum values of the curvature as expressed by the eigenvalues of the shape operator at that point. They measure how ...
s at , then
: and
are the corresponding two points of the focal surface.
Special cases
#The focal surface of asphere
A sphere (from Ancient Greek, Greek , ) is a surface (mathematics), surface analogous to the circle, a curve. In solid geometry, a sphere is the Locus (mathematics), set of points that are all at the same distance from a given point in three ...
consists of a single point, its center.
#One part of the focal surface of a surface of revolution
A surface of revolution is a Surface (mathematics), surface in Euclidean space created by rotating a curve (the ''generatrix'') one full revolution (unit), revolution around an ''axis of rotation'' (normally not Intersection (geometry), intersec ...
consists of the axis of rotation.
#The focal surface of a Torus
In geometry, a torus (: tori or toruses) is a surface of revolution generated by revolving a circle in three-dimensional space one full revolution about an axis that is coplanarity, coplanar with the circle. The main types of toruses inclu ...
consists of the directrix circle and the axis of rotation.
#The focal surface of a Dupin cyclide
In mathematics, a Dupin cyclide or cyclide of Dupin is any Inversive geometry, geometric inversion of a standard torus, Cylinder (geometry), cylinder or cone, double cone. In particular, these latter are themselves examples of Dupin cyclides. They ...
consists of a pair of focal conics. The Dupin cyclides are the only surfaces, whose focal surfaces degenerate into two curves.
#One part of the focal surface of a channel surface
In geometry and topology, a channel or canal surface is a surface formed as the Envelope (mathematics), envelope of a family of spheres whose centers lie on a space curve, its ''Generatrix, directrix''. If the radii of the generating spheres ar ...
degenerates to its directrix.
#Two confocal quadrics (for example an ellipsoid and a hyperboloid of one sheet) can be considered as focal surfaces of a surface.Hilbert Cohn-Vossen p. 197.
See also
*Focus (optics)
In geometrical optics, a focus, also called an image point, is a point where ray (optics), light rays originating from a point on the object vergence (optics), converge. Although the focus is conceptually a point, physically the focus has a s ...
*Evolute
In the differential geometry of curves, the evolute of a curve is the locus (mathematics), locus of all its Center of curvature, centers of curvature. That is to say that when the center of curvature of each point on a curve is drawn, the result ...
Notes
References
* . {{DEFAULTSORT:Focal Surface Surfaces