Cone condition on:
[Wikipedia]
[Google]
[Amazon]
 In
In
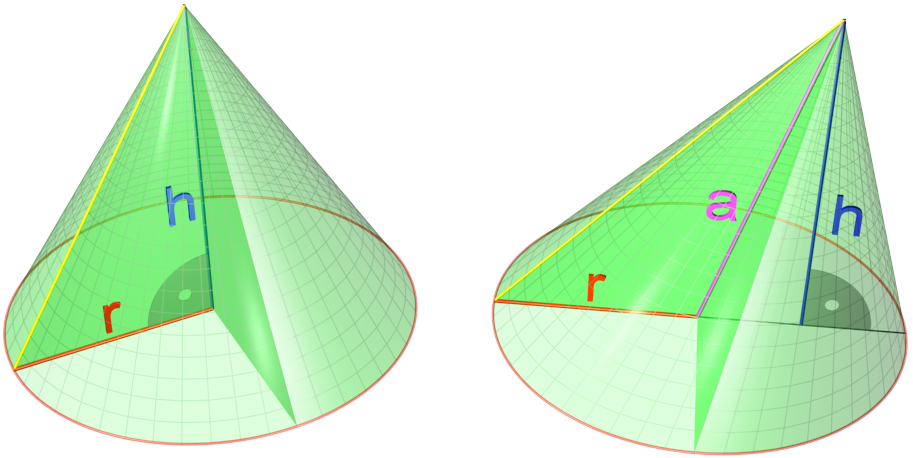
 *Radius and slant height
::
::
:where is the radius and is the slant height.
*Circumference and slant height
::
::
:where is the circumference and is the slant height.
*Apex angle and height
::
::
:where is the apex angle and is the height.
*Radius and slant height
::
::
:where is the radius and is the slant height.
*Circumference and slant height
::
::
:where is the circumference and is the slant height.
*Apex angle and height
::
::
:where is the apex angle and is the height.
 In
In
Spinning Cone
from Maths Is Fun
Paper model cone
Cut a Cone
An interactive demonstration of the intersection of a cone with a plane {{Authority control Elementary shapes Surfaces

 In
In geometry
Geometry (; ) is a branch of mathematics concerned with properties of space such as the distance, shape, size, and relative position of figures. Geometry is, along with arithmetic, one of the oldest branches of mathematics. A mathematician w ...
, a cone is a three-dimensional figure
Solid geometry or stereometry is the geometry of three-dimensional Euclidean space (3D space).
A solid figure is the region of 3D space bounded by a two-dimensional closed surface; for example, a solid ball consists of a sphere and its inte ...
that tapers smoothly from a flat base (typically a circle
A circle is a shape consisting of all point (geometry), points in a plane (mathematics), plane that are at a given distance from a given point, the Centre (geometry), centre. The distance between any point of the circle and the centre is cal ...
) to a point not contained in the base, called the ''apex
The apex is the highest point of something. The word may also refer to:
Arts and media Fictional entities
* Apex (comics)
A-Bomb
Abomination
Absorbing Man
Abraxas
Abyss
Abyss is the name of two characters appearing in Ameri ...
'' or '' vertex''.
A cone is formed by a set of line segment
In geometry, a line segment is a part of a line (mathematics), straight line that is bounded by two distinct endpoints (its extreme points), and contains every Point (geometry), point on the line that is between its endpoints. It is a special c ...
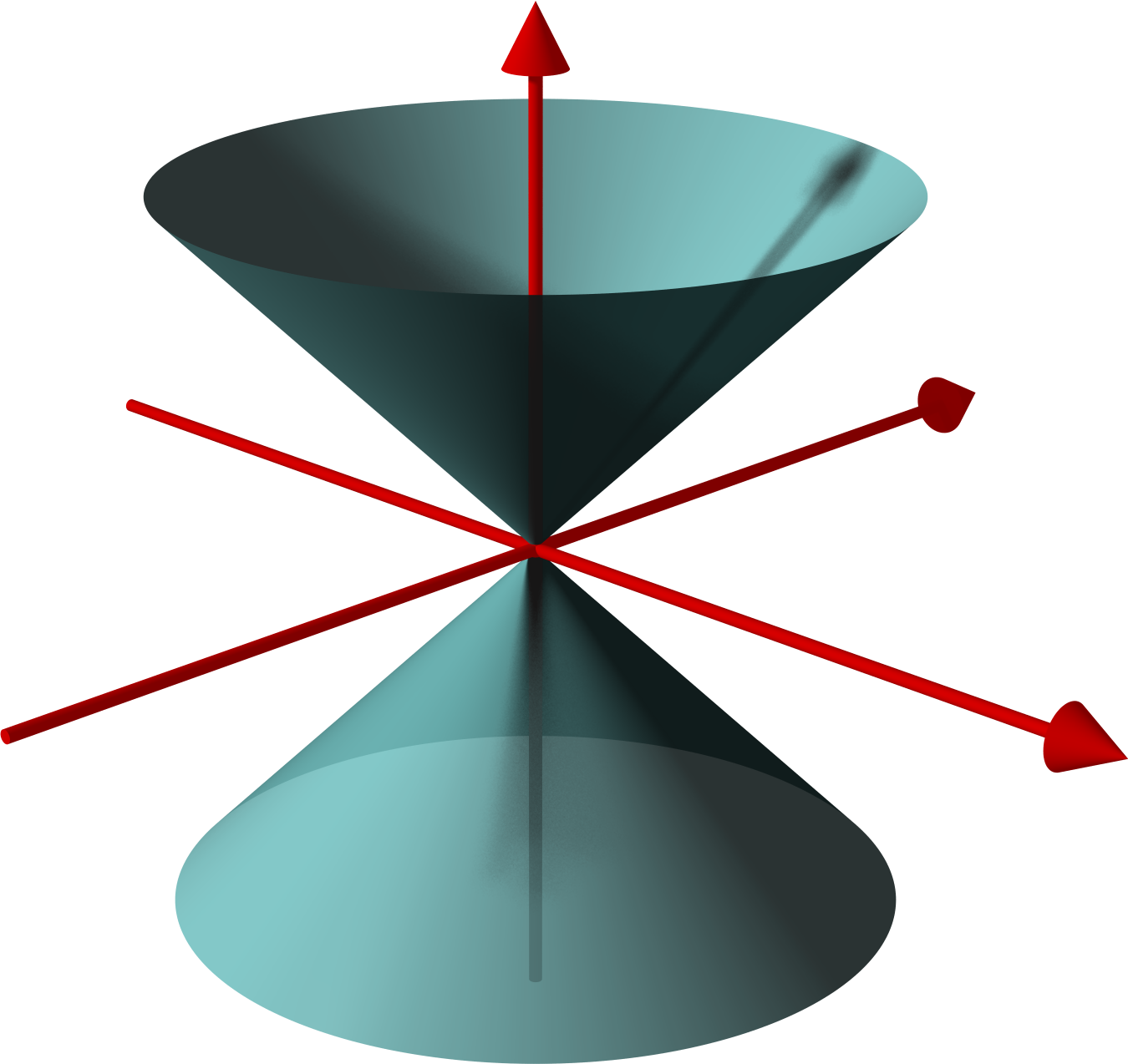
s, half-lines, or lines connecting a common point, the apex, to all of the points on a base. In the case of line segments, the cone does not extend beyond the base, while in the case of half-lines, it extends infinitely far. In the case of lines, the cone extends infinitely far in both directions from the apex, in which case it is sometimes called a ''double cone''. Each of the two halves of a double cone split at the apex is called a ''nappe''.
Depending on the author, the base may be restricted to a circle, any one-dimensional quadratic form
In mathematics, a quadratic form is a polynomial with terms all of degree two (" form" is another name for a homogeneous polynomial). For example,
4x^2 + 2xy - 3y^2
is a quadratic form in the variables and . The coefficients usually belong t ...
in the plane, any closed one-dimensional figure, or any of the above plus all the enclosed points. If the enclosed points are included in the base, the cone is a solid object
Solid geometry or stereometry is the geometry of three-dimensional Euclidean space (3D space).
A solid figure is the region of 3D space bounded by a two-dimensional closed surface; for example, a solid ball consists of a sphere and its inte ...
; otherwise it is an open surface, a two-dimensional
A two-dimensional space is a mathematical space with two dimensions, meaning points have two degrees of freedom: their locations can be locally described with two coordinates or they can move in two independent directions. Common two-dimension ...
object in three-dimensional space. In the case of a solid object, the boundary formed by these lines or partial lines is called the ''lateral surface''; if the lateral surface is unbounded, it is a ''conical surface
In geometry, a conical surface is an unbounded surface in three-dimensional space formed from the union of infinite lines that pass through a fixed point and a space curve.
Definitions
A (''general'') conical surface is the unbounded surface ...
''.
The axis
An axis (: axes) may refer to:
Mathematics
*A specific line (often a directed line) that plays an important role in some contexts. In particular:
** Coordinate axis of a coordinate system
*** ''x''-axis, ''y''-axis, ''z''-axis, common names ...
of a cone is the straight line passing through the apex about which the cone has a circular symmetry
In geometry, circular symmetry is a type of continuous symmetry for a Plane (geometry), planar object that can be rotational symmetry, rotated by any arbitrary angle and map onto itself.
Rotational circular symmetry is isomorphic with the circl ...
. In common usage in elementary geometry, cones are assumed to be ''right circular'', i.e., with a circle base perpendicular
In geometry, two geometric objects are perpendicular if they intersect at right angles, i.e. at an angle of 90 degrees or π/2 radians. The condition of perpendicularity may be represented graphically using the '' perpendicular symbol'', � ...
to the axis. If the cone is right circular the intersection of a plane with the lateral surface is a conic section
A conic section, conic or a quadratic curve is a curve obtained from a cone's surface intersecting a plane. The three types of conic section are the hyperbola, the parabola, and the ellipse; the circle is a special case of the ellipse, tho ...
. In general, however, the base may be any shapeGrünbaum, ''Convex Polytopes
''Convex Polytopes'' is a graduate-level mathematics textbook about convex polytopes, higher-dimensional generalizations of three-dimensional polyhedron, convex polyhedra. It was written by Branko Grünbaum, with contributions from Victor Klee, M ...
'', second edition, p. 23. and the apex may lie anywhere (though it is usually assumed that the base is bounded and therefore has finite area
Area is the measure of a region's size on a surface. The area of a plane region or ''plane area'' refers to the area of a shape or planar lamina, while '' surface area'' refers to the area of an open surface or the boundary of a three-di ...
, and that the apex lies outside the plane of the base). Contrasted with right cones are ''oblique cones'', in which the axis passes through the centre of the base non-perpendicularly.
Depending on context, ''cone'' may refer more narrowly to either a convex cone
In linear algebra, a cone—sometimes called a linear cone to distinguish it from other sorts of cones—is a subset of a real vector space that is closed under positive scalar multiplication; that is, C is a cone if x\in C implies sx\in C for e ...
or projective cone
A projective cone (or just cone) in projective geometry is the union of all lines that intersect a projective subspace ''R'' (the apex of the cone) and an arbitrary subset ''A'' (the basis) of some other subspace ''S'', disjoint from ''R''.
In ...
.
Cones can be generalized to higher dimensions
In physics and mathematics, the dimension of a mathematical space (or object) is informally defined as the minimum number of coordinates needed to specify any point within it. Thus, a line has a dimension of one (1D) because only one coordi ...
.
Further terminology
The perimeter of the base of a cone is called the ''directrix'', and each of the line segments between the directrix and apex is a ''generatrix'' or ''generating line'' of the lateral surface. (For the connection between this sense of the term ''directrix'' and the directrix of a conic section, seeDandelin spheres
In geometry, the Dandelin spheres are one or two spheres that are tangent both to a plane and to a cone that intersects the plane. The intersection of the cone and the plane is a conic section, and the point at which either sphere touches the pl ...
.)
The ''base radius'' of a circular cone is the radius
In classical geometry, a radius (: radii or radiuses) of a circle or sphere is any of the line segments from its Centre (geometry), center to its perimeter, and in more modern usage, it is also their length. The radius of a regular polygon is th ...
of its base; often this is simply called the radius of the cone. The ''aperture'' of a right circular cone is the maximum angle between two generatrix lines; if the generatrix makes an angle ''θ'' to the axis, the aperture is 2''θ''. In optics
Optics is the branch of physics that studies the behaviour and properties of light, including its interactions with matter and the construction of optical instruments, instruments that use or Photodetector, detect it. Optics usually describes t ...
, the angle ''θ'' is called the ''half-angle'' of the cone, to distinguish it from the aperture.
A cone with a region including its apex cut off by a plane is called a ''truncated cone''; if the truncation
In mathematics and computer science, truncation is limiting the number of digits right of the decimal point.
Truncation and floor function
Truncation of positive real numbers can be done using the floor function. Given a number x \in \mathbb ...
plane is parallel to the cone's base, it is called a ''frustum
In geometry, a ; (: frusta or frustums) is the portion of a polyhedron, solid (normally a pyramid (geometry), pyramid or a cone (geometry), cone) that lies between two parallel planes cutting the solid. In the case of a pyramid, the base faces a ...
''. An '' elliptical cone'' is a cone with an elliptical base. A ''generalized cone'' is the surface created by the set of lines passing through a vertex and every point on a boundary (see Visual hull).
Measurements and equations
Volume
Thevolume
Volume is a measure of regions in three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch) ...
of any conic solid is one third of the product of the area of the base and the height
In modern mathematics, this formula can easily be computed using calculus — it is, up to scaling, the integral
Without using calculus, the formula can be proven by comparing the cone to a pyramid and applying Cavalieri's principle
In geometry, Cavalieri's principle, a modern implementation of the method of indivisibles, named after Bonaventura Cavalieri, is as follows:
* 2-dimensional case: Suppose two regions in a plane are included between two parallel lines in that pl ...
– specifically, comparing the cone to a (vertically scaled) right square pyramid, which forms one third of a cube. This formula cannot be proven without using such infinitesimal arguments – unlike the 2-dimensional formulae for polyhedral area, though similar to the area of the circle – and hence admitted less rigorous proofs before the advent of calculus, with the ancient Greeks using the method of exhaustion
The method of exhaustion () is a method of finding the area of a shape by inscribing inside it a sequence of polygons (one at a time) whose areas converge to the area of the containing shape. If the sequence is correctly constructed, the differ ...
. This is essentially the content of Hilbert's third problem
The third of Hilbert's problems, Hilbert's list of mathematical problems, presented in 1900, was the first to be solved. The problem is related to the following question: given any two polyhedron, polyhedra of equal volume, is it always possible t ...
– more precisely, not all polyhedral pyramids are ''scissors congruent'' (can be cut apart into finite pieces and rearranged into the other), and thus volume cannot be computed purely by using a decomposition argument.
Center of mass
Thecenter of mass
In physics, the center of mass of a distribution of mass in space (sometimes referred to as the barycenter or balance point) is the unique point at any given time where the weight function, weighted relative position (vector), position of the d ...
of a conic solid of uniform density lies one-quarter of the way from the center of the base to the vertex, on the straight line joining the two.
Right circular cone
Volume
For a circular cone with radius and height , the base is a circle of area thus the formula for volume is:Slant height
The slant height of a right circular cone is the distance from any point on thecircle
A circle is a shape consisting of all point (geometry), points in a plane (mathematics), plane that are at a given distance from a given point, the Centre (geometry), centre. The distance between any point of the circle and the centre is cal ...
of its base to the apex via a line segment along the surface of the cone. It is given by , where is the radius
In classical geometry, a radius (: radii or radiuses) of a circle or sphere is any of the line segments from its Centre (geometry), center to its perimeter, and in more modern usage, it is also their length. The radius of a regular polygon is th ...
of the base and is the height. This can be proved by the Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposite t ...
.
Surface area
The lateral surface area of a right circular cone is where is the radius of the circle at the bottom of the cone and is the slant height of the cone. The surface area of the bottom circle of a cone is the same as for any circle, . Thus, the total surface area of a right circular cone can be expressed as each of the following: *Radius and height :: :(the area of the base plus the area of the lateral surface; the term is the slant height) :: :where is the radius and is the height.Circular sector
Thecircular sector
A circular sector, also known as circle sector or disk sector or simply a sector (symbol: ⌔), is the portion of a disk (a closed region bounded by a circle) enclosed by two radii and an arc, with the smaller area being known as the ''minor ...
is obtained by unfolding the surface of one nappe of the cone:
*radius ''R''
::
*arc length ''L''
::
*central angle ''φ'' in radians
::
Equation form
The surface of a cone can be parameterized as : where is the angle "around" the cone, and is the "height" along the cone. A right solid circular cone with height and aperture , whose axis is the coordinate axis and whose apex is the origin, is described parametrically as : where range over , , and , respectively. In Implicit function, implicit form, the same solid is defined by the inequalities : where : More generally, a right circular cone with vertex at the origin, axis parallel to the vector , and aperture , is given by the implicitvector
Vector most often refers to:
* Euclidean vector, a quantity with a magnitude and a direction
* Disease vector, an agent that carries and transmits an infectious pathogen into another living organism
Vector may also refer to:
Mathematics a ...
equation where
:
:
where , and denotes the dot product
In mathematics, the dot product or scalar productThe term ''scalar product'' means literally "product with a Scalar (mathematics), scalar as a result". It is also used for other symmetric bilinear forms, for example in a pseudo-Euclidean space. N ...
.
Projective geometry
 In
In projective geometry
In mathematics, projective geometry is the study of geometric properties that are invariant with respect to projective transformations. This means that, compared to elementary Euclidean geometry, projective geometry has a different setting (''p ...
, a cylinder
A cylinder () has traditionally been a three-dimensional solid, one of the most basic of curvilinear geometric shapes. In elementary geometry, it is considered a prism with a circle as its base.
A cylinder may also be defined as an infinite ...
is simply a cone whose apex is at infinity. Intuitively, if one keeps the base fixed and takes the limit as the apex goes to infinity, one obtains a cylinder, the angle of the side increasing as arctan
In mathematics, the inverse trigonometric functions (occasionally also called ''antitrigonometric'', ''cyclometric'', or ''arcus'' functions) are the inverse functions of the trigonometric functions, under suitably restricted domains. Specific ...
, in the limit forming a right angle
In geometry and trigonometry, a right angle is an angle of exactly 90 Degree (angle), degrees or radians corresponding to a quarter turn (geometry), turn. If a Line (mathematics)#Ray, ray is placed so that its endpoint is on a line and the ad ...
. This is useful in the definition of degenerate conic
In geometry, a degenerate conic is a conic (a second-degree plane curve, defined by a polynomial equation of degree two) that fails to be an irreducible curve. This means that the defining equation is factorable over the complex numbers (or more ...
s, which require considering the cylindrical conics.
According to G. B. Halsted, a cone is generated similarly to a Steiner conic only with a projectivity and axial pencils (not in perspective) rather than the projective ranges used for the Steiner conic:
"If two copunctual non-costraight axial pencils are projective but not perspective, the meets of correlated planes form a 'conic surface of the second order', or 'cone'." G. B. Halsted (1906) ''Synthetic Projective Geometry'', page 20
Generalizations
The definition of a cone may be extended to higher dimensions; seeconvex cone
In linear algebra, a cone—sometimes called a linear cone to distinguish it from other sorts of cones—is a subset of a real vector space that is closed under positive scalar multiplication; that is, C is a cone if x\in C implies sx\in C for e ...
. In this case, one says that a convex set
In geometry, a set of points is convex if it contains every line segment between two points in the set.
For example, a solid cube (geometry), cube is a convex set, but anything that is hollow or has an indent, for example, a crescent shape, is n ...
''C'' in the real vector space
In mathematics and physics, a vector space (also called a linear space) is a set (mathematics), set whose elements, often called vector (mathematics and physics), ''vectors'', can be added together and multiplied ("scaled") by numbers called sc ...
is a cone (with apex at the origin) if for every vector ''x'' in ''C'' and every nonnegative real number ''a'', the vector ''ax'' is in ''C''. In this context, the analogues of circular cones are not usually special; in fact one is often interested in polyhedral cones.
An even more general concept is the topological cone, which is defined in arbitrary topological spaces.
See also
*Bicone
In geometry, a bicone or dicone (from , and Greek: ''di-'', both meaning "two") is the three-dimensional surface of revolution of a rhombus around one of its axes of symmetry. Equivalently, a bicone is the surface created by joining two con ...
* Cone (linear algebra)
In linear algebra, a cone—sometimes called a linear cone to distinguish it from other sorts of cones—is a subset of a real vector space that is closed under positive scalar multiplication; that is, C is a cone if x\in C implies sx\in C for e ...
* Cylinder (geometry)
A cylinder () has traditionally been a three-dimensional solid, one of the most basic of curvilinear geometric shapes. In elementary geometry, it is considered a prism with a circle as its base.
A cylinder may also be defined as an infinite ...
* Democritus
Democritus (, ; , ''Dēmókritos'', meaning "chosen of the people"; – ) was an Ancient Greece, Ancient Greek Pre-Socratic philosophy, pre-Socratic philosopher from Abdera, Thrace, Abdera, primarily remembered today for his formulation of an ...
* Elliptic cone
* Generalized conic
* Hyperboloid
In geometry, a hyperboloid of revolution, sometimes called a circular hyperboloid, is the surface generated by rotating a hyperbola around one of its principal axes. A hyperboloid is the surface obtained from a hyperboloid of revolution by def ...
* List of shapes
* Pyrometric cone
Pyrometric cones are pyrometric devices that are used to gauge heatwork during the firing of ceramic materials in a kiln. The cones, often used in sets of three, are positioned in a kiln with the wares to be fired and, because the individual c ...
* Quadric
In mathematics, a quadric or quadric surface is a generalization of conic sections (ellipses, parabolas, and hyperbolas). In three-dimensional space, quadrics include ellipsoids, paraboloids, and hyperboloids.
More generally, a quadric hype ...
* Rotation of axes
In mathematics, a rotation of axes in two dimensions is a mapping from an ''xy''-Cartesian coordinate system to an ''x′y′''-Cartesian coordinate system in which the origin is kept fixed and the ''x′'' and ''y′'' axes ar ...
* Ruled surface
In geometry, a Differential geometry of surfaces, surface in 3-dimensional Euclidean space is ruled (also called a scroll) if through every Point (geometry), point of , there is a straight line that lies on . Examples include the plane (mathemat ...
* Translation of axes
In mathematics, a translation of axes in two dimensions is a mapping from an ''xy''-Cartesian coordinate system to an ''x'y-Cartesian coordinate system in which the ''x axis is parallel to the ''x'' axis and ''k'' units away, and the ''y' ...
Notes
References
*External links
* * * * An interactivSpinning Cone
from Maths Is Fun
Paper model cone
Cut a Cone
An interactive demonstration of the intersection of a cone with a plane {{Authority control Elementary shapes Surfaces