5-simplex T2 on:
[Wikipedia]
[Google]
[Amazon]
3,3,3,3 symmetry, order 1440. The intersection of these two 5-simplexes is a uniform birectified 5-simplex. = ∩ .
:
Polytopes of Various Dimensions
Jonathan Bowers
{{Polytopes 5-polytopes
In five-dimensional
3,2,3, order 72. It represents joining of 2 orthogonal triangles, orthogonally offset, with all pairs of vertices connected between.
The form ∨∨ has symmetry [2,2,2, order 48.
These are seen in the
and tritruncated regular 6-polytopes, like a bitruncated 6-cube and a tritruncated 6-simplex. The edge labels here represent the types of face along that direction, and thus represent different edge lengths.
The vertex figure of the omnitruncated 5-simplex honeycomb, , is a 5-simplx with a geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, a 5-simplex
In geometry, a simplex (plural: simplexes or simplices) is a generalization of the notion of a triangle or tetrahedron to arbitrary dimensions. The simplex is so-named because it represents the simplest possible polytope in any given dimension ...
is a self-dual regular
The term regular can mean normal or in accordance with rules. It may refer to:
People
* Moses Regular (born 1971), America football player
Arts, entertainment, and media Music
* "Regular" (Badfinger song)
* Regular tunings of stringed instrum ...
5-polytope
In geometry, a five-dimensional polytope (or 5-polytope) is a polytope in five-dimensional space, bounded by (4-polytope) facets, pairs of which share a polyhedral cell.
Definition
A 5-polytope is a closed five-dimensional figure with vertices ...
. It has six vertices, 15 edge
Edge or EDGE may refer to:
Technology Computing
* Edge computing, a network load-balancing system
* Edge device, an entry point to a computer network
* Adobe Edge, a graphical development application
* Microsoft Edge, a web browser developed b ...
s, 20 triangle faces, 15 tetrahedral cells
Cell most often refers to:
* Cell (biology), the functional basic unit of life
Cell may also refer to:
Locations
* Monastic cell, a small room, hut, or cave in which a religious recluse lives, alternatively the small precursor of a monastery w ...
, and 6 5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
facets. It has a dihedral angle
A dihedral angle is the angle between two intersecting planes or half-planes. In chemistry, it is the clockwise angle between half-planes through two sets of three atoms, having two atoms in common. In solid geometry, it is defined as the un ...
of cos−1(), or approximately 78.46°.
The 5-simplex is a solution to the problem: ''Make 20 equilateral triangles using 15 matchsticks, where each side of every triangle is exactly one matchstick.''
Alternate names
It can also be called a hexateron, or hexa-5-tope, as a 6- facetted polytope in 5-dimensions. The name ''hexateron'' is derived from ''hexa-'' for having six facets and ''teron
Teron is one of the five clans of the Karbi people in Northeast India and Bangladesh. Sometimes pronounce as ''Toron'' in Dumra
Dumra is a town and a notified area in Sitamarhi district in Bihar, India. Dumra is the headquarter of Sitamar ...
'' (with ''ter-'' being a corruption of ''tetra-
Numeral or number prefixes are prefixes derived from numerals or occasionally other numbers. In English and many other languages, they are used to coin numerous series of words. For example:
* unicycle, bicycle, tricycle (1-cycle, 2-cycle, 3-cy ...
'') for having four-dimensional facets.
By Jonathan Bowers, a hexateron is given the acronym hix.
As a configuration
This configuration matrix represents the 5-simplex. The rows and columns correspond to vertices, edges, faces, cells and 4-faces. The diagonal numbers say how many of each element occur in the whole 5-simplex. The nondiagonal numbers say how many of the column's element occur in or at the row's element. This self-dual simplex's matrix is identical to its 180 degree rotation.Regular hexateron cartesian coordinates
The ''hexateron'' can be constructed from a5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
by adding a 6th vertex such that it is equidistant from all the other vertices of the 5-cell.
The Cartesian coordinates
A Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of numerical coordinates, which are the signed distances to the point from two fixed perpendicular oriented lines, measured i ...
for the vertices of an origin-centered regular hexateron having edge length 2 are:
:
The vertices of the ''5-simplex'' can be more simply positioned on a hyperplane
In geometry, a hyperplane is a subspace whose dimension is one less than that of its '' ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hype ...
in 6-space as permutations of (0,0,0,0,0,1) ''or'' (0,1,1,1,1,1). These construction can be seen as facets of the 6-orthoplex or rectified 6-cube
In six-dimensional geometry, a rectified 6-cube is a convex uniform 6-polytope, being a rectification of the regular 6-cube.
There are unique 6 degrees of rectifications, the zeroth being the 6-cube, and the 6th and last being the 6-orthoplex. ...
respectively.
Projected images
Lower symmetry forms
A lower symmetry form is a ''5-cell pyramid'' ( )v, with ,3,3symmetry order 120, constructed as a5-cell
In geometry, the 5-cell is the convex 4-polytope with Schläfli symbol . It is a 5-vertex four-dimensional object bounded by five tetrahedral cells. It is also known as a C5, pentachoron, pentatope, pentahedroid, or tetrahedral pyramid. It is ...
base in a 4-space hyperplane
In geometry, a hyperplane is a subspace whose dimension is one less than that of its '' ambient space''. For example, if a space is 3-dimensional then its hyperplanes are the 2-dimensional planes, while if the space is 2-dimensional, its hype ...
, and an apex point ''above'' the hyperplane. The five ''sides'' of the pyramid are made of 5-cell cells. These are seen as vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
s of truncated regular 6-polytopes, like a truncated 6-cube.
Another form is ∨, with ,3,3symmetry order 48, the joining of an orthogonal digon and a tetrahedron, orthogonally offset, with all pairs of vertices connected between. Another form is ∨, with ,2,3
The comma is a punctuation mark that appears in several variants in different languages. It has the same shape as an apostrophe or single closing quotation mark () in many typefaces, but it differs from them in being placed on the baseline ...
symmetry order 36, and extended symmetry vertex figure
In geometry, a vertex figure, broadly speaking, is the figure exposed when a corner of a polyhedron or polytope is sliced off.
Definitions
Take some corner or vertex of a polyhedron. Mark a point somewhere along each connected edge. Draw lines ...
s of Bitruncation">bitruncated
In geometry, a bitruncation is an operation on regular polytopes. It represents a truncation beyond rectification. The original edges are lost completely and the original faces remain as smaller copies of themselves.
Bitruncated regular polyt ...petrie polygon
In geometry, a Petrie polygon for a regular polytope of dimensions is a skew polygon in which every consecutive sides (but no ) belongs to one of the facets. The Petrie polygon of a regular polygon is the regular polygon itself; that of a ...
cycle of 5 long edges.
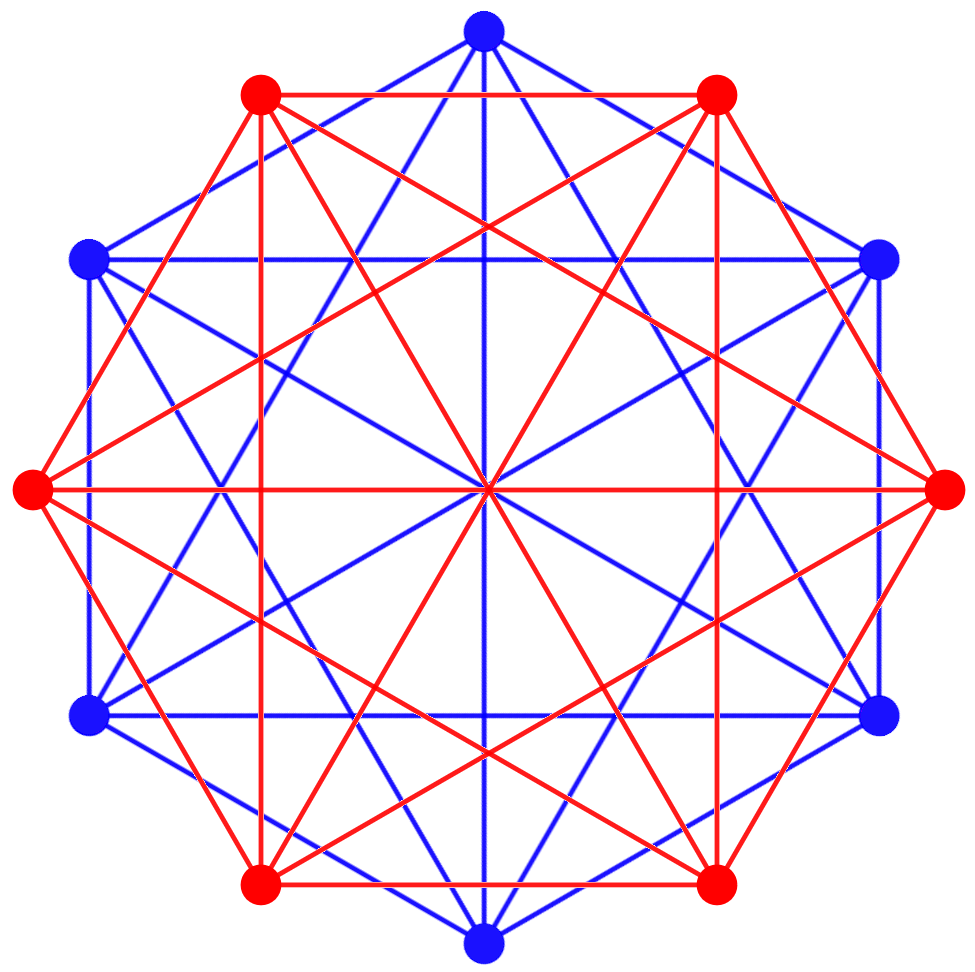
Compound
The compound of two 5-simplexes in dual configurations can be seen in this A6Coxeter plane
In mathematics, the Coxeter number ''h'' is the order of a Coxeter element of an irreducible Coxeter group. It is named after H.S.M. Coxeter.
Definitions
Note that this article assumes a finite Coxeter group. For infinite Coxeter groups, there a ...
projection, with a red and blue 5-simplex vertices and edges. This compound has 
Related uniform 5-polytopes
It is first in a dimensional series of uniform polytopes and honeycombs, expressed byCoxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
as 13k series. A degenerate 4-dimensional case exists as 3-sphere tiling, a tetrahedral hosohedron.
It is first in a dimensional series of uniform polytopes and honeycombs, expressed by Coxeter
Harold Scott MacDonald "Donald" Coxeter, (9 February 1907 – 31 March 2003) was a British and later also Canadian geometer. He is regarded as one of the greatest geometers of the 20th century.
Biography
Coxeter was born in Kensington to ...
as 3k1 series. A degenerate 4-dimensional case exists as 3-sphere tiling, a tetrahedral dihedron.
The 5-simplex, as 220 polytope is first in dimensional series 22k.
The regular 5-simplex is one of 19 uniform polytera based on the ,3,3,3Coxeter group
In mathematics, a Coxeter group, named after H. S. M. Coxeter, is an abstract group that admits a formal description in terms of reflections (or kaleidoscopic mirrors). Indeed, the finite Coxeter groups are precisely the finite Euclidean ref ...
, all shown here in A5 Coxeter plane
In mathematics, the Coxeter number ''h'' is the order of a Coxeter element of an irreducible Coxeter group. It is named after H.S.M. Coxeter.
Definitions
Note that this article assumes a finite Coxeter group. For infinite Coxeter groups, there a ...
orthographic projection
Orthographic projection (also orthogonal projection and analemma) is a means of representing three-dimensional objects in two dimensions. Orthographic projection is a form of parallel projection in which all the projection lines are orthogonal ...
s. (Vertices are colored by projection overlap order, red, orange, yellow, green, cyan, blue, purple having progressively more vertices)
See also
* 11-cellNotes
References
* * Coxeter, H.S.M.: ** ** *** (Paper 22) *** (Paper 23) *** (Paper 24) * * **External links
*Polytopes of Various Dimensions
Jonathan Bowers
{{Polytopes 5-polytopes