|
Antiprism
In geometry, an antiprism or is a polyhedron composed of two parallel direct copies (not mirror images) of an polygon, connected by an alternating band of triangles. They are represented by the Conway notation . Antiprisms are a subclass of prismatoids, and are a (degenerate) type of snub polyhedron. Antiprisms are similar to prisms, except that the bases are twisted relatively to each other, and that the side faces (connecting the bases) are triangles, rather than quadrilaterals. The dual polyhedron of an -gonal antiprism is an -gonal trapezohedron. History At the intersection of modern-day graph theory and coding theory, the triangulation of a set of points have interested mathematicians since Isaac Newton, who fruitlessly sought a mathematical proof of the kissing number problem in 1694. The existence of antiprisms was discussed, and their name was coined by Johannes Kepler, though it is possible that they were previously known to Archimedes, as they satisfy ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
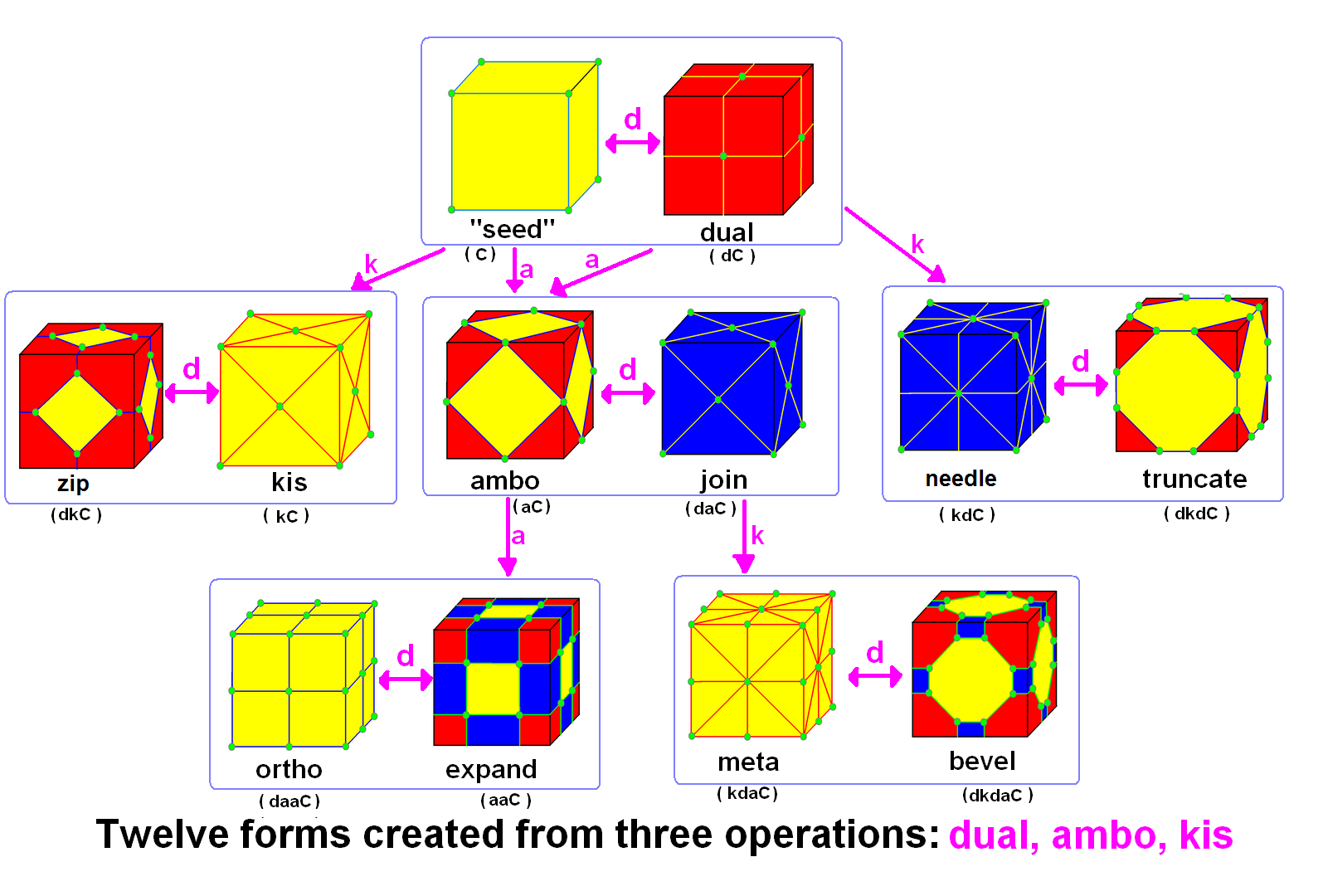
Conway Polyhedron Notation
In geometry, Conway polyhedron notation, invented by John Horton Conway and promoted by George W. Hart, is used to describe polyhedra based on a seed polyhedron modified by various prefix operations. Conway and Hart extended the idea of using operators, like truncation as defined by Kepler, to build related polyhedra of the same symmetry. For example, represents a truncated cube, and , parsed as , is (topologically) a truncated cuboctahedron. The simplest operator dual swaps vertex and face elements; e.g., a dual cube is an octahedron: . Applied in a series, these operators allow many higher order polyhedra to be generated. Conway defined the operators (ambo), (bevel), ( dual), (expand), (gyro), (join), (kis), (meta), (ortho), (snub), and ( truncate), while Hart added ( reflect) and (propellor). Later implementations named further operators, sometimes referred to as "extended" operators. Conway's basic operations are sufficient to generate the Archimedean and Cat ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hexagonal Antiprism
In geometry, the hexagonal antiprism is the 4th in an infinite set of antiprisms formed by an even-numbered sequence of triangle sides closed by two polygon caps. Antiprisms are similar to prisms except the bases are twisted relative to each other, and that the side faces are triangles, rather than quadrilaterals. In the case of a regular 6-sided base, one usually considers the case where its copy is twisted by an angle . Extra regularity is obtained by the line connecting the base centers being perpendicular to the base planes, making it a right antiprism. As faces, it has the two bases and, connecting those bases, isosceles triangles. If faces are all regular, it is a semiregular polyhedron. Crossed antiprism A crossed hexagonal antiprism is a star polyhedron, topologically identical to the convex ''hexagonal antiprism'' with the same vertex arrangement, but it can't be made uniform; the sides are isosceles triangles. Its vertex configuration is 3.3/2.3.6, with one tri ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Prismatoid
In geometry, a prismatoid is a polyhedron whose vertices all lie in two parallel planes. Its lateral faces can be trapezoids or triangles. If both planes have the same number of vertices, and the lateral faces are either parallelograms or trapezoids, it is called a prismoid. Volume If the areas of the two parallel faces are and , the cross-sectional area of the intersection of the prismatoid with a plane midway between the two parallel faces is , and the height (the distance between the two parallel faces) is , then the volume of the prismatoid is given by V = \fracB. E. Meserve, R. E. Pingry: ''Some Notes on the Prismoidal Formula''. The Mathematics Teacher, Vol. 45, No. 4 (April 1952), pp. 257-263 (This formula follows immediately by integrating the area parallel to the two planes of vertices by Simpson's rule, since that rule is exact for integration of polynomials of degree up to 3, and in this case the area is at most a quadratic function in the height.) Prismatoid famili ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Regular Polygon
In Euclidean geometry, a regular polygon is a polygon that is direct equiangular (all angles are equal in measure) and equilateral (all sides have the same length). Regular polygons may be either convex, star or skew. In the limit, a sequence of regular polygons with an increasing number of sides approximates a circle, if the perimeter or area is fixed, or a regular apeirogon (effectively a straight line), if the edge length is fixed. General properties ''These properties apply to all regular polygons, whether convex or star.'' A regular ''n''-sided polygon has rotational symmetry of order ''n''. All vertices of a regular polygon lie on a common circle (the circumscribed circle); i.e., they are concyclic points. That is, a regular polygon is a cyclic polygon. Together with the property of equal-length sides, this implies that every regular polygon also has an inscribed circle or incircle that is tangent to every side at the midpoint. Thus a regular polygon is a tangen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Trapezohedron
In geometry, an trapezohedron, -trapezohedron, -antidipyramid, -antibipyramid, or -deltohedron is the dual polyhedron of an antiprism. The faces of an are congruent and symmetrically staggered; they are called ''twisted kites''. With a higher symmetry, its faces are ''kites'' (also called ''trapezoids'', or ''deltoids''). The "" part of the name does not refer to faces here, but to two arrangements of each vertices around an axis of symmetry. The dual antiprism has two actual faces. An trapezohedron can be dissected into two equal pyramids and an antiprism. Terminology These figures, sometimes called deltohedra, must not be confused with deltahedra, whose faces are equilateral triangles. ''Twisted'' ''trigonal'', ''tetragonal'', and ''hexagonal trapezohedra'' (with six, eight, and twelve ''twisted'' congruent kite faces) exist as crystals; in crystallography (describing the crystal habits of minerals), they are just called ''trigonal'', ''tetragonal'', and ''he ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Regular Polygon
In Euclidean geometry, a regular polygon is a polygon that is direct equiangular (all angles are equal in measure) and equilateral (all sides have the same length). Regular polygons may be either convex, star or skew. In the limit, a sequence of regular polygons with an increasing number of sides approximates a circle, if the perimeter or area is fixed, or a regular apeirogon (effectively a straight line), if the edge length is fixed. General properties ''These properties apply to all regular polygons, whether convex or star.'' A regular ''n''-sided polygon has rotational symmetry of order ''n''. All vertices of a regular polygon lie on a common circle (the circumscribed circle); i.e., they are concyclic points. That is, a regular polygon is a cyclic polygon. Together with the property of equal-length sides, this implies that every regular polygon also has an inscribed circle or incircle that is tangent to every side at the midpoint. Thus a regular polygon is a tangen ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Prism (geometry)
In geometry, a prism is a polyhedron comprising an polygon base, a second base which is a translated copy (rigidly moved without rotation) of the first, and other faces, necessarily all parallelograms, joining corresponding sides of the two bases. All cross-sections parallel to the bases are translations of the bases. Prisms are named after their bases, e.g. a prism with a pentagonal base is called a pentagonal prism. Prisms are a subclass of prismatoids. Like many basic geometric terms, the word ''prism'' () was first used in Euclid's Elements. Euclid defined the term in Book XI as “a solid figure contained by two opposite, equal and parallel planes, while the rest are parallelograms”. However, this definition has been criticized for not being specific enough in relation to the nature of the bases, which caused confusion among later geometry writers. Oblique prism An oblique prism is a prism in which the joining edges and faces are ''not perpendicular'' to the bas ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Polyhedron
In geometry, a polyhedron (plural polyhedra or polyhedrons; ) is a three-dimensional shape with flat polygonal faces, straight edges and sharp corners or vertices. A convex polyhedron is the convex hull of finitely many points, not all on the same plane. Cubes and pyramids are examples of convex polyhedra. A polyhedron is a 3-dimensional example of a polytope, a more general concept in any number of dimensions. Definition Convex polyhedra are well-defined, with several equivalent standard definitions. However, the formal mathematical definition of polyhedra that are not required to be convex has been problematic. Many definitions of "polyhedron" have been given within particular contexts,. some more rigorous than others, and there is not universal agreement over which of these to choose. Some of these definitions exclude shapes that have often been counted as polyhedra (such as the self-crossing polyhedra) or include shapes that are often not considered as valid polyh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Uniform Polyhedron
In geometry, a uniform polyhedron has regular polygons as faces and is vertex-transitive (i.e., there is an isometry mapping any vertex onto any other). It follows that all vertices are congruent. Uniform polyhedra may be regular (if also face- and edge-transitive), quasi-regular (if also edge-transitive but not face-transitive), or semi-regular (if neither edge- nor face-transitive). The faces and vertices need not be convex, so many of the uniform polyhedra are also star polyhedra. There are two infinite classes of uniform polyhedra, together with 75 other polyhedra: *Infinite classes: ** prisms, ** antiprisms. * Convex exceptional: ** 5 Platonic solids: regular convex polyhedra, ** 13 Archimedean solids: 2 quasiregular and 11 semiregular convex polyhedra. * Star (nonconvex) exceptional: ** 4 Kepler–Poinsot polyhedra: regular nonconvex polyhedra, ** 53 uniform star polyhedra: 14 quasiregular and 39 semiregular. Hence 5 + 13 + 4 + 53 = 75. There are also many ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Point (geometry)
In classical Euclidean geometry, a point is a primitive notion that models an exact location in space, and has no length, width, or thickness. In modern mathematics, a point refers more generally to an element of some set called a space. Being a primitive notion means that a point cannot be defined in terms of previously defined objects. That is, a point is defined only by some properties, called axioms, that it must satisfy; for example, ''"there is exactly one line that passes through two different points"''. Points in Euclidean geometry Points, considered within the framework of Euclidean geometry, are one of the most fundamental objects. Euclid originally defined the point as "that which has no part". In two-dimensional Euclidean space, a point is represented by an ordered pair (, ) of numbers, where the first number conventionally represents the horizontal and is often denoted by , and the second number conventionally represents the vertical and is often denoted by ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Set (mathematics)
A set is the mathematical model for a collection of different things; a set contains ''elements'' or ''members'', which can be mathematical objects of any kind: numbers, symbols, points in space, lines, other geometrical shapes, variables, or even other sets. The set with no element is the empty set; a set with a single element is a singleton. A set may have a finite number of elements or be an infinite set. Two sets are equal if they have precisely the same elements. Sets are ubiquitous in modern mathematics. Indeed, set theory, more specifically Zermelo–Fraenkel set theory, has been the standard way to provide rigorous foundations for all branches of mathematics since the first half of the 20th century. History The concept of a set emerged in mathematics at the end of the 19th century. The German word for set, ''Menge'', was coined by Bernard Bolzano in his work '' Paradoxes of the Infinite''. Georg Cantor, one of the founders of set theory, gave the following ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Triangulation
In trigonometry and geometry, triangulation is the process of determining the location of a point by forming triangles to the point from known points. Applications In surveying Specifically in surveying, triangulation involves only angle measurements at known points, rather than measuring distances to the point directly as in trilateration; the use of both angles and distance measurements is referred to as triangulateration. In computer vision Computer stereo vision and optical 3D measuring systems use this principle to determine the spatial dimensions and the geometry of an item. Basically, the configuration consists of two sensors observing the item. One of the sensors is typically a digital camera device, and the other one can also be a camera or a light projector. The projection centers of the sensors and the considered point on the object's surface define a (spatial) triangle. Within this triangle, the distance between the sensors is the base ''b'' and must be k ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |