|
Natural Process Variation
Natural process variation, sometimes just called process variation, is the statistical description of natural fluctuations in process outputs. Equations The following equations are used for an ''x''-bar-control chart: :\bar x = \sum_^n x_i/n :\sigma_ = \sigma/\sqrt n In the example, with ''n'' = 10 samples, the targeted mean, \bar x, and standard error of the mean, \sigma_ are: :\bar x = 1 :\sigma_ = 0.1/\sqrt 10 = 0.0316 That is, independent 10-sample means should themselves have a standard deviation of 0.0316. It is natural that the means vary this much, for by the central limit theorem the means should have a normal distribution, regardless of the distribution of the samples themselves. The importance of knowing the natural process variation becomes clear when we apply statistical process control. In a stable process, the mean is on target; in the example, the target is the filling, set to 1 litre. The variation within the upper and lower control limits (UCL a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistics
Statistics (from German: '' Statistik'', "description of a state, a country") is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data. In applying statistics to a scientific, industrial, or social problem, it is conventional to begin with a statistical population or a statistical model to be studied. Populations can be diverse groups of people or objects such as "all people living in a country" or "every atom composing a crystal". Statistics deals with every aspect of data, including the planning of data collection in terms of the design of surveys and experiments.Dodge, Y. (2006) ''The Oxford Dictionary of Statistical Terms'', Oxford University Press. When census data cannot be collected, statisticians collect data by developing specific experiment designs and survey samples. Representative sampling assures that inferences and conclusions can reasonably extend from the sample to the population as a whole. An ex ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Control Chart
Control charts is a graph used in production control to determine whether quality and manufacturing processes are being controlled under stable conditions. (ISO 7870-1) The hourly status is arranged on the graph, and the occurrence of abnormalities is judged based on the presence of data that differs from the conventional trend or deviates from the control limit line. Control charts are classified into Shewhart individuals control chart (ISO 7870-2) and CUSUM(CUsUM)(or cumulative sum control chart)(ISO 7870-4). Control charts, also known as Shewhart charts (after Walter A. Shewhart) or process-behavior charts, are a statistical process control tool used to determine if a manufacturing or business process is in a state of control. It is more appropriate to say that the control charts are the graphical device for Statistical Process Monitoring (SPM). Traditional control charts are mostly designed to monitor process parameters when the underlying form of the process distributio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mean
There are several kinds of mean in mathematics, especially in statistics. Each mean serves to summarize a given group of data, often to better understand the overall value ( magnitude and sign) of a given data set. For a data set, the '' arithmetic mean'', also known as "arithmetic average", is a measure of central tendency of a finite set of numbers: specifically, the sum of the values divided by the number of values. The arithmetic mean of a set of numbers ''x''1, ''x''2, ..., x''n'' is typically denoted using an overhead bar, \bar. If the data set were based on a series of observations obtained by sampling from a statistical population, the arithmetic mean is the '' sample mean'' (\bar) to distinguish it from the mean, or expected value, of the underlying distribution, the '' population mean'' (denoted \mu or \mu_x).Underhill, L.G.; Bradfield d. (1998) ''Introstat'', Juta and Company Ltd.p. 181/ref> Outside probability and statistics, a wide range of other notions of m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Standard Error (statistics)
The standard error (SE) of a statistic (usually an estimate of a parameter) is the standard deviation of its sampling distribution or an estimate of that standard deviation. If the statistic is the sample mean, it is called the standard error of the mean (SEM). The sampling distribution of a mean is generated by repeated sampling from the same population and recording of the sample means obtained. This forms a distribution of different means, and this distribution has its own mean and variance. Mathematically, the variance of the sampling mean distribution obtained is equal to the variance of the population divided by the sample size. This is because as the sample size increases, sample means cluster more closely around the population mean. Therefore, the relationship between the standard error of the mean and the standard deviation is such that, for a given sample size, the standard error of the mean equals the standard deviation divided by the square root of the sample siz ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Standard Deviation
In statistics, the standard deviation is a measure of the amount of variation or dispersion of a set of values. A low standard deviation indicates that the values tend to be close to the mean (also called the expected value) of the set, while a high standard deviation indicates that the values are spread out over a wider range. Standard deviation may be abbreviated SD, and is most commonly represented in mathematical texts and equations by the lower case Greek letter σ (sigma), for the population standard deviation, or the Latin letter '' s'', for the sample standard deviation. The standard deviation of a random variable, sample, statistical population, data set, or probability distribution is the square root of its variance. It is algebraically simpler, though in practice less robust, than the average absolute deviation. A useful property of the standard deviation is that, unlike the variance, it is expressed in the same unit as the data. The standard deviation o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
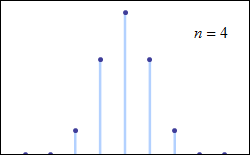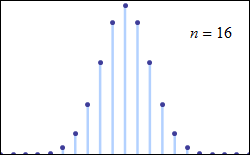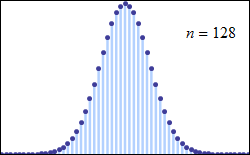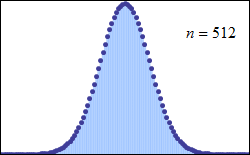
Central Limit Theorem
In probability theory, the central limit theorem (CLT) establishes that, in many situations, when independent random variables are summed up, their properly normalized sum tends toward a normal distribution even if the original variables themselves are not normally distributed. The theorem is a key concept in probability theory because it implies that probabilistic and statistical methods that work for normal distributions can be applicable to many problems involving other types of distributions. This theorem has seen many changes during the formal development of probability theory. Previous versions of the theorem date back to 1811, but in its modern general form, this fundamental result in probability theory was precisely stated as late as 1920, thereby serving as a bridge between classical and modern probability theory. If X_1, X_2, \dots, X_n, \dots are random samples drawn from a population with overall mean \mu and finite variance and if \bar_n is the sample mea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Normal Distribution
In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu is the mean or expectation of the distribution (and also its median and mode), while the parameter \sigma is its standard deviation. The variance of the distribution is \sigma^2. A random variable with a Gaussian distribution is said to be normally distributed, and is called a normal deviate. Normal distributions are important in statistics and are often used in the natural and social sciences to represent real-valued random variables whose distributions are not known. Their importance is partly due to the central limit theorem. It states that, under some conditions, the average of many samples (observations) of a random variable with finite mean and variance is itself a random variable—whose distribution converges to a normal dist ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Statistical Process Control
Statistical process control (SPC) or statistical quality control (SQC) is the application of statistical methods to monitor and control the quality of a production process. This helps to ensure that the process operates efficiently, producing more specification-conforming products with less waste (rework or scrap). SPC can be applied to any process where the "conforming product" (product meeting specifications) output can be measured. Key tools used in SPC include run charts, control charts, a focus on continuous improvement, and the design of experiments. An example of a process where SPC is applied is manufacturing lines. SPC must be practiced in two phases: The first phase is the initial establishment of the process, and the second phase is the regular production use of the process. In the second phase, a decision of the period to be examined must be made, depending upon the change in 5M&E conditions (Man, Machine, Material, Method, Movement, Environment) and wear rate ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Western Electric
The Western Electric Company was an American electrical engineering and manufacturing company officially founded in 1869. A wholly owned subsidiary of American Telephone & Telegraph for most of its lifespan, it served as the primary equipment manufacturer, supplier, and purchasing agent for the Bell System from 1881 to 1984 when it was dismantled. The company was responsible for many technological innovations as well as developments in industrial management. History In 1856, George Shawk, a craftsman and telegraph maker, purchased an electrical engineering business in Cleveland, Ohio. In January, 1869, Shawk had partnered with Enos M. Barton in the former Western Union repair shop of Cleveland, to manufacture burglar, fire alarms, and other electrical items. Both men were former Western Union employees. Shawk, was the Cleveland shop foreman and Barton, was a Rochester, New York telegrapher. During this Shawk and Barton partnership, one customer was an inventor sourcing parts and ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Process Variation
Natural process variation, sometimes just called process variation, is the statistical description of natural fluctuations in process outputs. Equations The following equations are used for an ''x''-bar-control chart: :\bar x = \sum_^n x_i/n :\sigma_ = \sigma/\sqrt n In the example, with ''n'' = 10 samples, the targeted mean, \bar x, and standard error of the mean, \sigma_ are: :\bar x = 1 :\sigma_ = 0.1/\sqrt 10 = 0.0316 That is, independent 10-sample means should themselves have a standard deviation of 0.0316. It is natural that the means vary this much, for by the central limit theorem the means should have a normal distribution In statistics, a normal distribution or Gaussian distribution is a type of continuous probability distribution for a real-valued random variable. The general form of its probability density function is : f(x) = \frac e^ The parameter \mu i ..., regardless of the distribution of the samples themselves. The importance of knowin ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Variance
In probability theory and statistics, variance is the expectation of the squared deviation of a random variable from its population mean or sample mean. Variance is a measure of dispersion, meaning it is a measure of how far a set of numbers is spread out from their average value. Variance has a central role in statistics, where some ideas that use it include descriptive statistics, statistical inference, hypothesis testing, goodness of fit, and Monte Carlo sampling. Variance is an important tool in the sciences, where statistical analysis of data is common. The variance is the square of the standard deviation, the second central moment of a distribution, and the covariance of the random variable with itself, and it is often represented by \sigma^2, s^2, \operatorname(X), V(X), or \mathbb(X). An advantage of variance as a measure of dispersion is that it is more amenable to algebraic manipulation than other measures of dispersion such as the expected absolute deviatio ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |