Umbilic on:
[Wikipedia]
[Google]
[Amazon]
 In the
In the
Image:TensorStar.png, Star
Image:TensorMonstar.png, Monstar
Image:TensorLemon.png, Lemon
 Any surface with an isolated umbilic point at the origin can be expressed as a Monge form parameterisation , where is the unique principal curvature. The type of umbilic is classified by the cubic form from the cubic part and corresponding Jacobian cubic form. Whilst principal directions are not uniquely defined at an umbilic the limits of the principal directions when following a ridge on the surface can be found and these correspond to the root-lines of the cubic form. The pattern of lines of curvature is determined by the Jacobian.
The classification of umbilic points is as follows:
*Inside inner deltoid - elliptical umbilics
**On inner circle - two ridge lines tangent
*On inner deltoid - parabolic umbilics
*Outside inner deltoid - hyperbolic umbilics
**Inside outer circle - star pattern
**On outer circle - birth of umbilics
**Between outer circle and outer deltoid - monstar pattern
**Outside outer deltoid - lemon pattern
* Cusps of the inner deltoid - cubic (symbolic) umbilics
*On the diagonals and the horizontal line - symmetrical umbilics with mirror symmetry
In a generic family of surfaces umbilics can be created, or destroyed, in pairs: the ''birth of umbilics'' transition. Both umbilics will be hyperbolic, one with a star pattern and one with a monstar pattern. The outer circle in the diagram, a right angle cubic form, gives these transitional cases. Symbolic umbilics are a special case of this.
Any surface with an isolated umbilic point at the origin can be expressed as a Monge form parameterisation , where is the unique principal curvature. The type of umbilic is classified by the cubic form from the cubic part and corresponding Jacobian cubic form. Whilst principal directions are not uniquely defined at an umbilic the limits of the principal directions when following a ridge on the surface can be found and these correspond to the root-lines of the cubic form. The pattern of lines of curvature is determined by the Jacobian.
The classification of umbilic points is as follows:
*Inside inner deltoid - elliptical umbilics
**On inner circle - two ridge lines tangent
*On inner deltoid - parabolic umbilics
*Outside inner deltoid - hyperbolic umbilics
**Inside outer circle - star pattern
**On outer circle - birth of umbilics
**Between outer circle and outer deltoid - monstar pattern
**Outside outer deltoid - lemon pattern
* Cusps of the inner deltoid - cubic (symbolic) umbilics
*On the diagonals and the horizontal line - symmetrical umbilics with mirror symmetry
In a generic family of surfaces umbilics can be created, or destroyed, in pairs: the ''birth of umbilics'' transition. Both umbilics will be hyperbolic, one with a star pattern and one with a monstar pattern. The outer circle in the diagram, a right angle cubic form, gives these transitional cases. Symbolic umbilics are a special case of this.

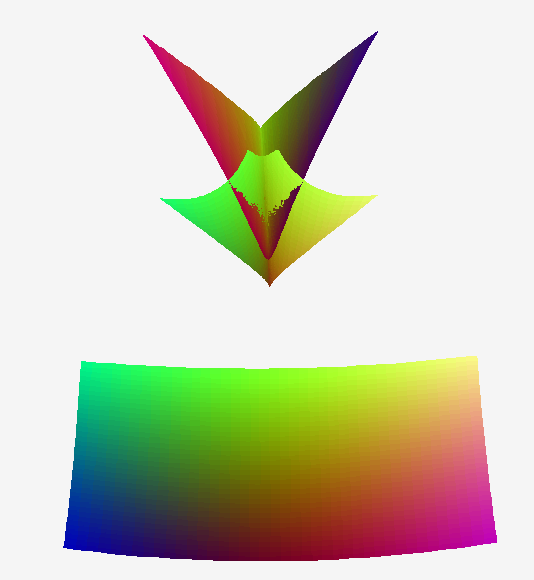 The elliptical umbilics and hyperbolic umbilics have distinctly different focal surfaces. A ridge on the surface corresponds to a cuspidal edges so each sheet of the elliptical focal surface will have three cuspidal edges which come together at the umbilic focus and then switch to the other sheet. For a hyperbolic umbilic there is a single cuspidal edge which switch from one sheet to the other.
The elliptical umbilics and hyperbolic umbilics have distinctly different focal surfaces. A ridge on the surface corresponds to a cuspidal edges so each sheet of the elliptical focal surface will have three cuspidal edges which come together at the umbilic focus and then switch to the other sheet. For a hyperbolic umbilic there is a single cuspidal edge which switch from one sheet to the other.
Volume IVolume IIVolume IIIVolume IV
, publisher=Gauthier-Villars
Differential geometry of surfaces Surfaces
differential geometry of surfaces
In mathematics, the differential geometry of surfaces deals with the differential geometry of smooth surfaces with various additional structures, most often, a Riemannian metric.
Surfaces have been extensively studied from various perspective ...
in three dimensions, umbilics or umbilical points are points on a surface that are locally spherical. At such points the normal curvatures in all directions are equal, hence, both principal curvature
In differential geometry, the two principal curvatures at a given point of a surface are the maximum and minimum values of the curvature as expressed by the eigenvalues of the shape operator at that point. They measure how the surface bends b ...
s are equal, and every tangent vector is a ''principal direction''. The name "umbilic" comes from the Latin ''umbilicus'' (navel
The navel (clinically known as the umbilicus, commonly known as the belly button or tummy button) is a protruding, flat, or hollowed area on the abdomen at the attachment site of the umbilical cord. All placental mammals have a navel, although ...
).
Umbilic points generally occur as isolated points in the elliptical region of the surface; that is, where the Gaussian curvature is positive.
The sphere
A sphere () is a geometrical object that is a three-dimensional analogue to a two-dimensional circle. A sphere is the set of points that are all at the same distance from a given point in three-dimensional space.. That given point is th ...
is the only surface with non-zero curvature where every point is umbilic. A flat umbilic is an umbilic with zero Gaussian curvature. The monkey saddle
In mathematics, the monkey saddle is the surface defined by the equation
: z = x^3 - 3xy^2, \,
or in cylindrical coordinates
:z = \rho^3 \cos(3\varphi).
It belongs to the class of saddle surfaces, and its name derives from the observation tha ...
is an example of a surface with a flat umbilic and on the plane
Plane(s) most often refers to:
* Aero- or airplane, a powered, fixed-wing aircraft
* Plane (geometry), a flat, 2-dimensional surface
Plane or planes may also refer to:
Biology
* Plane (tree) or ''Platanus'', wetland native plant
* ''Planes' ...
every point is a flat umbilic. A torus
In geometry, a torus (plural tori, colloquially donut or doughnut) is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle.
If the axis of revolution does not tou ...
can have no umbilics, but every closed surface of nonzero Euler characteristic, embedded smoothly into Euclidean space
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's ''Elements'', it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean ...
, has at least one umbilic. An unproven conjecture of Constantin Carathéodory
Constantin Carathéodory ( el, Κωνσταντίνος Καραθεοδωρή, Konstantinos Karatheodori; 13 September 1873 – 2 February 1950) was a Greek mathematician who spent most of his professional career in Germany. He made significant ...
states that every smooth topological sphere in Euclidean space has at least two umbilics.
The three main types of umbilic points are elliptical umbilics, parabolic umbilics and hyperbolic umbilics. Elliptical umbilics have the three ridge lines passing through the umbilic and hyperbolic umbilics have just one. Parabolic umbilics are a transitional case with two ridges one of which is singular. Other configurations are possible for transitional cases. These cases correspond to the ''D''4−, ''D''5 and ''D''4+ elementary catastrophes of René Thom's catastrophe theory.
Umbilics can also be characterised by the pattern of the principal direction vector field around the umbilic which typically form one of three configurations: star, lemon, and lemonstar (or monstar). The index of the vector field is either −½ (star) or ½ (lemon, monstar). Elliptical and parabolic umbilics always have the star pattern, whilst hyperbolic umbilics can be star, lemon, or monstar. This classification was first due to Darboux and the names come from Hannay.
For surfaces with genus
Genus ( plural genera ) is a taxonomic rank used in the biological classification of living and fossil organisms as well as viruses. In the hierarchy of biological classification, genus comes above species and below family. In binomial nom ...
0 with isolated umbilics, e.g. an ellipsoid, the index of the principal direction vector field must be 2 by the Poincaré–Hopf theorem. Generic genus 0 surfaces have at least four umbilics of index ½. An ellipsoid of revolution has two non-generic umbilics each of which has index 1.Porteous, p 208
Classification of umbilics
Cubic forms
The classification of umbilics is closely linked to the classification of real cubic forms . A cubic form will have a number of root lines such that the cubic form is zero for all real . There are a number of possibilities including: *Three distinct lines: an ''elliptical cubic form'', standard model . *Three lines, two of which are coincident: a ''parabolic cubic form'', standard model . *A single real line: a ''hyperbolic cubic form'', standard model . *Three coincident lines, standard model . The equivalence classes of such cubics under uniform scaling form a three-dimensional real projective space and the subset of parabolic forms define a surface – called the umbilic bracelet by Christopher Zeeman. Taking equivalence classes under rotation of the coordinate system removes one further parameter and a cubic forms can be represent by the complex cubic form with a single complex parameter . Parabolic forms occur when , the inner deltoid, elliptical forms are inside the deltoid and hyperbolic one outside. If and is not a cube root of unity then the cubic form is a ''right-angled cubic form'' which play a special role for umbilics. If then two of the root lines are orthogonal. A second cubic form, the ''Jacobian'' is formed by taking theJacobian determinant
In vector calculus, the Jacobian matrix (, ) of a vector-valued function of several variables is the matrix of all its first-order partial derivatives. When this matrix is square, that is, when the function takes the same number of variables ...
of the vector valued function , . Up to a constant multiple this is the cubic form . Using complex numbers the Jacobian is a parabolic cubic form when , the outer deltoid in the classification diagram.
Umbilic classification
Focal surface

 The elliptical umbilics and hyperbolic umbilics have distinctly different focal surfaces. A ridge on the surface corresponds to a cuspidal edges so each sheet of the elliptical focal surface will have three cuspidal edges which come together at the umbilic focus and then switch to the other sheet. For a hyperbolic umbilic there is a single cuspidal edge which switch from one sheet to the other.
The elliptical umbilics and hyperbolic umbilics have distinctly different focal surfaces. A ridge on the surface corresponds to a cuspidal edges so each sheet of the elliptical focal surface will have three cuspidal edges which come together at the umbilic focus and then switch to the other sheet. For a hyperbolic umbilic there is a single cuspidal edge which switch from one sheet to the other.
Definition in higher dimension in Riemannian manifolds
A point ''p'' in aRiemannian submanifold A Riemannian submanifold ''N'' of a Riemannian manifold ''M'' is a submanifold of ''M'' equipped with the Riemannian metric inherited from ''M''. The image of an isometric immersion
In mathematics, an embedding (or imbedding) is one instance of ...
is umbilical if, at ''p'', the (vector-valued) Second fundamental form
In differential geometry, the second fundamental form (or shape tensor) is a quadratic form on the tangent plane of a smooth surface in the three-dimensional Euclidean space, usually denoted by \mathrm (read "two"). Together with the first fundame ...
is some normal vector tensor the induced metric (First fundamental form
In differential geometry, the first fundamental form is the inner product on the tangent space of a surface in three-dimensional Euclidean space which is induced canonically from the dot product of . It permits the calculation of curvature and me ...
). Equivalently, for all vectors ''U'', ''V'' at ''p'', II(''U'', ''V'') = ''g''''p''(''U'', ''V''), where is the mean curvature vector at ''p''.
A submanifold is said to be umbilic (or all-umbilic) if this condition holds at every point "p". This is equivalent to saying that the submanifold can be made totally geodesic by an appropriate conformal change of the metric of the surrounding ("ambient") manifold. For example, a surface in Euclidean space is umbilic if and only if it is a piece of a sphere.
See also
* umbilical – an anatomical term meaning ''of, or relating to the navel''References
Volume I
, publisher=Gauthier-Villars
Differential geometry of surfaces Surfaces