Square root of two on:
[Wikipedia]
[Google]
[Amazon]
The square root of 2 (approximately 1.4142) is a positive
 The
The
 A simple proof is attributed by
A simple proof is attributed by  Another geometric
Another geometric
 One interesting property of is
:
since
:
This is related to the property of silver ratios.
can also be expressed in terms of copies of the
One interesting property of is
:
since
:
This is related to the property of silver ratios.
can also be expressed in terms of copies of the
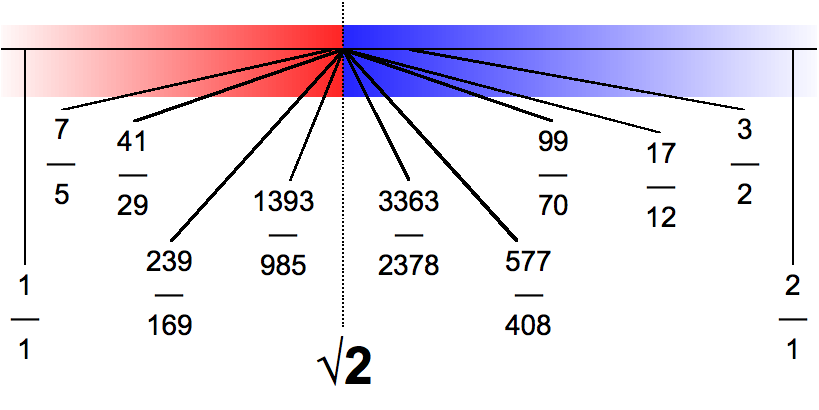 The square root of two has the following
The square root of two has the following
 In 1786, German physics professor Georg Christoph Lichtenberg found that any sheet of paper whose long edge is times longer than its short edge could be folded in half and aligned with its shorter side to produce a sheet with exactly the same proportions as the original. This ratio of lengths of the longer over the shorter side guarantees that cutting a sheet in half along a line results in the smaller sheets having the same (approximate) ratio as the original sheet. When Germany standardised
In 1786, German physics professor Georg Christoph Lichtenberg found that any sheet of paper whose long edge is times longer than its short edge could be folded in half and aligned with its shorter side to produce a sheet with exactly the same proportions as the original. This ratio of lengths of the longer over the shorter side guarantees that cutting a sheet in half along a line results in the smaller sheets having the same (approximate) ratio as the original sheet. When Germany standardised
Let shorter length and longer length of the sides of a sheet of paper, with
: as required by ISO 216. Let be the analogous ratio of the halved sheet, then
:.
The Square Root of Two to 5 million digits
by Jerry Bonnell and Robert J. Nemiroff. May, 1994.
Square root of 2 is irrational
a collection of proofs *
Search Engine
2 billion searchable digits of , and {{DEFAULTSORT:Square Root Of Two Quadratic irrational numbers Mathematical constants Pythagorean theorem Articles containing proofs
real number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every ...
that, when multiplied by itself, equals the number 2. It may be written in mathematics as or , and is an algebraic number
An algebraic number is a number that is a root of a non-zero polynomial in one variable with integer (or, equivalently, rational) coefficients. For example, the golden ratio, (1 + \sqrt)/2, is an algebraic number, because it is a root of th ...
. Technically, it should be called the principal square root
In mathematics, a square root of a number is a number such that ; in other words, a number whose '' square'' (the result of multiplying the number by itself, or ⋅ ) is . For example, 4 and −4 are square roots of 16, because .
...
of 2, to distinguish it from the negative number with the same property.
Geometrically, the square root of 2 is the length of a diagonal across a square with sides of one unit of length; this follows from the Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposit ...
. It was probably the first number known to be irrational
Irrationality is cognition, thinking, talking, or acting without inclusion of rationality. It is more specifically described as an action or opinion given through inadequate use of reason, or through emotional distress or cognitive deficiency. T ...
. The fraction (≈ 1.4142857) is sometimes used as a good rational approximation with a reasonably small denominator
A fraction (from la, fractus, "broken") represents a part of a whole or, more generally, any number of equal parts. When spoken in everyday English, a fraction describes how many parts of a certain size there are, for example, one-half, eight ...
.
Sequence in the On-Line Encyclopedia of Integer Sequences
The On-Line Encyclopedia of Integer Sequences (OEIS) is an online database of integer sequences. It was created and maintained by Neil Sloane while researching at AT&T Labs. He transferred the intellectual property and hosting of the OEIS to t ...
consists of the digits in the decimal expansion
A decimal representation of a non-negative real number is its expression as a sequence of symbols consisting of decimal digits traditionally written with a single separator:
r = b_k b_\ldots b_0.a_1a_2\ldots
Here is the decimal separator, ...
of the square root of 2, here truncated to 65 decimal places:
:
History
 The
The Babylonia
Babylonia (; Akkadian: , ''māt Akkadī'') was an ancient Akkadian-speaking state and cultural area based in the city of Babylon in central-southern Mesopotamia (present-day Iraq and parts of Syria). It emerged as an Amorite-ruled state c ...
n clay tablet YBC 7289
YBC 7289 is a Babylonian clay tablet notable for containing an accurate sexagesimal approximation to the square root of 2, the length of the diagonal of a unit square. This number is given to the equivalent of six decimal digits, "the greatest ...
(c. 1800–1600 BC) gives an approximation of in four sexagesimal
Sexagesimal, also known as base 60 or sexagenary, is a numeral system with sixty as its base. It originated with the ancient Sumerians in the 3rd millennium BC, was passed down to the ancient Babylonians, and is still used—in a modified form ...
figures, , which is accurate to about six decimal
The decimal numeral system (also called the base-ten positional numeral system and denary or decanary) is the standard system for denoting integer and non-integer numbers. It is the extension to non-integer numbers of the Hindu–Arabic numeral ...
digits, and is the closest possible three-place sexagesimal representation of :
:
Another early approximation is given in ancient Indian
The following outline is provided as an overview of and topical guide to ancient India:
Ancient India is the Indian subcontinent from prehistoric times to the start of Medieval India, which is typically dated (when the term is still used) to t ...
mathematical texts, the Sulbasutras (c. 800–200 BC), as follows: ''Increase the length f the side
F, or f, is the sixth letter in the Latin alphabet, used in the modern English alphabet, the alphabets of other western European languages and others worldwide. Its name in English is ''ef'' (pronounced ), and the plural is ''efs''.
His ...
by its third and this third by its own fourth less the thirty-fourth part of that fourth.'' That is,
:
This approximation is the seventh in a sequence of increasingly accurate approximations based on the sequence of Pell number
In mathematics, the Pell numbers are an infinite sequence of integers, known since ancient times, that comprise the denominators of the closest rational approximations to the square root of 2. This sequence of approximations begins , , , , an ...
s, which can be derived from the continued fraction
In mathematics, a continued fraction is an expression obtained through an iterative process of representing a number as the sum of its integer part and the reciprocal of another number, then writing this other number as the sum of its integer ...
expansion of . Despite having a smaller denominator, it is only slightly less accurate than the Babylonian approximation.
Pythagoreans
Pythagoreanism originated in the 6th century BC, based on and around the teachings and beliefs held by Pythagoras and his followers, the Pythagoreans. Pythagoras established the first Pythagorean community in the ancient Greek colony of Kroton, ...
discovered that the diagonal of a square
In Euclidean geometry, a square is a regular quadrilateral, which means that it has four equal sides and four equal angles (90- degree angles, π/2 radian angles, or right angles). It can also be defined as a rectangle with two equal-length a ...
is incommensurable with its side, or in modern language, that the square root of two is irrational
Irrationality is cognition, thinking, talking, or acting without inclusion of rationality. It is more specifically described as an action or opinion given through inadequate use of reason, or through emotional distress or cognitive deficiency. T ...
. Little is known with certainty about the time or circumstances of this discovery, but the name of Hippasus of Metapontum is often mentioned. For a while, the Pythagoreans treated as an official secret the discovery that the square root of two is irrational, and, according to legend, Hippasus was murdered for divulging it. The square root of two is occasionally called Pythagoras's number or Pythagoras's constant, for example by .
Ancient Roman architecture
Inancient Roman architecture
Ancient Roman architecture adopted the external language of classical Greek architecture for the purposes of the ancient Romans, but was different from Greek buildings, becoming a new architectural style. The two styles are often considered one ...
, Vitruvius
Vitruvius (; c. 80–70 BC – after c. 15 BC) was a Roman architect and engineer during the 1st century BC, known for his multi-volume work entitled '' De architectura''. He originated the idea that all buildings should have three attribut ...
describes the use of the square root of 2 progression or ''ad quadratum'' technique. It consists basically in a geometric, rather than arithmetic, method to double a square, in which the diagonal of the original square is equal to the side of the resulting square. Vitruvius attributes the idea to Plato
Plato ( ; grc-gre, Πλάτων ; 428/427 or 424/423 – 348/347 BC) was a Greek philosopher born in Athens during the Classical period in Ancient Greece. He founded the Platonist school of thought and the Academy, the first institution ...
. The system was employed to build pavements by creating a square tangent
In geometry, the tangent line (or simply tangent) to a plane curve at a given point is the straight line that "just touches" the curve at that point. Leibniz defined it as the line through a pair of infinitely close points on the curve. Mo ...
to the corners of the original square at 45 degrees of it. The proportion was also used to design atria by giving them a length equal to a diagonal taken from a square, whose sides are equivalent to the intended atrium's width.
Decimal value
Computation algorithms
There are a number ofalgorithm
In mathematics and computer science, an algorithm () is a finite sequence of rigorous instructions, typically used to solve a class of specific problems or to perform a computation. Algorithms are used as specifications for performing ...
s for approximating as a ratio of integer
An integer is the number zero (), a positive natural number (, , , etc.) or a negative integer with a minus sign ( −1, −2, −3, etc.). The negative numbers are the additive inverses of the corresponding positive numbers. In the languag ...
s or as a decimal. The most common algorithm for this, which is used as a basis in many computers and calculators, is the Babylonian method for computing square roots. It goes as follows:
First, pick a guess, ; the value of the guess affects only how many iterations are required to reach an approximation of a certain accuracy. Then, using that guess, iterate through the following recursive computation:
:
The more iterations through the algorithm (that is, the more computations performed and the greater ""), the better the approximation. Each iteration roughly doubles the number of correct digits. Starting with , the results of the algorithm are as follows:
* 1 ()
* = 1.5 ()
* = 1.416... ()
* = 1.414215... ()
* = 1.4142135623746... ()
Rational approximations
A simple rational approximation (≈ 1.4142857) is sometimes used. Despite having a denominator of only 70, it differs from the correct value by less than (approx. ). The next two better rational approximations are (≈ 1.4141414...) with a marginally smaller error (approx. ), and (≈ 1.4142012) with an error of approx . The rational approximation of the square root of two derived from four iterations of the Babylonian method after starting with () is too large by about ; its square is ≈ .Records in computation
In 1997 the value of was calculated to 137,438,953,444 decimal places by Yasumasa Kanada's team. In February 2006 the record for the calculation of was eclipsed with the use of a home computer. Shigeru Kondo calculated 1trillion
''Trillion'' is a number with two distinct definitions:
*1,000,000,000,000, i.e. one million million, or (ten to the twelfth power), as defined on the short scale. This is now the meaning in both American and British English.
* 1,000,000,000,00 ...
decimal places in 2010. Among mathematical constant
A mathematical constant is a key number whose value is fixed by an unambiguous definition, often referred to by a symbol (e.g., an alphabet letter), or by mathematicians' names to facilitate using it across multiple mathematical problems. Cons ...
s with computationally challenging decimal expansions, only , , and the golden ratio
In mathematics, two quantities are in the golden ratio if their ratio is the same as the ratio of their sum to the larger of the two quantities. Expressed algebraically, for quantities a and b with a > b > 0,
where the Greek letter phi ( ...
have been calculated more precisely as of March 2022. Such computations aim to check empirically whether such numbers are normal.
This is a table of recent records in calculating the digits of .
Proofs of irrationality
A shortproof
Proof most often refers to:
* Proof (truth), argument or sufficient evidence for the truth of a proposition
* Alcohol proof, a measure of an alcoholic drink's strength
Proof may also refer to:
Mathematics and formal logic
* Formal proof, a c ...
of the irrationality of can be obtained from the rational root theorem
In algebra, the rational root theorem (or rational root test, rational zero theorem, rational zero test or theorem) states a constraint on rational solutions of a polynomial equation
:a_nx^n+a_x^+\cdots+a_0 = 0
with integer coefficients a_i\in ...
, that is, if is a monic polynomial
In mathematics, a polynomial is an expression consisting of indeterminates (also called variables) and coefficients, that involves only the operations of addition, subtraction, multiplication, and positive-integer powers of variables. An exampl ...
with integer coefficient
In mathematics, a coefficient is a multiplicative factor in some term of a polynomial, a series, or an expression; it is usually a number, but may be any expression (including variables such as , and ). When the coefficients are themselves ...
s, then any rational
Rationality is the quality of being guided by or based on reasons. In this regard, a person acts rationally if they have a good reason for what they do or a belief is rational if it is based on strong evidence. This quality can apply to an abi ...
root
In vascular plants, the roots are the organs of a plant that are modified to provide anchorage for the plant and take in water and nutrients into the plant body, which allows plants to grow taller and faster. They are most often below the su ...
of is necessarily an integer. Applying this to the polynomial , it follows that is either an integer or irrational. Because is not an integer (2 is not a perfect square), must therefore be irrational. This proof can be generalized to show that any square root of any natural number
In mathematics, the natural numbers are those numbers used for counting (as in "there are ''six'' coins on the table") and ordering (as in "this is the ''third'' largest city in the country").
Numbers used for counting are called '' cardinal ...
that is not a perfect square is irrational.
For other proofs that the square root of any non-square natural number is irrational, see Quadratic irrational number or Infinite descent In mathematics, a proof by infinite descent, also known as Fermat's method of descent, is a particular kind of proof by contradiction used to show that a statement cannot possibly hold for any number, by showing that if the statement were to hold f ...
.
Proof by infinite descent
One proof of the number's irrationality is the following proof by infinite descent. It is also aproof by contradiction
In logic and mathematics, proof by contradiction is a form of proof that establishes the truth or the validity of a proposition, by showing that assuming the proposition to be false leads to a contradiction. Proof by contradiction is also known ...
, also known as an indirect proof, in that the proposition is proved by assuming that the opposite of the proposition is true and showing that this assumption is false, thereby implying that the proposition must be true.
# Assume that is a rational number, meaning that there exists a pair of integers whose ratio is exactly .
# If the two integers have a common factor, it can be eliminated using the Euclidean algorithm
In mathematics, the Euclidean algorithm,Some widely used textbooks, such as I. N. Herstein's ''Topics in Algebra'' and Serge Lang's ''Algebra'', use the term "Euclidean algorithm" to refer to Euclidean division or Euclid's algorithm, is an e ...
.
# Then can be written as an irreducible fraction such that and are coprime integers (having no common factor) which additionally means that at least one of or must be odd
Odd means unpaired, occasional, strange or unusual, or a person who is viewed as eccentric.
Odd may also refer to:
Acronym
* ODD (Text Encoding Initiative) ("One Document Does it all"), an abstracted literate-programming format for describing X ...
.
# It follows that and . ( ) ( are integers)
# Therefore, is even
Even may refer to:
General
* Even (given name), a Norwegian male personal name
* Even (surname)
* Even (people), an ethnic group from Siberia and Russian Far East
**Even language, a language spoken by the Evens
* Odd and Even, a solitaire game wh ...
because it is equal to . ( is necessarily even because it is 2 times another whole number.)
# It follows that must be even (as squares of odd integers are never even).
# Because is even, there exists an integer that fulfills .
# Substituting from step 7 for in the second equation of step 4: , which is equivalent to .
# Because is divisible by two and therefore even, and because , it follows that is also even which means that is even.
# By steps 5 and 8 and are both even, which contradicts that is irreducible as stated in step 3.
::''Q.E.D.
Q.E.D. or QED is an initialism of the Latin phrase , meaning "which was to be demonstrated". Literally it states "what was to be shown". Traditionally, the abbreviation is placed at the end of mathematical proofs and philosophical arguments in pri ...
''
Because there is a contradiction, the assumption (1) that is a rational number must be false. This means that is not a rational number. That is, is irrational.
This proof was hinted at by Aristotle
Aristotle (; grc-gre, Ἀριστοτέλης ''Aristotélēs'', ; 384–322 BC) was a Greek philosopher and polymath during the Classical period in Ancient Greece. Taught by Plato, he was the founder of the Peripatetic school of ...
, in his '' Analytica Priora'', §I.23. It appeared first as a full proof in Euclid
Euclid (; grc-gre, Εὐκλείδης; BC) was an ancient Greek mathematician active as a geometer and logician. Considered the "father of geometry", he is chiefly known for the '' Elements'' treatise, which established the foundations of ...
's '' Elements'', as proposition 117 of Book X. However, since the early 19th century, historians have agreed that this proof is an interpolation
In the mathematical field of numerical analysis, interpolation is a type of estimation, a method of constructing (finding) new data points based on the range of a discrete set of known data points.
In engineering and science, one often has ...
and not attributable to Euclid.
Proof by unique factorization
As with the proof by infinite descent, we obtain . Being the same quantity, each side has the sameprime factorization
In number theory, integer factorization is the decomposition of a composite number into a product of smaller integers. If these factors are further restricted to prime numbers, the process is called prime factorization.
When the numbers are s ...
by the fundamental theorem of arithmetic, and in particular, would have to have the factor 2 occur the same number of times. However, the factor 2 appears an odd number of times on the right, but an even number of times on the left—a contradiction.
Geometric proof
John Horton Conway
John Horton Conway (26 December 1937 – 11 April 2020) was an English mathematician active in the theory of finite groups, knot theory, number theory, combinatorial game theory and coding theory. He also made contributions to many branches ...
to Stanley Tennenbaum when the latter was a student in the early 1950s and whose most recent appearance is in an article by Noson Yanofsky in the May–June 2016 issue of ''American Scientist
__NOTOC__
''American Scientist'' (informally abbreviated ''AmSci'') is an American bimonthly science and technology magazine published since 1913 by Sigma Xi, The Scientific Research Society. In the beginning of 2000s the headquarters was in ...
''. Given two squares with integer sides respectively ''a'' and ''b'', one of which has twice the area
Area is the quantity that expresses the extent of a region on the plane or on a curved surface. The area of a plane region or ''plane area'' refers to the area of a shape or planar lamina, while '' surface area'' refers to the area of an op ...
of the other, place two copies of the smaller square in the larger as shown in Figure 1. The square overlap region in the middle () must equal the sum of the two uncovered squares (). However, these squares on the diagonal have positive integer sides that are smaller than the original squares. Repeating this process, there are arbitrarily small squares one twice the area of the other, yet both having positive integer sides, which is impossible since positive integers cannot be less than 1.
reductio ad absurdum
In logic, (Latin for "reduction to absurdity"), also known as (Latin for "argument to absurdity") or ''apagogical arguments'', is the form of argument that attempts to establish a claim by showing that the opposite scenario would lead to absu ...
argument showing that is irrational appeared in 2000 in the American Mathematical Monthly
''The American Mathematical Monthly'' is a mathematical journal founded by Benjamin Finkel in 1894. It is published ten times each year by Taylor & Francis for the Mathematical Association of America.
The ''American Mathematical Monthly'' is an ...
. It is also an example of proof by infinite descent. It makes use of classic compass and straightedge
In geometry, straightedge-and-compass construction – also known as ruler-and-compass construction, Euclidean construction, or classical construction – is the construction of lengths, angles, and other geometric figures using only an ideali ...
construction, proving the theorem by a method similar to that employed by ancient Greek geometers. It is essentially the same algebraic proof as in the previous paragraph, viewed geometrically in another way.
Let be a right isosceles triangle with hypotenuse length and legs as shown in Figure 2. By the Pythagorean theorem
In mathematics, the Pythagorean theorem or Pythagoras' theorem is a fundamental relation in Euclidean geometry between the three sides of a right triangle. It states that the area of the square whose side is the hypotenuse (the side opposit ...
, . Suppose and are integers. Let be a ratio
In mathematics, a ratio shows how many times one number contains another. For example, if there are eight oranges and six lemons in a bowl of fruit, then the ratio of oranges to lemons is eight to six (that is, 8:6, which is equivalent to the ...
given in its lowest terms.
Draw the arcs and with centre . Join . It follows that , and and coincide. Therefore, the triangle
A triangle is a polygon with three edges and three vertices. It is one of the basic shapes in geometry. A triangle with vertices ''A'', ''B'', and ''C'' is denoted \triangle ABC.
In Euclidean geometry, any three points, when non- colline ...
s and are congruent by SAS.
Because is a right angle and is half a right angle, is also a right isosceles triangle. Hence implies . By symmetry, , and is also a right isosceles triangle. It also follows that .
Hence, there is an even smaller right isosceles triangle, with hypotenuse length and legs . These values are integers even smaller than and and in the same ratio, contradicting the hypothesis that is in lowest terms. Therefore, and cannot be both integers, hence is irrational.
Constructive proof
In a constructive approach, one distinguishes between on the one hand not being rational, and on the other hand being irrational (i.e., being quantifiably apart from every rational), the latter being a stronger property. Let and be positive integers such that (as satisfies these bounds). Now and cannot be equal, since the first has an odd number of factors 2 whereas the second has an even number of factors 2. Thus . Multiplying the absolute difference by in the numerator and denominator, we get : the latter inequality being true because it is assumed that , giving (otherwise the quantitative apartness can be trivially established). This gives a lower bound of for the difference , yielding a direct proof of irrationality not relying on thelaw of excluded middle
In logic, the law of excluded middle (or the principle of excluded middle) states that for every proposition, either this proposition or its negation is true. It is one of the so-called three laws of thought, along with the law of noncontradi ...
; see Errett Bishop (1985, p. 18). This proof constructively exhibits a discrepancy between and any rational.
Proof by Pythagorean triples
This proof uses the following property of primitivePythagorean triple
A Pythagorean triple consists of three positive integers , , and , such that . Such a triple is commonly written , and a well-known example is . If is a Pythagorean triple, then so is for any positive integer . A primitive Pythagorean triple is ...
s:
: If , , and are coprime positive integers such that , then is never even.
This lemma can be used to show that two identical perfect squares can never be added to produce another perfect square.
Suppose the contrary that is rational. Therefore,
:
:where and
:Squaring both sides,
:
:
:
Here, is a primitive Pythagorean triple, and from the lemma is never even. However, this contradicts the equation which implies that must be even.
Multiplicative inverse
Themultiplicative inverse
In mathematics, a multiplicative inverse or reciprocal for a number ''x'', denoted by 1/''x'' or ''x''−1, is a number which when multiplied by ''x'' yields the multiplicative identity, 1. The multiplicative inverse of a fraction ''a''/' ...
(reciprocal) of the square root of two (i.e., the square root of ) is a widely used constant.
: ...
One-half of , also the reciprocal of , is a common quantity in geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
and trigonometry
Trigonometry () is a branch of mathematics that studies relationships between side lengths and angles of triangles. The field emerged in the Hellenistic world during the 3rd century BC from applications of geometry to astronomical studies. ...
because the unit vector
In mathematics, a unit vector in a normed vector space is a vector (often a spatial vector) of length 1. A unit vector is often denoted by a lowercase letter with a circumflex, or "hat", as in \hat (pronounced "v-hat").
The term ''direction v ...
that makes a 45° angle
In Euclidean geometry, an angle is the figure formed by two rays, called the '' sides'' of the angle, sharing a common endpoint, called the ''vertex'' of the angle.
Angles formed by two rays lie in the plane that contains the rays. Angles ...
with the axes in a plane has the coordinates
:
This number satisfies
:
Properties
imaginary unit
The imaginary unit or unit imaginary number () is a solution to the quadratic equation x^2+1=0. Although there is no real number with this property, can be used to extend the real numbers to what are called complex numbers, using addition an ...
using only the square root
In mathematics, a square root of a number is a number such that ; in other words, a number whose '' square'' (the result of multiplying the number by itself, or ⋅ ) is . For example, 4 and −4 are square roots of 16, because .
...
and arithmetic operations
Arithmetic () is an elementary part of mathematics that consists of the study of the properties of the traditional operations on numbers—addition, subtraction, multiplication, division, exponentiation, and extraction of roots. In the 19th ce ...
, if the square root symbol is interpreted suitably for the complex number
In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted , called the imaginary unit and satisfying the equation i^= -1; every complex number can be expressed in the fo ...
s and :
:
is also the only real number other than 1 whose infinite tetrate (i.e., infinite exponential tower) is equal to its square. In other words: if for , and for , the limit
Limit or Limits may refer to:
Arts and media
* ''Limit'' (manga), a manga by Keiko Suenobu
* ''Limit'' (film), a South Korean film
* Limit (music), a way to characterize harmony
* "Limit" (song), a 2016 single by Luna Sea
* "Limits", a 2019 ...
of as will be called (if this limit exists) . Then is the only number for which . Or symbolically:
:
appears in Viète's formula for :
:
for square roots and only one minus sign.
Similar in appearance but with a finite number of terms, appears in various trigonometric constants:
:
It is not known whether is a normal number, which is a stronger property than irrationality, but statistical analyses of its binary expansion are consistent with the hypothesis that it is normal to base two
A binary number is a number expressed in the base-2 numeral system or binary numeral system, a method of mathematical expression which uses only two symbols: typically "0" (zero) and "1" (one).
The base-2 numeral system is a positional notatio ...
.
Representations
Series and product
The identity , along with the infinite product representations for the sine and cosine, leads to products such as : and : or equivalently, : The number can also be expressed by taking theTaylor series
In mathematics, the Taylor series or Taylor expansion of a function is an infinite sum of terms that are expressed in terms of the function's derivatives at a single point. For most common functions, the function and the sum of its Taylor ser ...
of a trigonometric function
In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a right-angled triangle to ratios of two side lengths. They are widely used in a ...
. For example, the series for gives
:
The Taylor series of with and using the double factorial
In mathematics, the double factorial or semifactorial of a number , denoted by , is the product of all the integers from 1 up to that have the same parity (odd or even) as . That is,
:n!! = \prod_^ (n-2k) = n (n-2) (n-4) \cdots.
For even , the ...
gives
:
The convergence of this series can be accelerated with an Euler transform, producing
:
It is not known whether can be represented with a BBP-type formula. BBP-type formulas are known for and , however.
The number can be represented by an infinite series of Egyptian fractions, with denominators defined by 2''n'' th terms of a Fibonacci
Fibonacci (; also , ; – ), also known as Leonardo Bonacci, Leonardo of Pisa, or Leonardo Bigollo Pisano ('Leonardo the Traveller from Pisa'), was an Italian mathematician from the Republic of Pisa, considered to be "the most talented Wester ...
-like recurrence relation
In mathematics, a recurrence relation is an equation according to which the nth term of a sequence of numbers is equal to some combination of the previous terms. Often, only k previous terms of the sequence appear in the equation, for a parameter ...
''a''(''n'') = 34''a''(''n''−1) − ''a''(''n''−2), ''a''(0) = 0, ''a''(1) = 6.
:
Continued fraction
 The square root of two has the following
The square root of two has the following continued fraction
In mathematics, a continued fraction is an expression obtained through an iterative process of representing a number as the sum of its integer part and the reciprocal of another number, then writing this other number as the sum of its integer ...
representation:
:
The convergents formed by truncating this representation form a sequence of fractions that approximate the square root of two to increasing accuracy, and that are described by the Pell number
In mathematics, the Pell numbers are an infinite sequence of integers, known since ancient times, that comprise the denominators of the closest rational approximations to the square root of 2. This sequence of approximations begins , , , , an ...
s (i.e., ). The first convergents are: and the convergent following is . The convergent differs from by almost exactly , which follows from:
:
Nested square
The following nested square expressions converge to : :Applications
Paper size
paper size
Paper size standards govern the size of sheets of paper used as writing paper, stationery, cards, and for some printed documents.
The ISO 216 standard, which includes the commonly used A4 size, is the international standard for paper size. I ...
s at the beginning of the 20th century, they used Lichtenberg's ratio to create the "A" series of paper sizes. Today, the (approximate) aspect ratio of paper sizes under ISO 216
ISO 216 is an international standard for paper sizes, used around the world except in North America and parts of Latin America. The standard defines the "A", "B" and "C" series of paper sizes, including A4, the most commonly available paper si ...
(A4, A0, etc.) is 1:.
Proof:Let shorter length and longer length of the sides of a sheet of paper, with
: as required by ISO 216. Let be the analogous ratio of the halved sheet, then
:.
Physical sciences
There are some interesting properties involving the square root of 2 in thephysical sciences
Physical science is a branch of natural science that studies non-living systems, in contrast to life science. It in turn has many branches, each referred to as a "physical science", together called the "physical sciences".
Definition
Phy ...
:
* The square root of two is the frequency ratio of a tritone
In music theory, the tritone is defined as a musical interval composed of three adjacent whole tones (six semitones). For instance, the interval from F up to the B above it (in short, F–B) is a tritone as it can be decomposed into the three adj ...
interval in twelve-tone equal temperament
An equal temperament is a musical temperament or tuning system, which approximates just intervals by dividing an octave (or other interval) into equal steps. This means the ratio of the frequencies of any adjacent pair of notes is the same, ...
music.
* The square root of two forms the relationship of f-stops in photographic lenses, which in turn means that the ratio of ''areas'' between two successive aperture
In optics, an aperture is a hole or an opening through which light travels. More specifically, the aperture and focal length of an optical system determine the cone angle of a bundle of rays that come to a focus in the image plane.
An ...
s is 2.
* The celestial latitude (declination) of the Sun during a planet's astronomical cross-quarter day
The Wheel of the Year is an annual cycle of seasonal festivals, observed by many modern pagans, consisting of the year's chief solar events (solstices and equinoxes) and the midpoints between them. While names for each festival vary among di ...
points equals the tilt of the planet's axis divided by .
See also
* List of mathematical constants *Square root of 3
The square root of 3 is the positive real number that, when multiplied by itself, gives the number 3. It is denoted mathematically as \sqrt or 3^. It is more precisely called the principal square root of 3 to distinguish it from the negative nu ...
,
* Square root of 5,
*Gelfond–Schneider constant
The Gelfond–Schneider constant or Hilbert number is two to the power of the square root of two:
:2 = ...
which was proved to be a transcendental number by Rodion Kuzmin in 1930.
In 1934, Aleksandr Gelfond and Theodor Schneider independently pr ...
,
* Silver ratio,
Notes
References
* . * * Bishop, Errett (1985), Schizophrenia in contemporary mathematics. Errett Bishop: reflections on him and his research (San Diego, Calif., 1983), 1–32, Contemp. Math. 39, Amer. Math. Soc., Providence, RI. * . * . * . * .External links
* .The Square Root of Two to 5 million digits
by Jerry Bonnell and Robert J. Nemiroff. May, 1994.
Square root of 2 is irrational
a collection of proofs *
Search Engine
2 billion searchable digits of , and {{DEFAULTSORT:Square Root Of Two Quadratic irrational numbers Mathematical constants Pythagorean theorem Articles containing proofs