Knaster–Kuratowski–Mazurkiewicz lemma on:
[Wikipedia]
[Google]
[Amazon]
The Knaster–Kuratowski–Mazurkiewicz lemma is a basic result in mathematical
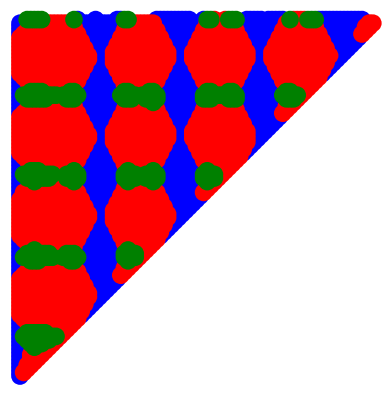
 The lemma is illustrated by the picture on the right, in which set #1 is blue, set #2 is red and set #3 is green. The KKM requirements are satisfied, since:
* Each vertex is covered by a unique color.
* Each edge is covered by the two colors of its two vertices.
* The triangle is covered by all three colors.
The KKM lemma states that there is a point covered by all three colors simultaneously; such a point is clearly visible in the picture.
Note that it is important that all sets are closed, i.e., contain their boundary. If, for example, the red set is not closed, then it is possible that the central point is contained only in the blue and green sets, and then the intersection of all three sets may be empty.
The lemma is illustrated by the picture on the right, in which set #1 is blue, set #2 is red and set #3 is green. The KKM requirements are satisfied, since:
* Each vertex is covered by a unique color.
* Each edge is covered by the two colors of its two vertices.
* The triangle is covered by all three colors.
The KKM lemma states that there is a point covered by all three colors simultaneously; such a point is clearly visible in the picture.
Note that it is important that all sets are closed, i.e., contain their boundary. If, for example, the red set is not closed, then it is possible that the central point is contained only in the blue and green sets, and then the intersection of all three sets may be empty.
 A connector of a simplex is a
A connector of a simplex is a
fixed-point theory Fixed point may refer to:
* Fixed point (mathematics), a value that does not change under a given transformation
* Fixed-point arithmetic, a manner of doing arithmetic on computers
* Fixed point, a benchmark (surveying) used by geodesists
* Fixed p ...
published in 1929 by Knaster, Kuratowski
Kazimierz Kuratowski (; 2 February 1896 – 18 June 1980) was a Polish mathematician and logician. He was one of the leading representatives of the Warsaw School of Mathematics.
Biography and studies
Kazimierz Kuratowski was born in Warsaw, ( ...
and Mazurkiewicz.
The KKM lemma can be proved from Sperner's lemma and can be used to prove the Brouwer fixed-point theorem
Brouwer's fixed-point theorem is a fixed-point theorem in topology, named after L. E. J. (Bertus) Brouwer. It states that for any continuous function f mapping a compact convex set to itself there is a point x_0 such that f(x_0)=x_0. The simples ...
.
Statement
Let be an -dimensional simplex with ''n'' vertices labeled as . A KKM covering is defined as a set of closed sets such that for any , the convex hull of the vertices corresponding to is covered by . The KKM lemma says that in every KKM covering, the common intersection of all ''n'' sets is nonempty, i.e: :Example
When , the KKM lemma considers the simplex which is a triangle, whose vertices can be labeled 1, 2 and 3. We are given three closed sets such that: * covers vertex 1, covers vertex 2, covers vertex 3. * The edge 12 (from vertex 1 to vertex 2) is covered by the sets and , the edge 23 is covered by the sets and , the edge 31 is covered by the sets and . * The union of all three sets covers the entire triangle The KKM lemma states that the sets have at least one point in common. The lemma is illustrated by the picture on the right, in which set #1 is blue, set #2 is red and set #3 is green. The KKM requirements are satisfied, since:
* Each vertex is covered by a unique color.
* Each edge is covered by the two colors of its two vertices.
* The triangle is covered by all three colors.
The KKM lemma states that there is a point covered by all three colors simultaneously; such a point is clearly visible in the picture.
Note that it is important that all sets are closed, i.e., contain their boundary. If, for example, the red set is not closed, then it is possible that the central point is contained only in the blue and green sets, and then the intersection of all three sets may be empty.
The lemma is illustrated by the picture on the right, in which set #1 is blue, set #2 is red and set #3 is green. The KKM requirements are satisfied, since:
* Each vertex is covered by a unique color.
* Each edge is covered by the two colors of its two vertices.
* The triangle is covered by all three colors.
The KKM lemma states that there is a point covered by all three colors simultaneously; such a point is clearly visible in the picture.
Note that it is important that all sets are closed, i.e., contain their boundary. If, for example, the red set is not closed, then it is possible that the central point is contained only in the blue and green sets, and then the intersection of all three sets may be empty.
Equivalent results
Generalizations
Rainbow KKM lemma (Gale)
David Gale
David (; , "beloved one") (traditional spelling), , ''Dāwūd''; grc-koi, Δαυΐδ, Dauíd; la, Davidus, David; gez , ዳዊት, ''Dawit''; xcl, Դաւիթ, ''Dawitʿ''; cu, Давíдъ, ''Davidŭ''; possibly meaning "beloved one". w ...
proved the following generalization of the KKM lemma. Suppose that, instead of one KKM covering, we have ''n'' different KKM coverings: . Then, there exists a permutation of the coverings with a non-empty intersection, i.e:
:.
The name "rainbow KKM lemma" is inspired by Gale's description of his lemma:"A colloquial statement of this result is... if each of three people paint a triangle red, white and blue according to the KKM rules, then there will be a point which is in the red set of one person, the white set of another, the blue of the third".The rainbow KKM lemma can be proved using a rainbow generalization of Sperner's lemma. The original KKM lemma follows from the rainbow KKM lemma by simply picking ''n'' identical coverings.
Connector-free lemma (Bapat)
 A connector of a simplex is a
A connector of a simplex is a connected set
In topology and related branches of mathematics, a connected space is a topological space that cannot be represented as the union of two or more disjoint non-empty open subsets. Connectedness is one of the principal topological properties th ...
that touches all ''n'' faces of the simplex.
A connector-free covering is a covering in which no contains a connector.
Any KKM covering is a connector-free covering, since in a KKM covering, no even touches all ''n'' faces. However, there are connector-free coverings that are not KKM coverings. An example is illustrated at the right. There, the red set touches all three faces, but it does not contain any connector, since no connected component of it touches all three faces.
A theorem of Ravindra Bapat
Ravindra B. Bapat is an Indian mathematician known for the Bapat–Beg theorem.
Education
He obtained B.Sc. from University of Mumbai, M.Stat. from the Indian Statistical Institute, New Delhi and Ph.D. from the University of Illinois at Chica ...
, generalizing Sperner's lemma, implies the KKM lemma extends to connector-free coverings (he proved his theorem for ).
The connector-free variant also has a permutation variant, so that both these generalizations can be used simultaneously.
KKMS theorem
The KKMS theorem is a generalization of the KKM lemma byLloyd Shapley
Lloyd Stowell Shapley (; June 2, 1923 – March 12, 2016) was an American mathematician and Nobel Prize-winning economist. He contributed to the fields of mathematical economics and especially game theory. Shapley is generally considered one of ...
. It is useful in economics
Economics () is the social science that studies the production, distribution, and consumption of goods and services.
Economics focuses on the behaviour and interactions of economic agents and how economies work. Microeconomics analyzes ...
, especially in cooperative game theory
In game theory, a cooperative game (or coalitional game) is a game with competition between groups of Player (game), players ("coalitions") due to the possibility of external enforcement of cooperative behavior (e.g. through contract law). Those ...
.
While a KKM covering contains ''n'' closed sets, a KKMS covering contains closed sets - indexed by the nonempty subsets of