|
First-countable Space
In topology, a branch of mathematics, a first-countable space is a topological space satisfying the "first axiom of countability". Specifically, a space X is said to be first-countable if each point has a countable neighbourhood basis (local base). That is, for each point x in X there exists a sequence N_1, N_2, \ldots of neighbourhoods of x such that for any neighbourhood N of x there exists an integer i with N_i contained in N. Since every neighborhood of any point contains an open neighborhood of that point, the neighbourhood basis can be chosen without loss of generality to consist of open neighborhoods. Examples and counterexamples The majority of 'everyday' spaces in mathematics are first-countable. In particular, every metric space is first-countable. To see this, note that the set of open balls centered at x with radius 1/n for integers form a countable local base at x. An example of a space that is not first-countable is the cofinite topology on an uncountable s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Topology
Topology (from the Greek language, Greek words , and ) is the branch of mathematics concerned with the properties of a Mathematical object, geometric object that are preserved under Continuous function, continuous Deformation theory, deformations, such as Stretch factor, stretching, Torsion (mechanics), twisting, crumpling, and bending; that is, without closing holes, opening holes, tearing, gluing, or passing through itself. A topological space is a Set (mathematics), set endowed with a structure, called a ''Topology (structure), topology'', which allows defining continuous deformation of subspaces, and, more generally, all kinds of List of continuity-related mathematical topics, continuity. Euclidean spaces, and, more generally, metric spaces are examples of topological spaces, as any distance or metric defines a topology. The deformations that are considered in topology are homeomorphisms and Homotopy, homotopies. A property that is invariant under such deformations is a to ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
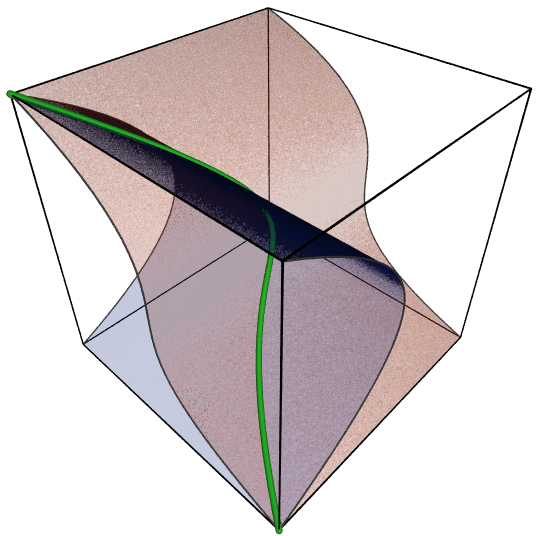
Algebraic Variety
Algebraic varieties are the central objects of study in algebraic geometry, a sub-field of mathematics. Classically, an algebraic variety is defined as the solution set, set of solutions of a system of polynomial equations over the real number, real or complex numbers. Modern definitions generalize this concept in several different ways, while attempting to preserve the geometric intuition behind the original definition. Conventions regarding the definition of an algebraic variety differ slightly. For example, some definitions require an algebraic variety to be Irreducible component, irreducible, which means that it is not the Union (set theory), union of two smaller Set (mathematics), sets that are Closed set, closed in the Zariski topology. Under this definition, non-irreducible algebraic varieties are called algebraic sets. Other conventions do not require irreducibility. The fundamental theorem of algebra establishes a link between algebra and geometry by showing that a mon ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Limit Of A Function
Although the function is not defined at zero, as becomes closer and closer to zero, becomes arbitrarily close to 1. In other words, the limit of as approaches zero, equals 1. In mathematics, the limit of a function is a fundamental concept in calculus and analysis concerning the behavior of that function near a particular input which may or may not be in the domain of the function. Formal definitions, first devised in the early 19th century, are given below. Informally, a function assigns an output to every input . We say that the function has a limit at an input , if gets closer and closer to as moves closer and closer to . More specifically, the output value can be made ''arbitrarily'' close to if the input to is taken ''sufficiently'' close to . On the other hand, if some inputs very close to are taken to outputs that stay a fixed distance apart, then we say the limit ''does not exist''. The notion of a limit has many applications in modern calc ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Sequential Space
In topology and related fields of mathematics, a sequential space is a topological space whose topology can be completely characterized by its convergent/divergent sequences. They can be thought of as spaces that satisfy a very weak axiom of countability, and all first-countable spaces (notably metric spaces) are sequential. In any topological space (X, \tau), if a convergent sequence is contained in a closed set C, then the limit of that sequence must be contained in C as well. Sets with this property are known as sequentially closed. Sequential spaces are precisely those topological spaces for which sequentially closed sets are in fact closed. (These definitions can also be rephrased in terms of sequentially open sets; see below.) Said differently, any topology can be described in terms of nets (also known as Moore–Smith sequences), but those sequences may be "too long" (indexed by too large an ordinal) to compress into a sequence. Sequential spaces are those topological spa ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Limit Of A Sequence
As the positive integer n becomes larger and larger, the value n\times \sin\left(\tfrac1\right) becomes arbitrarily close to 1. We say that "the limit of the sequence n \times \sin\left(\tfrac1\right) equals 1." In mathematics, the limit of a sequence is the value that the terms of a sequence "tend to", and is often denoted using the \lim symbol (e.g., \lim_a_n).Courant (1961), p. 29. If such a limit exists and is finite, the sequence is called convergent. A sequence that does not converge is said to be divergent. The limit of a sequence is said to be the fundamental notion on which the whole of mathematical analysis ultimately rests. Limits can be defined in any metric space, metric or topological space, but are usually first encountered in the real numbers. History The Greek philosopher Zeno of Elea is famous for formulating Zeno's paradoxes, paradoxes that involve limiting processes. Leucippus, Democritus, Antiphon (person), Antiphon, Eudoxus of Cnidus, Eudoxus, a ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Closure (topology)
In topology, the closure of a subset of points in a topological space consists of all points in together with all limit points of . The closure of may equivalently be defined as the union of and its boundary, and also as the intersection of all closed sets containing . Intuitively, the closure can be thought of as all the points that are either in or "very near" . A point which is in the closure of is a point of closure of . The notion of closure is in many ways dual to the notion of interior. Definitions Point of closure For S as a subset of a Euclidean space, x is a point of closure of S if every open ball centered at x contains a point of S (this point can be x itself). This definition generalizes to any subset S of a metric space X. Fully expressed, for X as a metric space with metric d, x is a point of closure of S if for every r > 0 there exists some s \in S such that the distance d(x, s) < r ( is allowed). Another way to expre ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Discrete Space
In topology, a discrete space is a particularly simple example of a topological space or similar structure, one in which the points form a , meaning they are '' isolated'' from each other in a certain sense. The discrete topology is the finest topology that can be given on a set. Every subset is open in the discrete topology so that in particular, every singleton subset is an open set in the discrete topology. Definitions Given a set X: A metric space (E,d) is said to be '' uniformly discrete'' if there exists a ' r > 0 such that, for any x,y \in E, one has either x = y or d(x,y) > r. The topology underlying a metric space can be discrete, without the metric being uniformly discrete: for example the usual metric on the set \left\. Properties The underlying uniformity on a discrete metric space is the discrete uniformity, and the underlying topology on a discrete uniform space is the discrete topology. Thus, the different notions of discrete space are compatible with on ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |
Second-countable Space
In topology, a second-countable space, also called a completely separable space, is a topological space whose topology has a countable base. More explicitly, a topological space T is second-countable if there exists some countable collection \mathcal = \_^ of open subsets of T such that any open subset of T can be written as a union of elements of some subfamily of \mathcal. A second-countable space is said to satisfy the second axiom of countability. Like other countability axioms, the property of being second-countable restricts the number of open subsets that a space can have. Many "well-behaved" spaces in mathematics are second-countable. For example, Euclidean space (R''n'') with its usual topology is second-countable. Although the usual base of open balls is uncountable, one can restrict this to the collection of all open balls with rational radii and whose centers have rational coordinates. This restricted collection is countable and still forms a basis. Properties ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] [Amazon] |