|
Torus
In geometry, a torus (plural tori, colloquially donut or doughnut) is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle. If the axis of revolution does not touch the circle, the surface has a ring shape and is called a torus of revolution. If the axis of revolution is tangent to the circle, the surface is a horn torus. If the axis of revolution passes twice through the circle, the surface is a spindle torus. If the axis of revolution passes through the center of the circle, the surface is a degenerate torus, a double-covered sphere. If the revolved curve is not a circle, the surface is called a '' toroid'', as in a square toroid. Real-world objects that approximate a torus of revolution include swim rings, inner tubes and ringette rings. Eyeglass lenses that combine spherical and cylindrical correction are toric lenses. A torus should not be confused with a '' solid torus'', which is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Torus
In geometry, a torus (plural tori, colloquially donut or doughnut) is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle. If the axis of revolution does not touch the circle, the surface has a ring shape and is called a torus of revolution. If the axis of revolution is tangent to the circle, the surface is a horn torus. If the axis of revolution passes twice through the circle, the surface is a spindle torus. If the axis of revolution passes through the center of the circle, the surface is a degenerate torus, a double-covered sphere. If the revolved curve is not a circle, the surface is called a '' toroid'', as in a square toroid. Real-world objects that approximate a torus of revolution include swim rings, inner tubes and ringette rings. Eyeglass lenses that combine spherical and cylindrical correction are toric lenses. A torus should not be confused with a '' solid torus'', which is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
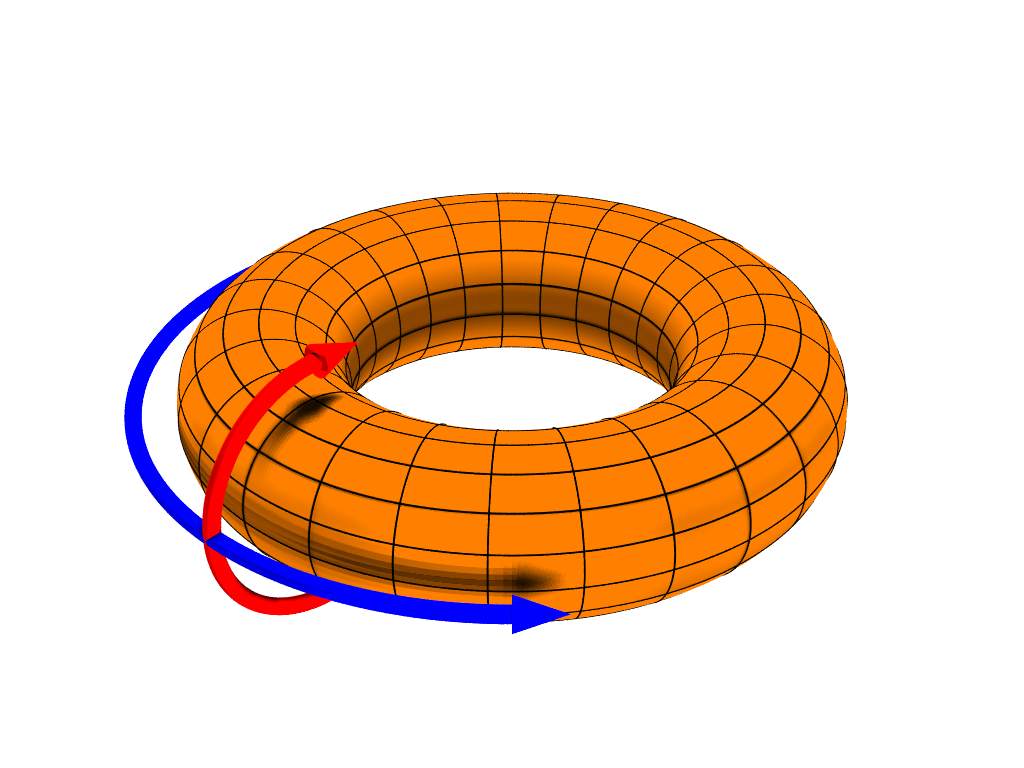
Torus Cycles
In geometry, a torus (plural tori, colloquially donut or doughnut) is a surface of revolution generated by revolving a circle in three-dimensional space about an axis that is coplanar with the circle. If the axis of revolution does not touch the circle, the surface has a ring shape and is called a torus of revolution. If the axis of revolution is tangent to the circle, the surface is a horn torus. If the axis of revolution passes twice through the circle, the surface is a spindle torus. If the axis of revolution passes through the center of the circle, the surface is a degenerate torus, a double-covered sphere. If the revolved curve is not a circle, the surface is called a ''toroid'', as in a square toroid. Real-world objects that approximate a torus of revolution include swim rings, inner tubes and ringette rings. Eyeglass lenses that combine spherical and cylindrical correction are toric lenses. A torus should not be confused with a ''solid torus'', which is formed by ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Clifford Torus
In geometric topology, the Clifford torus is the simplest and most symmetric flat embedding of the cartesian product of two circles ''S'' and ''S'' (in the same sense that the surface of a cylinder is "flat"). It is named after William Kingdon Clifford. It resides in R4, as opposed to in R3. To see why R4 is necessary, note that if ''S'' and ''S'' each exists in its own independent embedding space R and R, the resulting product space will be R4 rather than R3. The historically popular view that the cartesian product of two circles is an R3 torus in contrast requires the highly asymmetric application of a rotation operator to the second circle, since that circle will only have one independent axis ''z'' available to it after the first circle consumes ''x'' and ''y''. Stated another way, a torus embedded in R3 is an asymmetric reduced-dimension projection of the maximally symmetric Clifford torus embedded in R4. The relationship is similar to that of projecting the edges o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Toric Lens
A toric lens is a lens with different optical power and focal length in two orientations perpendicular to each other. One of the lens surfaces is shaped like a "cap" from a torus (see figure at right), and the other one is usually spherical. Such a lens behaves like a combination of a spherical lens and a cylindrical lens. Toric lenses are used primarily in eyeglasses, contact lenses and intraocular lenses to correct astigmatism. Torus A torus is the surface of revolution resulting when a circle with radius ''r'' rotates around an axis lying within the same plane as the circle, at a distance ''R'' from the circle's centre (see figure at right). If ''R'' > ''r'', a ''ring torus'' is produced. If ''R'' = ''r'', a ''horn torus'' is produced, where the opening is contracted into a single point. ''R'' < ''r'' results in a ''spindle torus'', where only two "dips" remain from the opening; these dips become less deep as ''R'' approaches 0. When ''R''&nbs ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Solid Torus
In mathematics, a solid torus is the topological space formed by sweeping a disk around a circle. It is homeomorphic to the Cartesian product S^1 \times D^2 of the disk and the circle, endowed with the product topology. A standard way to visualize a solid torus is as a toroid, embedded in 3-space. However, it should be distinguished from a torus, which has the same visual appearance: the torus is the two-dimensional space on the boundary of a toroid, while the solid torus includes also the compact interior space enclosed by the torus. Topological properties The solid torus is a connected, compact, orientable 3-dimensional manifold with boundary. The boundary is homeomorphic to S^1 \times S^1, the ordinary torus. Since the disk D^2 is contractible, the solid torus has the homotopy type of a circle, S^1.. Therefore the fundamental group and homology groups are isomorphic to those of the circle: \begin \pi_1\left(S^1 \times D^2\right) &\cong \pi_1\left(S^1\right) \cong \m ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Genus (mathematics)
In mathematics, genus (plural genera) has a few different, but closely related, meanings. Intuitively, the genus is the number of "holes" of a surface. A sphere has genus 0, while a torus has genus 1. Topology Orientable surfaces The genus of a connected, orientable surface is an integer representing the maximum number of cuttings along non-intersecting closed simple curves without rendering the resultant manifold disconnected. It is equal to the number of handles on it. Alternatively, it can be defined in terms of the Euler characteristic ''χ'', via the relationship ''χ'' = 2 − 2''g'' for closed surfaces, where ''g'' is the genus. For surfaces with ''b'' boundary components, the equation reads ''χ'' = 2 − 2''g'' − ''b''. In layman's terms, it's the number of "holes" an object has ("holes" interpreted in the sense of doughnut holes; a hollow sphere would be considered as having zero holes in this sense). A torus has 1 su ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Topology
In mathematics, topology (from the Greek words , and ) is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing holes, opening holes, tearing, gluing, or passing through itself. A topological space is a set endowed with a structure, called a '' topology'', which allows defining continuous deformation of subspaces, and, more generally, all kinds of continuity. Euclidean spaces, and, more generally, metric spaces are examples of a topological space, as any distance or metric defines a topology. The deformations that are considered in topology are homeomorphisms and homotopies. A property that is invariant under such deformations is a topological property. Basic examples of topological properties are: the dimension, which allows distinguishing between a line and a surface; compactness, which allows distinguishing between a line and a circle; ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Toroid
In mathematics, a toroid is a surface of revolution with a hole in the middle. The axis of revolution passes through the hole and so does not intersect the surface. For example, when a rectangle is rotated around an axis parallel to one of its edges, then a hollow rectangle-section ring is produced. If the revolved figure is a circle, then the object is called a torus. The term ''toroid'' is also used to describe a toroidal polyhedron. In this context a toroid need not be circular and may have any number of holes. A ''g''-holed ''toroid'' can be seen as approximating the surface of a torus having a topological genus, ''g'', of 1 or greater. The Euler characteristic χ of a ''g'' holed toroid is 2(1-''g'').Stewart, B.; "Adventures Among the Toroids:A Study of Orientable Polyhedra with Regular Faces", 2nd Edition, Stewart (1980). The torus is an example of a toroid, which is the surface of a doughnut. Doughnuts are an example of a solid torus created by rotating a disk, and s ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Möbius Strip
In mathematics, a Möbius strip, Möbius band, or Möbius loop is a surface that can be formed by attaching the ends of a strip of paper together with a half-twist. As a mathematical object, it was discovered by Johann Benedict Listing and August Ferdinand Möbius in 1858, but it had already appeared in Roman mosaics from the third century CE. The Möbius strip is a non-orientable surface, meaning that within it one cannot consistently distinguish clockwise from counterclockwise turns. Every non-orientable surface contains a Möbius strip. As an abstract topological space, the Möbius strip can be embedded into three-dimensional Euclidean space in many different ways: a clockwise half-twist is different from a counterclockwise half-twist, and it can also be embedded with odd numbers of twists greater than one, or with a knotted centerline. Any two embeddings with the same knot for the centerline and the same number and direction of twists are topologically equivalent. All o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Surface Of Revolution
A surface of revolution is a surface in Euclidean space created by rotating a curve (the generatrix) around an axis of rotation. Examples of surfaces of revolution generated by a straight line are cylindrical and conical surfaces depending on whether or not the line is parallel to the axis. A circle that is rotated around any diameter generates a sphere of which it is then a great circle, and if the circle is rotated around an axis that does not intersect the interior of a circle, then it generates a torus which does not intersect itself (a ring torus). Properties The sections of the surface of revolution made by planes through the axis are called ''meridional sections''. Any meridional section can be considered to be the generatrix in the plane determined by it and the axis. The sections of the surface of revolution made by planes that are perpendicular to the axis are circles. Some special cases of hyperboloids (of either one or two sheets) and elliptic paraboloids are sur ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Homeomorphism
In the mathematical field of topology, a homeomorphism, topological isomorphism, or bicontinuous function is a bijective and continuous function between topological spaces that has a continuous inverse function. Homeomorphisms are the isomorphisms in the category of topological spaces—that is, they are the mappings that preserve all the topological properties of a given space. Two spaces with a homeomorphism between them are called homeomorphic, and from a topological viewpoint they are the same. The word ''homeomorphism'' comes from the Greek words '' ὅμοιος'' (''homoios'') = similar or same and '' μορφή'' (''morphē'') = shape or form, introduced to mathematics by Henri Poincaré in 1895. Very roughly speaking, a topological space is a geometric object, and the homeomorphism is a continuous stretching and bending of the object into a new shape. Thus, a square and a circle are homeomorphic to each other, but a sphere and a torus are not. However, ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Ringette Ring
Ringette is a non-contact winter team sport played on ice hockey rinks using ice hockey skates, straight sticks with drag-tips, and a blue, rubber, pneumatic ring designed for use on ice surfaces. The sport is among a small number of organized team sports created exclusively for female competitors. Though ice hockey rinks are used, ringette rinks use markings specific to ringette and the sport uses strategic play which more closely resembles basketball than ice hockey. The sport was created in Canada for girls in 1963 by Sam Jacks from West Ferris, Ontario and Red McCarthy from Espanola, Ontario. In 2018, over 50,000 players registered to play the sport. Ringette is played predominantly in Canada and Finland with both countries forming the sport's top international teams on a regular basis. Several other countries currently organize and compete in the sport including Sweden, the United States, the Czech Republic, and Slovakia. The sport has continued to grow and has sprea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |