divisor (algebraic geometry) on:
[Wikipedia]
[Google]
[Amazon]
In
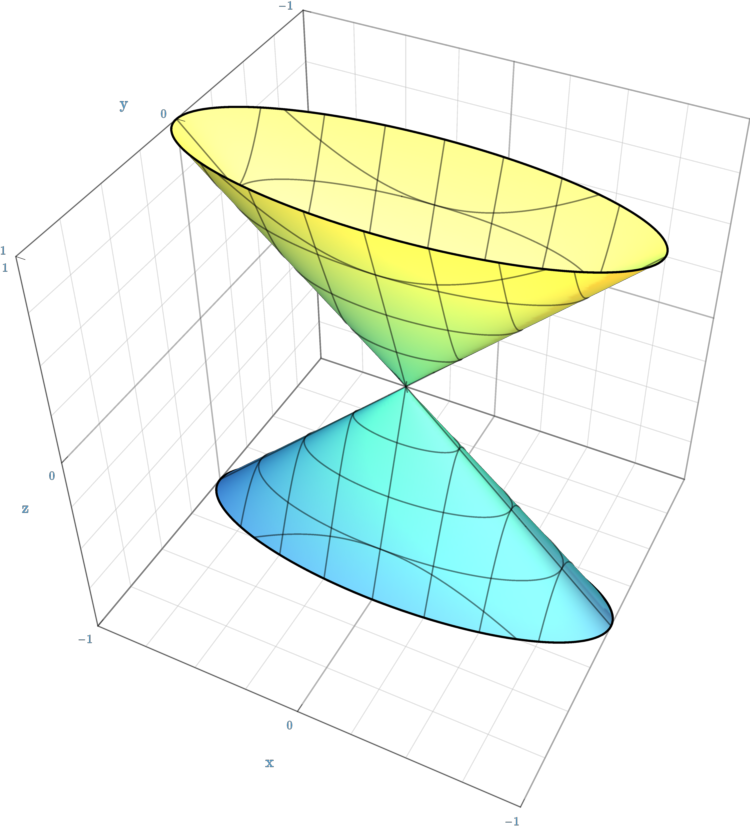 Let ''X'' be the quadric cone of dimension 2, defined by the equation ''xy'' = ''z''2 in affine 3-space over a field. Then the line ''D'' in ''X'' defined by ''x'' = ''z'' = 0 is not principal on ''X'' near the origin. Note that ''D'' ''can'' be defined as a set by one equation on ''X'', namely ''x'' = 0; but the function ''x'' on ''X'' vanishes to order 2 along ''D'', and so we only find that 2''D'' is Cartier (as defined below) on ''X''. In fact, the divisor class group Cl(''X'') is isomorphic to the cyclic group Z/2, generated by the class of ''D''.
* Let ''X'' be the quadric cone of dimension 3, defined by the equation ''xy'' = ''zw'' in affine 4-space over a field. Then the plane ''D'' in ''X'' defined by ''x'' = ''z'' = 0 cannot be defined in ''X'' by one equation near the origin, even as a set. It follows that ''D'' is not Q-Cartier on ''X''; that is, no positive multiple of ''D'' is Cartier. In fact, the divisor class group Cl(''X'') is isomorphic to the integers Z, generated by the class of ''D''.
Let ''X'' be the quadric cone of dimension 2, defined by the equation ''xy'' = ''z''2 in affine 3-space over a field. Then the line ''D'' in ''X'' defined by ''x'' = ''z'' = 0 is not principal on ''X'' near the origin. Note that ''D'' ''can'' be defined as a set by one equation on ''X'', namely ''x'' = 0; but the function ''x'' on ''X'' vanishes to order 2 along ''D'', and so we only find that 2''D'' is Cartier (as defined below) on ''X''. In fact, the divisor class group Cl(''X'') is isomorphic to the cyclic group Z/2, generated by the class of ''D''.
* Let ''X'' be the quadric cone of dimension 3, defined by the equation ''xy'' = ''zw'' in affine 4-space over a field. Then the plane ''D'' in ''X'' defined by ''x'' = ''z'' = 0 cannot be defined in ''X'' by one equation near the origin, even as a set. It follows that ''D'' is not Q-Cartier on ''X''; that is, no positive multiple of ''D'' is Cartier. In fact, the divisor class group Cl(''X'') is isomorphic to the integers Z, generated by the class of ''D''.
algebraic geometry
Algebraic geometry is a branch of mathematics, classically studying zeros of multivariate polynomials. Modern algebraic geometry is based on the use of abstract algebraic techniques, mainly from commutative algebra, for solving geometrical ...
, divisors are a generalization of codimension
In mathematics, codimension is a basic geometric idea that applies to subspaces in vector spaces, to submanifolds in manifolds, and suitable subsets of algebraic varieties.
For affine and projective algebraic varieties, the codimension equals ...
-1 subvarieties of algebraic varieties. Two different generalizations are in common use, Cartier divisors and Weil divisors (named for Pierre Cartier and André Weil
André Weil (; ; 6 May 1906 – 6 August 1998) was a French mathematician, known for his foundational work in number theory and algebraic geometry. He was a founding member and the ''de facto'' early leader of the mathematical Bourbaki group. Th ...
by David Mumford). Both are derived from the notion of divisibility in the integer
An integer is the number zero (), a positive natural number (, , , etc.) or a negative integer with a minus sign ( −1, −2, −3, etc.). The negative numbers are the additive inverses of the corresponding positive numbers. In the languag ...
s and algebraic number fields.
Globally, every codimension-1 subvariety of projective space
In mathematics, the concept of a projective space originated from the visual effect of perspective, where parallel lines seem to meet ''at infinity''. A projective space may thus be viewed as the extension of a Euclidean space, or, more generally ...
is defined by the vanishing of one homogeneous polynomial
In mathematics, a homogeneous polynomial, sometimes called quantic in older texts, is a polynomial whose nonzero terms all have the same degree. For example, x^5 + 2 x^3 y^2 + 9 x y^4 is a homogeneous polynomial of degree 5, in two variables; ...
; by contrast, a codimension-''r'' subvariety need not be definable by only ''r'' equations when ''r'' is greater than 1. (That is, not every subvariety of projective space is a complete intersection
In mathematics, an algebraic variety ''V'' in projective space is a complete intersection if the ideal of ''V'' is generated by exactly ''codim V'' elements. That is, if ''V'' has dimension ''m'' and lies in projective space ''P'n'', there shou ...
.) Locally, every codimension-1 subvariety of a smooth variety can be defined by one equation in a neighborhood of each point. Again, the analogous statement fails for higher-codimension subvarieties. As a result of this property, much of algebraic geometry studies an arbitrary variety by analysing its codimension-1 subvarieties and the corresponding line bundle
In mathematics, a line bundle expresses the concept of a line that varies from point to point of a space. For example, a curve in the plane having a tangent line at each point determines a varying line: the '' tangent bundle'' is a way of organisi ...
s.
On singular varieties, this property can also fail, and so one has to distinguish between codimension-1 subvarieties and varieties which can locally be defined by one equation. The former are Weil divisors while the latter are Cartier divisors.
Topologically, Weil divisors play the role of homology classes, while Cartier divisors represent cohomology
In mathematics, specifically in homology theory and algebraic topology, cohomology is a general term for a sequence of abelian groups, usually one associated with a topological space, often defined from a cochain complex. Cohomology can be view ...
classes. On a smooth variety (or more generally a regular scheme
In algebraic geometry, a regular scheme is a locally Noetherian scheme whose local rings are regular everywhere. Every smooth scheme is regular, and every regular scheme of finite type over a perfect field is smooth..
For an example of a regul ...
), a result analogous to Poincaré duality says that Weil and Cartier divisors are the same.
The name "divisor" goes back to the work of Dedekind and Weber
Weber (, or ; German: ) is a surname of German origin, derived from the noun meaning " weaver". In some cases, following migration to English-speaking countries, it has been anglicised to the English surname 'Webber' or even 'Weaver'.
Notable pe ...
, who showed the relevance of Dedekind domains to the study of algebraic curves. The group of divisors on a curve (the free abelian group generated by all divisors) is closely related to the group of fractional ideals for a Dedekind domain.
An algebraic cycle In mathematics, an algebraic cycle on an algebraic variety ''V'' is a formal linear combination of subvarieties of ''V''. These are the part of the algebraic topology of ''V'' that is directly accessible by algebraic methods. Understanding the al ...
is a higher codimension generalization of a divisor; by definition, a Weil divisor is a cycle of codimension 1.
Divisors on a Riemann surface
ARiemann surface
In mathematics, particularly in complex analysis, a Riemann surface is a connected one-dimensional complex manifold. These surfaces were first studied by and are named after Bernhard Riemann. Riemann surfaces can be thought of as deformed ver ...
is a 1-dimensional complex manifold
In differential geometry and complex geometry, a complex manifold is a manifold with an atlas of charts to the open unit disc in \mathbb^n, such that the transition maps are holomorphic.
The term complex manifold is variously used to mean a ...
, and so its codimension-1 submanifolds have dimension 0. The group of divisors on a compact
Compact as used in politics may refer broadly to a pact or treaty; in more specific cases it may refer to:
* Interstate compact
* Blood compact, an ancient ritual of the Philippines
* Compact government, a type of colonial rule utilized in Britis ...
Riemann surface ''X'' is the free abelian group on the points of ''X''.
Equivalently, a divisor on a compact Riemann surface ''X'' is a finite linear combination of points of ''X'' with integer
An integer is the number zero (), a positive natural number (, , , etc.) or a negative integer with a minus sign ( −1, −2, −3, etc.). The negative numbers are the additive inverses of the corresponding positive numbers. In the languag ...
coefficients. The degree of a divisor on ''X'' is the sum of its coefficients.
For any nonzero meromorphic function ''f'' on ''X'', one can define the order of vanishing of ''f'' at a point ''p'' in ''X'', ord''p''(''f''). It is an integer, negative if ''f'' has a pole at ''p''. The divisor of a nonzero meromorphic function ''f'' on the compact Riemann surface ''X'' is defined as
:
which is a finite sum. Divisors of the form (''f'') are also called principal divisors. Since (''fg'') = (''f'') + (''g''), the set of principal divisors is a subgroup of the group of divisors. Two divisors that differ by a principal divisor are called linearly equivalent.
On a compact Riemann surface, the degree of a principal divisor is zero; that is, the number of zeros of a meromorphic function is equal to the number of poles, counted with multiplicity. As a result, the degree is well-defined on linear equivalence classes of divisors.
Given a divisor ''D'' on a compact Riemann surface ''X'', it is important to study the complex vector space
In mathematics and physics, a vector space (also called a linear space) is a set whose elements, often called '' vectors'', may be added together and multiplied ("scaled") by numbers called ''scalars''. Scalars are often real numbers, but can ...
of meromorphic functions on ''X'' with poles at most given by ''D'', called ''H''0(''X'', ''O''(''D'')) or the space of sections of the line bundle associated to ''D''. The degree of ''D'' says a lot about the dimension of this vector space. For example, if ''D'' has negative degree, then this vector space is zero (because a meromorphic function cannot have more zeros than poles). If ''D'' has positive degree, then the dimension of ''H''0(''X'', ''O''(''mD'')) grows linearly in ''m'' for ''m'' sufficiently large. The Riemann–Roch theorem is a more precise statement along these lines. On the other hand, the precise dimension of ''H''0(''X'', ''O''(''D'')) for divisors ''D'' of low degree is subtle, and not completely determined by the degree of ''D''. The distinctive features of a compact Riemann surface are reflected in these dimensions.
One key divisor on a compact Riemann surface is the canonical divisor In mathematics, the canonical bundle of a non-singular algebraic variety V of dimension n over a field is the line bundle \,\!\Omega^n = \omega, which is the ''n''th exterior power of the cotangent bundle Ω on ''V''.
Over the complex numbers ...
. To define it, one first defines the divisor of a nonzero meromorphic 1-form
In differential geometry, a one-form on a differentiable manifold is a smooth section of the cotangent bundle. Equivalently, a one-form on a manifold M is a smooth mapping of the total space of the tangent bundle of M to \R whose restriction to ...
along the lines above. Since the space of meromorphic 1-forms is a 1-dimensional vector space over the field of meromorphic functions, any two nonzero meromorphic 1-forms yield linearly equivalent divisors. Any divisor in this linear equivalence class is called the canonical divisor of ''X'', ''K''''X''. The genus
Genus ( plural genera ) is a taxonomic rank used in the biological classification of living and fossil organisms as well as viruses. In the hierarchy of biological classification, genus comes above species and below family. In binomial nom ...
''g'' of ''X'' can be read from the canonical divisor: namely, ''K''''X'' has degree 2''g'' − 2. The key trichotomy among compact Riemann surfaces ''X'' is whether the canonical divisor has negative degree (so ''X'' has genus zero), zero degree (genus one), or positive degree (genus at least 2). For example, this determines whether ''X'' has a Kähler metric with positive curvature
In mathematics, curvature is any of several strongly related concepts in geometry. Intuitively, the curvature is the amount by which a curve deviates from being a straight line, or a surface deviates from being a plane.
For curves, the can ...
, zero curvature, or negative curvature. The canonical divisor has negative degree if and only if ''X'' is isomorphic to the Riemann sphere
In mathematics, the Riemann sphere, named after Bernhard Riemann, is a model of the extended complex plane: the complex plane plus one point at infinity. This extended plane represents the extended complex numbers, that is, the complex numbers ...
CP1.
Weil divisors
Let ''X'' be anintegral
In mathematics, an integral assigns numbers to functions in a way that describes displacement, area, volume, and other concepts that arise by combining infinitesimal data. The process of finding integrals is called integration. Along with ...
locally Noetherian scheme. A prime divisor or irreducible divisor on ''X'' is an integral
In mathematics, an integral assigns numbers to functions in a way that describes displacement, area, volume, and other concepts that arise by combining infinitesimal data. The process of finding integrals is called integration. Along with ...
closed subscheme ''Z'' of codimension
In mathematics, codimension is a basic geometric idea that applies to subspaces in vector spaces, to submanifolds in manifolds, and suitable subsets of algebraic varieties.
For affine and projective algebraic varieties, the codimension equals ...
1 in ''X''. A Weil divisor on ''X'' is a formal sum over the prime divisors ''Z'' of ''X'',
:
where the collection is locally finite. If ''X'' is quasi-compact, local finiteness is equivalent to being finite. The group of all Weil divisors is denoted . A Weil divisor ''D'' is effective if all the coefficients are non-negative. One writes if the difference is effective.
For example, a divisor on an algebraic curve over a field is a formal sum of finitely many closed points. A divisor on is a formal sum of prime numbers with integer coefficients and therefore corresponds to a non-zero fractional ideal in Q. A similar characterization is true for divisors on where ''K'' is a number field.
If ''Z'' ⊂ ''X'' is a prime divisor, then the local ring has Krull dimension one. If is non-zero, then the order of vanishing of ''f'' along ''Z'', written , is the length
Length is a measure of distance. In the International System of Quantities, length is a quantity with dimension distance. In most systems of measurement a base unit for length is chosen, from which all other units are derived. In the Inte ...
of This length is finite, and it is additive with respect to multiplication, that is, . If ''k''(''X'') is the field of rational functions on ''X'', then any non-zero may be written as a quotient , where ''g'' and ''h'' are in and the order of vanishing of ''f'' is defined to be . With this definition, the order of vanishing is a function . If ''X'' is normal, then the local ring is a discrete valuation ring, and the function is the corresponding valuation. For a non-zero rational function ''f'' on ''X'', the principal Weil divisor associated to ''f'' is defined to be the Weil divisor
:
It can be shown that this sum is locally finite and hence that it indeed defines a Weil divisor. The principal Weil divisor associated to ''f'' is also notated . If ''f'' is a regular function, then its principal Weil divisor is effective, but in general this is not true. The additivity of the order of vanishing function implies that
:
Consequently is a homomorphism, and in particular its image is a subgroup of the group of all Weil divisors.
Let ''X'' be a normal integral Noetherian scheme. Every Weil divisor ''D'' determines a coherent sheaf
In mathematics, especially in algebraic geometry and the theory of complex manifolds, coherent sheaves are a class of sheaves closely linked to the geometric properties of the underlying space. The definition of coherent sheaves is made with ref ...
on ''X''. Concretely it may be defined as subsheaf of the sheaf of rational functionsKollár (2013), Notation 1.2.
:
That is, a nonzero rational function ''f'' is a section of over ''U'' if and only if for any prime divisor ''Z'' intersecting ''U'',
:
where ''nZ'' is the coefficient of ''Z'' in ''D''. If ''D'' is principal, so ''D'' is the divisor of a rational function ''g'', then there is an isomorphism
:
since is an effective divisor and so is regular thanks to the normality of ''X''. Conversely, if is isomorphic to as an -module, then ''D'' is principal. It follows that ''D'' is locally principal if and only if is invertible; that is, a line bundle.
If ''D'' is an effective divisor that corresponds to a subscheme of ''X'' (for example ''D'' can be a reduced divisor or a prime divisor), then the ideal sheaf of the subscheme ''D'' is equal to This leads to an often used short exact sequence,
:
The sheaf cohomology In mathematics, sheaf cohomology is the application of homological algebra to analyze the global sections of a sheaf on a topological space. Broadly speaking, sheaf cohomology describes the obstructions to solving a geometric problem globally whe ...
of this sequence shows that contains information on whether regular functions on ''D'' are the restrictions of regular functions on ''X''.
There is also an inclusion of sheaves
:
This furnishes a canonical element of namely, the image of the global section 1. This is called the ''canonical section'' and may be denoted ''sD''. While the canonical section is the image of a nowhere vanishing rational function, its image in vanishes along ''D'' because the transition functions vanish along ''D''. When ''D'' is a smooth Cartier divisor, the cokernel of the above inclusion may be identified; see #Cartier divisors below.
Assume that ''X'' is a normal integral separated scheme of finite type over a field. Let ''D'' be a Weil divisor. Then is a rank one reflexive sheaf In algebraic geometry, a reflexive sheaf is a coherent sheaf that is isomorphic to its second dual (as a sheaf of modules) via the canonical map. The second dual of a coherent sheaf is called the reflexive hull of the sheaf. A basic example of a ref ...
, and since is defined as a subsheaf of it is a fractional ideal sheaf (see below). Conversely, every rank one reflexive sheaf corresponds to a Weil divisor: The sheaf can be restricted to the regular locus, where it becomes free and so corresponds to a Cartier divisor (again, see below), and because the singular locus has codimension at least two, the closure of the Cartier divisor is a Weil divisor.
Divisor class group
The Weil divisor class group Cl(''X'') is the quotient of Div(''X'') by the subgroup of all principal Weil divisors. Two divisors are said to be linearly equivalent if their difference is principal, so the divisor class group is the group of divisors modulo linear equivalence. For a variety ''X'' of dimension ''n'' over a field, the divisor class group is aChow group
In algebraic geometry, the Chow groups (named after Wei-Liang Chow by ) of an algebraic variety over any field are algebro-geometric analogs of the homology of a topological space. The elements of the Chow group are formed out of subvarieties (so- ...
; namely, Cl(''X'') is the Chow group CH''n''−1(''X'') of (''n''−1)-dimensional cycles.
Let ''Z'' be a closed subset of ''X''. If ''Z'' is irreducible of codimension one, then Cl(''X'' − ''Z'') is isomorphic to the quotient group of Cl(''X'') by the class of ''Z''. If ''Z'' has codimension at least 2 in ''X'', then the restriction Cl(''X'') → Cl(''X'' − ''Z'') is an isomorphism. (These facts are special cases of the localization sequence for Chow groups.)
On a normal integral Noetherian scheme ''X'', two Weil divisors ''D'', ''E'' are linearly equivalent if and only if and are isomorphic as -modules. Isomorphism classes of reflexive sheaves on ''X'' form a monoid with product given as the reflexive hull of a tensor product. Then defines a monoid isomorphism from the Weil divisor class group of ''X'' to the monoid of isomorphism classes of rank-one reflexive sheaves on ''X''.
Examples
* Let ''k'' be a field, and let ''n'' be a positive integer. Since the polynomial ring ''k'' 'x''1, ..., ''xn''is a unique factorization domain, the divisor class group of affine space A''n'' over ''k'' is equal to zero. Sinceprojective space
In mathematics, the concept of a projective space originated from the visual effect of perspective, where parallel lines seem to meet ''at infinity''. A projective space may thus be viewed as the extension of a Euclidean space, or, more generally ...
P''n'' over ''k'' minus a hyperplane ''H'' is isomorphic to A''n'', it follows that the divisor class group of P''n'' is generated by the class of ''H''. From there, it is straightforward to check that Cl(P''n'') is in fact isomorphic to the integers Z, generated by ''H''. Concretely, this means that every codimension-1 subvariety of P''n'' is defined by the vanishing of a single homogeneous polynomial.
* Let ''X'' be an algebraic curve over a field ''k''. Every closed point ''p'' in ''X'' has the form Spec ''E'' for some finite extension field ''E'' of ''k'', and the degree of ''p'' is defined to be the degree of ''E'' over ''k''. Extending this by linearity gives the notion of degree for a divisor on ''X''. If ''X'' is a projective curve over ''k'', then the divisor of a nonzero rational function ''f'' on ''X'' has degree zero. As a result, for a projective curve ''X'', the degree gives a homomorphism deg: Cl(''X'') → Z.
* For the projective line P1 over a field ''k'', the degree gives an isomorphism Cl(P1) ≅ Z. For any smooth projective curve ''X'' with a ''k''- rational point, the degree homomorphism is surjective, and the kernel is isomorphic to the group of ''k''-points on the Jacobian variety of ''X'', which is an abelian variety
In mathematics, particularly in algebraic geometry, complex analysis and algebraic number theory, an abelian variety is a projective algebraic variety that is also an algebraic group, i.e., has a group law that can be defined by regular functi ...
of dimension equal to the genus of ''X''. It follows, for example, that the divisor class group of a complex elliptic curve
In mathematics, an elliptic curve is a smooth, projective, algebraic curve of genus one, on which there is a specified point . An elliptic curve is defined over a field and describes points in , the Cartesian product of with itself. I ...
is an uncountable abelian group.
* Generalizing the previous example: for any smooth projective variety ''X'' over a field ''k'' such that ''X'' has a ''k''-rational point, the divisor class group Cl(''X'') is an extension of a finitely generated abelian group
In abstract algebra, an abelian group (G,+) is called finitely generated if there exist finitely many elements x_1,\dots,x_s in G such that every x in G can be written in the form x = n_1x_1 + n_2x_2 + \cdots + n_sx_s for some integers n_1,\dots, ...
, the Néron–Severi group In algebraic geometry, the Néron–Severi group of a variety is
the group of divisors modulo algebraic equivalence; in other words it is the group of components of the Picard scheme of a variety. Its rank is called the Picard number. It is nam ...
, by the group of ''k''-points of a connected group scheme For ''k'' of characteristic zero, is an abelian variety, the Picard variety
In mathematics, the Picard group of a ringed space ''X'', denoted by Pic(''X''), is the group of isomorphism classes of invertible sheaves (or line bundles) on ''X'', with the group operation being tensor product. This construction is a global ...
of ''X''.
*For ''R'' the ring of integers of a number field, the divisor class group Cl(''R'') := Cl(Spec ''R'') is also called the ideal class group of ''R''. It is a finite abelian group. Understanding ideal class groups is a central goal of algebraic number theory
Algebraic number theory is a branch of number theory that uses the techniques of abstract algebra to study the integers, rational numbers, and their generalizations. Number-theoretic questions are expressed in terms of properties of algebraic o ...
.
* The canonical divisor
Let ''X'' be a normal variety over a perfect field. The smooth locus ''U'' of ''X'' is an open subset whose complement has codimension at least 2. Let ''j'': ''U'' → ''X'' be the inclusion map, then the restriction homomorphism: : is an isomorphism, since ''X'' − ''U'' has codimension at least 2 in ''X''. For example, one can use this isomorphism to define thecanonical divisor In mathematics, the canonical bundle of a non-singular algebraic variety V of dimension n over a field is the line bundle \,\!\Omega^n = \omega, which is the ''n''th exterior power of the cotangent bundle Ω on ''V''.
Over the complex numbers ...
''K''''X'' of ''X'': it is the Weil divisor (up to linear equivalence) corresponding to the line bundle of differential forms of top degree on ''U''. Equivalently, the sheaf on ''X'' is the direct image sheaf where ''n'' is the dimension of ''X''.
Example: Let ''X'' = P''n'' be the projective ''n''-space with the homogeneous coordinates ''x''0, ..., ''xn''. Let ''U'' = . Then ''U'' is isomorphic to the affine ''n''-space with the coordinates ''yi'' = ''xi''/''x''0. Let
:
Then ω is a rational differential form on ''U''; thus, it is a rational section of which has simple poles along ''Zi'' = , ''i'' = 1, ..., ''n''. Switching to a different affine chart changes only the sign of ω and so we see ω has a simple pole along ''Z''0 as well. Thus, the divisor of ω is
:
and its divisor class is
: