Torus on:
[Wikipedia]
[Google]
[Amazon]

 In
In
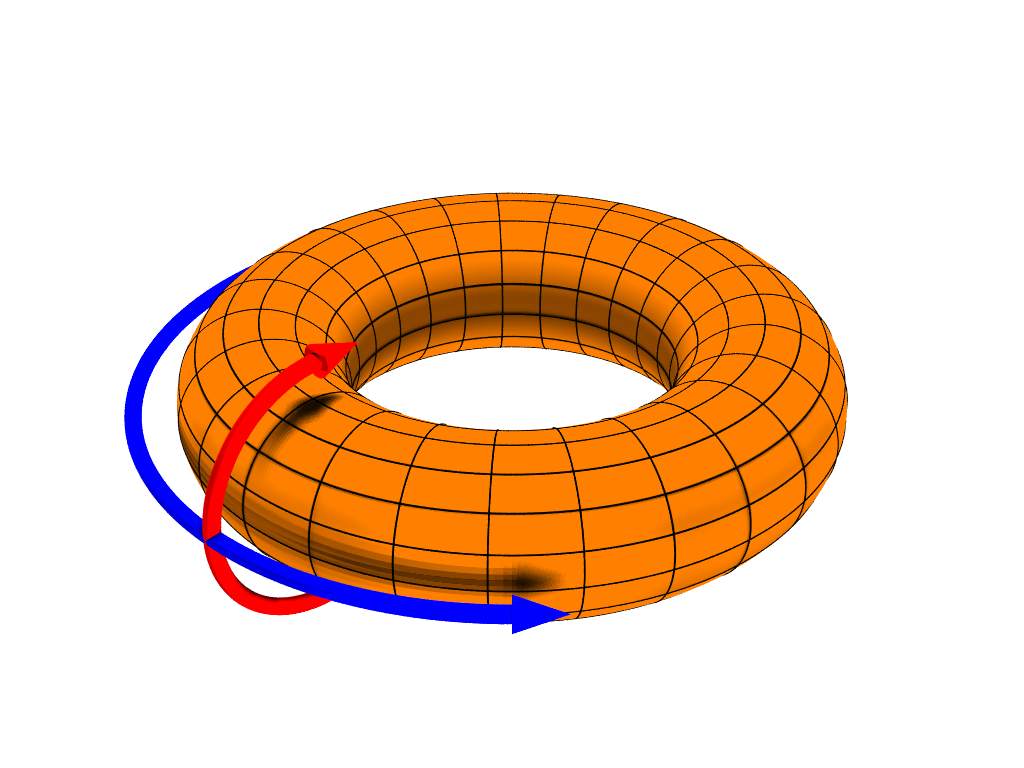 As a torus is the product of two circles, a modified version of the
As a torus is the product of two circles, a modified version of the
 The fundamental group of the torus is just the direct product of the fundamental group of the circle with itself:
:
Intuitively speaking, this means that a closed path that circles the torus' "hole" (say, a circle that traces out a particular latitude) and then circles the torus' "body" (say, a circle that traces out a particular longitude) can be deformed to a path that circles the body and then the hole. So, strictly 'latitudinal' and strictly 'longitudinal' paths commute. An equivalent statement may be imagined as two shoelaces passing through each other, then unwinding, then rewinding.
If a torus is punctured and turned inside out then another torus results, with lines of latitude and longitude interchanged. This is equivalent to building a torus from a cylinder, by joining the circular ends together, in two ways: around the outside like joining two ends of a garden hose, or through the inside like rolling a sock (with the toe cut off). Additionally, if the cylinder was made by gluing two opposite sides of a rectangle together, choosing the other two sides instead will cause the same reversal of orientation.
The first homology group of the torus is isomorphic to the fundamental group (this follows from Hurewicz theorem since the fundamental group is
The fundamental group of the torus is just the direct product of the fundamental group of the circle with itself:
:
Intuitively speaking, this means that a closed path that circles the torus' "hole" (say, a circle that traces out a particular latitude) and then circles the torus' "body" (say, a circle that traces out a particular longitude) can be deformed to a path that circles the body and then the hole. So, strictly 'latitudinal' and strictly 'longitudinal' paths commute. An equivalent statement may be imagined as two shoelaces passing through each other, then unwinding, then rewinding.
If a torus is punctured and turned inside out then another torus results, with lines of latitude and longitude interchanged. This is equivalent to building a torus from a cylinder, by joining the circular ends together, in two ways: around the outside like joining two ends of a garden hose, or through the inside like rolling a sock (with the toe cut off). Additionally, if the cylinder was made by gluing two opposite sides of a rectangle together, choosing the other two sides instead will cause the same reversal of orientation.
The first homology group of the torus is isomorphic to the fundamental group (this follows from Hurewicz theorem since the fundamental group is
 The torus has a generalization to higher dimensions, the , often called the or for short. (This is the more typical meaning of the term "''n''-torus", the other referring to ''n'' holes or of genus ''n''.) Recalling that the torus is the product space of two circles, the ''n''-dimensional torus is the product of ''n'' circles. That is:
:
The standard 1-torus is just the circle: . The torus discussed above is the standard 2-torus, . And similar to the 2-torus, the ''n''-torus, can be described as a quotient of under integral shifts in any coordinate. That is, the ''n''-torus is modulo the action of the integer lattice (with the action being taken as vector addition). Equivalently, the ''n''-torus is obtained from the ''n''-dimensional
The torus has a generalization to higher dimensions, the , often called the or for short. (This is the more typical meaning of the term "''n''-torus", the other referring to ''n'' holes or of genus ''n''.) Recalling that the torus is the product space of two circles, the ''n''-dimensional torus is the product of ''n'' circles. That is:
:
The standard 1-torus is just the circle: . The torus discussed above is the standard 2-torus, . And similar to the 2-torus, the ''n''-torus, can be described as a quotient of under integral shifts in any coordinate. That is, the ''n''-torus is modulo the action of the integer lattice (with the action being taken as vector addition). Equivalently, the ''n''-torus is obtained from the ''n''-dimensional
 As the ''n''-torus is the ''n''-fold product of the circle, the ''n''-torus is the configuration space of ''n'' ordered, not necessarily distinct points on the circle. Symbolically, . The configuration space of ''unordered'', not necessarily distinct points is accordingly the orbifold , which is the quotient of the torus by the
As the ''n''-torus is the ''n''-fold product of the circle, the ''n''-torus is the configuration space of ''n'' ordered, not necessarily distinct points on the circle. Symbolically, . The configuration space of ''unordered'', not necessarily distinct points is accordingly the orbifold , which is the quotient of the torus by the


 A flat torus is a torus with the metric inherited from its representation as the quotient, /L, where L is a discrete subgroup of isomorphic to . This gives the quotient the structure of a Riemannian manifold. Perhaps the simplest example of this is when : , which can also be described as the Cartesian plane under the identifications . This particular flat torus (and any uniformly scaled version of it) is known as the "square" flat torus.
This metric of the square flat torus can also be realised by specific embeddings of the familiar 2-torus into Euclidean 4-space or higher dimensions. Its surface has zero Gaussian curvature everywhere. Its surface is flat in the same sense that the surface of a cylinder is flat. In 3 dimensions, one can bend a flat sheet of paper into a cylinder without stretching the paper, but this cylinder cannot be bent into a torus without stretching the paper (unless some regularity and differentiability conditions are given up, see below).
A simple 4-dimensional Euclidean embedding of a rectangular flat torus (more general than the square one) is as follows:
:
where ''R'' and ''P'' are positive constants determining the aspect ratio. It is diffeomorphic to a regular torus but not
A flat torus is a torus with the metric inherited from its representation as the quotient, /L, where L is a discrete subgroup of isomorphic to . This gives the quotient the structure of a Riemannian manifold. Perhaps the simplest example of this is when : , which can also be described as the Cartesian plane under the identifications . This particular flat torus (and any uniformly scaled version of it) is known as the "square" flat torus.
This metric of the square flat torus can also be realised by specific embeddings of the familiar 2-torus into Euclidean 4-space or higher dimensions. Its surface has zero Gaussian curvature everywhere. Its surface is flat in the same sense that the surface of a cylinder is flat. In 3 dimensions, one can bend a flat sheet of paper into a cylinder without stretching the paper, but this cylinder cannot be bent into a torus without stretching the paper (unless some regularity and differentiability conditions are given up, see below).
A simple 4-dimensional Euclidean embedding of a rectangular flat torus (more general than the square one) is as follows:
:
where ''R'' and ''P'' are positive constants determining the aspect ratio. It is diffeomorphic to a regular torus but not
 Polyhedra with the topological type of a torus are called toroidal polyhedra, and have Euler characteristic ''V'' − ''E'' + ''F'' = 0. For any number of holes, the formula generalizes to ''V'' − ''E'' + ''F'' = 2 − 2''N'', where ''N'' is the number of holes.
The term "toroidal polyhedron" is also used for higher-genus polyhedra and for immersions of toroidal polyhedra.
Polyhedra with the topological type of a torus are called toroidal polyhedra, and have Euler characteristic ''V'' − ''E'' + ''F'' = 0. For any number of holes, the formula generalizes to ''V'' − ''E'' + ''F'' = 2 − 2''N'', where ''N'' is the number of holes.
The term "toroidal polyhedron" is also used for higher-genus polyhedra and for immersions of toroidal polyhedra.

 In combinatorial mathematics, a ''de Bruijn torus'' is an array of symbols from an alphabet (often just 0 and 1) that contains every ''m''-by-''n''
In combinatorial mathematics, a ''de Bruijn torus'' is an array of symbols from an alphabet (often just 0 and 1) that contains every ''m''-by-''n''
''Algebraic Topology''
Cambridge University Press, 2002. . *V. V. Nikulin, I. R. Shafarevich. ''Geometries and Groups''. Springer, 1987. , .
"Tore (notion géométrique)" at ''Encyclopédie des Formes Mathématiques Remarquables''
Creation of a torus
at cut-the-knot
"4D torus"
Fly-through cross-sections of a four-dimensional torus
"Relational Perspective Map"
Visualizing high dimensional data with flat torus
*Archived a
Ghostarchive
and th
Wayback Machine
* {{Authority control Surfaces
 In
In geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, a torus (plural tori, colloquially donut or doughnut) is a surface of revolution generated by revolving a circle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
in three-dimensional space about an axis that is coplanar with the circle.
If the axis of revolution does not touch the circle, the surface has a ring shape and is called a torus of revolution. If the axis of revolution is tangent to the circle, the surface is a horn torus. If the axis of revolution passes twice through the circle, the surface is a spindle torus. If the axis of revolution passes through the center of the circle, the surface is a degenerate torus, a double-covered sphere
A sphere () is a Geometry, geometrical object that is a solid geometry, three-dimensional analogue to a two-dimensional circle. A sphere is the Locus (mathematics), set of points that are all at the same distance from a given point in three ...
. If the revolved curve is not a circle, the surface is called a '' toroid'', as in a square toroid.
Real-world objects that approximate a torus of revolution include swim ring
A swim ring (also known as a swimming ring, swim tube, rubber ring, water donut, floatie, inner tube, or, in the United States, a lifesaver) is a toroid-shaped (hence the name "ring" or "doughnut") inflatable water toy. The swim ring was derived ...
s, inner tubes and ringette ring
Ringette is a non-contact winter team sport played on ice hockey rinks using ice hockey skates, straight sticks with drag-tips, and a blue, rubber, pneumatic ring designed for use on ice surfaces. The sport is among a small number of organiz ...
s. Eyeglass lenses that combine spherical and cylindrical correction are toric lenses.
A torus should not be confused with a ''solid torus
In mathematics, a solid torus is the topological space formed by sweeping a disk around a circle. It is homeomorphic to the Cartesian product S^1 \times D^2 of the disk and the circle, endowed with the product topology.
A standard way to visuali ...
'', which is formed by rotating a disk, rather than a circle, around an axis. A solid torus is a torus plus the volume
Volume is a measure of occupied three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch). Th ...
inside the torus. Real-world objects that approximate a ''solid torus'' include O-rings, non-inflatable lifebuoys, ring doughnuts, and bagels.
In topology
In mathematics, topology (from the Greek words , and ) is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing ho ...
, a ring torus is homeomorphic to the Cartesian product
In mathematics, specifically set theory, the Cartesian product of two sets ''A'' and ''B'', denoted ''A''×''B'', is the set of all ordered pairs where ''a'' is in ''A'' and ''b'' is in ''B''. In terms of set-builder notation, that is
: A\ ...
of two circle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
s: , and the latter is taken to be the definition in that context. It is a compact 2-manifold of genus 1. The ring torus is one way to embed this space into Euclidean space
Euclidean space is the fundamental space of geometry, intended to represent physical space. Originally, that is, in Euclid's ''Elements'', it was the three-dimensional space of Euclidean geometry, but in modern mathematics there are Euclidean sp ...
, but another way to do this is the Cartesian product of the embedding of in the plane with itself. This produces a geometric object called the Clifford torus, a surface in 4-space.
In the field of topology
In mathematics, topology (from the Greek words , and ) is concerned with the properties of a geometric object that are preserved under continuous deformations, such as stretching, twisting, crumpling, and bending; that is, without closing ho ...
, a torus is any topological space that is homeomorphic to a torus. The surface of a coffee cup and a doughnut are both topological tori with genus
Genus ( plural genera ) is a taxonomic rank used in the biological classification of living and fossil organisms as well as viruses. In the hierarchy of biological classification, genus comes above species and below family. In binomial n ...
one.
An example of a torus can be constructed by taking a rectangular strip of flexible material, for example, a rubber sheet, and joining the top edge to the bottom edge, and the left edge to the right edge, without any half-twists (compare Möbius strip).
Geometry
A torus can be defined parametrically by: where *, are angles which make a full circle, so their values start and end at the same point, * is the distance from the center of the tube to the center of the torus, * is the radius of the tube. Angle represents rotation around the tube, whereas represents rotation around the torus' axis of revolution. is known as the "major radius" and is known as the "minor radius". The ratio divided by is known as the "aspect ratio". The typical doughnut confectionery has an aspect ratio of about 3 to 2. An implicit function, implicit equation in Cartesian coordinates for a torus radially symmetric about the -coordinate axis, axis is or the solution of , where Algebraically eliminating the square root gives a quartic equation, The three classes of standard tori correspond to the three possible aspect ratios between and : *When , the surface will be the familiar ring torus or anchor ring. * corresponds to the horn torus, which in effect is a torus with no "hole". * describes the self-intersecting spindle torus; its inner shell is a '' lemon'' and its outer shell is an ''apple
An apple is an edible fruit produced by an apple tree (''Malus domestica''). Apple trees are cultivated worldwide and are the most widely grown species in the genus '' Malus''. The tree originated in Central Asia, where its wild ances ...
''
*When , the torus degenerates to the sphere.
When , the interior
Interior may refer to:
Arts and media
* ''Interior'' (Degas) (also known as ''The Rape''), painting by Edgar Degas
* ''Interior'' (play), 1895 play by Belgian playwright Maurice Maeterlinck
* ''The Interior'' (novel), by Lisa See
* Interior de ...
of this torus is diffeomorphic (and, hence, homeomorphic) to a product of a Euclidean open disk and a circle. The volume
Volume is a measure of occupied three-dimensional space. It is often quantified numerically using SI derived units (such as the cubic metre and litre) or by various imperial or US customary units (such as the gallon, quart, cubic inch). Th ...
of this solid torus and the surface area of its torus are easily computed using Pappus's centroid theorem, giving:
These formulas are the same as for a cylinder of length and radius , obtained from cutting the tube along the plane of a small circle, and unrolling it by straightening out (rectifying) the line running around the center of the tube. The losses in surface area and volume on the inner side of the tube exactly cancel out the gains on the outer side.
Expressing the surface area and the volume by the distance of an outermost point on the surface of the torus to the center, and the distance of an innermost point to the center (so that and ), yields
 As a torus is the product of two circles, a modified version of the
As a torus is the product of two circles, a modified version of the spherical coordinate system
In mathematics, a spherical coordinate system is a coordinate system for three-dimensional space where the position of a point is specified by three numbers: the ''radial distance'' of that point from a fixed origin, its ''polar angle'' me ...
is sometimes used.
In traditional spherical coordinates there are three measures, , the distance from the center of the coordinate system, and and , angles measured from the center point.
As a torus has, effectively, two center points, the centerpoints of the angles are moved; measures the same angle as it does in the spherical system, but is known as the "toroidal" direction. The center point of is moved to the center of , and is known as the "poloidal" direction. These terms were first used in a discussion of the Earth's magnetic field, where "poloidal" was used to denote "the direction toward the poles".
In modern use, toroidal and poloidal are more commonly used to discuss magnetic confinement fusion devices.
Topology
Topologically, a torus is a closed surface defined as the product of twocircle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is const ...
s: ''S''1 × ''S''1. This can be viewed as lying in C2 and is a subset of the 3-sphere ''S''3 of radius √2. This topological torus is also often called the Clifford torus. In fact, ''S''3 is filled out by a family of nested tori in this manner (with two degenerate circles), a fact which is important in the study of ''S''3 as a fiber bundle over ''S''2 (the Hopf bundle).
The surface described above, given the relative topology from , is homeomorphic to a topological torus as long as it does not intersect its own axis. A particular homeomorphism is given by stereographically projecting the topological torus into from the north pole of ''S''3.
The torus can also be described as a quotient of the Cartesian plane under the identifications
:
or, equivalently, as the quotient of the unit square
In mathematics, a unit square is a square whose sides have length . Often, ''the'' unit square refers specifically to the square in the Cartesian plane with corners at the four points ), , , and .
Cartesian coordinates
In a Cartesian coordina ...
by pasting the opposite edges together, described as a fundamental polygon ''ABA''−1''B''−1.
 The fundamental group of the torus is just the direct product of the fundamental group of the circle with itself:
:
Intuitively speaking, this means that a closed path that circles the torus' "hole" (say, a circle that traces out a particular latitude) and then circles the torus' "body" (say, a circle that traces out a particular longitude) can be deformed to a path that circles the body and then the hole. So, strictly 'latitudinal' and strictly 'longitudinal' paths commute. An equivalent statement may be imagined as two shoelaces passing through each other, then unwinding, then rewinding.
If a torus is punctured and turned inside out then another torus results, with lines of latitude and longitude interchanged. This is equivalent to building a torus from a cylinder, by joining the circular ends together, in two ways: around the outside like joining two ends of a garden hose, or through the inside like rolling a sock (with the toe cut off). Additionally, if the cylinder was made by gluing two opposite sides of a rectangle together, choosing the other two sides instead will cause the same reversal of orientation.
The first homology group of the torus is isomorphic to the fundamental group (this follows from Hurewicz theorem since the fundamental group is
The fundamental group of the torus is just the direct product of the fundamental group of the circle with itself:
:
Intuitively speaking, this means that a closed path that circles the torus' "hole" (say, a circle that traces out a particular latitude) and then circles the torus' "body" (say, a circle that traces out a particular longitude) can be deformed to a path that circles the body and then the hole. So, strictly 'latitudinal' and strictly 'longitudinal' paths commute. An equivalent statement may be imagined as two shoelaces passing through each other, then unwinding, then rewinding.
If a torus is punctured and turned inside out then another torus results, with lines of latitude and longitude interchanged. This is equivalent to building a torus from a cylinder, by joining the circular ends together, in two ways: around the outside like joining two ends of a garden hose, or through the inside like rolling a sock (with the toe cut off). Additionally, if the cylinder was made by gluing two opposite sides of a rectangle together, choosing the other two sides instead will cause the same reversal of orientation.
The first homology group of the torus is isomorphic to the fundamental group (this follows from Hurewicz theorem since the fundamental group is abelian
Abelian may refer to:
Mathematics Group theory
* Abelian group, a group in which the binary operation is commutative
** Category of abelian groups (Ab), has abelian groups as objects and group homomorphisms as morphisms
* Metabelian group, a grou ...
).
Two-sheeted cover
The 2-torus double-covers the 2-sphere, with fourramification point
In geometry, ramification is 'branching out', in the way that the square root function, for complex numbers, can be seen to have two ''branches'' differing in sign. The term is also used from the opposite perspective (branches coming together) as ...
s. Every conformal structure on the 2-torus can be represented as a two-sheeted cover of the 2-sphere. The points on the torus corresponding to the ramification points are the Weierstrass point In mathematics, a Weierstrass point P on a nonsingular algebraic curve C defined over the complex numbers is a point such that there are more functions on C, with their poles restricted to P only, than would be predicted by the Riemann–Roch theore ...
s. In fact, the conformal type of the torus is determined by the cross-ratio of the four points.
''n''-dimensional torus
 The torus has a generalization to higher dimensions, the , often called the or for short. (This is the more typical meaning of the term "''n''-torus", the other referring to ''n'' holes or of genus ''n''.) Recalling that the torus is the product space of two circles, the ''n''-dimensional torus is the product of ''n'' circles. That is:
:
The standard 1-torus is just the circle: . The torus discussed above is the standard 2-torus, . And similar to the 2-torus, the ''n''-torus, can be described as a quotient of under integral shifts in any coordinate. That is, the ''n''-torus is modulo the action of the integer lattice (with the action being taken as vector addition). Equivalently, the ''n''-torus is obtained from the ''n''-dimensional
The torus has a generalization to higher dimensions, the , often called the or for short. (This is the more typical meaning of the term "''n''-torus", the other referring to ''n'' holes or of genus ''n''.) Recalling that the torus is the product space of two circles, the ''n''-dimensional torus is the product of ''n'' circles. That is:
:
The standard 1-torus is just the circle: . The torus discussed above is the standard 2-torus, . And similar to the 2-torus, the ''n''-torus, can be described as a quotient of under integral shifts in any coordinate. That is, the ''n''-torus is modulo the action of the integer lattice (with the action being taken as vector addition). Equivalently, the ''n''-torus is obtained from the ''n''-dimensional hypercube
In geometry, a hypercube is an ''n''-dimensional analogue of a square () and a cube (). It is a closed, compact, convex figure whose 1-skeleton consists of groups of opposite parallel line segments aligned in each of the space's dimensions ...
by gluing the opposite faces together.
An ''n''-torus in this sense is an example of an ''n-''dimensional compact manifold. It is also an example of a compact abelian
Abelian may refer to:
Mathematics Group theory
* Abelian group, a group in which the binary operation is commutative
** Category of abelian groups (Ab), has abelian groups as objects and group homomorphisms as morphisms
* Metabelian group, a grou ...
Lie group. This follows from the fact that the unit circle
In mathematics, a unit circle is a circle of unit radius—that is, a radius of 1. Frequently, especially in trigonometry, the unit circle is the circle of radius 1 centered at the origin (0, 0) in the Cartesian coordinate system in the Eucli ...
is a compact abelian Lie group (when identified with the unit complex number
In mathematics, a complex number is an element of a number system that extends the real numbers with a specific element denoted , called the imaginary unit and satisfying the equation i^= -1; every complex number can be expressed in the for ...
s with multiplication). Group multiplication on the torus is then defined by coordinate-wise multiplication.
Toroidal groups play an important part in the theory of compact Lie groups. This is due in part to the fact that in any compact Lie group ''G'' one can always find a maximal torus; that is, a closed subgroup which is a torus of the largest possible dimension. Such maximal tori ''T'' have a controlling role to play in theory of connected ''G''. Toroidal groups are examples of protori, which (like tori) are compact connected abelian groups, which are not required to be manifolds.
Automorphisms of ''T'' are easily constructed from automorphisms of the lattice , which are classified by invertible integral matrices of size ''n'' with an integral inverse; these are just the integral matrices with determinant ±1. Making them act on in the usual way, one has the typical ''toral automorphism'' on the quotient.
The fundamental group of an ''n''-torus is a free abelian group of rank ''n''. The ''k''-th homology group of an ''n''-torus is a free abelian group of rank ''n'' choose ''k''. It follows that the Euler characteristic of the ''n''-torus is 0 for all ''n''. The cohomology ring ''H''•(, Z) can be identified with the exterior algebra over the Z- module whose generators are the duals of the ''n'' nontrivial cycles.
Configuration space
 As the ''n''-torus is the ''n''-fold product of the circle, the ''n''-torus is the configuration space of ''n'' ordered, not necessarily distinct points on the circle. Symbolically, . The configuration space of ''unordered'', not necessarily distinct points is accordingly the orbifold , which is the quotient of the torus by the
As the ''n''-torus is the ''n''-fold product of the circle, the ''n''-torus is the configuration space of ''n'' ordered, not necessarily distinct points on the circle. Symbolically, . The configuration space of ''unordered'', not necessarily distinct points is accordingly the orbifold , which is the quotient of the torus by the symmetric group
In abstract algebra, the symmetric group defined over any set is the group whose elements are all the bijections from the set to itself, and whose group operation is the composition of functions. In particular, the finite symmetric group ...
on ''n'' letters (by permuting the coordinates).
For ''n'' = 2, the quotient is the Möbius strip, the edge corresponding to the orbifold points where the two coordinates coincide. For ''n'' = 3 this quotient may be described as a solid torus with cross-section an equilateral triangle, with a twist; equivalently, as a triangular prism whose top and bottom faces are connected with a 1/3 twist (120°): the 3-dimensional interior corresponds to the points on the 3-torus where all 3 coordinates are distinct, the 2-dimensional face corresponds to points with 2 coordinates equal and the 3rd different, while the 1-dimensional edge corresponds to points with all 3 coordinates identical.
These orbifolds have found significant applications to music theory in the work of Dmitri Tymoczko and collaborators (Felipe Posada, Michael Kolinas, et al.), being used to model musical triads.
Flat torus


 A flat torus is a torus with the metric inherited from its representation as the quotient, /L, where L is a discrete subgroup of isomorphic to . This gives the quotient the structure of a Riemannian manifold. Perhaps the simplest example of this is when : , which can also be described as the Cartesian plane under the identifications . This particular flat torus (and any uniformly scaled version of it) is known as the "square" flat torus.
This metric of the square flat torus can also be realised by specific embeddings of the familiar 2-torus into Euclidean 4-space or higher dimensions. Its surface has zero Gaussian curvature everywhere. Its surface is flat in the same sense that the surface of a cylinder is flat. In 3 dimensions, one can bend a flat sheet of paper into a cylinder without stretching the paper, but this cylinder cannot be bent into a torus without stretching the paper (unless some regularity and differentiability conditions are given up, see below).
A simple 4-dimensional Euclidean embedding of a rectangular flat torus (more general than the square one) is as follows:
:
where ''R'' and ''P'' are positive constants determining the aspect ratio. It is diffeomorphic to a regular torus but not
A flat torus is a torus with the metric inherited from its representation as the quotient, /L, where L is a discrete subgroup of isomorphic to . This gives the quotient the structure of a Riemannian manifold. Perhaps the simplest example of this is when : , which can also be described as the Cartesian plane under the identifications . This particular flat torus (and any uniformly scaled version of it) is known as the "square" flat torus.
This metric of the square flat torus can also be realised by specific embeddings of the familiar 2-torus into Euclidean 4-space or higher dimensions. Its surface has zero Gaussian curvature everywhere. Its surface is flat in the same sense that the surface of a cylinder is flat. In 3 dimensions, one can bend a flat sheet of paper into a cylinder without stretching the paper, but this cylinder cannot be bent into a torus without stretching the paper (unless some regularity and differentiability conditions are given up, see below).
A simple 4-dimensional Euclidean embedding of a rectangular flat torus (more general than the square one) is as follows:
:
where ''R'' and ''P'' are positive constants determining the aspect ratio. It is diffeomorphic to a regular torus but not isometric
The term ''isometric'' comes from the Greek for "having equal measurement".
isometric may mean:
* Cubic crystal system, also called isometric crystal system
* Isometre, a rhythmic technique in music.
* "Isometric (Intro)", a song by Madeon from ...
. It can not be analytically embedded ( smooth of class ) into Euclidean 3-space. Mapping it into ''3''-space requires one to stretch it, in which case it looks like a regular torus. For example, in the following map:
:
If ''R'' and ''P'' in the above flat torus parametrization form a unit vector then ''u'', ''v'', and 0 < ''η'' < /2 parameterize the unit 3-sphere as Hopf coordinates. In particular, for certain very specific choices of a square flat torus in the 3-sphere ''S''3, where above, the torus will partition the 3-sphere into two congruent solid tori subsets with the aforesaid flat torus surface as their common boundary. One example is the torus T defined by
:
Other tori in ''S''3 having this partitioning property include the square tori of the form ''Q''⋅T, where ''Q'' is a rotation of 4-dimensional space , or in other words ''Q'' is a member of the Lie group SO(4).
It is known that there exists no ''C''2 (twice continuously differentiable) embedding of a flat torus into 3-space. (The idea of the proof is to take a large sphere containing such a flat torus in its interior, and shrink the radius of the sphere until it just touches the torus for the first time. Such a point of contact must be a tangency. But that would imply that part of the torus, since it has zero curvature everywhere, must lie strictly outside the sphere, which is a contradiction.) On the other hand, according to the Nash-Kuiper theorem, which was proven in the 1950s, an isometric ''C''1 embedding exists. This is solely an existence proof and does not provide explicit equations for such an embedding.
In April 2012, an explicit ''C''1 (continuously differentiable) embedding of a flat torus into 3-dimensional Euclidean space was found. It is a flat torus in the sense that as metric spaces, it is isometric to a flat square torus. It is similar in structure to a fractal
In mathematics, a fractal is a geometric shape containing detailed structure at arbitrarily small scales, usually having a fractal dimension strictly exceeding the topological dimension. Many fractals appear similar at various scales, as il ...
as it is constructed by repeatedly corrugating an ordinary torus. Like fractals, it has no defined Gaussian curvature. However, unlike fractals, it does have defined surface normals, yielding a so-called "smooth fractal". The key to obtain the smoothness of this corrugated torus is to have the amplitudes of successive corrugations decreasing faster than their "wavelengths". (These infinitely recursive corrugations are used only for embedding into three dimensions; they are not an intrinsic feature of the flat torus.) This is the first time that any such embedding was defined by explicit equations or depicted by computer graphics.
Genus ''g'' surface
In the theory of surfaces there is another object, the "genus
Genus ( plural genera ) is a taxonomic rank used in the biological classification of living and fossil organisms as well as viruses. In the hierarchy of biological classification, genus comes above species and below family. In binomial n ...
" ''g'' surface. Instead of the product of ''n'' circles, a genus ''g'' surface is the connected sum of ''g'' two-tori. To form a connected sum of two surfaces, remove from each the interior of a disk and "glue" the surfaces together along the boundary circles. To form the connected sum of more than two surfaces, sum two of them at a time until they are all connected. In this sense, a genus ''g'' surface resembles the surface of ''g'' doughnuts stuck together side by side, or a 2-sphere with ''g'' handles attached.
As examples, a genus zero surface (without boundary) is the two-sphere while a genus one surface (without boundary) is the ordinary torus. The surfaces of higher genus are sometimes called ''n''-holed tori (or, rarely, ''n''-fold tori). The terms double torus and triple torus are also occasionally used.
The classification theorem for surfaces states that every compact connected surface is topologically equivalent to either the sphere or the connect sum of some number of tori, disks, and real projective planes.
Toroidal polyhedra
 Polyhedra with the topological type of a torus are called toroidal polyhedra, and have Euler characteristic ''V'' − ''E'' + ''F'' = 0. For any number of holes, the formula generalizes to ''V'' − ''E'' + ''F'' = 2 − 2''N'', where ''N'' is the number of holes.
The term "toroidal polyhedron" is also used for higher-genus polyhedra and for immersions of toroidal polyhedra.
Polyhedra with the topological type of a torus are called toroidal polyhedra, and have Euler characteristic ''V'' − ''E'' + ''F'' = 0. For any number of holes, the formula generalizes to ''V'' − ''E'' + ''F'' = 2 − 2''N'', where ''N'' is the number of holes.
The term "toroidal polyhedron" is also used for higher-genus polyhedra and for immersions of toroidal polyhedra.
Automorphisms
Thehomeomorphism group In mathematics, particularly topology, the homeomorphism group of a topological space is the group consisting of all homeomorphisms from the space to itself with function composition as the group operation. Homeomorphism groups are very important in ...
(or the subgroup of diffeomorphisms) of the torus is studied in geometric topology
In mathematics, geometric topology is the study of manifolds and maps between them, particularly embeddings of one manifold into another.
History
Geometric topology as an area distinct from algebraic topology may be said to have originat ...
. Its mapping class group (the connected components of the homeomorphism group) is surjective onto the group of invertible integer matrices, which can be realized as linear maps on the universal covering space that preserve the standard lattice (this corresponds to integer coefficients) and thus descend to the quotient.
At the level of homotopy and homology, the mapping class group can be identified as the action on the first homology (or equivalently, first cohomology, or on the fundamental group, as these are all naturally isomorphic; also the first cohomology group generates the cohomology algebra:
:
Since the torus is an Eilenberg–MacLane space
In mathematics, specifically algebraic topology, an Eilenberg–MacLane space Saunders Mac Lane originally spelt his name "MacLane" (without a space), and co-published the papers establishing the notion of Eilenberg–MacLane spaces under this nam ...
''K''(''G'', 1), its homotopy equivalences, up to homotopy, can be identified with automorphisms of the fundamental group); all homotopy equivalences of the torus can be realized by homeomorphisms – every homotopy equivalence is homotopic to a homeomorphism.
Thus the short exact sequence of the mapping class group splits (an identification of the torus as the quotient of gives a splitting, via the linear maps, as above):
:
The mapping class group of higher genus surfaces is much more complicated, and an area of active research.
Coloring a torus
The torus's chromatic number is seven, meaning every graph that can be embedded on the torus has a chromatic number of at most seven. (Since thecomplete graph
In the mathematical field of graph theory, a complete graph is a simple undirected graph in which every pair of distinct vertices is connected by a unique edge. A complete digraph is a directed graph in which every pair of distinct vertices ...
can be embedded on the torus, and , the upper bound is tight.) Equivalently, in a torus divided into regions, it is always possible to color the regions using no more than seven colors so that no neighboring regions are the same color. (Contrast with the four color theorem
In mathematics, the four color theorem, or the four color map theorem, states that no more than four colors are required to color the regions of any map so that no two adjacent regions have the same color. ''Adjacent'' means that two regions sh ...
for the plane.)

de Bruijn torus
 In combinatorial mathematics, a ''de Bruijn torus'' is an array of symbols from an alphabet (often just 0 and 1) that contains every ''m''-by-''n''
In combinatorial mathematics, a ''de Bruijn torus'' is an array of symbols from an alphabet (often just 0 and 1) that contains every ''m''-by-''n'' matrix
Matrix most commonly refers to:
* ''The Matrix'' (franchise), an American media franchise
** '' The Matrix'', a 1999 science-fiction action film
** "The Matrix", a fictional setting, a virtual reality environment, within ''The Matrix'' (franchi ...
exactly once. It is a torus because the edges are considered wraparound for the purpose of finding matrices. Its name comes from the De Bruijn sequence, which can be considered a special case where ''n'' is 1 (one dimension).
Cutting a torus
A solid torus of revolution can be cut by ''n'' (> 0) planes into maximally : parts. The first 11 numbers of parts, for 0 ≤ ''n'' ≤ 10 (including the case of ''n'' = 0, not covered by the above formulas), are as follows: :1, 2, 6, 13, 24, 40, 62, 91, 128, 174, 230, ... .See also
Notes
*''Nociones de Geometría Analítica y Álgebra Lineal'', , Author: Kozak Ana Maria, Pompeya Pastorelli Sonia, Verdanega Pedro Emilio, Editorial: McGraw-Hill, Edition 2007, 744 pages, language: Spanish *Allen Hatcher''Algebraic Topology''
Cambridge University Press, 2002. . *V. V. Nikulin, I. R. Shafarevich. ''Geometries and Groups''. Springer, 1987. , .
"Tore (notion géométrique)" at ''Encyclopédie des Formes Mathématiques Remarquables''
References
External links
Creation of a torus
at cut-the-knot
"4D torus"
Fly-through cross-sections of a four-dimensional torus
"Relational Perspective Map"
Visualizing high dimensional data with flat torus
*Archived a
Ghostarchive
and th
Wayback Machine
* {{Authority control Surfaces