Spherical symmetry on:
[Wikipedia]
[Google]
[Amazon]
In
In 3-dimensions, a surface or
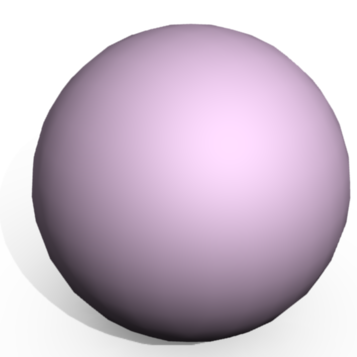An analogous 3-dimensional equivalent term is spherical symmetry.
Rotational spherical symmetry is isomorphic with the rotation group SO(3), and can be parametrized by the
geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, circular symmetry is a type of continuous symmetry for a planar object that can be rotated by any arbitrary angle
In Euclidean geometry, an angle is the figure formed by two rays, called the '' sides'' of the angle, sharing a common endpoint, called the '' vertex'' of the angle.
Angles formed by two rays lie in the plane that contains the rays. Angles ...
and map onto itself.
Rotational circular symmetry is isomorphic with the circle group in the complex plane, or the special orthogonal group
In mathematics, the orthogonal group in dimension , denoted , is the group of distance-preserving transformations of a Euclidean space of dimension that preserve a fixed point, where the group operation is given by composing transformations. T ...
SO(2), and unitary group U(1). Reflective circular symmetry is isomorphic with the orthogonal group
In mathematics, the orthogonal group in dimension , denoted , is the Group (mathematics), group of isometry, distance-preserving transformations of a Euclidean space of dimension that preserve a fixed point, where the group operation is given by ...
O(2).
Two dimensions
A 2-dimensional object with circular symmetry would consist of concentric circles and annular domains. Rotational circular symmetry has all cyclic symmetry, Z''n'' as subgroup symmetries. Reflective circular symmetry has all dihedral symmetry, Dih''n'' as subgroup symmetries.Three dimensions
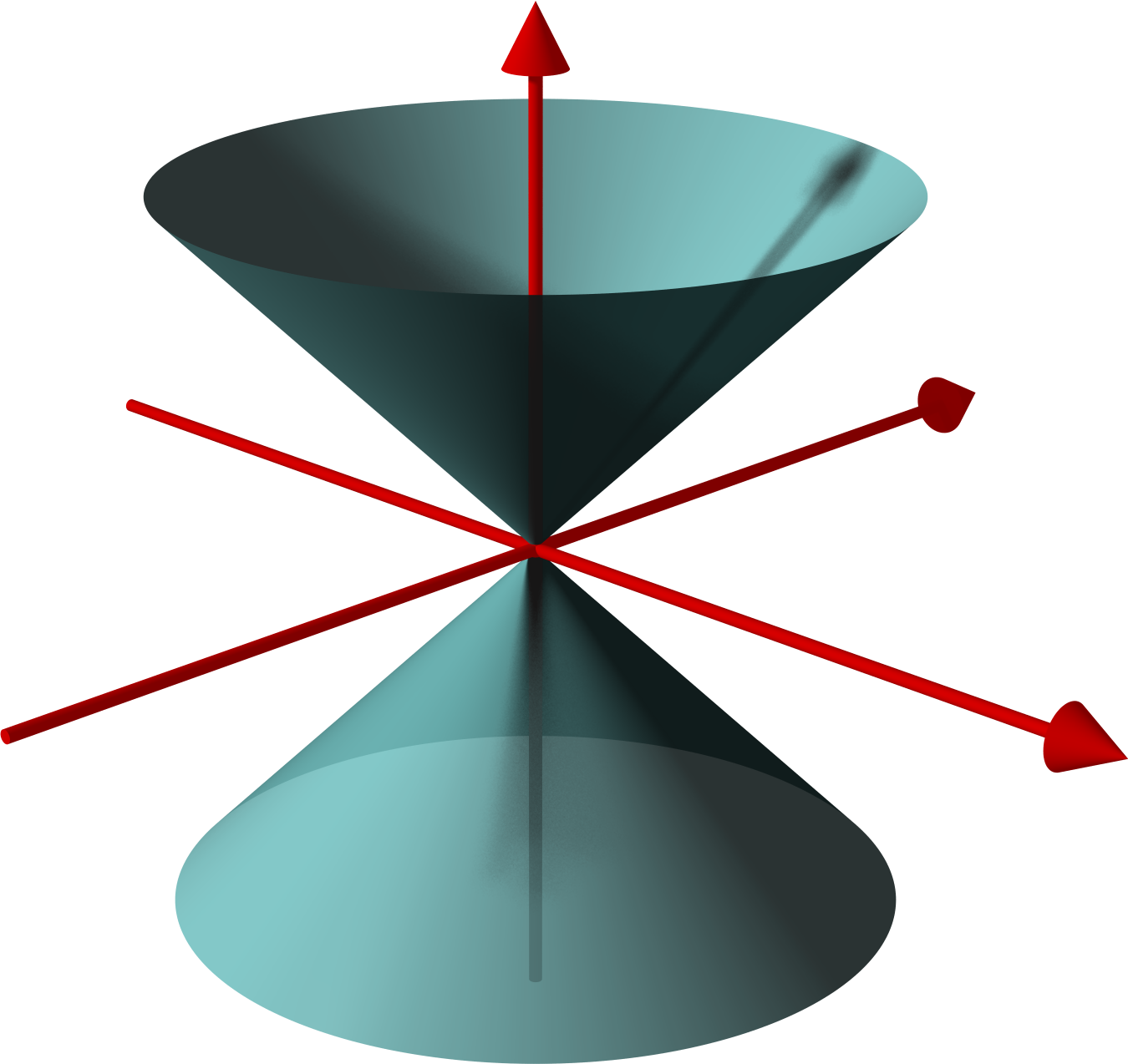
In 3-dimensions, a surface or solid of revolution
In geometry, a solid of revolution is a solid figure obtained by rotating a plane figure around some straight line (the ''axis of revolution'') that lies on the same plane. The surface created by this revolution and which bounds the solid is the ...
has circular symmetry around an axis, also called cylindrical symmetry or axial symmetry. An example is a right circular cone. Circular symmetry in 3 dimensions has all pyramidal symmetry, C''n''v as subgroups.
A double-cone, bicone, cylinder, toroid and spheroid have circular symmetry, and in addition have a bilateral symmetry perpendular to the axis of system (or half cylindrical symmetry). These reflective circular symmetries have all discrete prismatic symmetries, D''n''h as subgroups.
Four dimensions
In four dimensions, an object can have circular symmetry, on two orthogonal axis planes, or duocylindrical symmetry. For example, the duocylinder and Clifford torus have circular symmetry in two orthogonal axes. A spherinder has spherical symmetry in one 3-space, and circular symmetry in the orthogonal direction.Spherical symmetry
An analogous 3-dimensional equivalent term is spherical symmetry.
Rotational spherical symmetry is isomorphic with the rotation group SO(3), and can be parametrized by the Davenport chained rotations In physics and engineering, Davenport chained rotations are three chained intrinsic rotations about body-fixed specific axes. Euler rotations and Tait–Bryan rotations are particular cases of the Davenport general rotation decomposition. The angle ...
pitch, yaw, and roll. Rotational spherical symmetry has all the discrete chiral 3D point group
In geometry, a point group is a mathematical group of symmetry operations (isometries in a Euclidean space) that have a fixed point in common. The coordinate origin of the Euclidean space is conventionally taken to be a fixed point, and every p ...
s as subgroups. Reflectional spherical symmetry is isomorphic with the orthogonal group
In mathematics, the orthogonal group in dimension , denoted , is the Group (mathematics), group of isometry, distance-preserving transformations of a Euclidean space of dimension that preserve a fixed point, where the group operation is given by ...
O(3) and has the 3-dimensional discrete point groups as subgroups.
A scalar field
In mathematics and physics, a scalar field is a function (mathematics), function associating a single number to every point (geometry), point in a space (mathematics), space – possibly physical space. The scalar may either be a pure Scalar ( ...
has spherical symmetry if it depends on the distance to the origin only, such as the potential of a central force. A vector field has spherical symmetry if it is in radially inward or outward direction with a magnitude and orientation (inward/outward) depending on the distance to the origin only, such as a central force.
See also
* Isotropy * Rotational symmetry * Particle in a spherically symmetric potential * Gauss's theoremReferences
* * *{{springer, title=Orthogonal group, id=p/o070300 Symmetry Rotation