Poincaré Disk Model on:
[Wikipedia]
[Google]
[Amazon]

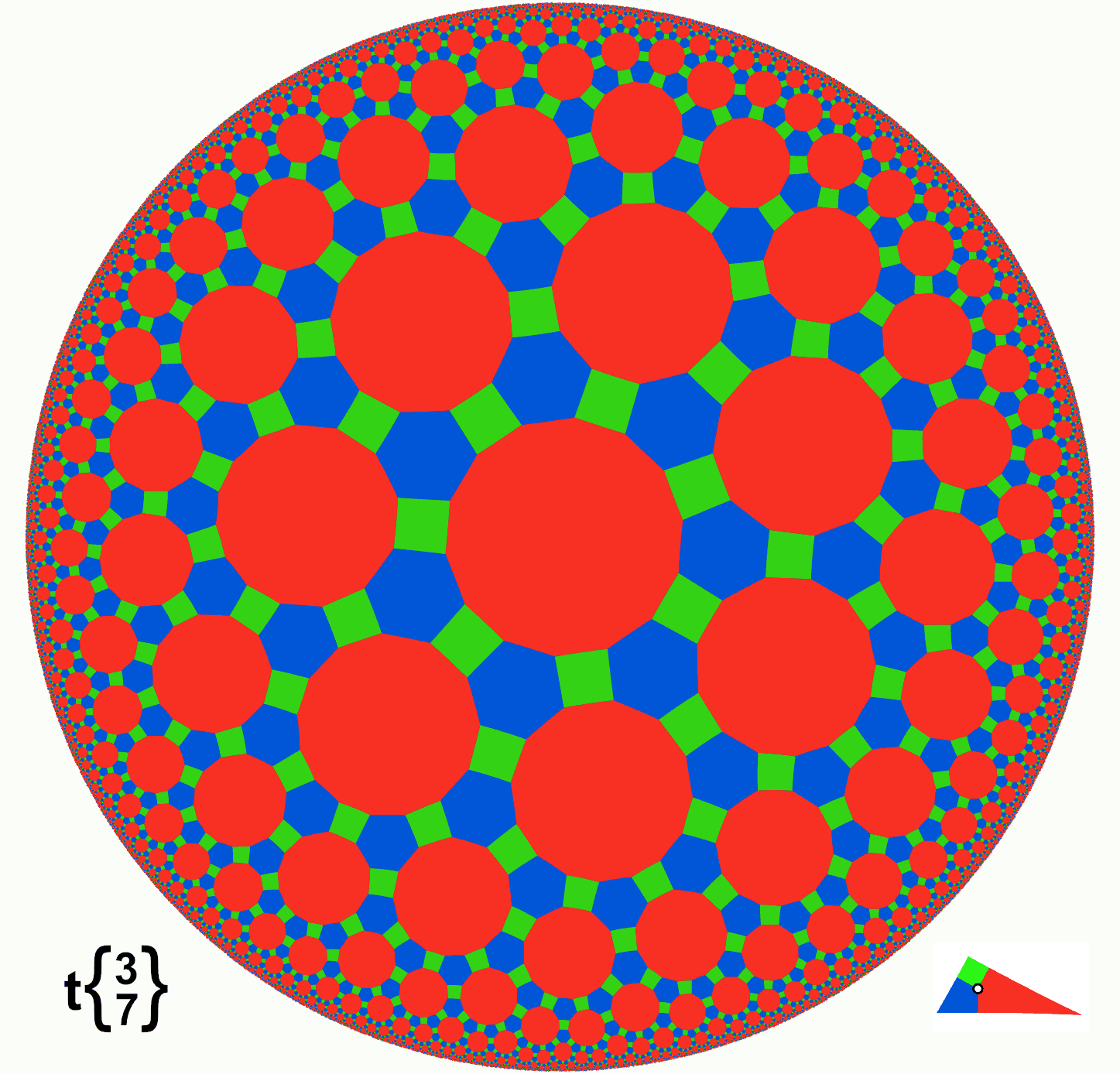 In
In
 Hyperbolic straight lines consist of all arcs of Euclidean circles contained within the disk that are
Hyperbolic straight lines consist of all arcs of Euclidean circles contained within the disk that are
 If ''u'' and ''v'' are two vectors in real ''n''-dimensional vector space R''n'' with the usual Euclidean norm, both of which have norm less than 1, then we may define an isometric invariant by
:
where denotes the usual Euclidean norm. Then the distance function is
:
Such a distance function is defined for any two vectors of norm less than one, and makes the set of such vectors into a metric space which is a model of hyperbolic space of constant curvature −1. The model has the conformal property that the angle between two intersecting curves in hyperbolic space is the same as the angle in the model.
The associated
If ''u'' and ''v'' are two vectors in real ''n''-dimensional vector space R''n'' with the usual Euclidean norm, both of which have norm less than 1, then we may define an isometric invariant by
:
where denotes the usual Euclidean norm. Then the distance function is
:
Such a distance function is defined for any two vectors of norm less than one, and makes the set of such vectors into a metric space which is a model of hyperbolic space of constant curvature −1. The model has the conformal property that the angle between two intersecting curves in hyperbolic space is the same as the angle in the model.
The associated

 The Poincaré disk model, as well as the Klein model, are related to the
The Poincaré disk model, as well as the Klein model, are related to the
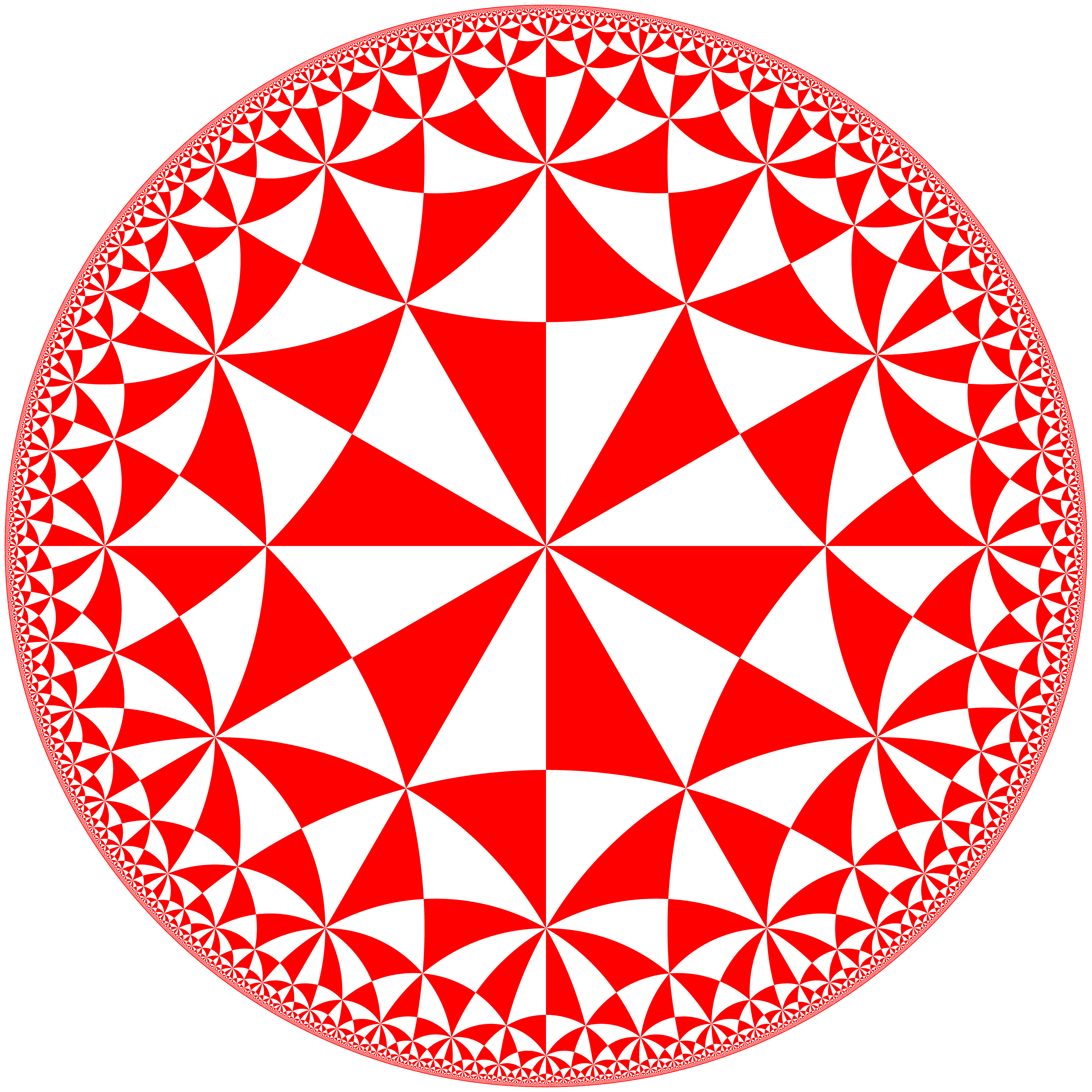
Circle Limit IV: Heaven and Hell
' in 1960.Escher's Circle Limit Exploration
/ref> According to Bruno Ernst, the best of them is ''
 In
In geometry
Geometry (; ) is, with arithmetic, one of the oldest branches of mathematics. It is concerned with properties of space such as the distance, shape, size, and relative position of figures. A mathematician who works in the field of geometry is c ...
, the Poincaré disk model, also called the conformal disk model, is a model of 2-dimensional hyperbolic geometry in which all points
Point or points may refer to:
Places
* Point, Lewis, a peninsula in the Outer Hebrides, Scotland
* Point, Texas, a city in Rains County, Texas, United States
* Point, the NE tip and a ferry terminal of Lismore, Inner Hebrides, Scotland
* Points ...
are inside the unit disk, and straight lines are either circular arcs contained within the disk that are orthogonal
In mathematics, orthogonality is the generalization of the geometric notion of '' perpendicularity''.
By extension, orthogonality is also used to refer to the separation of specific features of a system. The term also has specialized meanings in ...
to the unit circle or diameter
In geometry, a diameter of a circle is any straight line segment that passes through the center of the circle and whose endpoints lie on the circle. It can also be defined as the longest chord of the circle. Both definitions are also valid f ...
s of the unit circle.
The group of orientation preserving isometries of the disk model is given by the projective special unitary group , the quotient of the special unitary group SU(1,1) by its center .
Along with the Klein model and the Poincaré half-space model
Poincaré is a French surname. Notable people with the surname include:
* Henri Poincaré (1854–1912), French physicist, mathematician and philosopher of science
* Henriette Poincaré (1858-1943), wife of Prime Minister Raymond Poincaré
* Luci ...
, it was proposed by Eugenio Beltrami who used these models to show that hyperbolic geometry was equiconsistent with Euclidean geometry. It is named after Henri Poincaré
Jules Henri Poincaré ( S: stress final syllable ; 29 April 1854 – 17 July 1912) was a French mathematician, theoretical physicist, engineer, and philosopher of science. He is often described as a polymath, and in mathematics as "Th ...
, because his rediscovery of this representation fourteen years later became better known than the original work of Beltrami.
The Poincaré ball model is the similar model for ''3'' or ''n''-dimensional hyperbolic geometry in which the points of the geometry are in the ''n''-dimensional unit ball
Unit may refer to:
Arts and entertainment
* UNIT, a fictional military organization in the science fiction television series ''Doctor Who''
* Unit of action, a discrete piece of action (or beat) in a theatrical presentation
Music
* ''Unit'' (a ...
.
History
The Poincaré disk was described by Henri Poincaré in his 1882 treatment of hyperbolic, parabolic and elliptic functions, but became widely known following Poincaré's presentation in his 1905 philosophical treatise, '' Science and Hypothesis''. There he describes a world, now known as the Poincaré disk, in which space was Euclidean, but which appeared to its inhabitants to satisfy the axioms of hyperbolic geometry:"Suppose, for example, a world enclosed in a large sphere and subject to the following laws: The temperature is not uniform; it is greatest at their centre, and gradually decreases as we move towards the circumference of the sphere, where it isPoincaré's disk was an important piece of evidence for the hypothesis that the choice of spatial geometry is conventional rather than factual, especially in the influential philosophical discussions ofabsolute zero Absolute zero is the lowest limit of the thermodynamic temperature scale, a state at which the enthalpy and entropy of a cooled ideal gas reach their minimum value, taken as zero kelvin. The fundamental particles of nature have minimum vibra .... The law of this temperature is as follows: If be the radius of the sphere, and the distance of the point considered from the centre, the absolute temperature will be proportional to . Further, I shall suppose that in this world all bodies have the same co-efficient of dilatation, so that the linear dilatation of any body is proportional to its absolute temperature. Finally, I shall assume that a body transported from one point to another of different temperature is instantaneously in thermal equilibrium with its new environment. ... If they construct a geometry, it will not be like ours, which is the study of the movements of our invariable solids; it will be the study of the changes of position which they will have thus distinguished, and will be 'non-Euclidean displacements,' and ''this will be non-Euclidean geometry''. So that beings like ourselves, educated in such a world, will not have the same geometry as ours." (pp.65-68)
Rudolf Carnap
Rudolf Carnap (; ; 18 May 1891 – 14 September 1970) was a German-language philosopher who was active in Europe before 1935 and in the United States thereafter. He was a major member of the Vienna Circle and an advocate of logical positivism. ...
and of Hans Reichenbach.
Properties
Lines
orthogonal
In mathematics, orthogonality is the generalization of the geometric notion of '' perpendicularity''.
By extension, orthogonality is also used to refer to the separation of specific features of a system. The term also has specialized meanings in ...
to the boundary of the disk, plus all diameters of the disk.
Compass and straightedge construction
The unique hyperbolic line through two points and not on a diameter of the boundary circle can be constructed by: * let be theinversion
Inversion or inversions may refer to:
Arts
* , a French gay magazine (1924/1925)
* ''Inversion'' (artwork), a 2005 temporary sculpture in Houston, Texas
* Inversion (music), a term with various meanings in music theory and musical set theory
* ...
in the boundary circle of point
* let be the inversion in the boundary circle of point
* let be the midpoint of segment
* let be the midpoint of segment
* Draw line through perpendicular
In elementary geometry, two geometric objects are perpendicular if they intersect at a right angle (90 degrees or π/2 radians). The condition of perpendicularity may be represented graphically using the '' perpendicular symbol'', ⟂. It c ...
to segment
* Draw line through perpendicular to segment
* let be where line and line intersect.
* Draw circle with center and going through (and ).
* The part of circle that is inside the disk is the hyperbolic line.
If P and Q are on a diameter of the boundary circle that diameter is the hyperbolic line.
Another way is:
* let be the midpoint of segment
* Draw line m through perpendicular
In elementary geometry, two geometric objects are perpendicular if they intersect at a right angle (90 degrees or π/2 radians). The condition of perpendicularity may be represented graphically using the '' perpendicular symbol'', ⟂. It c ...
to segment
* let be the inversion
Inversion or inversions may refer to:
Arts
* , a French gay magazine (1924/1925)
* ''Inversion'' (artwork), a 2005 temporary sculpture in Houston, Texas
* Inversion (music), a term with various meanings in music theory and musical set theory
* ...
in the boundary circle of point
* let be the midpoint of segment
* Draw line through perpendicular to segment
* let be where line and line intersect.
* Draw circle with center and going through (and ).
* The part of circle that is inside the disk is the hyperbolic line.
Distance
Distances in this model areCayley–Klein metric
In mathematics, a Cayley–Klein metric is a metric on the complement of a fixed quadric in a projective space which is defined using a cross-ratio. The construction originated with Arthur Cayley's essay "On the theory of distance"Cayley (1859), ...
s.
Given two distinct points ''p'' and ''q'' inside the disk, the unique hyperbolic line connecting them intersects the boundary at two ideal point
In hyperbolic geometry, an ideal point, omega point or point at infinity is a well-defined point outside the hyperbolic plane or space.
Given a line ''l'' and a point ''P'' not on ''l'', right- and left- limiting parallels to ''l'' through '' ...
s, ''a'' and ''b'', label them so that the points are, in order, ''a'', ''p'', ''q'', ''b'' and and .
The hyperbolic distance between ''p'' and ''q'' is then
The vertical bars indicate Euclidean length of the line segment connecting the points between them in the model (not along the circle arc), ln is the natural logarithm
The natural logarithm of a number is its logarithm to the base of the mathematical constant , which is an irrational and transcendental number approximately equal to . The natural logarithm of is generally written as , , or sometimes, if ...
.
Another way to calculate the hyperbolic distance between two points is
where and are the distances of ''p'' respective ''q'' to the centre of the disk, the distance between ''p'' and ''q'', the radius of the boundary circle of the disk and is the inverse hyperbolic function of hyperbolic cosine
In mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle. Just as the points form a circle with a unit radius, the points form the right half of the ...
.
When the disk used is the open unit disk and one of the points is the origin and the Euclidean distance between the points is ''r'' then the hyperbolic distance is:
where is the inverse hyperbolic function of the hyperbolic tangent
In mathematics, hyperbolic functions are analogues of the ordinary trigonometric functions, but defined using the hyperbola rather than the circle. Just as the points form a circle with a unit radius, the points form the right half of the ...
.
When the disk used is the open unit disk and point lies between the origin and point (i.e. the two points are on the same radius, have the same polar angle and ), their hyperbolic distance is
This reduces to the previous formula if .
Circles
Acircle
A circle is a shape consisting of all points in a plane that are at a given distance from a given point, the centre. Equivalently, it is the curve traced out by a point that moves in a plane so that its distance from a given point is cons ...
(the set of all points in a plane that are at a given distance from a given point, its center) is a circle completely inside the disk not touching or intersecting its boundary. The hyperbolic center of the circle in the model does in general not correspond to the Euclidean center of the circle, but they are on the same radius of the boundary circle.
Hypercycles
A hypercycle (the set of all points in a plane that are on one side and at a given distance from a given line, its axis) is a Euclidean circle arc or chord of the boundary circle that intersects the boundary circle at a non-right angle
In geometry and trigonometry, a right angle is an angle of exactly 90 degrees or radians corresponding to a quarter turn. If a ray is placed so that its endpoint is on a line and the adjacent angles are equal, then they are right angles. Th ...
. Its axis is the hyperbolic line that shares the same two ideal point
In hyperbolic geometry, an ideal point, omega point or point at infinity is a well-defined point outside the hyperbolic plane or space.
Given a line ''l'' and a point ''P'' not on ''l'', right- and left- limiting parallels to ''l'' through '' ...
s. This is also known as an equidistant curve.
Horocycles
Ahorocycle
In hyperbolic geometry, a horocycle (), sometimes called an oricycle, oricircle, or limit circle, is a curve whose normal or perpendicular geodesics all converge asymptotically in the same direction. It is the two-dimensional case of a horosph ...
(a curve whose normal or perpendicular
In elementary geometry, two geometric objects are perpendicular if they intersect at a right angle (90 degrees or π/2 radians). The condition of perpendicularity may be represented graphically using the '' perpendicular symbol'', ⟂. It c ...
geodesics all converge asymptotically in the same direction), is a circle inside the disk that touches the boundary circle of the disk. The point where it touches the boundary circle is not part of the horocycle. It is an ideal point
In hyperbolic geometry, an ideal point, omega point or point at infinity is a well-defined point outside the hyperbolic plane or space.
Given a line ''l'' and a point ''P'' not on ''l'', right- and left- limiting parallels to ''l'' through '' ...
and is the hyperbolic center of the horocycle.
Euclidean synopsis
A Euclidean circle: * that is completely inside the disk is a hyperbolic circle. :(When the center of the disk is not inside the circle, the Euclidean center is always closer to the center of the disk than what the hyperbolic center is, i.e. holds.) * that is inside the disk and touches the boundary is a horocycle; * that intersects the boundaryorthogonal
In mathematics, orthogonality is the generalization of the geometric notion of '' perpendicularity''.
By extension, orthogonality is also used to refer to the separation of specific features of a system. The term also has specialized meanings in ...
ly is a hyperbolic line; and
* that intersects the boundary non-orthogonally is a hypercycle.
A Euclidean chord of the boundary circle:
* that goes through the center is a hyperbolic line; and
* that does not go through the center is a hypercycle.
Metric and curvature
 If ''u'' and ''v'' are two vectors in real ''n''-dimensional vector space R''n'' with the usual Euclidean norm, both of which have norm less than 1, then we may define an isometric invariant by
:
where denotes the usual Euclidean norm. Then the distance function is
:
Such a distance function is defined for any two vectors of norm less than one, and makes the set of such vectors into a metric space which is a model of hyperbolic space of constant curvature −1. The model has the conformal property that the angle between two intersecting curves in hyperbolic space is the same as the angle in the model.
The associated
If ''u'' and ''v'' are two vectors in real ''n''-dimensional vector space R''n'' with the usual Euclidean norm, both of which have norm less than 1, then we may define an isometric invariant by
:
where denotes the usual Euclidean norm. Then the distance function is
:
Such a distance function is defined for any two vectors of norm less than one, and makes the set of such vectors into a metric space which is a model of hyperbolic space of constant curvature −1. The model has the conformal property that the angle between two intersecting curves in hyperbolic space is the same as the angle in the model.
The associated metric tensor
In the mathematical field of differential geometry, a metric tensor (or simply metric) is an additional structure on a manifold (such as a surface) that allows defining distances and angles, just as the inner product on a Euclidean space allow ...
of the Poincaré disk model is given by
:
where the ''x''''i'' are the Cartesian coordinates of the ambient Euclidean space. The geodesic
In geometry, a geodesic () is a curve representing in some sense the shortest path ( arc) between two points in a surface, or more generally in a Riemannian manifold. The term also has meaning in any differentiable manifold with a connecti ...
s of the disk model are circles perpendicular to the boundary sphere ''S''''n''−1.
An orthonormal frame with respect to this Riemannian metric is given by
:
with dual coframe of 1-forms
:
In two dimensions
In two dimensions, with respect to these frames and theLevi-Civita connection
In Riemannian or pseudo Riemannian geometry (in particular the Lorentzian geometry of general relativity), the Levi-Civita connection is the unique affine connection on the tangent bundle of a manifold (i.e. affine connection) that preserves ...
, the connection forms are given by the unique skew-symmetric matrix of 1-forms that is torsion-free, i.e., that satisfies the matrix equation . Solving this equation for yields
:
where the curvature matrix is
:
Therefore, the curvature of the hyperbolic disk is
:
Relation to other models of hyperbolic geometry

Relation to the Klein disk model
The Klein disk model (also known as the Beltrami–Klein model) and the Poincaré disk model are both models that project the whole hyperbolic plane in a disk. The two models are related through a projection on or from the hemisphere model. The Klein disk model is anorthographic projection
Orthographic projection (also orthogonal projection and analemma) is a means of representing three-dimensional objects in two dimensions. Orthographic projection is a form of parallel projection in which all the projection lines are orthogona ...
to the hemisphere model while the Poincaré disk model is a stereographic projection
In mathematics, a stereographic projection is a perspective projection of the sphere, through a specific point on the sphere (the ''pole'' or ''center of projection''), onto a plane (the ''projection plane'') perpendicular to the diameter thro ...
.
An advantage of the Klein disk model is that lines in this model are Euclidean straight chords. A disadvantage is that the Klein disk model is not conformal (circles and angles are distorted).
When projecting the same lines in both models on one disk both lines go through the same two ideal point
In hyperbolic geometry, an ideal point, omega point or point at infinity is a well-defined point outside the hyperbolic plane or space.
Given a line ''l'' and a point ''P'' not on ''l'', right- and left- limiting parallels to ''l'' through '' ...
s. (the ideal points remain on the same spot) also the pole of the chord in the Klein disk model is the center of the circle that contains the arc in the Poincaré disk model.
A point (''x'',''y'') in the Poincaré disk model maps to in the Klein model.
A point (''x'',''y'') in the Klein model maps to in the Poincaré disk model.
For ideal points and the formulas become so the points are fixed.
If is a vector of norm less than one representing a point of the Poincaré disk model, then the corresponding point of the Klein disk model is given by:
Conversely, from a vector of norm less than one representing a point of the Beltrami–Klein model, the corresponding point of the Poincaré disk model is given by:
Relation to the Poincaré half-plane model
The Poincaré disk model and the Poincaré half-plane model are both named afterHenri Poincaré
Jules Henri Poincaré ( S: stress final syllable ; 29 April 1854 – 17 July 1912) was a French mathematician, theoretical physicist, engineer, and philosopher of science. He is often described as a polymath, and in mathematics as "Th ...
.
If is a complex number of norm less than one representing a point of the Poincaré disk model, then the corresponding point of the half-plane model is given by the inverse of the Cayley transform:
A point (''x'',''y'') in the disk model maps to in the halfplane model.
A point (''x'',''y'') in the halfplane model maps to in the disk model.
Relation to the hyperboloid model
 The Poincaré disk model, as well as the Klein model, are related to the
The Poincaré disk model, as well as the Klein model, are related to the hyperboloid model
In geometry, the hyperboloid model, also known as the Minkowski model after Hermann Minkowski, is a model of ''n''-dimensional hyperbolic geometry in which points are represented by points on the forward sheet ''S''+ of a two-sheeted hyperbo ...
projectively. If we have a point 't'', ''x''1, ..., ''x''''n''on the upper sheet of the hyperboloid of the hyperboloid model, thereby defining a point in the hyperboloid model, we may project it onto the hyperplane ''t'' = 0 by intersecting it with a line drawn through ��1, 0, ..., 0 The result is the corresponding point of the Poincaré disk model.
For Cartesian coordinates
A Cartesian coordinate system (, ) in a plane is a coordinate system that specifies each point uniquely by a pair of numerical coordinates, which are the signed distances to the point from two fixed perpendicular oriented lines, measured in ...
(''t'', ''xi'') on the hyperboloid and (''yi'') on the plane, the conversion formulas are:
Compare the formulas for stereographic projection
In mathematics, a stereographic projection is a perspective projection of the sphere, through a specific point on the sphere (the ''pole'' or ''center of projection''), onto a plane (the ''projection plane'') perpendicular to the diameter thro ...
between a sphere and a plane.
Analytic geometry constructions in the hyperbolic plane
A basic construction ofanalytic geometry
In classical mathematics, analytic geometry, also known as coordinate geometry or Cartesian geometry, is the study of geometry using a coordinate system. This contrasts with synthetic geometry.
Analytic geometry is used in physics and enginee ...
is to find a line through two given points. In the Poincaré disk model, lines in the plane are defined by portions of circles having equations of the form
:
which is the general form of a circle orthogonal to the unit circle, or else by diameters. Given two points ''u = (u1,u2)'' and ''v = (v1,v2)'' in the disk which do not lie on a diameter, we can solve for the circle of this form passing through both points, and obtain
:
If the points ''u'' and ''v'' are points on the boundary of the disk not lying at the endpoints of a diameter, the above simplifies to
:
Angles
We may compute the angle between the circular arc whose endpoints (''ideal points'') are given by unit vectors ''u'' and ''v'', and the arc whose endpoints are ''s'' and ''t'', by means of a formula. Since the ideal points are the same in the Klein model and the Poincaré disk model, the formulas are identical for each model. If both models' lines are diameters, so that ''v'' = −''u'' and ''t'' = −''s'', then we are merely finding the angle between two unit vectors, and the formula for the angle θ is : If ''v'' = −''u'' but not ''t'' = −''s'', the formula becomes, in terms of thewedge product
A wedge is a triangular shaped tool, and is a portable inclined plane, and one of the six simple machines. It can be used to separate two objects or portions of an object, lift up an object, or hold an object in place. It functions by convert ...
(),
:
where
:
:
:
If both chords are not diameters, the general formula obtains
:
where
:
:
:
Using the Binet–Cauchy identity and the fact that these are unit vectors we may rewrite the above expressions purely in terms of the dot product
In mathematics, the dot product or scalar productThe term ''scalar product'' means literally "product with a scalar as a result". It is also used sometimes for other symmetric bilinear forms, for example in a pseudo-Euclidean space. is an alg ...
, as
:
:
:
Artistic realizations

M. C. Escher
Maurits Cornelis Escher (; 17 June 1898 – 27 March 1972) was a Dutch graphic artist who made mathematically inspired woodcuts, lithographs, and mezzotints.
Despite wide popular interest, Escher was for most of his life neglected in t ...
explored the concept of representing infinity on a two-dimensional plane. Discussions with Canadian mathematician H.S.M. Coxeter around 1956 inspired Escher's interest in hyperbolic tessellations, which are regular tilings of the hyperbolic plane. Escher's wood engravings ''Circle Limit I–IV'' demonstrate this concept between 1958 and 1960, the final one being Circle Limit IV: Heaven and Hell
' in 1960.
/ref> According to Bruno Ernst, the best of them is ''
Circle Limit III
''Circle Limit III'' is a woodcut made in 1959 by Dutch artist M. C. Escher, in which "strings of fish shoot up like rockets from infinitely far away" and then "fall back again whence they came".Escher, as quoted by .
It is one of a series of f ...
''.
See also
* Hyperbolic geometry * Beltrami–Klein model * Poincaré half-plane model * Poincaré metric * Pseudosphere *Hyperboloid model
In geometry, the hyperboloid model, also known as the Minkowski model after Hermann Minkowski, is a model of ''n''-dimensional hyperbolic geometry in which points are represented by points on the forward sheet ''S''+ of a two-sheeted hyperbo ...
* Inversive geometry
Inversive activities are processes which self internalise the action concerned. For example, a person who has an Inversive personality internalises his emotion
Emotions are mental states brought on by neurophysiological changes, variou ...
* Uniform tilings in hyperbolic plane
References
Further reading
* James W. Anderson, ''Hyperbolic Geometry'', second edition, Springer, 2005. * Eugenio Beltrami, ''Teoria fondamentale degli spazii di curvatura costante'', Annali. di Mat., ser II 2 (1868), 232–255. * Saul Stahl, ''The Poincaré Half-Plane'', Jones and Bartlett, 1993.External links
* {{Commons category-inline, Poincaré disk models Multi-dimensional geometry Hyperbolic geometry Disk