|
Heteroclinic Orbit
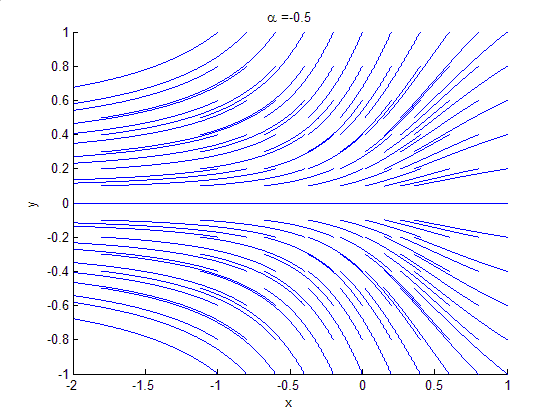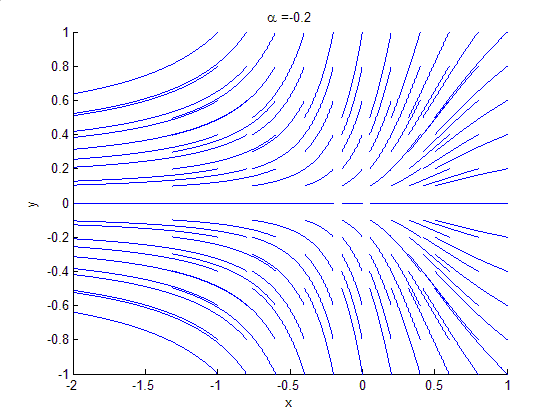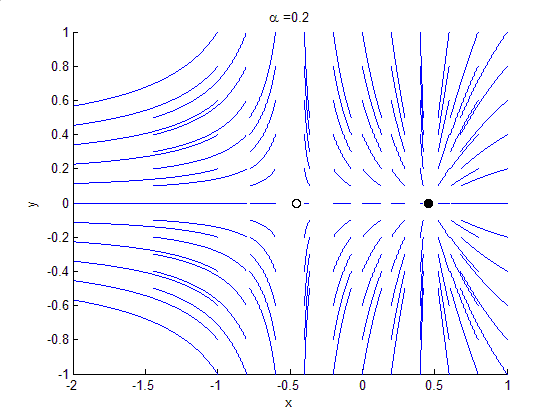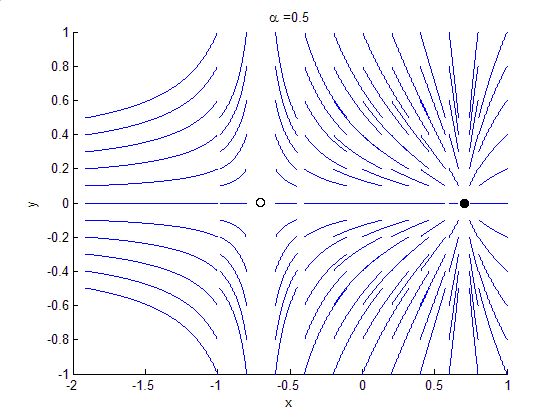
In mathematics, in the phase portrait of a dynamical system, a heteroclinic orbit (sometimes called a heteroclinic connection) is a path in phase space which joins two different equilibrium points. If the equilibrium points at the start and end of the orbit are the same, the orbit is a homoclinic orbit. Consider the continuous dynamical system described by the ODE ::\dot x=f(x) Suppose there are equilibria at x=x_0 and x=x_1, then a solution \phi(t) is a heteroclinic orbit from x_0 to x_1 if ::\phi(t)\rightarrow x_0\quad \mathrm\quad t\rightarrow-\infty and ::\phi(t)\rightarrow x_1\quad \mathrm\quad t\rightarrow+\infty This implies that the orbit is contained in the stable manifold of x_1 and the unstable manifold of x_0. Symbolic dynamics By using the Markov partition, the long-time behaviour of hyperbolic system can be studied using the techniques of symbolic dynamics. In this case, a heteroclinic orbit has a particularly simple and clear representation. Suppose that S=\ is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heteroclinic Orbit In Pendulum Phaseportrait
In mathematics, in the phase portrait of a dynamical system, a heteroclinic orbit (sometimes called a heteroclinic connection) is a path in phase space which joins two different equilibrium points. If the equilibrium points at the start and end of the orbit are the same, the orbit is a homoclinic orbit. Consider the continuous dynamical system described by the ODE ::\dot x=f(x) Suppose there are equilibria at x=x_0 and x=x_1, then a solution \phi(t) is a heteroclinic orbit from x_0 to x_1 if ::\phi(t)\rightarrow x_0\quad \mathrm\quad t\rightarrow-\infty and ::\phi(t)\rightarrow x_1\quad \mathrm\quad t\rightarrow+\infty This implies that the orbit is contained in the stable manifold of x_1 and the unstable manifold of x_0. Symbolic dynamics By using the Markov partition, the long-time behaviour of hyperbolic system can be studied using the techniques of symbolic dynamics. In this case, a heteroclinic orbit has a particularly simple and clear representation. Suppose that S=\ is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Symbolic Dynamics
In mathematics, symbolic dynamics is the practice of modeling a topological or smooth dynamical system by a discrete space consisting of infinite sequences of abstract symbols, each of which corresponds to a state of the system, with the dynamics (evolution) given by the shift operator. Formally, a Markov partition is used to provide a finite cover for the smooth system; each set of the cover is associated with a single symbol, and the sequences of symbols result as a trajectory of the system moves from one covering set to another. History The idea goes back to Jacques Hadamard's 1898 paper on the geodesics on surfaces of negative curvature. It was applied by Marston Morse in 1921 to the construction of a nonperiodic recurrent geodesic. Related work was done by Emil Artin in 1924 (for the system now called Artin billiard), Pekka Myrberg, Paul Koebe, Jakob Nielsen, G. A. Hedlund. The first formal treatment was developed by Morse and Hedlund in their 1938 paper. George Birkh ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
John Guckenheimer
John Mark Guckenheimer (born 1945) joined the Department of Mathematics at Cornell University in 1985. He was previously at the University of California, Santa Cruz (1973-1985). He was a Guggenheim fellow in 1984, and was elected president of the Society for Industrial and Applied Mathematics (SIAM), serving from 1997 to 1998. Guckenheimer received his A.B. in 1966 from Harvard and his Ph.D. in 1970 from Berkeley, where his Ph.D. thesis advisor was Stephen Smale. His book ''Nonlinear Oscillations, Dynamical Systems and Bifurcation of Vector Fields'' (with Philip Holmes) is an extensively cited work on dynamical systems. Research Dr. John Guckenheimer's research has focused on three areas — neuroscience, algorithms for periodic orbits, and dynamics in systems with multiple time scales. Neuroscience Guckenheimer studies dynamical models of a small neural system, the stomatogastric ganglion of crustaceans — attempting to learn more about neuromodulation, the ways in whic ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Traveling Wave
In physics, mathematics, and related fields, a wave is a propagating dynamic disturbance (change from equilibrium) of one or more quantities. Waves can be periodic, in which case those quantities oscillate repeatedly about an equilibrium (resting) value at some frequency. When the entire waveform moves in one direction, it is said to be a ''traveling wave''; by contrast, a pair of superimposed periodic waves traveling in opposite directions makes a ''standing wave''. In a standing wave, the amplitude of vibration has nulls at some positions where the wave amplitude appears smaller or even zero. Waves are often described by a ''wave equation'' (standing wave field of two opposite waves) or a one-way wave equation for single wave propagation in a defined direction. Two types of waves are most commonly studied in classical physics. In a '' mechanical wave'', stress and strain fields oscillate about a mechanical equilibrium. A mechanical wave is a local deformation (strain) i ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Homoclinic Orbit
In mathematics, a homoclinic orbit is a trajectory of a flow of a dynamical system which joins a saddle equilibrium point to itself. More precisely, a homoclinic orbit lies in the intersection of the stable manifold and the unstable manifold of an equilibrium. Consider the continuous dynamical system described by the ODE :\dot x=f(x) Suppose there is an equilibrium at x=x_0, then a solution \Phi(t) is a homoclinic orbit if :\Phi(t)\rightarrow x_0\quad \mathrm\quad t\rightarrow\pm\infty If the phase space has three or more dimensions, then it is important to consider the topology of the unstable manifold of the saddle point. The figures show two cases. First, when the stable manifold is topologically a cylinder, and secondly, when the unstable manifold is topologically a Möbius strip; in this case the homoclinic orbit is called ''twisted''. Discrete dynamical system Homoclinic orbits and homoclinic points are defined in the same way for iterated functions, as the inte ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heteroclinic Bifurcation
Bifurcation theory is the mathematical study of changes in the qualitative or topological structure of a given family of curves, such as the integral curves of a family of vector fields, and the solutions of a family of differential equations. Most commonly applied to the mathematical study of dynamical systems, a bifurcation occurs when a small smooth change made to the parameter values (the bifurcation parameters) of a system causes a sudden 'qualitative' or topological change in its behavior. Bifurcations occur in both continuous systems (described by ordinary, delay or partial differential equations) and discrete systems (described by maps). The name "bifurcation" was first introduced by Henri Poincaré in 1885 in the first paper in mathematics showing such a behavior. Henri Poincaré also later named various types of stationary points and classified them . Bifurcation types It is useful to divide bifurcations into two principal classes: * Local bifurcations, which can be ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heteroclinic Cycle
In mathematics, a heteroclinic cycle is an invariant set in the phase space of a dynamical system. It is a topological circle of equilibrium points and connecting heteroclinic orbits. If a heteroclinic cycle is asymptotically stable, approaching trajectories spend longer and longer periods of time in a neighbourhood of successive equilibria. In generic dynamical systems heteroclinic connections are of high co-dimension, that is, they will not persist if parameters are varied. Robust heteroclinic cycles A robust heteroclinic cycle is one which persists under small changes in the underlying dynamical system. Robust cycles often arise in the presence of symmetry or other constraints which force the existence of invariant hyperplanes. A prototypical example of a robust heteroclinic cycle is the Guckenheimer–Holmes cycle. This cycle has also been studied in the context of rotating convection, and as three competing species in population dynamics. See also * Heteroclinic b ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Heteroclinic Connection
{{unreferenced, date=December 2010 In dynamical systems, a branch of mathematics, a structure formed from the stable manifold and unstable manifold of a fixed point. Definition for maps Let f:M\to M be a map defined on a manifold M, with a fixed point p. Let W^s(f,p) and W^u(f,p) be the stable manifold and the unstable manifold of the fixed point p, respectively. Let V be a connected invariant manifold such that : V\subseteq W^s(f,p)\cap W^u(f,p) Then V is called a homoclinic connection. Heteroclinic connection It is a similar notion, but it refers to two fixed points, p and q. The condition satisfied by V is replaced with: :V\subseteq W^s(f,p)\cap W^u(f,q) This notion is not symmetric with respect to p and q. Homoclinic and heteroclinic intersections When the invariant manifolds W^s(f,p) and W^u(f,q), possibly with p=q, intersect but there is no homoclinic/heteroclinic connection, a different structure is formed by the two manifolds, sometimes referred to as the homo ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Periodic Point
In mathematics, in the study of iterated functions and dynamical systems, a periodic point of a function is a point which the system returns to after a certain number of function iterations or a certain amount of time. Iterated functions Given a mapping ''f'' from a set ''X'' into itself, :f: X \to X, a point ''x'' in ''X'' is called periodic point if there exists an ''n'' so that :\ f_n(x) = x where f_n is the ''n''th iterate of ''f''. The smallest positive integer ''n'' satisfying the above is called the ''prime period'' or ''least period'' of the point ''x''. If every point in ''X'' is a periodic point with the same period ''n'', then ''f'' is called ''periodic'' with period ''n'' (this is not to be confused with the notion of a periodic function). If there exist distinct ''n'' and ''m'' such that :f_n(x) = f_m(x) then ''x'' is called a preperiodic point. All periodic points are preperiodic. If ''f'' is a diffeomorphism of a differentiable manifold, so that the derivative f_ ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Bi-infinite String
In mathematics, a sequence is an enumerated collection of objects in which repetitions are allowed and order matters. Like a set, it contains members (also called ''elements'', or ''terms''). The number of elements (possibly infinite) is called the ''length'' of the sequence. Unlike a set, the same elements can appear multiple times at different positions in a sequence, and unlike a set, the order does matter. Formally, a sequence can be defined as a function from natural numbers (the positions of elements in the sequence) to the elements at each position. The notion of a sequence can be generalized to an indexed family, defined as a function from an ''arbitrary'' index set. For example, (M, A, R, Y) is a sequence of letters with the letter 'M' first and 'Y' last. This sequence differs from (A, R, M, Y). Also, the sequence (1, 1, 2, 3, 5, 8), which contains the number 1 at two different positions, is a valid sequence. Sequences can be ''finite'', as in these examples, or ''infinit ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Finite Set
In mathematics, particularly set theory, a finite set is a set that has a finite number of elements. Informally, a finite set is a set which one could in principle count and finish counting. For example, :\ is a finite set with five elements. The number of elements of a finite set is a natural number (possibly zero) and is called the ''cardinality (or the cardinal number)'' of the set. A set that is not a finite set is called an '' infinite set''. For example, the set of all positive integers is infinite: :\. Finite sets are particularly important in combinatorics, the mathematical study of counting. Many arguments involving finite sets rely on the pigeonhole principle, which states that there cannot exist an injective function from a larger finite set to a smaller finite set. Definition and terminology Formally, a set is called finite if there exists a bijection :f\colon S\to\ for some natural number . The number is the set's cardinality, denoted as . The empty set o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Hyperbolic System
In mathematics, a hyperbolic partial differential equation of order n is a partial differential equation (PDE) that, roughly speaking, has a well-posed initial value problem for the first n-1 derivatives. More precisely, the Cauchy problem can be locally solved for arbitrary initial data along any non-characteristic hypersurface. Many of the equations of mechanics are hyperbolic, and so the study of hyperbolic equations is of substantial contemporary interest. The model hyperbolic equation is the wave equation. In one spatial dimension, this is : \frac = c^2 \frac The equation has the property that, if ''u'' and its first time derivative are arbitrarily specified initial data on the line (with sufficient smoothness properties), then there exists a solution for all time ''t''. The solutions of hyperbolic equations are "wave-like". If a disturbance is made in the initial data of a hyperbolic differential equation, then not every point of space feels the disturbance at once. Rel ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |