|
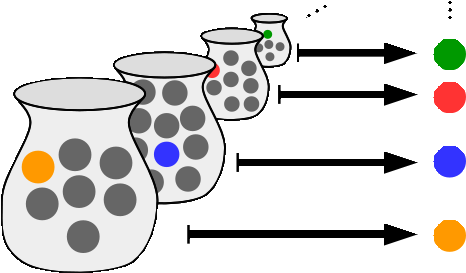
Axiom Of Choice
In mathematics, the axiom of choice, or AC, is an axiom of set theory equivalent to the statement that ''a Cartesian product of a collection of non-empty sets is non-empty''. Informally put, the axiom of choice says that given any collection of sets, each containing at least one element, it is possible to construct a new set by arbitrarily choosing one element from each set, even if the collection is infinite. Formally, it states that for every indexed family (S_i)_ of nonempty sets, there exists an indexed set (x_i)_ such that x_i \in S_i for every i \in I. The axiom of choice was formulated in 1904 by Ernst Zermelo in order to formalize his proof of the well-ordering theorem. In many cases, a set arising from choosing elements arbitrarily can be made without invoking the axiom of choice; this is, in particular, the case if the number of sets from which to choose the elements is finite, or if a canonical rule on how to choose the elements is available – some distinguis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Axiom Of Choice
In mathematics, the axiom of choice, or AC, is an axiom of set theory equivalent to the statement that ''a Cartesian product of a collection of non-empty sets is non-empty''. Informally put, the axiom of choice says that given any collection of sets, each containing at least one element, it is possible to construct a new set by arbitrarily choosing one element from each set, even if the collection is infinite. Formally, it states that for every indexed family (S_i)_ of nonempty sets, there exists an indexed set (x_i)_ such that x_i \in S_i for every i \in I. The axiom of choice was formulated in 1904 by Ernst Zermelo in order to formalize his proof of the well-ordering theorem. In many cases, a set arising from choosing elements arbitrarily can be made without invoking the axiom of choice; this is, in particular, the case if the number of sets from which to choose the elements is finite, or if a canonical rule on how to choose the elements is available – some distinguis ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Non-measurable Set
In mathematics, a non-measurable set is a set which cannot be assigned a meaningful "volume". The mathematical existence of such sets is construed to provide information about the notions of length, area and volume in formal set theory. In Zermelo–Fraenkel set theory, the axiom of choice entails that non-measurable subsets of \mathbb exist. The notion of a non-measurable set has been a source of great controversy since its introduction. Historically, this led Borel and Kolmogorov to formulate probability theory on sets which are constrained to be measurable. The measurable sets on the line are iterated countable unions and intersections of intervals (called Borel sets) plus-minus null sets. These sets are rich enough to include every conceivable definition of a set that arises in standard mathematics, but they require a lot of formalism to prove that sets are measurable. In 1970, Robert M. Solovay constructed the Solovay model, which shows that it is consistent with standar ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Interval (mathematics)
In mathematics, a (real) interval is a set of real numbers that contains all real numbers lying between any two numbers of the set. For example, the set of numbers satisfying is an interval which contains , , and all numbers in between. Other examples of intervals are the set of numbers such that , the set of all real numbers \R, the set of nonnegative real numbers, the set of positive real numbers, the empty set, and any singleton (set of one element). Real intervals play an important role in the theory of integration, because they are the simplest sets whose "length" (or "measure" or "size") is easy to define. The concept of measure can then be extended to more complicated sets of real numbers, leading to the Borel measure and eventually to the Lebesgue measure. Intervals are central to interval arithmetic, a general numerical computing technique that automatically provides guaranteed enclosures for arbitrary formulas, even in the presence of uncertainties, mathematical ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Real Number
In mathematics, a real number is a number that can be used to measure a ''continuous'' one-dimensional quantity such as a distance, duration or temperature. Here, ''continuous'' means that values can have arbitrarily small variations. Every real number can be almost uniquely represented by an infinite decimal expansion. The real numbers are fundamental in calculus (and more generally in all mathematics), in particular by their role in the classical definitions of limits, continuity and derivatives. The set of real numbers is denoted or \mathbb and is sometimes called "the reals". The adjective ''real'' in this context was introduced in the 17th century by René Descartes to distinguish real numbers, associated with physical reality, from imaginary numbers (such as the square roots of ), which seemed like a theoretical contrivance unrelated to physical reality. The real numbers include the rational numbers, such as the integer and the fraction . The rest of the rea ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Axiom Of Dependent Choice
In mathematics, the axiom of dependent choice, denoted by \mathsf , is a weak form of the axiom of choice ( \mathsf ) that is still sufficient to develop most of real analysis. It was introduced by Paul Bernays in a 1942 article that explores which set-theoretic axioms are needed to develop analysis."The foundation of analysis does not require the full generality of set theory but can be accomplished within a more restricted frame." The axiom of dependent choice is stated on p. 86. Formal statement A homogeneous relation R on X is called a total relation if for every a \in X, there exists some b \in X such that a\,R~b is true. The axiom of dependent choice can be stated as follows: For every nonempty set X and every total relation R on X, there exists a sequence (x_n)_ in X such that :x_n\, R~x_ for all n \in \N. ''x''0 may be taken to be any desired element of ''X''. If the set X above is restricted to be the set of all real numbers, then the resulting axiom is ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Axiom Of Countable Choice
The axiom of countable choice or axiom of denumerable choice, denoted ACω, is an axiom of set theory that states that every countable collection of non-empty sets must have a choice function. That is, given a function ''A'' with domain N (where N denotes the set of natural numbers) such that ''A''(''n'') is a non-empty set for every ''n'' ∈ N, there exists a function ''f'' with domain N such that ''f''(''n'') ∈ ''A''(''n'') for every ''n'' ∈ N. Overview The axiom of countable choice (ACω) is strictly weaker than the axiom of dependent choice (DC), which in turn is weaker than the axiom of choice (AC). Paul Cohen showed that ACω is not provable in Zermelo–Fraenkel set theory (ZF) without the axiom of choice . ACω holds in the Solovay model. ZF+ACω suffices to prove that the union of countably many countable sets is countable. It also suffices to prove that every infinite set is Dedekind-infinite (equivalently: has a countably infinite ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Zermelo
Ernst Friedrich Ferdinand Zermelo (, ; 27 July 187121 May 1953) was a German logician and mathematician, whose work has major implications for the foundations of mathematics. He is known for his role in developing Zermelo–Fraenkel axiomatic set theory and his proof of the well-ordering theorem. Furthermore, his 1929 work on ranking chess players is the first description of a model for pairwise comparison that continues to have a profound impact on various applied fields utilizing this method. Life Ernst Zermelo graduated from Berlin's Luisenstädtisches Gymnasium (now ) in 1889. He then studied mathematics, physics and philosophy at the University of Berlin, the University of Halle, and the University of Freiburg. He finished his doctorate in 1894 at the University of Berlin, awarded for a dissertation on the calculus of variations (''Untersuchungen zur Variationsrechnung''). Zermelo remained at the University of Berlin, where he was appointed assistant to Planck, under whose ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Mathematical Induction
Mathematical induction is a method for proving that a statement ''P''(''n'') is true for every natural number ''n'', that is, that the infinitely many cases ''P''(0), ''P''(1), ''P''(2), ''P''(3), ... all hold. Informal metaphors help to explain this technique, such as falling dominoes or climbing a ladder: A proof by induction consists of two cases. The first, the base case, proves the statement for ''n'' = 0 without assuming any knowledge of other cases. The second case, the induction step, proves that ''if'' the statement holds for any given case ''n'' = ''k'', ''then'' it must also hold for the next case ''n'' = ''k'' + 1. These two steps establish that the statement holds for every natural number ''n''. The base case does not necessarily begin with ''n'' = 0, but often with ''n'' = 1, and possibly with any fixed natural number ''n'' = ''N'', establishing the truth of the statement for all natu ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Finite Set
In mathematics, particularly set theory, a finite set is a set that has a finite number of elements. Informally, a finite set is a set which one could in principle count and finish counting. For example, :\ is a finite set with five elements. The number of elements of a finite set is a natural number (possibly zero) and is called the ''cardinality (or the cardinal number)'' of the set. A set that is not a finite set is called an '' infinite set''. For example, the set of all positive integers is infinite: :\. Finite sets are particularly important in combinatorics, the mathematical study of counting. Many arguments involving finite sets rely on the pigeonhole principle, which states that there cannot exist an injective function from a larger finite set to a smaller finite set. Definition and terminology Formally, a set is called finite if there exists a bijection :f\colon S\to\ for some natural number . The number is the set's cardinality, denoted as . The empty set o ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Power Set
In mathematics, the power set (or powerset) of a set is the set of all subsets of , including the empty set and itself. In axiomatic set theory (as developed, for example, in the ZFC axioms), the existence of the power set of any set is postulated by the axiom of power set. The powerset of is variously denoted as , , , \mathbb(S), or . The notation , meaning the set of all functions from S to a given set of two elements (e.g., ), is used because the powerset of can be identified with, equivalent to, or bijective to the set of all the functions from to the given two elements set. Any subset of is called a ''family of sets'' over . Example If is the set , then all the subsets of are * (also denoted \varnothing or \empty, the empty set or the null set) * * * * * * * and hence the power set of is . Properties If is a finite set with the cardinality (i.e., the number of all elements in the set is ), then the number of all the subsets of is . This fact as we ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |
Partition Of A Set
In mathematics, a partition of a set is a grouping of its elements into non-empty subsets, in such a way that every element is included in exactly one subset. Every equivalence relation on a set defines a partition of this set, and every partition defines an equivalence relation. A set equipped with an equivalence relation or a partition is sometimes called a setoid, typically in type theory and proof theory. Definition and Notation A partition of a set ''X'' is a set of non-empty subsets of ''X'' such that every element ''x'' in ''X'' is in exactly one of these subsets (i.e., ''X'' is a disjoint union of the subsets). Equivalently, a family of sets ''P'' is a partition of ''X'' if and only if all of the following conditions hold: *The family ''P'' does not contain the empty set (that is \emptyset \notin P). *The union of the sets in ''P'' is equal to ''X'' (that is \textstyle\bigcup_ A = X). The sets in ''P'' are said to exhaust or cover ''X''. See also collectively ... [...More Info...] [...Related Items...] OR: [Wikipedia] [Google] [Baidu] |